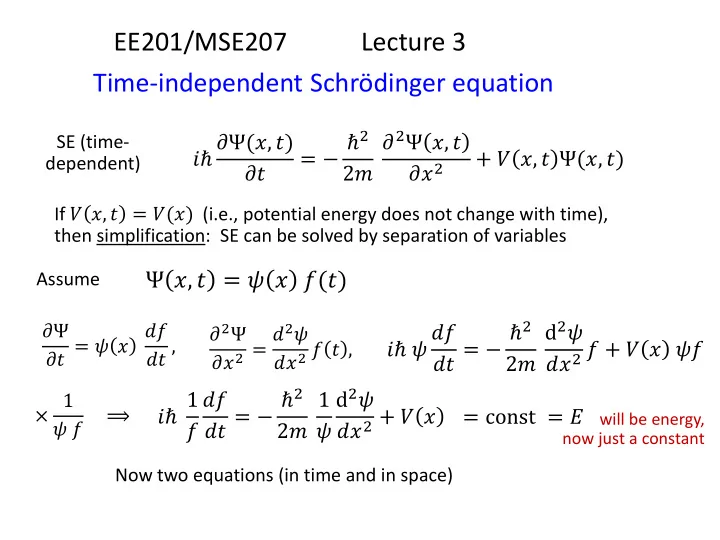
EE201/MSE207 Lecture 3 Time-independent Schrdinger equation = 2 - PowerPoint PPT Presentation

EE201/MSE207 Lecture 3 Time-independent Schrödinger equation = − ℏ 2 𝜖 2 Ψ 𝑦, 𝑢 𝑗ℏ 𝜖Ψ(𝑦, 𝑢) SE (time- + 𝑊 𝑦, 𝑢 Ψ(𝑦, 𝑢) dependent) 𝜖𝑦 2 𝜖𝑢 2𝑛 If 𝑊 𝑦, 𝑢 = 𝑊(𝑦) (i.e., potential energy does not change with time), then simplification: SE can be solved by separation of variables Ψ 𝑦, 𝑢 = 𝜔 𝑦 𝑔(𝑢) Assume 𝑒𝑢 = − ℏ 2 d 2 𝜔 𝜖Ψ 𝑒𝑔 𝑗ℏ 𝜔 𝑒𝑔 𝜖 2 Ψ 𝜖𝑦 2 = 𝑒 2 𝜔 𝜖𝑢 = 𝜔 𝑦 𝑒𝑢 , 𝑒𝑦 2 𝑔 + 𝑊 𝑦 𝜔𝑔 𝑒𝑦 2 𝑔 𝑢 , 2𝑛 𝑒𝑢 = − ℏ 2 d 2 𝜔 𝑗ℏ 1 𝑒𝑔 1 × 1 ⟹ 𝑒𝑦 2 + 𝑊 𝑦 = const = 𝐹 will be energy, 𝜔 𝑔 𝑔 2𝑛 𝜔 now just a constant Now two equations (in time and in space)

𝑒𝑢 = − ℏ 2 d 2 𝜔 𝑗ℏ 1 𝑒𝑔 1 Ψ 𝑦, 𝑢 = 𝜔 𝑦 𝑔(𝑢) 𝑒𝑦 2 + 𝑊 𝑦 = 𝐹 𝑔 2𝑛 𝜔 −𝑗𝐹 𝑒𝑔 𝑒𝑢 = −𝑗 𝐹 Can choose 𝐷 = 1 ℏ 𝑢 𝑔 𝑢 = 𝐷 𝑓 First equation: ℏ 𝑔 ⟹ 𝐹 must be real (for normalization) 𝑔 𝑢 = exp −𝑗 𝐹 ℏ 𝑢 − ℏ 2 d 2 𝜔 𝑦 Second 𝐼𝜔 = 𝐹 𝜔 + 𝑊 𝑦 𝜔 𝑦 = 𝐹 𝜔 𝑦 equation: 𝑒𝑦 2 2𝑛 (this hints that Time-independent Schrödinger equation (TISE) E is energy) Ψ 𝑦, 𝑢 = 𝜔 𝑦 exp −𝑗 𝐹 ℏ 𝑢 ∞ exp −𝑗 𝐹 Ψ 2 𝑒𝑦 = 1, Normalization ℏ 𝑢 = 1 while −∞ ∞ 𝜔 normalized in 𝜔(𝑦) 2 𝑒𝑦 = 1 therefore the same way as Ψ −∞

Stationary state Ψ 𝑦, 𝑢 = 𝜔 𝑦 exp −𝑗 𝐹 ℏ 𝑢 For any observable 𝑅(𝑦, 𝑞) , the average 𝑅(𝑦, −𝑗ℏ 𝜖 𝜖𝑦) does not depend on time ⇒ stationary state ∞ 𝜔 𝑦 𝑓 −𝑗𝐹𝑢 ℏ ∗ 𝑅 𝑦, −𝑗ℏ 𝜖 −𝑗𝐹𝑢 ℏ ] 𝑒𝑦 𝑅 = 𝜖𝑦 [𝜔 𝑦 𝑓 time-dependence −∞ cancels Let us show that E is energy ∞ 𝜔 ∗ ∞ 𝜔 ∗ 𝐹𝜔 𝑒𝑦 = 𝐼𝜔 = 𝐹𝜔 𝐼 = 𝐼𝜔 𝑒𝑦 = Average energy: −∞ −∞ ∞ 𝜔 2 𝑒𝑦 = 𝐹 ( H is operator of total energy) = 𝐹 −∞ ∞ 𝜔 ∗ ∞ 𝜔 ∗ 𝐹𝐹𝜔 𝑒𝑦 = 𝐹 2 𝐼 2 = 𝐼 𝐼𝜔 𝑒𝑦 = Similarly −∞ −∞ No variance ⟹ energy is E (definite energy, “well - defined” energy, rare case in QM)

General solution of SE (for time-independent 𝑊(𝑦) ) TISE solutions (infinitely many): − ℏ 2 d 2 𝜔 𝑜 𝑒𝑦 2 + 𝑊(𝑦) 𝜔 𝑜 = 𝐹 𝑜 𝜔 𝑜 𝑜 = 1, 2, 3, … 2𝑛 SE is linear ⟹ any linear combination of solutions is a solution ∞ 𝑑 𝑜 𝜔 𝑜 𝑦 exp −𝑗 𝐹 𝑜 Ψ 𝑦, 𝑢 = ℏ 𝑢 𝑜=1 Any complex coefficients 𝑑 𝑜 ; however, Ψ should be normalized. As we will see, with normalized 𝜔 𝑜 , this requires normalization for 𝑑 𝑜 as ∞ 𝑑 𝑜 2 = 1 𝑜=1

Orthogonality of stationary states Stationary states 𝜔 𝑜 (𝑦) and 𝜔 𝑛 𝑦 with different energies, Theorem ∞ 𝜔 𝑛 ∗ 𝑦 𝜔 𝑜 𝑦 𝑒𝑦 = 0. 𝐹 𝑜 ≠ 𝐹 𝑛 , are orthogonal: −∞ Remarks: - orthonormal (since normalized) - we treat functions as vectors, inner product (generalized dot-product) ∞ 𝑔 ∗ 𝑦 𝑕 𝑦 𝑒𝑦 , orthogonal: 𝑔 𝑕 = 0 𝑔 𝑕 ≡ −∞ Start with − ℏ 2 d 2 𝜔 𝑜 𝑒𝑦 2 + 𝑊 𝜔 𝑜 = 𝐹 𝑜 𝜔 𝑜 Proof 2𝑛 ∗ , integrate: − ℏ 2 ∗ d 2 𝜔 𝑜 ∗ 𝑊𝜔 𝑜 𝑒𝑦 = 𝐹 𝑜 𝜔 𝑛 ∗ 𝜔 𝑜 𝑒𝑦 2𝑛 𝜔 𝑛 𝑒𝑦 2 𝑒𝑦 + 𝜔 𝑛 × 𝜔 𝑛 ⇒ also equal equal equal (by parts) exchange 𝑜 ↔ 𝑛, − ℏ 2 d 2 𝜔 𝑛 ∗ ∗ 𝑒𝑦 = 𝐹 𝑛 𝜔 𝑜 𝜔 𝑛 ∗ 𝑒𝑦 2𝑛 𝜔 𝑜 𝑒𝑦 2 𝑒𝑦 + 𝜔 𝑜 𝑊𝜔 𝑛 conjugate: ∗ 𝜔 𝑜 𝑒𝑦 = 0 ∗ 𝜔 𝑜 𝑒𝑦 = 0 (𝐹 𝑜 −𝐹 𝑛 ) 𝜔 𝑛 If 𝐹 𝑜 ≠ 𝐹 𝑛 , then 𝜔 𝑛 Q.E.D. If energies are the same, usually also choose orthogonal functions 𝜔 𝑜 (𝑦)

Normalization for coefficients 𝑑 𝑜 ∞ 𝑑 𝑜 𝜔 𝑜 𝑦 exp −𝑗 𝐹 𝑜 Use orthogonality of 𝜔 𝑜 Ψ 𝑦, 𝑢 = ℏ 𝑢 only n = m 𝑜=1 ∞ ∞ ∗ 𝑑 𝑜 exp −𝑗 𝐹 𝑜 − 𝐹 𝑛 ∗ 𝑦 𝜔 𝑜 𝑦 𝑒𝑦 Ψ(𝑦, 𝑢) 2 𝑒𝑦 = 1 = 𝑑 𝑛 𝑢 𝜔 𝑛 ℏ −∞ −∞ 𝑜,𝑛 ∞ 𝑑 𝑜 2 × 1 × 2 𝑒𝑦 = 𝑑 𝑜 2 = 𝜔 𝑜 𝑦 𝑜 −∞ 𝑜 This is how we obtain normalization for 𝑑 𝑜 : ∞ 𝑑 𝑜 2 = 1 𝑜=1

General solution for evolution of Ψ(𝑦, 𝑢) Suppose we know wave function at 𝑢 = 0 : Ψ(𝑦, 0) Then we can find coefficients 𝑑 𝑜 in Ψ 𝑦, 0 = 𝑜 𝑑 𝑜 𝜔 𝑜 𝑦 ∗ (𝑦) and integrate Again use orthogonality: multiply by 𝜔 𝑛 ∗ 𝑦 Ψ 𝑦, 0 𝑒𝑦 = 𝑜 𝑑 𝑜 𝜔 𝑛 ∗ 𝑦 𝜔 𝑜 𝑦 𝑒𝑦 = 𝑑 𝑛 𝜔 𝑛 𝜀 𝑜𝑛 ∞ 𝜔 𝑜 ∗ 𝑦 Ψ 𝑦, 0 𝑒𝑦 𝑑 𝑜 = Therefore −∞ Then we can find Ψ(𝑦, 𝑢) at any time 𝑢 : ∞ 𝑑 𝑜 𝜔 𝑜 𝑦 exp −𝑗 𝐹 𝑜 Ψ 𝑦, 𝑢 = ℏ 𝑢 𝑜=1

Example: Infinite square well 𝑊(𝑦) (one of the most important systems in this course) 𝑊 𝑦 = 0, 0 ≤ 𝑦 ≤ 𝑏 ∞, otherwise 0 x (similar to quantum well in semiconductors, a except different effective mass and finite depth) Solve TISE inside − ℏ 2 d 2 𝜔 𝑦 = 𝐹 𝜔 𝑦 ⇒ 𝜔 𝑦 = 𝐵 sin 𝑙𝑦 + 𝐶 cos(𝑙𝑦) 𝑒𝑦 2 2𝑛 2𝑛𝐹 𝜔 0 = 0 𝑙 = Boundary ℏ conditions: 𝜔 𝑏 = 0 (because 𝜔 𝑦 = 0 outside and 𝜔(𝑦) is always continuous) 𝑙 𝑜 = 𝜌𝑜 𝜔 𝑦 = 𝐵 sin 𝑙 𝑜 𝑦 therefore 𝑏 , 𝑜 = 1, 2, 3, … 𝐹 𝑜 = ℏ 2 𝑙 𝑜 2𝑛 = 𝑜 2 𝜌 2 ℏ 2 2 2𝑛𝑏 2

Infinite square well 𝑊(𝑦) 𝑙 𝑜 = 𝑜𝜌 𝑜 = 1, 2, 3, … 𝜔 𝑜 𝑦 = 𝐵 sin 𝑙 𝑜 𝑦 , 𝑏 𝐹 𝑜 = ℏ 2 𝑙 𝑜 2𝑛 = 𝑜 2 𝜌 2 ℏ 2 2 2𝑛𝑏 2 0 x a 𝑏 𝜔 𝑦 2 𝑒𝑦 = 𝐵 2 1 1 = 2 𝑏 ⇒ 𝐵 = 2 𝑏 Normalization 0 (because sin 2 (𝑦) = 1/2) 𝑏 sin 𝑜𝜌 2 𝜔 𝑜 𝑦 = 𝑏 𝑦 𝜔 𝑜 (𝑦) n =1  Similar to atom states 1 n =1 – lowest energy,   Even lowest state has “ground state”    n  2 – “excited states” 0 a positive energy (understanding    0 n =2 n =3  from uncertainty principle) 

Infinite square well: Summary 𝑊(𝑦) 𝑜 = 1, 2, 3, … 2 𝑏 sin 𝑜𝜌 𝜔 𝑜 𝑦 = 𝑏 𝑦 𝑙 𝑜 = 𝑜𝜌 0 x a 𝑏 𝐹 𝑜 = 𝑜 2 𝜌 2 ℏ 2 2𝑛𝑏 2 n =3 𝜔 𝑜 (𝑦)   𝐹 3     4 n =1     9 n =2 1 n =1 – lowest energy,   𝐹 2   “ground state”    n =1   n  2 – “excited states” 0 a    0 𝐹 1   n =2 n =3   

Infinite square well: properties of stationary states 𝑊(𝑦) 𝑏 sin 𝑜𝜌 2 𝜔 𝑜 𝑦 = 𝑏 𝑦 𝑜 = 1, 2, 3, …  orthogonal to each other, 0 x a 𝑏 𝜔 𝑛 ∗ 𝑦 𝜔 𝑜 (𝑦) 𝑒𝑦 = 𝜀 𝑛𝑜 0 𝜔 𝑜 (𝑦)  complete set, any 𝑔 𝑦 can be represented as n =1 ∞ 𝑔 𝑦 = 𝑜=1 𝑑 𝑜 𝜔 𝑜 𝑦 if 𝑔 0 = 𝑔 𝑏 = 0 1  (i.e., orthonormal basis)    0 a    0 How to find 𝑑 𝑜 ? Already know the trick: n =2 n =3  ∗ 𝑦 𝑔(𝑦) 𝑒𝑦 = 𝑜 𝑑 𝑜 𝜔 𝑛 ∗ 𝑦 𝜔 𝑜 𝑦 𝑒𝑦 = 𝑑 𝑛 𝜔 𝑛  𝑏 ∗ 𝑦 𝑔(𝑦) 𝑒𝑦 𝑑 𝑜 = 𝜔 𝑜 Therefore 0

General solution 𝑊(𝑦) ∞ exp −𝑗 𝑜 2 𝜌 2 ℏ 2 𝑏 sin 𝑜𝜌 Ψ 𝑦, 𝑢 = 𝑑 𝑜 𝑏 𝑦 2𝑛𝑏 2 𝑢 𝑜=1 𝑑 𝑜 2 = 1 ∞ 𝑜=1 0 x a If only one coefficient 𝑑 𝑜 is non-zero, then stationary state If more than one level occupied (“ superposition ”), then non-stationary, state evolves in time (interference) n =3 Classical motion can be represented as combination   𝐹 3 of many high-numbered states (transition to classical     4 picture when energies are ≫ 𝐹 1,2,3 )     9 n =2  𝐹 2   Is a general (superposition) state localized in space? – No n =1   Is it localized in energy? – Also no! 𝐹 1   However, if we measure energy, then we will get some result: some 𝐹 𝑜 (nothing in between!)  If measure again – will get the same result (collapse)