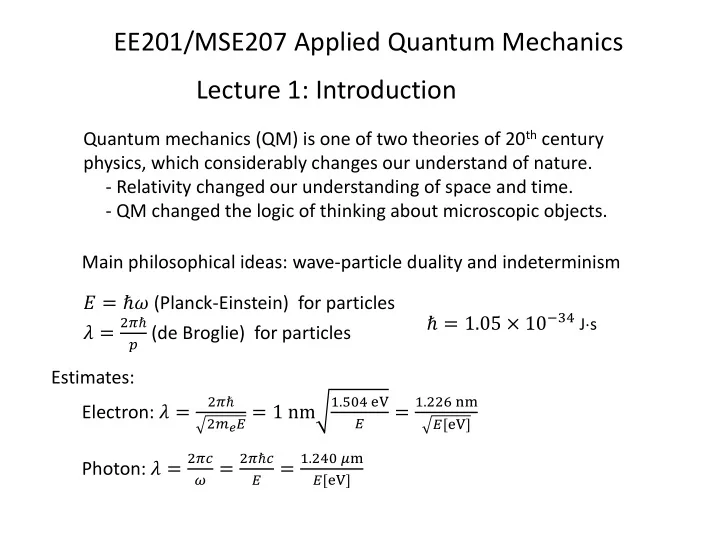
lecture 1 introduction
play

Lecture 1: Introduction Quantum mechanics (QM) is one of two theories - PowerPoint PPT Presentation

Mar 22, 2024 β€’373 likes β€’406 views

EE201/MSE207 Applied Quantum Mechanics Lecture 1: Introduction Quantum mechanics (QM) is one of two theories of 20 th century physics, which considerably changes our understand of nature. - Relativity changed our understanding of space and time.

  1. EE201/MSE207 Applied Quantum Mechanics Lecture 1: Introduction Quantum mechanics (QM) is one of two theories of 20 th century physics, which considerably changes our understand of nature. - Relativity changed our understanding of space and time. - QM changed the logic of thinking about microscopic objects. Main philosophical ideas: wave-particle duality and indeterminism 𝐹 = β„πœ• (Planck-Einstein) for particles ℏ = 1.05 Γ— 10 βˆ’34 J οƒ— s 2πœŒβ„ πœ‡ = π‘ž (de Broglie) for particles Estimates: 2πœŒβ„ 1.504 eV 1.226 nm Electron: πœ‡ = 2𝑛 𝑓 𝐹 = 1 nm = 𝐹 𝐹[eV] 2πœŒπ‘‘ 2πœŒβ„π‘‘ 1.240 𝜈m Photon: πœ‡ = πœ• = = 𝐹 𝐹[eV]

  2. Prehistory of Quantum Mechanics 1) 1900 Max Planck: suggested discrete absorption and emission of light to explain experimental formula for black-body radiation (Nobel Prize 1918) 𝐹 = β„Žπœ‰ = β„πœ• β„Ž = 6.63 Γ— 10 βˆ’34 J οƒ— s ℏ = 1.05 Γ— 10 βˆ’34 J οƒ— s 2) 1905 Albert Einstein: theory of photoelectric effect (Nobel Prize 1921) 𝑛𝑀 2 β„Žπœ‰ = Ξ¦ + Ξ¦ is work function (ionization energy) 2 3) 1913 Niels Bohr: model of atom (Nobel Prize 1922) 𝑛𝑀𝑠 = π‘œβ„ Discrete atomic spectra and Rutherford’s experiments (1910 -1911, N.P. 1908) 4) 1923 Arthur Compton: scattering of X-rays on electrons (Nobel Prize 1927) 5) 1923 Louis de Broglie: matter waves (theory only, in 1927 confirmed for πœ‡ = 2πœŒβ„ electrons, Nobel Prize 1929) π‘ž β€œBirth” of Quantum mechanics: 1927 (5 th Solvay conference, Brussels)

  3. Classical mechanics vs. Quantum Mechanics (one particle in one dimension) Classical mechanics: 𝑒 2 𝑦 𝑒𝑦 Position 𝑦(𝑒) , velocity 𝑀 𝑒 = 𝑒𝑒 , acceleration 𝑏 𝑒 = 𝑒𝑒 2 π‘’π‘Š(𝑦) 𝐺 = βˆ’ for a conservative system, π‘Š(𝑦) is potential energy 𝑒𝑦 𝐺 Main evolution equation: 𝑏 = 𝑛 𝑒 2 𝑦 𝑒𝑒 2 = βˆ’ 1 π‘’π‘Š initial conditions: οƒž 𝑦(𝑒) 𝑦 0 , 𝑦(0) 𝑛 𝑒𝑦 Quantum mechanics: Main evolution equation: SchrΓΆdinger equation πœ–π‘’ = βˆ’ ℏ 2 πœ– 2 Ξ¨ 𝑗ℏ πœ–Ξ¨ Ξ¨(𝑦, 𝑒) is a complex function, characterizing πœ–π‘¦ 2 + π‘ŠΞ¨ 2𝑛 the particle state (wave function) 2 𝑒𝑦 is the probability to find the particle between 𝑦 and 𝑦 + 𝑒𝑦 Ξ¨ 𝑦, 𝑒 at time 𝑒 (if observed!) Indeterminacy: particle does not have position (still debates about philosophy)

Recommend

Introduction to Numerical Optimization Biostatistics 615/815 Lecture 14 Lecture 14 Course is

Introduction to Numerical Optimization Biostatistics 615/815 Lecture 14 Lecture 14 Course is

Introduction to Numerical Optimization Biostatistics 615/815 Lecture 14 Lecture 14 Course is More Than Half Done! If you have comments they are very welcome y y Lectures Lecture notes Weekly Homework Midterm

795 views β€’ 42 slides
Introduction to AI & Intelligent Agents This Lecture Chapters 1 and 2 Next Lecture

Introduction to AI & Intelligent Agents This Lecture Chapters 1 and 2 Next Lecture

Introduction to AI & Intelligent Agents This Lecture Chapters 1 and 2 Next Lecture Chapter 3.1 to 3.4 (Please read lecture topic material before and after each lecture on that topic) What is Artificial Intelligence? Thought

636 views β€’ 31 slides
CSC321 Lecture 1: Introduction Roger Grosse Roger Grosse CSC321 Lecture 1: Introduction 1 / 29

CSC321 Lecture 1: Introduction Roger Grosse Roger Grosse CSC321 Lecture 1: Introduction 1 / 29

CSC321 Lecture 1: Introduction Roger Grosse Roger Grosse CSC321 Lecture 1: Introduction 1 / 29 What is machine learning? For many problems, its difficult to program the correct behavior by hand recognizing people and objects

474 views β€’ 35 slides
CSC2541 Lecture 1 Introduction Roger Grosse Roger Grosse CSC2541 Lecture 1 Introduction 1 / 36

CSC2541 Lecture 1 Introduction Roger Grosse Roger Grosse CSC2541 Lecture 1 Introduction 1 / 36

CSC2541 Lecture 1 Introduction Roger Grosse Roger Grosse CSC2541 Lecture 1 Introduction 1 / 36 Motivation Recent success stories of machine learning, and neural nets in particular But our algorithms still struggle with a decades-old

876 views β€’ 49 slides
CSC321 Lecture 1: Introduction Roger Grosse Roger Grosse CSC321 Lecture 1: Introduction 1 / 26

CSC321 Lecture 1: Introduction Roger Grosse Roger Grosse CSC321 Lecture 1: Introduction 1 / 26

CSC321 Lecture 1: Introduction Roger Grosse Roger Grosse CSC321 Lecture 1: Introduction 1 / 26 What is machine learning? For many problems, its difficult to program the correct behavior by hand recognizing people and objects

451 views β€’ 31 slides
Lecture Capture Introduction to Lecture Capture Learning Outcomes What will lecture capture

Lecture Capture Introduction to Lecture Capture Learning Outcomes What will lecture capture

ISDS & Digital Business Skills Lecture Capture Introduction to Lecture Capture Learning Outcomes What will lecture capture actually do? What happens before a lecture? What happens during a lecture? What does the button do?

527 views β€’ 18 slides
Proteomics Steven Meinhardt Lectures Lecture 1 Introduction review of proteins

Proteomics Steven Meinhardt Lectures Lecture 1 Introduction review of proteins

11/29/2012 Proteomics Steven Meinhardt Lectures Lecture 1 Introduction review of proteins Lecture 2 Gel Electrophoresis Lecture 3 Mass Spectrometry Lecture 4 Programs for MS analysis Lecture 5 MIAPE (Minimum

900 views β€’ 8 slides
Psycholiguistics Lecture 7 By Dr. Chelli Introduction to second language acquisition Lecture

Psycholiguistics Lecture 7 By Dr. Chelli Introduction to second language acquisition Lecture

Psycholiguistics Lecture 7 By Dr. Chelli Introduction to second language acquisition Lecture Objectives This lecture helps students to understand second language theories and how second language is processed. Introduction Second

618 views β€’ 38 slides
1 Plan Friday Lecture 1 Introduction in 2D History Lecture 2: Mesh, 3D cases

1 Plan Friday Lecture 1 Introduction in 2D History Lecture 2: Mesh, 3D cases

1 Plan Friday Lecture 1 Introduction in 2D History Lecture 2: Mesh, 3D cases Generalities Installation procedures Tutorial 1: simple cases Syntax Saturday Example 1: Laplaces equation Lecture 4: Fluid mechanics

630 views β€’ 38 slides
Lecture 2- Introduction to Latex (1) Lecture 0 slide 1 The objectives To strengthen the

Lecture 2- Introduction to Latex (1) Lecture 0 slide 1 The objectives To strengthen the

ECON 6009 Graduate Seminar Memorial University of Newfoundland Lecture 2- Introduction to Latex (1) Lecture 0 slide 1 The objectives To strengthen the knowledge the student gained in previous Economics modules about how to conduct

592 views β€’ 25 slides
Lecture Outline Regeltechniek Lecture 1 Introduction Information about the course.

Lecture Outline Regeltechniek Lecture 1 Introduction Information about the course.

Lecture Outline Regeltechniek Lecture 1 Introduction Information about the course. Robert Babu ska Why is control essential. Delft Center for Systems and Control Faculty of Mechanical Engineering Basic elements of feedback.

403 views β€’ 11 slides
Previous Lecture Introduction to SMIL 2 Introduction to W3C and XML Introduction to SMIL

Previous Lecture Introduction to SMIL 2 Introduction to W3C and XML Introduction to SMIL

Previous Lecture Introduction to SMIL 2 Introduction to W3C and XML Introduction to SMIL Writing a SMIL file Adding Media Objects Martin Halvey Clipping media files January 2008 Timing and Synchronising Layout

322 views β€’ 8 slides
Lecture 4: Introduction to Regression CS109A Introduction to Data Science Pavlos Protopapas,

Lecture 4: Introduction to Regression CS109A Introduction to Data Science Pavlos Protopapas,

Lecture 4: Introduction to Regression CS109A Introduction to Data Science Pavlos Protopapas, Kevin Rader and Chris Tanner Background Roadmap: Lecture 1 What is Data Science? Lecture 2 Data: types, formats, issues, etc, and briefly

643 views β€’ 45 slides
CSN08101 Digital Forensics Lecture 1A: Introduction to Forensics Lecture 1A: Introduction to

CSN08101 Digital Forensics Lecture 1A: Introduction to Forensics Lecture 1A: Introduction to

CSN08101 Digital Forensics Lecture 1A: Introduction to Forensics Lecture 1A: Introduction to Forensics Module Leader: Dr Gordon Russell Lecturers: Robert Ludwiniak Digital Forensics You will learn in this module: The principals of

1.09k views β€’ 34 slides
T odays lecture: Introduction to objects and classes Announcements: Try to

T odays lecture: Introduction to objects and classes Announcements: Try to

Previous lecture: File I/O, sort T odays lecture: Introduction to objects and classes Announcements: Try to finish Exercise 11 during DIS section ahead of Thursdays lecture. Test 2A will be released on Canvas at

396 views β€’ 23 slides
Lecture outline Introduction to the course Introduction to Machine Learning Least squares 3

Lecture outline Introduction to the course Introduction to Machine Learning Least squares 3

1 Introduction to Machine Learning Lecture 1: Introduction and Linear Regression Iasonas Kokkinos Iasonas.kokkinos@gmail.com University College London 2 Lecture outline Introduction to the course Introduction to Machine Learning Least

1.15k views β€’ 95 slides
CISC883: LECTURE 1 INTRODUCTION TO ULSS Cor-Paul Bezemer 2 Todays lecture Course

CISC883: LECTURE 1 INTRODUCTION TO ULSS Cor-Paul Bezemer 2 Todays lecture Course

CISC883: LECTURE 1 INTRODUCTION TO ULSS Cor-Paul Bezemer 2 Todays lecture Course summary Introduction to ULSS 3 Course Summary Course Notes: Ultra-Large-Scale Systems: The Software Challenge of the Future, Linda Northrop

1.21k views β€’ 43 slides
Introduction CSCE CSCE 496/896 496/896 Lecture 9: Lecture 9: word2vec and word2vec and To

Introduction CSCE CSCE 496/896 496/896 Lecture 9: Lecture 9: word2vec and word2vec and To

Introduction CSCE CSCE 496/896 496/896 Lecture 9: Lecture 9: word2vec and word2vec and To apply recurrent architectures to text (e.g., NLM), CSCE 496/896 Lecture 9: node2vec node2vec need numeric representation of words Stephen Scott

394 views β€’ 4 slides
LECTURE 4 LECTURE 4 Introduction to the Parton Model and Perturbative QCD Fred Olness (SMU)

LECTURE 4 LECTURE 4 Introduction to the Parton Model and Perturbative QCD Fred Olness (SMU)

CTEQ-MCnet school on QCD Analysis and Phenomenology and the Physics and Techniques of Event Generators LECTURE 4 LECTURE 4 Introduction to the Parton Model and Perturbative QCD Fred Olness (SMU) Lauterbad (Black Forest), Germany 26 July -

754 views β€’ 46 slides
Lecture 1 - Introduction Welcome! , = (, ) ,

Lecture 1 - Introduction Welcome! , = (, ) ,

INFOMAGR Advanced Graphics Jacco Bikker - November 2019 - February 2020 Lecture 1 - Introduction Welcome! , = (, ) , + , , ,

659 views β€’ 41 slides
Lecture 1 - Introduction Welcome! , = (, ) ,

Lecture 1 - Introduction Welcome! , = (, ) ,

INFOMAGR Advanced Graphics Jacco Bikker - November 2016 - February 2017 Lecture 1 - Introduction Welcome! , = (, ) , + , , ,

1.04k views β€’ 49 slides
Lecture 01: Introduction 2014-04-29 Dr. Bernd Westphal 01 2014-04-29 main

Lecture 01: Introduction 2014-04-29 Dr. Bernd Westphal 01 2014-04-29 main

Real-Time Systems Lecture 01: Introduction 2014-04-29 Dr. Bernd Westphal 01 2014-04-29 main Albert-Ludwigs-Universit at Freiburg, Germany Contents & Goals Last Lecture: ./. This Lecture: Educational Objectives:

1.07k views β€’ 20 slides
Lecture 01: Introduction 2014-04-29 Dr. Bernd Westphal 01 2014-04-29 main

Lecture 01: Introduction 2014-04-29 Dr. Bernd Westphal 01 2014-04-29 main

Real-Time Systems Lecture 01: Introduction 2014-04-29 Dr. Bernd Westphal 01 2014-04-29 main Albert-Ludwigs-Universit at Freiburg, Germany Contents & Goals Last Lecture: ./. This Lecture: Educational Objectives:

766 views β€’ 40 slides
Lecture 1 Andreas Habegger Introduction Zynq Introduction Zynq Introduction Zynq PS vs. PL

Lecture 1 Andreas Habegger Introduction Zynq Introduction Zynq Introduction Zynq PS vs. PL

Introduction Zynq Lecture 1 Andreas Habegger Introduction Zynq Introduction Zynq Introduction Zynq PS vs. PL Processing System Processor Peripherals Data Buses AXI Bus Conclusion BTE5380 - Embedded Systems Octobre 2014 Andreas Habegger

1.81k views β€’ 30 slides

More recommend