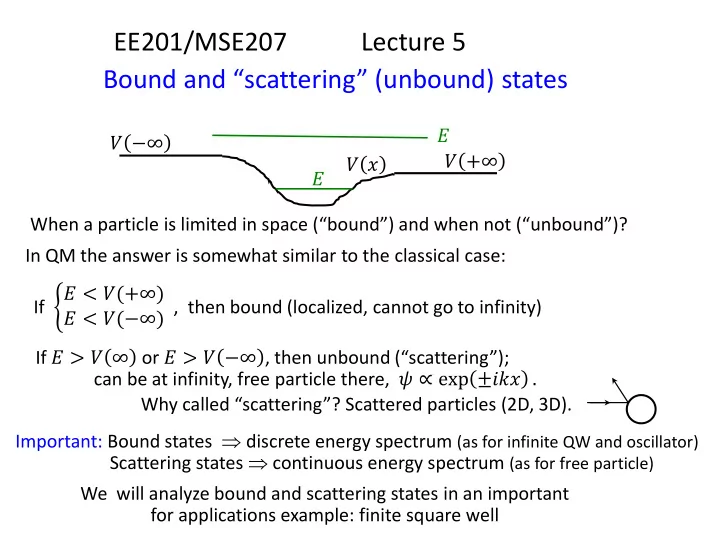
EE201/MSE207 Lecture 5 Bound and scattering (unbound) states - PowerPoint PPT Presentation

EE201/MSE207 Lecture 5 Bound and “scattering” (unbound) states 𝐹 𝑊 −∞ 𝑊 +∞ 𝑊 𝑦 𝐹 When a particle is limited in space (“bound”) and when not (“unbound”)? In QM the answer is somewhat similar to the classical case: If 𝐹 < 𝑊(+∞) 𝐹 < 𝑊(−∞) , then bound (localized, cannot go to infinity) If 𝐹 > 𝑊 ∞ or 𝐹 > 𝑊 −∞ , then unbound (“scattering”); can be at infinity, free particle there, 𝜔 ∝ exp ±𝑗𝑙𝑦 . Why called “scattering”? Scattered particles (2D, 3D). Important: Bound states  discrete energy spectrum (as for infinite QW and oscillator) Scattering states  continuous energy spectrum (as for free particle) We will analyze bound and scattering states in an important for applications example: finite square well

Finite square well 𝑊(𝑦) 0 𝑊 𝑦 = −𝑊 0 , −𝑏 < 𝑦 < 𝑏 0 , 𝑦 > 𝑏 width 2𝑏 (it was 𝑏 for infinite well) −𝑊 0 depth 𝑊 −𝑏 𝑏 0 0 Today: Bound states, 𝐹 < 0 ( 𝐹 < 𝐹 ±∞ = 0 ) 0 𝐹 − ℏ 2 𝑒 2 𝜔 𝐹 < 0 𝑒𝑦 2 + 𝑊(𝑦)𝜔 = 𝐹𝜔 TISE 𝐹 + 𝑊 2𝑛 0 𝑊 0 > 0 −𝑊 0 𝐹 + 𝑊 0 > 0 Three regions: (1) 𝑦 < −𝑏 , (2) −𝑏 < 𝑦 < 𝑏 , (1) (2) (3) (3) 𝑦 > 𝑏

Solving TISE in 3 regions 0 𝐹 − ℏ 2 𝑒 2 𝜔 𝑒𝑦 2 + 𝑊(𝑦)𝜔 = 𝐹𝜔 𝐹 + 𝑊 0 2𝑛 −𝑊 (1) (2) (3) 0 𝑒 2 𝜔 𝑒𝑦 2 = − 2𝑛𝐹 𝐹 < 0 , 𝑊 0 > 0 ,  𝑊 = 0  𝜔 (1) 𝑦 < −𝑏 ℏ 2 𝐹 + 𝑊 0 > 0 > 0 , = 𝑙 2 −2𝑛𝐹 𝜔 𝑦 = 𝐵 𝑓 −𝑙𝑦 + 𝐶 𝑓 𝑙𝑦 , 𝑙 = ℏ 𝐵 = 0 because 𝜔 −∞ = 0 𝑒 2 𝜔 𝑒𝑦 2 = − 2𝑛(𝑊 0 + 𝐹) 𝜔   (2) −𝑏 < 𝑦 < 𝑏 𝑊 = −𝑊 ℏ 2 0 < 0 , = −𝑚 2 2𝑛(𝑊 0 + 𝐹) 𝜔 𝑦 = 𝐷 sin(𝑚𝑦) + 𝐸 cos(𝑚𝑦) 𝑚 = ℏ ( sin and cos are more convenient for bound states, 𝑓 ±𝑗𝑚𝑦 more convenient for scattering states) 𝜔 𝑦 = 𝐺 𝑓 −𝑙𝑦 + 𝐻 𝑓 𝑙𝑦 (the same 𝑙 )  𝑊 = 0  (3) 𝑦 > 𝑏 𝐻 = 0 because 𝜔 +∞ = 0

0 −2𝑛𝐹 𝐹 𝜔 𝑦 = 𝐶 𝑓 𝑙𝑦 , (1) 𝑦 < −𝑏 𝑙 = 𝐹 + 𝑊 ℏ 0 −𝑊 (1) (2) (3) 0 (2) −𝑏 < 𝑦 < 𝑏 𝜔 𝑦 = 𝐷 sin(𝑚𝑦) + 𝐸 cos(𝑚𝑦) 𝑚 = 2𝑛(𝑊 0 + 𝐹) ℏ (3) 𝑦 > 𝑏 𝜔 𝑦 = 𝐺 𝑓 −𝑙𝑦 1) 𝜔 𝑦 is continuous Boundary conditions: 2) 𝑒𝜔/𝑒𝑦 is also continuous 2’) actually, in semiconductors condition 2) is different: 1 𝑒𝜔 (this is not discussed 𝑒𝑦 is continuous in Griffiths’ book) 𝑛 𝑓𝑔𝑔 2𝑛 𝜔 𝑒𝜔 ∗ 𝑗ℏ 𝑒𝑦 − 𝜔 ∗ 𝑒𝜔 Follows from continuity of probability current 𝑒𝑦 We have 5 equations (4 boundary conditions and normalization) and 5 unknowns ( 𝐶, 𝐷, 𝐸, 𝐺, and 𝐹 ). Possible to solve, but too many. 𝑔 −𝑦 = 𝑔(𝑦) even Simplification: trick of odd and even functions 𝑔 −𝑦 = −𝑔(𝑦) odd

Trick of odd and even functions for even potential, 𝑊 −𝑦 = 𝑊(𝑦) In our case 𝑊(𝑦) is even Theorem is a solution of TISE with energy 𝐹 , If 𝑊 −𝑦 = 𝑊(𝑦) and 𝜔 𝑦 𝐼𝜔 = 𝐹𝜔 , then 𝜔(−𝑦) is also a solution with the same energy, 𝐼𝜔(−𝑦) = 𝐹𝜔(−𝑦) . (simple to prove, and also quite obvious) Then 𝜔 𝑦 + 𝜔(−𝑦) is also a solution, and 𝜔 𝑦 − 𝜔(−𝑦) is also a solution (because TISE is linear in 𝜔 ), (not necessarily normalized, but not a problem) 𝜔 𝑦 + 𝜔(−𝑦) is even 𝜔 𝑦 − 𝜔(−𝑦) is odd (actually, if 𝜔(𝑦) is even or odd, then one of the combinations is zero) Therefore, it is sufficient to find only even and odd solutions of TISE

Even solutions for finite square well 𝐶 exp 𝑙𝑦 , 𝑦 < −𝑏 only 3 unknowns: 𝜔 𝑦 = 𝐸 cos 𝑚𝑦 , 𝑦 < 𝑏 (no sin-term) 𝐶, 𝐸, 𝐹 𝐶 exp −𝑙𝑦 , 𝑦 > 𝑏 (the same factor 𝐶 ) Boundary condition at 𝑦 = 𝑏 (b.c. at 𝑦 = −𝑏 gives the same): 𝐶 exp(−𝑙𝑏) = 𝐸 cos(𝑚𝑏) −𝑙𝐶 exp −𝑙𝑏 = −𝑚𝐸 sin(𝑚𝑏) 𝑙 = 𝑚 tan(𝑚𝑏) Divide equations: This equation gives energy 𝐹 since 𝑙(𝐹) , 𝑚(𝐹) Rewrite: tan(𝑚𝑏) = 𝑙 −2𝑛𝐹/ℏ −𝐹 𝑊 𝑊 0 2𝑛 0 𝑚 = = 0 + 𝐹 = 0 + 𝐹 − 1 = 𝑚 2 ℏ 2 − 1 𝑊 𝑊 2𝑛 𝑊 0 + 𝐹 /ℏ 𝑏 2 𝑊 0 2𝑛 ℏ 2 tan(𝑚𝑏) = − 1 𝑚𝑏 2

Even solutions for finite square well 𝑏 2 𝑊 0 2𝑛 ℏ 2 tan(𝑚𝑏) = − 1 Solve graphically 𝑚𝑏 2 2𝑛(𝑊 0 + 𝐹) 𝑚 = ℏ 𝑏 2𝑛𝑊 0 ℏ 𝑂 even = int + 1 Finite number of solutions: 𝜌 𝑏 2 𝑊 0 2𝑛 Limiting cases 1) ≫ 1 (wide, deep well) ℏ 2 0 = 𝑚 2 ℏ 2 2𝑛 ≈ 2𝑜 + 1 2 𝜌 2 ℏ 2 Low levels: 𝑚𝑏 ≈ 2𝑜 + 1 𝜌/2 , 𝐹 𝑜 + 𝑊 2𝑛 2𝑏 2 (similar to infinite well, but only odd states and 𝑏 → 2𝑏) 𝑏 2 𝑊 0 2𝑛 (shallow, narrow well) Only one level: 𝑚𝑏 ≈ 𝑏 2𝑛𝑊 0 /ℏ ≪ 1, ≪ 1 2) ℏ 2 𝐹 ≪ 𝑊 0

Even solutions for finite square well: normalization (not important) 𝐶 exp 𝑙𝑦 , 𝑦 < −𝑏 𝜔 𝑦 = 𝐸 cos 𝑚𝑦 , 𝑦 < 𝑏 𝐶 exp −𝑙𝑦 , 𝑦 > 𝑏 Normalization 𝐶 = exp 𝑙𝑏 cos(𝑚𝑏) ∞ 𝑏 + 1/𝑙 2 𝑒𝑦 = 1 𝜔 𝑦 ⟹ 1 −∞ 𝐸 = 𝑏 + 1/𝑙

Odd solutions (similar) 𝐶 exp 𝑙𝑦 , 𝑦 < −𝑏 𝜔 𝑦 = 𝐷 sin(𝑚𝑦), 𝑦 < 𝑏 (no cos-term) −𝐶 exp −𝑙𝑦 , 𝑦 > 𝑏 ( −𝐶 since odd) Boundary condition at 𝑦 = 𝑏 : −𝐶 exp −𝑙𝑏 = 𝐷 sin(𝑚𝑏) 𝑙𝐶 exp −𝑙𝑏 = 𝐷𝑚 cos(𝑚𝑏) 𝑙 = −𝑚 cot(𝑚𝑏) Divide equations: 𝑏 2 𝑊 0 2𝑛 ℏ 2 −cot(𝑚𝑏) = − 1 After some algebra: 𝑚𝑏 2 Similar to the even case, the only difference: tan 𝑚𝑏 → −cot(𝑚𝑏) (just shifted by 𝜌/2 )

Odd solutions for finite square well 𝑏 2 𝑊 0 2𝑛 ℏ 2 −cot(𝑚𝑏) = − 1 𝑚𝑏 2 Number of solutions: 𝑏 2𝑛𝑊 + 1 0 ℏ 𝑂 odd = int 𝜌 2 Total number of solutions: 𝑏 2𝑛𝑊 0 ℏ 𝑂 even + 𝑂 odd = int + 1 𝜌/2 𝑏 2 𝑊 0 2𝑛 Limiting cases 1) ≫ 1 (wide, deep well) ℏ 2 0 = 𝑚 2 ℏ 2 2𝑛 ≈ 2𝑜 2 𝜌 2 ℏ 2 𝐹 𝑜 + 𝑊 Low levels: 𝑚𝑏 ≈ 𝑜𝜌 , 2𝑛 2𝑏 2 (these are remaining solutions for infinite well with 𝑏 → 2𝑏) 0 < 𝜌 2 ℏ 2 2) 𝑊 Shallow, narrow well No odd solutions if 8𝑛𝑏 2

Digression: some integrals (easy to derive by squaring and considering as a ∞ 𝜌 𝑓 −𝑏𝑦 2 𝑒𝑦 = double-integral; also from normalization of a 𝑏 ∞ 1 2𝜌𝐸 𝑓 −𝑦 2 /2𝐸 𝑒𝑦 = 1 . −∞ Gaussian: −∞ Take derivative in respect to parameter 𝑏 ∞ ∞ 𝜌 𝜌 −𝑦 2 𝑓 −𝑏𝑦 2 𝑒𝑦 = − 𝑦 2 𝑓 −𝑏𝑦 2 𝑒𝑦 = ⇒ 2𝑏 3/2 2𝑏 3/2 −∞ −∞ Take another derivative with respect to 𝑏 , similarly: ∞ 𝑦 4 𝑓 −𝑏𝑦 2 𝑒𝑦 = 3 𝜌 (and so on: 𝑦 6 , 𝑦 8 , etc.) 4𝑏 5/2 −∞ ∞ 𝑦 𝑓 −𝑏𝑦 2 𝑒𝑦 = 1 Can construct a similar series, starting with 2𝑏 0