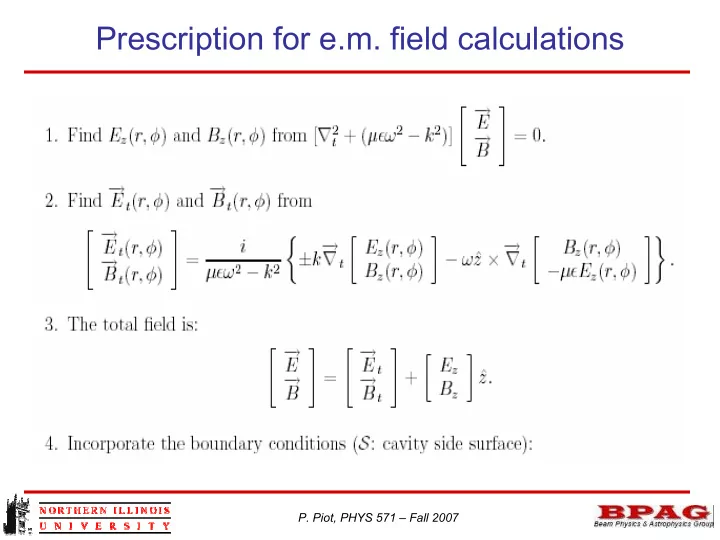

Prescription for e.m. field calculations P. Piot, PHYS 571 – Fall 2007
Boundary conditions I • Electric field components z z S • Magnetic field components end plates Proof: start with what we had last lesson ⇔ P. Piot, PHYS 571 – Fall 2007
Boundary conditions II • Which gives But On Surface S This is the gradient of B z projected on n • So our the boundary conditions are P. Piot, PHYS 571 – Fall 2007
Resonant mode types • Resonant mode categorization The two aforementioned boundary conditions cannot be satisfied simultaneously. To each of this boundary condition corresponds a mode type: • In old books/literature this is referred to as H and E modes P. Piot, PHYS 571 – Fall 2007
Wave equation in ( r, φ, z ) and its solutions I • We first want to find E z or B z . • Boundary condition at end plates z 0 L • Defining the wave equation takes the form P. Piot, PHYS 571 – Fall 2007
Wave equation in ( r, φ, z ) and its solutions II Once Ψ( r ,φ) is found the transverse field can be computed from • In ( r, φ ,z ) the wave equation is: • P. Piot, PHYS 571 – Fall 2007
Wave equation in ( r, φ, z ) and its solutions III • We now further assume an harmonic azimutal dependence • The wave equation simplifies to the Bessel’s differential equation [ see Arken & Weber (6 th Edition) p. 678 ] • Which has solution of the form since P. Piot, PHYS 571 – Fall 2007
Wave equation in ( r, φ, z ) – Bessel Functions Second kind Bessel Functions Y ≡ N First kind Bessel Functions P. Piot, PHYS 571 – Fall 2007
Wave equation in ( r, φ, z ) and its solutions IV and resonant frequencies • So finally where – ......... – R is the cavity radius, γ nm is defined to insure Ψ→ 0 as r → R. – • From the definition we note that only discrete frequencies are allowed: P. Piot, PHYS 571 – Fall 2007
TM mode • For TM modes so that • The transverse E-field is using P. Piot, PHYS 571 – Fall 2007
TM mode: transverse E-field P. Piot, PHYS 571 – Fall 2007
TM mode: transverse B-field P. Piot, PHYS 571 – Fall 2007
TM mode: summary P. Piot, PHYS 571 – Fall 2007
TE mode: summary P. Piot, PHYS 571 – Fall 2007
Physical insights: exampleTM 010 mode Courtesy of H. Padamsee, Cornell University P. Piot, PHYS 571 – Fall 2007
Recommend
More recommend