Finite square well: scattering states > 0 = 0 , < < - PowerPoint PPT Presentation

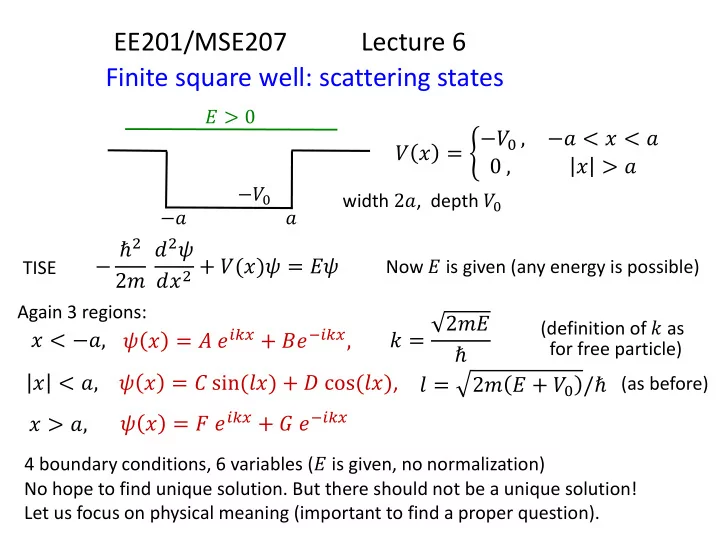
EE201/MSE207 Lecture 6 Finite square well: scattering states 𝐹 > 0 𝑊 𝑦 = −𝑊 0 , −𝑏 < 𝑦 < 𝑏 0 , 𝑦 > 𝑏 −𝑊 width 2𝑏 , depth 𝑊 0 0 −𝑏 𝑏 − ℏ 2 𝑒 2 𝜔 𝑒𝑦 2 + 𝑊(𝑦)𝜔 = 𝐹𝜔 Now 𝐹 is given (any energy is possible) TISE 2𝑛 Again 3 regions: 2𝑛𝐹 (definition of 𝑙 as 𝜔 𝑦 = 𝐵 𝑓 𝑗𝑙𝑦 + 𝐶𝑓 −𝑗𝑙𝑦 , 𝑦 < −𝑏, 𝑙 = for free particle) ℏ 𝑦 < 𝑏, 𝜔 𝑦 = 𝐷 sin(𝑚𝑦) + 𝐸 cos(𝑚𝑦) , 𝑚 = 2𝑛 𝐹 + 𝑊 0 /ℏ (as before) 𝜔 𝑦 = 𝐺 𝑓 𝑗𝑙𝑦 + 𝐻 𝑓 −𝑗𝑙𝑦 𝑦 > 𝑏, 4 boundary conditions, 6 variables ( 𝐹 is given, no normalization) No hope to find unique solution. But there should not be a unique solution! Let us focus on physical meaning (important to find a proper question).

Add time dependence 𝑗𝑙 𝑦 − ℏ𝑙 −𝑗𝑙 𝑦 + ℏ𝑙 2𝑛 𝑢 + 𝐶 𝑓 2𝑛 𝑢 𝑦 < −𝑏, Ψ 𝑦, 𝑢 = 𝐵 𝑓 𝐵 outgoing wave incident wave 𝐶 (as for free particle; different phase and group velocities, but the same direction) 𝐵 𝐺 Similarly for 𝑦 > 𝑏 𝐶 𝐻 (if necessary, wave packets can be constructed later; in reality nobody usually does it because it is too complicated; instead, people work with unnormalized states) Assume that the wave is incident from the left, then 𝐻 = 0 𝐵 𝐺 𝐵 is incident wave amplitude 𝐶 𝐶 is reflected wave amplitude 𝐺 is transmitted wave amplitude We have 5 variables ( 𝐵, 𝐶, 𝐷, 𝐸, 𝐺 ) and 4 equations. Equations are linear. Can express 𝐶, 𝐷, 𝐸, 𝐺 as functions of 𝐵 (incident amplitude).

Proper questions 𝐵 𝐺 𝐵 is incident wave amplitude (assume a wave incident 𝐶 𝐶 is reflected wave amplitude from the left) 𝐺 is transmitted wave amplitude Goal: find ratios 𝑠 = 𝐶 and 𝑢 = 𝐺 (these ratios are called reflection 𝐵 𝐵 and transmission amplitudes) 𝑆 = 𝑠 2 = 𝐶 2 Reflection coefficient (probability of reflection) 𝐵 2 𝑈 = 𝑢 2 = 𝐺 2 Transmission coefficient (probability of transmission) 𝐵 2 𝑈 + 𝑆 = 1 From physical meaning Remark 1. Definition of 𝑈 is sometimes different (discuss later, × 𝑤 𝑠 /𝑤 𝑚 ) Remark 2. Terminology: Reflection/transmission amplitudes ( 𝑠, 𝑢 ) and coefficients ( 𝑆, 𝑈 ) Remark 3. We defined 𝑆 and 𝑈 as ratios; they become probabilities for wave packets (possible to show). Quadratic because probability ∝ Ψ 2 .

𝐵 𝐺 Finding 𝑈 and 𝑆 𝐶 𝜔 𝑦 = 𝐵 𝑓 𝑗𝑙𝑦 + 𝐶 𝑓 −𝑗𝑙𝑦 𝑦 < −𝑏, 𝑦 < 𝑏, 𝜔 𝑦 = 𝐷 sin(𝑚𝑦) + 𝐸 cos(𝑚𝑦) 𝜔 𝑦 = 𝐺 𝑓 𝑗𝑙𝑦 𝑦 > 𝑏, 𝐵 𝑓 −𝑗𝑙𝑏 + 𝐶𝑓 𝑗𝑙𝑏 = −𝐷 sin 𝑚𝑏 + 𝐸 cos 𝑚𝑏 Boundary conditions: 𝑗𝑙 𝐵 𝑓 −𝑗𝑙𝑏 − 𝐶𝑓 𝑗𝑙𝑏 = 𝑚 [𝐷 cos 𝑚𝑏 + 𝐸 sin 𝑚𝑏 ] 𝑦 = −𝑏 𝐷 sin 𝑚𝑏 + 𝐸 cos 𝑚𝑏 = 𝐺 𝑓 𝑗𝑙𝑏 𝑦 = 𝑏 𝑚 [𝐷 cos 𝑚𝑏 − 𝐸 sin 𝑚𝑏 = 𝑗𝑙 𝐺 𝑓 𝑗𝑙𝑏 Simple to exclude 𝐷 and 𝐸 (similar combinations), then 2 equations with 𝐵, 𝐶, 𝐺 𝑓 −2𝑗𝑙𝑏 2𝑛𝐹 Finally 𝐺 = 𝐵 𝑙 = cos 2𝑚𝑏 − 𝑗 sin 2𝑚𝑏 (𝑙 2 + 𝑚 2 ) ℏ 2𝑙𝑚 2𝑛 𝐹 + 𝑊 𝐶 = 𝑗 sin 2𝑚𝑏 0 𝑚 = (𝑚 2 − 𝑙 2 ) 𝐺 ℏ 2𝑙𝑚

Finding 𝑈 and 𝑆 𝐵 𝐺 𝜔 𝑦 = 𝐵 𝑓 𝑗𝑙𝑦 + 𝐶 𝑓 −𝑗𝑙𝑦 𝐹 𝑦 < −𝑏, 𝐶 𝜔 𝑦 = 𝐺 𝑓 𝑗𝑙𝑦 𝑦 > 𝑏, −𝑊 0 𝑈 = 𝐺 2 1 Transmission 𝐵 2 = probability 2 𝑊 0 ) sin 2 2𝑏 0 1 + 2𝑛 𝐹 + 𝑊 0 ℏ 4𝐹(𝐹 + 𝑊 Reflection (too long from 𝐶 2 / 𝐵 2 ) 𝑆 = 1 − 𝑈 probability 0 = 𝑜 2 𝜌 2 ℏ 2 2𝑏 2𝑛(𝐹 + 𝑊 0 ) = 𝑜𝜌 ⟺ 𝐹 + 𝑊 Remark. 𝑈 = 1 if 2𝑛 2𝑏 2 ℏ This is exactly the “simple” energy quantization (in infinite well). Explanation: destructive interference of reflected waves (similar to anti-reflective coating with quarter-wavelength films).

Now wave incident from the right 𝐺 𝐹 𝐶 𝐻 𝜔 𝑦 = 𝐶 𝑓 −𝑗𝑙𝑦 𝑦 < −𝑏, −𝑊 𝜔 𝑦 = 𝐺 𝑓 𝑗𝑙𝑦 + 𝐻 𝑓 −𝑗𝑙𝑦 0 𝑦 > 𝑏, Similarly, we can find transmission and reflection coefficients r = 𝑢 r 2 = 𝐶 2 r 2 = 𝐺 2 𝑈 𝑆 r = 𝑠 𝑈 r + 𝑆 r = 1 𝐻 2 𝐻 2 In our case because of symmetry 𝑈 r = 𝑈 l = 𝑈 𝑆 r = 𝑈 l = 𝑆 However, this is always true (for any potential 𝑊 𝑦 and possibly different masses)

𝑈 and 𝑆 in general case 𝐹 𝑊 −∞ ≠ 𝑊 ∞ 𝑊 −∞ 𝑊 +∞ 𝑊 𝑦 and/or 𝑛 1 ≠ 𝑛 2 𝑛 1 𝑛 2 𝐺 𝐵 𝐶 𝑆 = 𝐶 2 𝐵 2 = 𝑠 2 𝑈 + 𝑆 = 1 𝑈 = 𝐺 2 = 𝐺 2 = 𝐺 2 𝐹 − 𝑊(+∞) 𝑛 1 𝑙 2 /𝑛 2 𝑤 2 = 𝑢 2 𝑤 2 𝐵 2 𝐵 2 𝐵 2 𝐹 − 𝑊(−∞) 𝑛 2 𝑙 1 /𝑛 1 𝑤 1 𝑤 1 2𝑛 𝜔 𝑒𝜔 ∗ 𝐾 = 𝑗ℏ 𝑒𝑦 − 𝜔 ∗ 𝑒𝜔 (remember that 𝑛 −1 𝑒𝜔 Why? Probability current 𝑒𝑦 is 𝑒𝑦 𝑒𝜔 𝑒𝑦 ) 𝐾 = 𝐵 2 ℏ𝑙 continuous, not just If 𝜔 𝑦 = 𝐵 𝑓 𝑗𝑙𝑦 , then 𝑛 = 𝐵 2 𝑤 The same velocity for reflection, but may be different for transmission

Transmission/reflection for  -potential 𝛽 𝜀 𝑦 𝐵 𝑓 𝑗𝑙𝑦 𝐺 𝑓 𝑗𝑙𝑦 𝑙 = 2𝑛𝐹/ℏ 𝑊 𝑦 = 𝛽 𝜀 𝑦 𝐹 > 0 𝐶 𝑓 −𝑗𝑙𝑦 𝐻 𝑓 −𝑗𝑙𝑦 − ℏ 2 𝑒 2 𝜔 𝑒𝑦 2 + 𝑊(𝑦)𝜔 = 𝐹𝜔 TISE 2𝑛 𝜁 − ℏ 2 2𝑛 𝜔 ′ 𝜁 − 𝜔 ′ −𝜁 + 𝛽 𝜔(0) = 0 … ⇒ Integrate TISE near zero, 𝜁 → 0 −𝜁 𝑒𝜔(+0) − 𝑒𝜔 −0 = 2𝑛𝛽 ℏ 2 𝜔(0) 𝑒𝑦 𝑒𝑦 With  -potential, 𝑒𝜔/𝑒𝑦 has a step (not continuous). Boundary conditions 𝐵 + 𝐶 = 𝐺 + 𝐻 𝑗𝑙 𝐺 − 𝐻 − 𝑗𝑙(𝐵 − 𝐶) = 2𝑛𝛽 ℏ 2 (𝐵 + 𝐶) −𝑗 𝑛𝛽 If 𝐻 = 0 (incident 𝐶 𝐺 1 ℏ 2 𝑙 𝐵 = 𝐵 = , from the left), then 1 + 𝑗 𝑛𝛽 1 + 𝑗 𝑛𝛽 ℏ 2 𝑙 ℏ 2 𝑙

[not included into this course] Scattering matrix (now waves incident from both sides) 𝐵 𝐺 For simplicity assume 𝐻 𝐶 𝑊 −∞ = 𝑊 ∞ , 𝑛 1 = 𝑛 2 𝑊 𝑦 𝑦 → −∞ 𝑦 → ∞ 𝜔 𝑦 = 𝐵𝑓 𝑗𝑙𝑦 + 𝐶𝑓 −𝑗𝑙𝑦 𝜔 𝑦 = 𝐺𝑓 𝑗𝑙𝑦 + 𝐻𝑓 −𝑗𝑙𝑦 Out of 4 wave amplitudes ( 𝐵, 𝐶, 𝐺, 𝐻 ), 2 free parameters, and the other 2 can be calculated (linear relations) Why 2 free parameters? 1) it was 2 in rectangular well 2) TISE is a second-order dif. eq.  2 boundary conditions Suppose we found transmission/reflection amplitudes ( 𝑢 𝑚 , 𝑠 𝑚 ) for the wave incident from the left and also from the right ( 𝑢 𝑠 , 𝑠 𝑠 ). It is convenient to write these 4 complex numbers as a 2 × 2 matrix. 𝐶 𝑇 𝐵 𝑇 = 𝑠 𝑢 𝑠 = 𝑇 11 𝑇 12 = 𝑚 What is the meaning? 𝐺 𝐻 𝑢 𝑚 𝑠 𝑇 21 𝑇 22 𝑠 (outgoing via incoming) (scattering matrix)

Scattering matrix ( S -matrix) 𝐵 𝐺 For simplicity assume 𝐻 𝐶 𝑊 −∞ = 𝑊 ∞ , 𝑛 1 = 𝑛 2 𝑊 𝑦 𝑦 → −∞ 𝑦 → ∞ 𝜔 𝑦 = 𝐵𝑓 𝑗𝑙𝑦 + 𝐶𝑓 −𝑗𝑙𝑦 𝜔 𝑦 = 𝐺𝑓 𝑗𝑙𝑦 + 𝐻𝑓 −𝑗𝑙𝑦 𝐶 𝑇 𝐵 𝑇 = 𝑠 𝑢 𝑠 = 𝑇 11 𝑇 12 = 𝑚 What is the meaning? 𝐺 𝐻 𝑢 𝑚 𝑠 𝑇 21 𝑇 22 𝑠 (outgoing via incoming) = 𝑠 𝑚 𝐵 𝐶 = 𝑢 𝑠 𝐻 𝐶 Suppose 𝐻 = 0 , then Suppose A = 0 , then 𝑢 𝑚 𝐵 𝐺 𝑠 𝑠 𝐻 𝐺 𝑚 = 𝑢 𝑚 2 = 𝑇 21 2 , 𝑆 𝑚 = 𝑠 𝑚 2 = 𝑇 11 2 𝑈 (remember that formulas for 𝑈 𝑠 = 𝑢 𝑠 2 = 𝑇 12 2 , 𝑆 𝑠 = 𝑠 𝑠 2 = 𝑇 22 2 in general case are different) 𝑈 𝑆 𝑚 = 𝑆 𝑠 Let us prove (for brevity will use notation: 𝑢 𝑚 = 𝑢 , 𝑠 𝑚 = 𝑠 ) 𝑈 𝑚 = 𝑈 symmetry: 𝑠

Symmetry of the scattering matrix Our proof will use “graphical operations” with solutions of TISE 𝐵 = 1 (conjugation = “time reversal”) TISE 𝑢 𝑢 𝑠 +  Conjugate solution of TISE 𝑠 − 1 − 𝑢 ∗ 1 𝑢 ∗ 𝑠 ∗ × −1 𝑠 ∗ −𝑠 ∗ 𝑠 ∗ 𝑠 ∗ Now multiply by 𝑢 ∗ −𝑠 ∗ 𝑢  𝑢 𝑠 = 𝑢 therefore 𝑢 ∗ 𝑠 = −𝑠 ∗ 𝑢 𝑠 𝑢 ∗ −𝑠 ∗ 𝑠 − 1 1 = 𝑢 ∗ 𝑠 ∗ 𝑠 𝑢 −𝑠 ∗ 𝑢 = 1 − |𝑠| 2 = 𝑢 2 𝑇 = 𝑢 𝑢 ∗ = 𝑢 𝑢 ∗ 𝑢 ∗ 𝑈 𝑠 = 𝑈 𝑚  𝑆 𝑠 = 𝑆 𝑚

Symmetry of the S -matrix in general case 𝑊 −∞ ≠ 𝑊 ∞ , 𝑛 1 ≠ 𝑛 2 Without derivation, just a result 𝑠 = −𝑠 ∗ 𝑢 𝑠 (velocity 𝑤 1 at the left, 𝑤 2 at the right) Still 𝑢 ∗ 𝑢 2 𝑤 2 𝑢 𝑠 = 1 − 𝑠 2 𝑤 2 = 𝑙 2 /𝑛 2 = 𝑢 𝑤 2 𝑤 1 = But 𝑤 1 𝑙 1 /𝑛 1 𝑢 ∗ 𝑢 ∗ 𝑤 1 𝑢 𝑤 2 𝑠 𝑤 1 𝑇 = −𝑠 ∗ 𝑢 𝑢 𝑢 ∗ 𝑠 = 𝑢 𝑠 2 𝑤 1 = 𝑢 2 𝑤 2 𝑈 = 𝑈 𝑚 Then 𝑈 𝑠 = 𝑈 𝑚 𝑤 2 𝑤 1  still 𝑆 𝑠 = 𝑆 𝑚 𝑆 𝑠 = 𝑆 𝑚