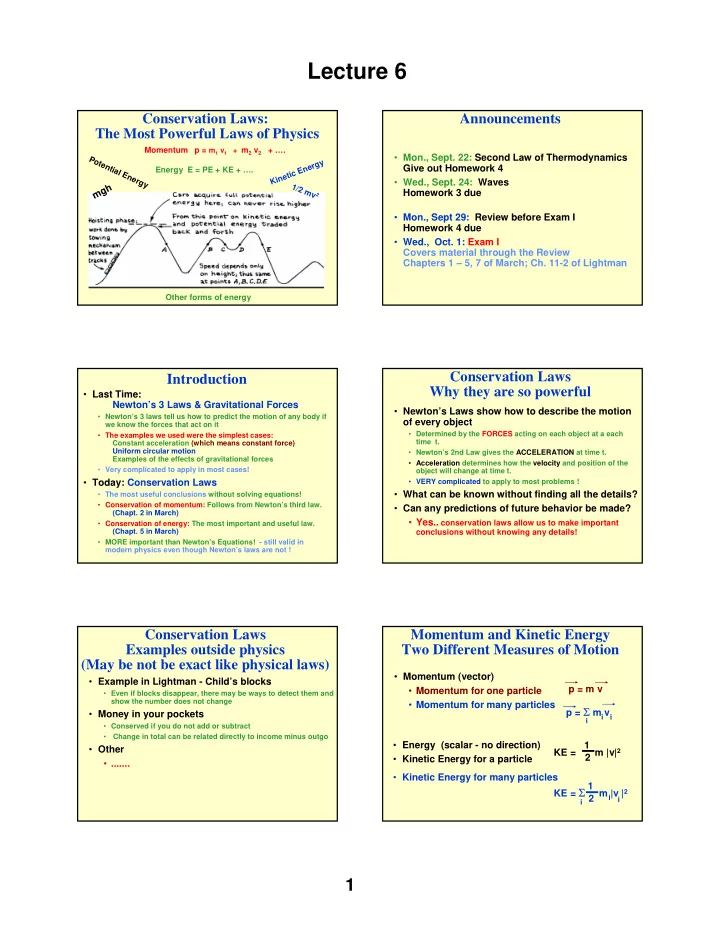

Lecture 6 Conservation Laws: Announcements The Most Powerful Laws of Physics Momentum p = m 1 v 1 + m 2 v 2 + …. • Mon., Sept. 22: Second Law of Thermodynamics Potential Energy Kinetic Energy Give out Homework 4 Energy E = PE + KE + …. • Wed., Sept. 24: Waves mgh 1 / 2 m Homework 3 due v 2 • Mon., Sept 29: Review before Exam I Homework 4 due • Wed., Oct. 1: Exam I Covers material through the Review Chapters 1 – 5, 7 of March; Ch. 11-2 of Lightman Other forms of energy Conservation Laws Introduction Why they are so powerful • Last Time: Newton’s 3 Laws & Gravitational Forces • Newton’s Laws show how to describe the motion • Newton’s 3 laws tell us how to predict the motion of any body if of every object we know the forces that act on it • Determined by the FORCES acting on each object at a each • The examples we used were the simplest cases: time t. Constant acceleration (which means constant force) Uniform circular motion • Newton’s 2nd Law gives the ACCELERATION at time t. Examples of the effects of gravitational forces • Acceleration determines how the velocity and position of the • Very complicated to apply in most cases! object will change at time t. • Today: Conservation Laws • VERY complicated to apply to most problems ! • What can be known without finding all the details? • The most useful conclusions without solving equations! • Conservation of momentum: Follows from Newton’s third law. • Can any predictions of future behavior be made? (Chapt. 2 in March) • Yes.. conservation laws allow us to make important • Conservation of energy: The most important and useful law. (Chapt. 5 in March) conclusions without knowing any details! • MORE important than Newton’s Equations! - still valid in modern physics even though Newton’s laws are not ! Conservation Laws Momentum and Kinetic Energy Examples outside physics Two Different Measures of Motion (May be not be exact like physical laws) • Momentum (vector) • Example in Lightman - Child’s blocks p = m v • Momentum for one particle • Even if blocks disappear, there may be ways to detect them and show the number does not change • Momentum for many particles p = Σ m v i • Money in your pockets i i • Conserved if you do not add or subtract Change in total can be related directly to income minus outgo • • Energy (scalar - no direction) 1 • Other KE = m |v| 2 2 • Kinetic Energy for a particle • ....... • Kinetic Energy for many particles 1 KE = Σ m |v | 2 2 i i i 1
Lecture 6 Conservation of Momentum Conservation of Momentum • As discussed previously, Newton’s 3rd Law (in • Momentum is conserved in both cases, even though conjunction with the 2nd Law) implies that the total in the both cases complicated things are going on momentum of two interacting objects is conserved the causes the cars to bounce or to stick together. ( ie does not change in time). • For an isolated system (no external forecs) • Air Track Demos: momentum is conserved no matter how complicated! • “Elastic Collision” of two equal mass objects: v 0 m m v 0 m m • “Totally Inelastic Collision” - equal mass objects: v 0 Rocket + fuel m m v 0 / 2 m m Momentum is conserved in both these cases, but the final motions are quite different. How do we Momentum is conserved in all these cases. understand the origin of this difference? Conservation of Momentum What about Energy? • Exercise - List examples • “Elastic Collision” of two equal mass objects: • For an isolated system (no external forces) m m momentum is conserved no matter how v 0 complicated! m m • Kinetic Energy: 2 same as After: (1/2)mv 0 • Put list on board Before: (1/2)mv 0 2 • “Totally Inelastic Collision” - equal mass objects: v 0 m m v 0 / 2 m m • Kinetic Energy: Before: (1/2)mv 0 2 (1/2)(2m)(v 0 /2) 2 = (1/4)mv 0 After: 2 Kinetic Energy NOT the same after collision! Conservation of Energy Conservation of Energy • Exercise - List examples First Law of Thermodynamics • For an isolated system (no exchange with the rest of • Total energy is conserved - this is even more basic the world ) energy is conserved no matter how than Newton’s laws complicated! • Types of energy • Holistic Law • Put list on board • Energy comes in many forms. One form can be converted into another, but the total never changes! • Kinetic energy: energy of motion Potential energy: Stored energy (due to gravity, compressed springs, batteries, chemical reactions, . . . .) Heat: Hotter objects contain more energy Other: Nuclear, . . . . (Later we will see that Einstein showed a different • interpretation of this idea, but nevertheless the conservation law still applies!) 2
Lecture 6 Gravitational Potential Energy Conservation of energy (continued) • How do we describe freely falling bodies in terms of energy? • The conservation of energy is one of the most • Initially, if released from rest, there is NO kinetic energy. important laws in physics - One of the most • When the body falls, the kinetic energy increases. important for society as well! • Where does this energy come from? What has changed? Only • Energy is the engine of modern society the position of the body with respect to the surface of the Earth! • Conversion of energy from one form to another is • Define the gravitational potential energy of mass the “infrastructure” of nations m near the surface of the Earth as: Oil to kinetic energy Potential Energy = mgh • Gravity to lights in your home • where h = height of mass above some reference point ( e.g. floor) Sun’s energy to food • • All uses of energy have some loss - to friction - that • As the mass falls, its potential energy is converted wind up as heat to kinetic energy. This energy can be recovered! • Reducing losses (for example by thermal insulation, • Works for complex problems - like roller coasters efficient motors, . . .) is a key goal for the future Gravitational Potential Energy Gravitational Potential Energy & Kinetic Energy • Example of conservation of energy • Assume the energy is all gravitational or kinetic energy. • Derivation of formulas for conservation of energy • That is we assume there is no input from an engine, no loss to • Assume the energy is all gravitational or kinetic energy. heat or other conversion of energy to other forms • We know • Use conservation of E = mgh + ½ m v 2 v f – v i = g(t f – t i ) and h i – h f = ½ (v f + v i )(t f – t i ) 2 – v i • Thus h i – h f = ½ (v f + v i )(v f – v i )/g = (v f 2 )/2g 2 – v i And mg(h i – h f ) = ½ m (v f 2 ) h i • If v= 0 at h = h top , • Then E = mgh i + ½ m v i2 v i what is v at h = h top - 1 m? = mgh f + ½ m v f2 • What is v at h = h top - 2 m? 1 m • PE + KE is conserved v f h f 2 m Other types of Potential Energy Work • Work is the transfer of energy by force acting on • A compressed (or extended) spring an object that is displaced For a high quality spring essentially all the energy • Work is a form of energy: conservation of energy required to deform it can be recovered - i.e., it is means that the energy of a system increases by useful potential energy the amount of work done on it • Example: it takes work to raise a body and • Twisted rubber band increase its potential energy • Work is needed to raise a roller coaster to the top • Bending of the bow which transfers energy to the • Formula: W = F x cos( θ ) arrow • W = 0 for force perpendicular to displacement (such as the effect of a centripetal force on a body moving in a circle) • W = Fx for force parallel to displacement 3
Lecture 6 Work Solving problems using Cons. of Energy • Formula: W = F x cos( θ ) • The Lever Principle (Lightman) • W = 0 for force perpendicular to displacement (such as the effect of a centripetal force on a body moving in a circle) • W = Fx for force parallel to displacement Displacement 3 1 Displacement 1 Force 1 m Force 2 m Work to raise ball 2 m is Work =0 to keep ball moving W = mg(2m) in circle at constant v Heat First law of thermodynamics • Conservation of Energy is The First Law • Heat is a form of energy – internal energy of a material made up of atoms in motion • Heat was very important in generalizing the (Atoms? More about them later) conservation law to ALL forms of energy Hotter Colder Hotter Colder • Heat is due to motions of atoms in random directions - cannot be completely channeled into • Heat is not obviously visible like mechanical useful work motion of a large object • Why? This brings in new concepts and the second law of thermodynamics – Next time. • Julius Meyer is credited with formulating the law as conservation of all forms of energy • Friction causes conversion of mechanical energy to heat. Exercise: Cons. of Energy Conservation of Energy: Roller Coaster • An automobile of mass 2000 kg goes from rest Energy at top = mgh + (1/2) mv 2 + Heat energy to 30 m/s on a level road. Work done by Engine to lift cars • What is the change in kinetic energy? • This kinetic energy is transformed from another form of energy. What is that form? • The car moving at 30 m/s now starts up a hill. If no energy is supplied by the engine, what is Potential energy largest = mgh the maximum height to which the car can Brakes convert remaining Kinetic energy largest = 1/2 mv 2 Kinetic energy to heat coast. 4
Recommend
More recommend