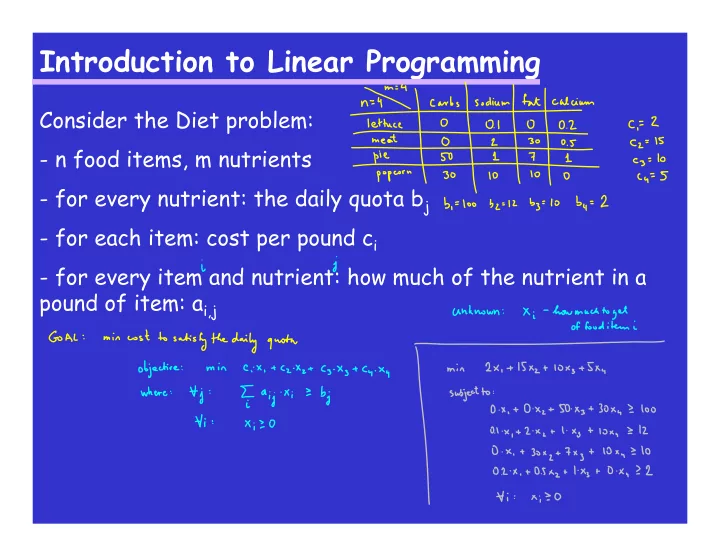
Introduction to Linear Programming Consider the Diet problem: - n - PowerPoint PPT Presentation

Introduction to Linear Programming Consider the Diet problem: - n food items, m nutrients - for every nutrient: the daily quota b j - for each item: cost per pound c i - for every item and nutrient: how much of the nutrient in a pound of item: a i,j

Introduction to Linear Programming

Introduction to Linear Programming A linear program looks like this: Find x 1 , x 2 , …, x m which • maximize c 1 x 1 + c 2 x 2 + … + c m x m • and satisfy these constraints: a 1,1 x 1 + a 1,2 x 2 + … + a 1,m x m · b 1 a 2,1 x 1 + a 2,2 x 2 + … + a 2,m x m · b 2 … a n,1 x 1 + a n,2 x 2 + … + a n,m x m · b n

Introduction to Linear Programming A linear program in compressed form: Given a vector c in R m , a vector b in R n and a matrix A in R n x m , find a vector x in R m which satisfies xA T · b and maximizes cx T . Thm: Exists a polynomial-time algorithm solving linear programs. Caveat: Sometimes need integer programs (no algorithm for integer programs is likely to exist) !

Introduction to Linear Programming Modeling via LP: Shortest paths

Introduction to Linear Programming Modeling via LP: Vertex Cover