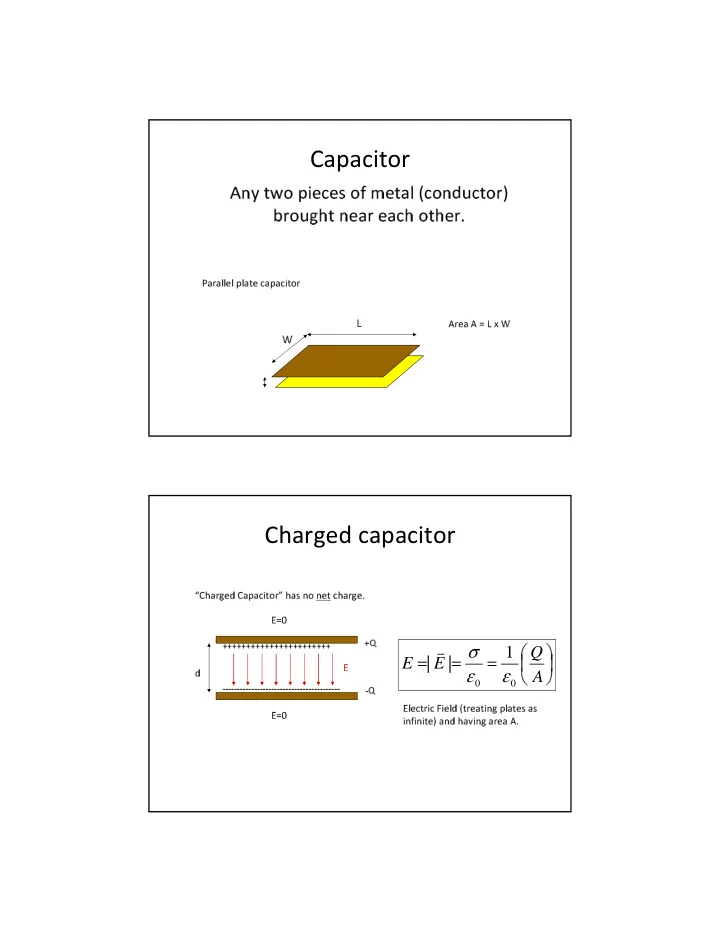
Capacitor Any two pieces of metal (conductor) brought near each - PDF document

Capacitor Any two pieces of metal (conductor) brought near each other. Parallel plate capacitor L Area A = L x W W Charged capacitor “Charged Capacitor” has no net charge. E=0 +Q σ 1 Q +++++++++++++++++++++++ ⎛ ⎞ = = = E | E | v ⎜ ⎟ E ε ε A ⎝ ⎠ d 0 0 ----------------------------------------- -Q Electric Field (treating plates as E=0 infinite) and having area A.

Capacitance σ 1 ⎛ Q ⎞ = = E ⎜ ⎟ +Q +++++++++++++++++++++++++++ ε ε A ⎝ ⎠ d 0 0 = ∆ = ⋅ = ---------------------------------------------- -Q V V E v d r Ed v ∫ Q → = V ε ( A d ) 0 Q C ≡ We define “capacitance” V ε A ≡ C 0 For parallel plate capacitor d Capacitance Can think of capacitor as a device for storing charge Q C ≡ (and also energy). V Units [Capacitance] = [Coulomb/Volt] = [Farad]

CPS Question A parallel-plate capacitor has square plates of edge length L, separated by a distance d. If we doubled the dimension L and halve the dimension d, by what factor have we changed the capacitance? L L A) 1.0 B) 2.0 C) 4.0 d D) 8.0 E) 16.0 ( ) 2 ε ε ε 2 2L ε 2 A L L = = → o = C o o 8 o d d d / 2 d CPS question ε A ≡ 0 C How big is a one Farad Capacitor? d Assume you have two parallel plates that are separated by 1 mm. If you estimate ε 0 ~ 10 -11 , what is the area of the plates to have a 1.0 Farad capacitance? A) 100,000,000 square meters B) 1,000 square meters C) 1 square meters D) 0.1 square meters E) 0.001 square meters

Commercial capacitors Micro-farad capacitors in small packages are made by making d very small ~ atomic dimensions. Energy Storage Capacitor stores both charge and energy. Suppose the capacitor is not fully charged. How much work is required to move a little bit +dq across? +Q +++++++++++++++++++++++++++ = = = Work dW dU Vdq dq ⎛ q ⎞ = ---------------------------------------------- -Q dU ⎜ ⎟ dq C ⎝ ⎠ Each next piece +dq gets harder Q 2 ⎛ q ⎞ Q and harder. = = = U dU ⎜ ⎟ d q ∫ ∫ C 2 C ⎝ ⎠ 0 2 1 Q 1 1 Potential Energy stored in the = = 2 = U CV QV capacitor. 2 C 2 2

CPS Question A parallel plate capacitor is charge up (+Q on one plate and –Q on the other). The plates are isolated so the charge Q cannot change. The plates are then pulled apart so that the plate separation d increases. The total electrostatic energy stored in the capacitor? A) Increases +Q +++++++++++++++++++++++ 2 1 Q 1 1 B) Decreases = = = 2 U CV QV d E C) Remains constant 2 C 2 2 ----------------------------------------- -Q Electric Field energy Where is this energy U? Answer = the electric field has energy. ε 1 A ⎛ ⎞ 1 ( ) 2 0 Ed 2 ⎜ ⎟ CV energy U 2 d ⎝ ⎠ 2 = = u Energy density of E-field = = = volume Vol . ⋅ Vol . A d 1 = ε 2 E 0 2 Turns out work to charge capacitor is equal to energy stored. This is valid for any electric field configuration in vacuum: “empty” space contains energy!!!

Spring analogy +Q +++++++++++++++++++++++++++ ---------------------------------------------- -Q 1 kx 1 1 ⎛ ⎞ U = 2 = 2 U Q ⎜ ⎟ 2 2 C ⎝ ⎠ Electric field energy Elastic energy Applications What is this device?

Applications (cont) Capacitors are good for storing large amounts of energy, which can then be accessed quickly (e.g. camera flash). Capacitors are also ideal transducers. Devices that covert physical quantities into electrical signals (e.g. computer keyboard, elevator buttons). A ε A ≡ C 0 d d Circuits with capacitors Circuit symbol for a capacitor More than one capacitor?...

Capacitors in parallel = = = V V V V 1 2 ab → = = Q C V ; Q C V 1 1 2 2 ( ) = + = + Q Q Q C C V total 1 2 1 2 Q → = + C C 1 2 V → = + C C C eq 1 2 Capacitors in parallel (cont) Think of two capacitors in parallel as adding the area of the two capacitors Many capacitors in parallel: = + + + C eq C C C ... 1 2 3

Capacitors in series = Q Q 1 2 Q Q → = = = = V V ; V V ac 1 cb 2 C C 1 2 ⎛ ⎞ 1 1 = = + = ⎜ + ⎟ V V V V Q ⎜ ⎟ ab 1 2 C C ⎝ ⎠ 1 2 V 1 1 1 → = = + Q C C C eq 1 2 Capacitors in series (cont) 1 1 1 = + C C C eq 1 2 or 1 = C eq 1 1 + C C 1 2 Many capacitors in series: 1 1 1 1 = + + + ... C eq C C C 1 2 3

CPS question A bank of three capacitors (C 1 , C 2 , C 3 ) is connected as follows. C 2 =1 F What is the effective total capacitance of the bank? A) C = 0 Farad B) C = 1 Farad C) C = 1.5 Farad C 1 =2 F D) C = 2.0 Farad C 3 =1 F E) C = 0.5 Farad 19 Dielectrics What if the gap between the capacitor plates is not empty?

Dielectrics (cont) Without dielectric: C without = Q / V 0 = C Q / V With dielectric: < but V V 0 → > C C without Increases the capacitance!!! Dielectric constant C V = = K 0 Dielectric constant: C V 0 V = V 0 K With the dielectric present, the potential difference for a given charge Q is reduced by a factor K

Dielectric constants for some materials Induced charge and polarization

Polarization We assume that the induced surface charge density is directly proportional to the electric field magnitude in the material σ = E Without dielectric: 0 ε 0 σ − σ E = = E 0 induced With dielectric: K ε 0 ⎛ − 1 ⎞ From these we σ = σ ⎜ 1 ⎟ induced K obtain: ⎝ ⎠ Permittivity σ E = = E 0 We have found: ε K K 0 ε = ε K Defining: permittivity 0 σ = E We obtain: ε A A = = ε = ε C KC K and 0 0 Capacitance d d 1 1 = ε = ε 2 2 u K E E 0 2 2 Energy density Easy rule of thumb: replace ε by ε 0 in all the equations