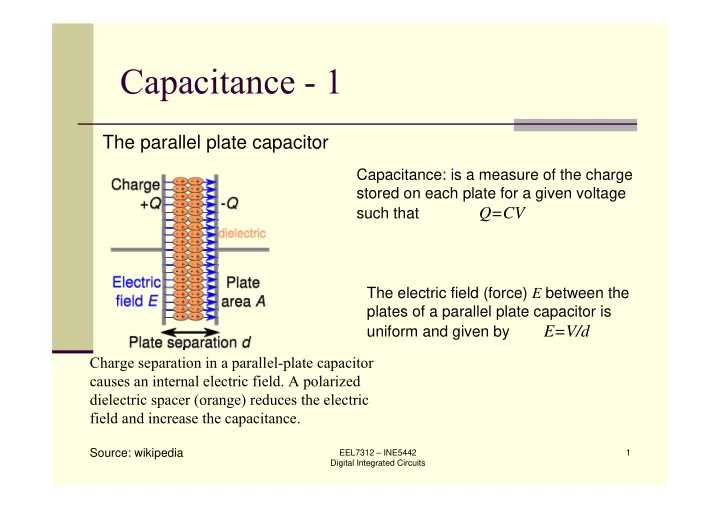
Capacitance - 1 The parallel plate capacitor Capacitance: is a - PowerPoint PPT Presentation

Capacitance - 1 The parallel plate capacitor Capacitance: is a measure of the charge stored on each plate for a given voltage such that Q=CV The electric field (force) E between the plates of a parallel plate capacitor is uniform and given by E=V/d Charge separation in a parallel-plate capacitor causes an internal electric field. A polarized dielectric spacer (orange) reduces the electric field and increase the capacitance. Source: wikipedia EEL7312 – INE5442 1 Digital Integrated Circuits

Capacitance - 2 Current flow L Electrical-field lines W H t di Dielectric Substrate ε ε = 2 = fF/ μ m ox C di c WL ox t int t ox Defined by foundry di Source: Rabaey EEL7312 – INE5442 2 Digital Integrated Circuits

Capacitance - 3 Source: Rabaey EEL7312 – INE5442 3 Digital Integrated Circuits

Capacitance - 4 Fabrication Gate oxide Capacitance process thickness / area (fF/ μ m 2 ) (CMOS) (nm) AMIS 1.5 32 1.1 μ m IBM 0.25 6.3 5.5 μ m IBM 0.13 3.2 11 μ m Source: MOSIS Source: Intel Tech. Journal EEL7312 – INE5442 4 Digital Integrated Circuits

Capacitance - 5 = = = ; / ( )/ Q CV I dQ dt d CV dt = / I CdV dt for constant capacitance For constant V → I =0, i.e. a capacitor behaves as an open circuit at I + dc. V Capacitors are energy-storage (memory) devices used in filters, - oscillators, power sources. Ideal capacitors are not dissipative (and not noisy) but charging and discharging them causes heating through dissipative devices connected to the capacitors. EEL7312 – INE5442 5 Digital Integrated Circuits

The RC circuit - 1 + V R - = = / / KCL I CdV dt V R = + / C R I + V RCdV dt V R = + V V V S C C V S + KVL V C - S C R C - Assume that V S = 0 for t <0, V S = A for t ≥ 0 (and V C (0)=0). ( ) = ⎡ − − τ ⎤ ≥ 1 exp / 0; V A ⎣ t ⎦ t t d C = < 0 0 50% V t C ( ) = = − τ ≥ / exp / / 0 I CdV dt A t R t C t d = τ ln2 ≅ 0.69 τ τ = RC EEL7312 – INE5442 6 Digital Integrated Circuits

The RC circuit - 2 ( ) ( ) + V R - = − − t τ = − τ ≥ ⎡ ⎤ exp / / 0 1 exp / V A I A t R t ⎣ ⎦ C I + The power dissipation p (electric power converted into R V S + V C heat) in the resistor is - C 2 exp ( ) - = 2 = − τ 2 / / p RI A t R The energy converted into heat in the resistor is Assume that V S = 0 for t <0, ∞ ∞ 2 2 ⎛ 2 ⎞ A t CA ∫ ∫ V S =A for t ≥ 0 (and V C (0)=0). = = − = exp ⎜ ⎟ E pdt dt τ R ⎝ ⎠ 2 R 0 0 The energy stored in the capacitor (for t →∞ ) 2 2 CV CA E = = C C 2 2 Exercise: (a) Using the energy conservation principle calculate the energy delivered by the source. (b) Calculate the energy E S delivered by the source using the formula below ∞ = ∫ E V Idt S S 0 EEL7312 – INE5442 7 Digital Integrated Circuits

Simulation 4.2 RC1 + V R - 1 2 * this is RC1.cir file I + v0 1 0 dc 0 pulse 0 1V 0 10ps 10ps 10ns 20ns R V S + R 1 2 1k V C - C C 2 0 1p - 0 .end EEL7312 – INE5442 8 Digital Integrated Circuits

Exercise 4.2 Run SpiceOpus to determine the voltages at the intermediate nodes 2 and 3 for the stimulus of simulation 4.2 2 1 3 R= 1 k Ω I R/ 2 R/ 2 V S + - C= 1 pF C/ 2 C/ 2 0 SpiceOpus (c) 1 -> source RC2.cir SpiceOpus (c) 2 -> tran 0.1ns 20ns SpiceOpus (c) 3 -> setplot new New plot V (3) Current tran1RC2 (Transient Analysis) V (2) const Constant values (constants) SpiceOpus (c) 4 -> plot v(1) v(2) v(3) xlabel time[s] ylabel Outputs[V] EEL7312 – INE5442 9 Digital Integrated Circuits

Comparison between exercises 4.1 and 4.2 2 1 3 4 1 I R= 1 k Ω I R/ 2 R/ 2 R V S + V S + - - C C/ 2 C= 1 pF C/ 2 0 0 V(3) V(4) EEL7312 – INE5442 10 Digital Integrated Circuits

Capacitance - 6 Fringing Capacitance w= W-H/2 W H t di thick oxide capacitance/unit length substrate (a) H W - H/2 + (b) Source: Rabaey EEL7312 – INE5442 11 Digital Integrated Circuits

Capacitance - 7 Interwire Capacitance Crosstalk: a signal can affect another nearby signal. fringing parallel Substrate noise coupling Source: Rabaey EEL7312 – INE5442 12 Digital Integrated Circuits

Capacitance - 8 Wiring Capacitances (0.25 μ m CMOS) aF/ μ m 2 aF/ μ m Source: Rabaey EEL7312 – INE5442 13 Digital Integrated Circuits

Exercise 4.3 Estimate the capacitance of the wires specified below: 1. Polysilicon, W= 0.25 μ m, L=1 mm; 2. Polysilicon, W= 0.25 μ m, L=10 mm; 3. Metal 1, W= 0.25 μ m, L=1 mm; 4. Metal 1, W= 0.25 μ m, L=10 mm. In each case, calculate the delay time assuming a lumped RC model for the wire and the capacitance with the substrate. Assume that the sheet resistances for polysilicon (with silicide) and metal 1 are 5 Ω and 0.1 Ω , respectively. ⎛ ⎞ ⎛ ⎞ C C = = ; fringe PP ⎜ ⎟ ⎜ ⎟ C WL C L PP fringe ⎝ ⎠ ⎝ ⎠ area length C C L = = 2 88 aF/ μ m fringe 54 aF/ μ m PP W area length L = + = H R R W C C C ฀ wire PP fringe wire thick oxide ≅ 0.69 substrate t R C d wire wire Source: Rabaey EEL7312 – INE5442 14 Digital Integrated Circuits

Exercise 4.3 - Answer 1. C wire =76 fF, R wire = 20 k Ω , R wire C wire = 1520 ps, t d = 0.69R wire C wire =1050 ps 2. C wire =760 fF, R wire = 200 k Ω , R wire C wire = 152 ns, t d = 0.69R wire C wire =105 ns 3. C wire =47.5 fF, R wire = 400 Ω , R wire C wire = 19 ps, t d = 0.69R wire C wire =13 ps 4. C wire =475 fF, R wire = 4 k Ω , R wire C wire = 1.9 ns, t d = 0.69R wire C wire =1.3 ns Note that the delay time increases proportionally with the square of the wire length. Why? So far we have considered that the distributed RC line can be represented by a lumped RC model (pessimistic view) and that the drive signal is a step supplied by an ideal voltage source (optimistic view). EEL7312 – INE5442 15 Digital Integrated Circuits

RC delay - 1 Influence of the output resistance of the driver V out Example: R driver =100 k Ω , c wire and 1- μ m-wide, 10-mm- Driver long Al1 wire. What’s t pd ? C C = = 2 30 aF/ μ m fringe 40 aF/ μ m PP area length R wire << R driver ⎛ ⎞ ⎛ ⎞ C C = = = = 0.3 pF; fringe 0.4 pF ⎜ PP ⎟ ⎜ ⎟ C WL C L R PP ⎝ ⎠ fringe ⎝ ⎠ area length driver V out = + = 0.7 pF C C C wire PP fringe ≅ t ≅ 0.69 50 ns t R C V in d driver wire d C lumped What’s the approximate maximum operating frequency of the input such that the output can detect the correct value of the input? Source: Rabaey EEL7312 – INE5442 16 Digital Integrated Circuits

RC delay – 2: The Elmore delay -1 Elmore delay model *– method to determine the approximate delay time in an RC network; it avoids running costly simulations for calculation of delay time. Useful for determining delays in transmission lines, gates, clock distribution networks,… Sources: Rabaey & *W. C. Elmore, “The transient response EEL7312 – INE5442 17 of damped linear networks with particular regard to wideband Digital Integrated Circuits amplifiers,” J. Applied Physics, vol. 19, Jan 1948

RC delay – 3: The Elmore delay -2 τ = + + + + = R C R C R C R C R C 1 1 2 2 3 3 4 4 Di i i i i ii i ( ) path s → i + + + R ii =R 1 +R 3 +R i R R R C 1 3 i i + path s → 1 R i1 =R 1 R C 1 1 + path s → 2 R i2 =R 1 R C 1 2 ( ) + + path s → 3 R i3 =R 1 +R 3 R R C 1 3 3 ( ) + + path s → 4 R i4 =R 1 +R 3 R R C 1 3 4 Sources: Rabaey & *W. C. Elmore, “The transient response EEL7312 – INE5442 18 of damped linear networks with particular regard to wideband Digital Integrated Circuits amplifiers,” J. Applied Physics, vol. 19, Jan 1948