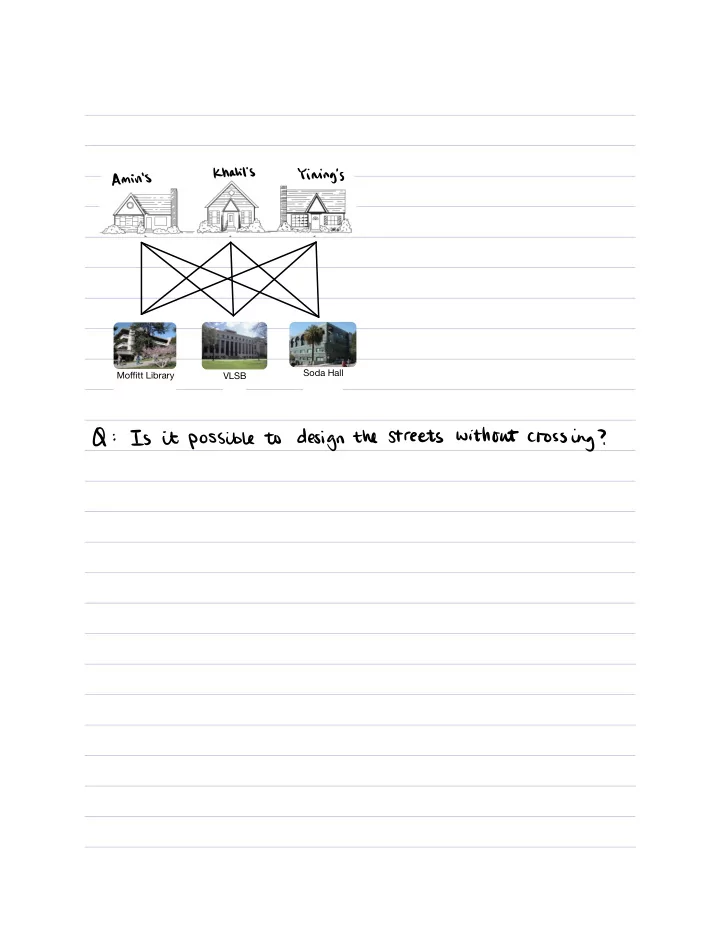

Khalil's Yining's Amin's Soda Hall Mo ffi tt Library VLSB it possible to design the streets without crossing Q Is
1 Planar Graphs IDefI A graph is called plane if it can be drawn in the plane without any edges crossing Such a drawing is called a planar representation of the graph v f e EI no 3 2 I t 2 3 Lf 3 t 2 3 go.IT I 4 4 6 2 HHMI Euler's Formula G is a connected planar graph Let V vertices edges e a planar representation of G faces in f e t f Then v 2 We'll do induction on PI E E 0 1 Pease O I 2 Consider G connected has e edges Tneduaintep If G is tree then V f e ti I e t l Since ett 2 the formula holds
To If CT is not a tree there's a cycle E Take any cycle and remove an edge from it The resulting graph has V vertices e 1 edges and f t faces e tf le Dt Cf D By IH V V 2 B v f e ti t th f things tf DY 2dim Ree 2 O dim I dim things things Does 2 feel unnatural shape of Ri U 003 It comes from the surface of a sphere Similarly different integers can be assigned to different surfaces For example take a square e if V 4 e 4 f I v 4 4 1 1 go.BZ 0 a is donut surface torus Or take a v a s In F I e t f p f I 21 1 2 I v e 0 And This notion generalizes to higherdimensional objects bo b t b z by t X Euler characteristic
1 1 Sparsity etf 2w µ Cor For a connected planar graph we have is ee 3 v 6 Define the degree of a face to be the edges on the PI boundary of the face e g 9 BRI AII.EE 3 deg IFI 5 degCFi o Then EE.de Fi 2 Since 33 for any E 2e 33 deg CFI f e 5 e V etf f 2 By planarity 2 e v v e Ee 7 Zte E 3 V G e T Rene This corollary says planar graph has few edges Is Leggs planar planar e e 3 v 6 EI 3 V 6 3 6 6 a co e 9 12 e E 3 V G We don't know
Is Ks planar 3V 6 e 10 3 5 6 9 T.IE e 3v 6 non planar ICorI For a connectednoon planar bipartite graph with v 33 we have e E ZU 4 HIT tf PI dg 2 Similarly 34 for alli Now deg Fi 8t4e 45 4 e Zte v Kv 83 2e w e E 4 B Is Ks 3 non planar EI e g 2 6 W 4 8 4 2V 4 E K 3,3 is nonplanar
1 2 Kuratowski's Theorem C IDefI An operation on G by removing an edge u v and w together with edges new vertex adding a u w v w is an dementarysub division GTF Tf Remi If G is planar after performing an elementary subdivision on G CT remains planar IDefI G and Ga are if they can be obtained from the same graph by a sequence of elementary subdivisions EI I TTT 1Thm A graph is nonplanar if and only if it contains a subgraph homeomorphic to kz.rs or Ks The Peterson Graph is non EI planar
2 Graph Coloring e g vertices students coloring edges friends breakout room IDefI A coloring of a graph G is the assignment of a color no two adjacent vertices are to each vertex such that assigned the same color The chromaticnumber X G is the least number of colors needed for a coloring of this graph EI gII2o XC G 4 ooo p G is a planar graph E 6g Eudegat X G LProp vertices PI eE3V totalidegee 2e E lov 12 Since 6 6V 6 ku 26 average degree F ut V E5 s t degCV We'll now do induction on Ivf Bang 14 X G L 1 Inductivestep Remove a vertex 1 with degree E5 a 6 By IH the resulting subgraph G has XCG Color CT using E 6 colors 3 Ci CzCz Cox Cs Co Now color V Since deg u e5 there's an available color among 4 gg
5 color Theorem G is a planar graph X G IThm E5 Again we'll do induction on IV l PI 14 1 X G Bates L Remove a vertex V with deg E 5 Induc tivestep color the resulting CT using By IH gci Cs Now need to color v neighbors of V don't use up all five colors color If a remaining color using neighbors of v use up 94 If G 3 C Planar representation qq.cs.no Cy sis soon a TITA Ks a d 11 so no valid coloring for this vertex Either Yo D color Theorem CT is a planar graph hm.TL 4 l Xl G say Remi 5 colortheorem was proven in 18005 19 76 4 color theorem was proven in Remi 4 color theorem tells us 4 colors are enough to colormaps o.nu graph map t.EE a
Recommend
More recommend