Probing Axion-like Particles via CMB Polarization Collaborators: - PowerPoint PPT Presentation

1 Probing Axion-like Particles via CMB Polarization Collaborators: Tomohiro Fujita, Yuto Minami, Kai Murai, arxiv:2008.02473 Speaker: Hiromasa Nakatsuka ICRR, The University of Tokyo 2 nd year PhD student PPP,2020-09-03

2 Axion n QCD axion • Strong CP problem: C. A. Baker, et al. (2006) , by the electric dipole moment of neutron • One of solutions is QCD axion: n Axion-like particles by String Axiverse A. Arvanitaki, et al. (2009) “ String theory suggests the simultaneous presence of many ultralight axions ” • Axions have mass nonperturbatively, which is exponentially suppressed: " ∝ 𝜈 ! 𝑓 !& !"#$ 𝑛 % 𝑔 " David J. E. Marsh (2015) • Axion as Dark Matter: 10 !"" eV ≲ 𝑛 • Axion as Dark Energy: 𝑛 ≲ 𝐼 # ∼ 10 !$$ eV

3 Axion-like Particles n Axion-Photon coupling , 𝜚 :axion(ALP) • Axion-photon conversion By background B field: ('()) 𝐵 % 𝜚 𝐵 % • Rotation of polarization angle Observed polarization Polarization of Initial photon 𝜚 # 𝜚 $ ! ! ⃗ 𝛽 = " Δ𝜚 = " 𝜚 # − 𝜚 $ 𝐵 𝛽 ⃗ 𝐵 Δ𝜚 D.Harari&P.Sikivie (1992)

4 Axion-photon conversion n Axion Helioscope (e.g., CAST, CAST Collaboration (2005) ) solar axion flux 𝑪 Magnet coil X-ray x-ray detector n Axion Dark Matter eXperiment (ADMX) for Axion DM S.J. Asztalos, et al. (2009) • The microwave cavity for resonant conversion n X-ray space telescope: Chandra observatory M. Berg, et al. (2016) AGN of the Perseus cluster n o i x a magnetic field X-ray flux 𝑪 x-ray space telescope X-ray flux in galaxy cluster (Chandra)

5 Polarization rotation n Ground-based experiment: Laser technique • The background axion DM rotates the polarization angle of laser. • Laser cavity can detect the small rotation angle. H. Liu, et al. (2018), I. Obata, et al. (2018), K. Nagano, et al. (2019) n Astronomical source (e.g. proto-planetary disc) J.Hashimoto, et al. (2011), • Flattened gaseous object surrounding a young star • The background axion DM rotates the direction of scattering polarization. J. Hashimoto, et al. (2011), T. Fujita, et al. (2018), S. Chigusa et al. (2019) n Cosmological source: CMB S. M. Carroll (1998), A. Lue, et al. (1999), M. A. Fedderke, et al. (2019), G. Sigl&P. Trivedi (2018) • and This work

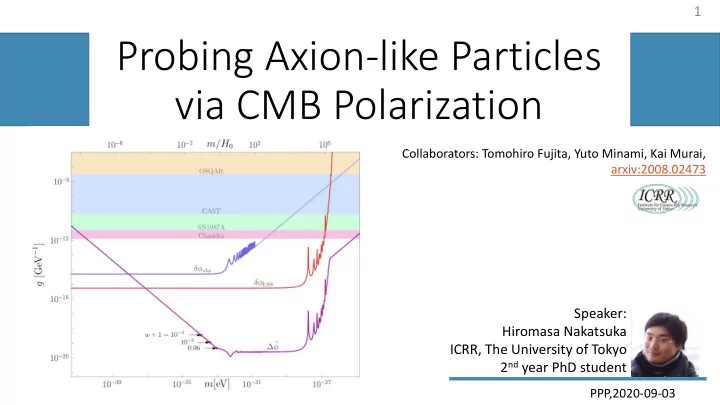
6 The constraints of axion-photon coupling This work M.Berg, et.al. (2016)

7 Cosmic Birefringence n CMB polarization E-mode B-mode Axion induces Parity Even Parity Odd EB-correlation n Cosmic Birefringence S.M.Carroll (1998), A. Lue, et.al. (1999) ● Last Scattering Surface(LSS): 𝑢 #$$ ∼ 3.8×10 % yr ● Observer: 𝑢 & ∼ 13.8×10 ' yr uncorrelated E&B-mode correlated E&B-mode 𝜚 ① anisotropic rotation 𝜚 345 (direction dependent) . 𝜚 677 𝑜 ≡ − ! . 𝛽 , " 𝜀𝜚 #$$ (, 𝑜) 𝜚 345 ② isotropic rotation 𝛽 ≡ ! 𝑦 " ( 2 𝜚 %&' − 2 𝜀𝜚 #$$ (, 𝑜) 𝑦 2 𝜚 #$$ + 𝜀𝜚 %&' ) 𝜀𝜚 %&'

8 Field Dynamics ・ >𝜚 #$$ = 2 𝜚 𝑢 #$$ + 𝜀𝜚 #$$ 𝑢 #$$ , , 𝑜 n Birefringence by Δ ) 𝜚, 𝜀𝜚 456 and 𝜀𝜚 788 𝜚 %&' = 2 𝜚 𝑢 ( + 𝜀𝜚 %&' 𝑢 ( , 𝑦 = 0 • Potential term : V 𝜚 = 8 " 𝑛 " 𝜚 " ・ 𝐼 ( : (current Hubble parameter) • Background motion : Δ 2 𝜚 ≡ 2 𝜚 𝑢 ( − 2 𝜚 𝑢 #$$ , constant (𝑛 < 𝐼 𝑢 ) 9 ・ Dynamics ： 𝜚 𝑢 ∝ < 𝑏 𝑢 ( ! " sin 𝑛𝑢 𝐼 𝑢 < 𝑛 */" , Ω ! ∼ # 0.7 m ≲ 𝐼 " ・ Amplitude ： | 2 𝜚| ∝ Ω ) R.Hlozek, et.al.(2015) 0.01 (𝐼 " ≲ m ≲ 10 #$% eV) • Perturbation: 𝜀𝜚 )*+ & 𝜀𝜚 #$$ , 𝑠 : tensor to scalar ratio, we use 𝑠 = 0.06 ・ 𝑜 ≡ − K ① anisotropic rotation (direction dependent ) : 𝛽 1 " 𝜀𝜚 677 (1 𝑜) 𝛽 ≡ K " (Δ . ② isotropic rotation : . 𝜚 + 𝜀𝜚 345 )

9 Field Dynamics n Fluctuation at observer: 𝜀𝜚 456 The Fourier mode O 𝜚 , • 𝜚 677 𝜚 345 (- , 𝜚 #$$ ≃ 𝜚 )*+ For 𝑙 < 𝑒 #$$ (- , 𝜚 #$$ ≠ 𝜚 )*+ For 𝑙 > 𝑒 #$$ 𝑒 #$$ , • #( #( 𝑒 &'' 𝑒 &'' n Damping effect by the width of LSS ● Last Scattering Surface(LSS): 𝑢 #$$ ∼ 3.8×10 % yr ● Observer: 𝑢 & ∼ 13.8×10 ' yr present < LSS > For 𝑛 > 10 (". eV, 𝜚 oscillates at LSS: • , visibility function:

10 Sensitivity n Current sensitivity from Planck, SPTpol & ACTPol N. Aghanim, et al. (2016), F. Bianchini, et al. (2020), T. Namikawa, et al. (2020) 𝑜 ≡ − ! ① anisotropic rotation (direction dependent ) : 𝛽 , " 𝜀𝜚 #$$ (, 𝑜) -- ≡ 1∗ , * ∗ ",.* ∑ / 𝑏 - ,/ 𝑏 - ,/ 𝐷 , , 𝑏 - ,1 ≡ ∫ dΩ 𝛽 , 𝑜 𝑍 𝑜 , • For flat power spectrum, ** 𝐵 - ≡ , *., 2 ) "3 • SPTpol & ACTPol 2020: 𝐵 - < 8.3×10 45 deg " (68%CL) 𝛽 ≡ ! ② isotropic rotation : 2 " (Δ 2 𝜚 + 𝜀𝜚 678 ) • Planck2016: 2 𝛽 < 0.6 ° (68%CL)

11 Sensitivity 𝐼 ( : (current Hubble parameter) n Current sensitivity from Planck & SPTpol N. Aghanim et al. 2016, F. Bianchini et al.2020, T. Namikawa et al. 2020 Damp by l Red line by 𝜀𝜚 788 oscillation For 10 !"U eV < 𝑛, 𝜚 oscillates during LSS, and the averaged rotation angle damps. l Purple line by Δ ) 𝜚 For 𝑛 < 𝐼 # , . 𝜚 does not roll down the potential, and Δ . 𝜚 ∝ 𝑛/𝐼 # oscillation during LSS l Blue line by 𝜀𝜚 456 not roll down For 𝐼 # < 𝑛, 𝜀𝜚 345 starts oscillating and damps.

12 Sensitivity 𝐼 ( : (current Hubble parameter) n Current sensitivity from Planck & SPTpol • Even if no BG axion Ω X → 0 , we ubiquitously have 𝜀𝜚 788 & 𝜀𝜚 456 from inflation. ∝ 𝑠 (-/" • 𝜀𝜚 677 :anisotropic birefringence • 𝜀𝜚 345 :isotropic birefringence (-/" ∝ Ω /

13 Future sensitivity Table in our paper, arxiv:2008.02473 • Here, 𝑠 = 10 45 in the reach of LiteBIRD

14 Discussion ! What if we detect ... ？ 𝛽 " 𝑜 ≡ − " 𝜀𝜚 #$$ (" 𝑜) ! " (Δ * 𝛽 ≡ * 𝜚 + 𝜀𝜚 %&' ) ① anisotropic birefringence ② Only isotropic (no anisotropic) birefringence ③ Only anisotropic (no isotropic) birefringence

15 Discussion ! What if we detect ... ？ 𝛽 " 𝑜 ≡ − " 𝜀𝜚 #$$ (" 𝑜) ① anisotropic birefringence by 𝜀𝜚 #$$ ∝ 9 + ! " (Δ * 𝛽 ≡ * 𝜚 + 𝜀𝜚 %&' ) "3 • we can fix “ 𝑕 " ×𝑠 ”, " "#$ Z ! ! d [.\×]^ %& _'` ' = a.a×]^ %() b'c %( ]^ %& Observable upper bound (e.g. Chandra) 𝑕 < 𝑕 :;< &'( X % 𝑠 > 5×10 !W ⇒ Y×8# )* Z[\ + ü CMB experiments can investigate 𝑠 from below by Birefringence! (and from above by the primordial GW )

16 Discussion ! What if we detect ... ？ 𝛽 " 𝑜 ≡ − " 𝜀𝜚 #$$ (" 𝑜) ② Only isotropic birefringence by 𝜀𝜚 456 or Δ ) 𝜚 ! " (Δ * 𝛽 ≡ * 𝜚 + 𝜀𝜚 %&' ) • Non-detection of 𝜀𝜚 677 means Δ . 𝜚 , not 𝜀𝜚 345 • 𝑕 has upper bound, then 0.3° < 𝑛 | . 𝛽| | . 𝛽| 10 !U < 10 U 𝐼 # 0.3° ü We can investigate the mass of axion DE, including very small Equation of State 𝑥 !

17 Discussion What if we detect ... ？ ! 𝛽 " 𝑜 ≡ − " 𝜀𝜚 #$$ (" 𝑜) ③ Only anisotropic birefringence ! " (Δ * 𝛽 ≡ * 𝜚 + 𝜀𝜚 %&' ) • Non-detection of 𝜀𝜚 345 means 1 ≲ 𝑛 𝐼 # • Non-detection of Δ . 𝜚 means 4* " =>? 𝛽 2 𝐵 - 𝑠 Ω ) ℎ " ≲ 2×10 4*5 4×10 45 deg " 0.05° 0.06 ü We can put a stringent constraint on the energy fraction of the axion! Ω / ∼ 0.01

Conclusion • Future CMB experiments investigates the broad range of axion-photon coupling, including • dark energy axion • axion with tiny energy fraction • Detection of birefringence provides valuable information; • through anisotropic birefringence, we can search small-scale inflation with 𝑠 > 5×10 !W . • through isotropic rotation, we can search tiny energy fraction of axion with Ω % ℎ " ≲ 2×10 !8$ . Detailed calculations in arxiv:2008.02473

19 Backup

20 About CMB observation 𝐷 12,4 = tan 4𝛽 5 + sin 4𝛽 𝐷 11,4 − 𝐷 22,4 𝐷 11,672 − 𝐷 22,672 2 2cos(4𝛽 5 ) 𝛽 5 : rotation of polarization sensitive detector 𝛽 : cosmic birefringence Y.Minami, et.al.(2019)