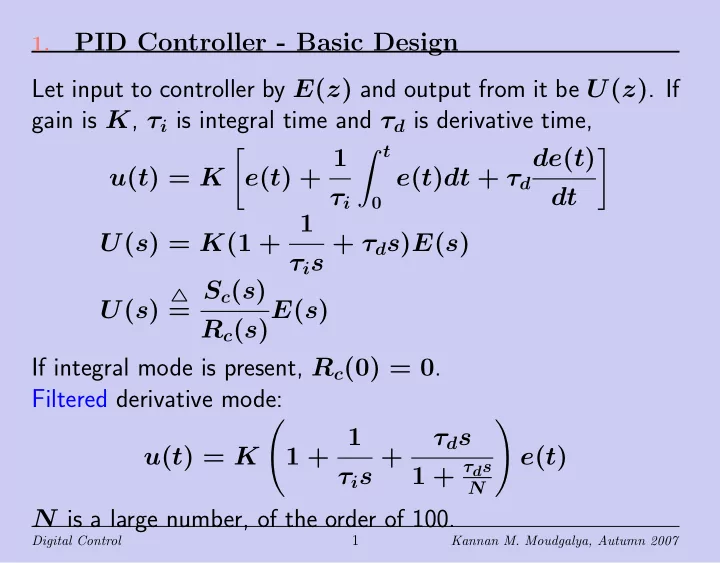
PID Controller - Basic Design 1. Let input to controller by E ( z ) - PowerPoint PPT Presentation

PID Controller - Basic Design 1. Let input to controller by E ( z ) and output from it be U ( z ) . If gain is K , τ i is integral time and τ d is derivative time, � t e ( t ) + 1 de ( t ) � � u ( t ) = K e ( t ) dt + τ d τ i dt 0 U ( s ) = K (1 + 1 τ i s + τ d s ) E ( s ) = S c ( s ) △ U ( s ) R c ( s ) E ( s ) If integral mode is present, R c (0) = 0 . Filtered derivative mode: � � 1 + 1 τ d s u ( t ) = K τ i s + e ( t ) 1 + τ d s N N is a large number, of the order of 100. 1 Digital Control Kannan M. Moudgalya, Autumn 2007

Derivative Mode 2. • Integral Mode using Tustin/Bilinear: 1 s ↔ T s z + 1 2 z − 1 • Derivative Mode – Reciprocal results in wildly oscillating control effort. Rect- angular approximation solves this problem. – Backward difference formula: 1 z s ↔ T s z − 1 – Forward difference formula: 1 T s s ↔ z − 1 – Both are used • Rectangular approximation is used for integral mode as well 2 Digital Control Kannan M. Moudgalya, Autumn 2007

2-DOF Controller 3. y r T c u G = B R c A − S c R c u = T c r − S c y R c R c It is easy to arrive at the following relation between r and y . y = T c B/A BT c r = r R c 1 + BS c /AR c AR c + BS c Error e , given by r − y is given by BT c r = AR c + BS c − BT c � � e = 1 − r AR c + BS c AR c + BS c 3 Digital Control Kannan M. Moudgalya, Autumn 2007

Offset-Free Tracking of Steps with Integral 4. Recall expression for E , showing the dependence on z : E ( z ) = A ( z ) R c ( z ) + B ( z ) S c ( z ) − B ( z ) T c ( z ) R ( z ) A ( z ) R c ( z ) + B ( z ) S c ( z ) z − 1 A ( z ) R c ( z ) + B ( z ) S c ( z ) − B ( z ) T c ( z ) z n →∞ e ( n ) = lim lim z A ( z ) R c ( z ) + B ( z ) S c ( z ) z − 1 z → 1 Because the controller has an integral action, R c (1) = 0 : e ( ∞ ) = S c ( z ) − T c ( z ) � = S c (1) − T c (1) � � S c ( z ) S c (1) � z =1 This condition can be satisfied if one of the following is met: T c = S c T c = S c (1) T c (1) = S c (1) 4 Digital Control Kannan M. Moudgalya, Autumn 2007

T c = S c : Offset-Free Tracking with Integral 5. y r T c u G = B R c A − u = T c r − S c y S c R c R c R c How do we obtain the standard configuration, with e = r − y ? v G c ( z ) = S c G ( z ) = B r e u y R c A − Answer: T c = S c . Substituting this in the above equation, u = S c ( r − y ) = S c e = T c e R c R c R c T c = S c ensures offset free tracking! 5 Digital Control Kannan M. Moudgalya, Autumn 2007

T c = S c : Offset-Free Tracking with Integral 6. • Recall: T c = S c results in offset free tracking and u = S c e = T c e R c R c • Precisely what is available from standard PID controller: U ( s ) = K (1 + 1 τ i s + τ d s ) E ( s ) • Recall approximations: Integral: 1 s ↔ T s z + 1 Derivative: s ↔ 1 z − 1 z − 1 , 2 T s z • Substituting, 1 + 1 T s z + 1 z − 1 + τ d z − 1 � � U ( z ) = K E ( z ) τ i 2 T s z 6 Digital Control Kannan M. Moudgalya, Autumn 2007

T c = S c : Offset-Free Tracking with Integral 7. Recall from the previous slide: 1 + 1 T s z + 1 z − 1 + τ d z − 1 � � U ( z ) = K E ( z ) τ i 2 T s z Cross multiplying and taking inverse Z-transform, obtain u ( n + 1) = u ( n ) + s 0 e ( n + 1) + s 1 e ( n ) + s 2 e ( n − 1) 1 + T s + τ d � � s 0 = K 2 τ i T s − 1 + T s − 2 τ d � � s 1 = K 2 τ i T s s 2 = K τ d T s Smooth transfer from manual to auto mode. Bumpless transfer. 7 Digital Control Kannan M. Moudgalya, Autumn 2007

PID Controller with Filtering and T c = S c 8. Recall filtered derivative mode: � � 1 + 1 τ d s u ( t ) = K τ i s + e ( t ) 1 + τ d s N Use backward approximation 1 z 1 s ↔ T s z − 1 = T s 1 − z − 1 for all: 1 + τ d s/N ↔ − NT s 1 r 1 1 + r 1 z − 1 τ d where τ d /N r 1 = − τ d /N + T s Control law becomes: 1 − z − 1 − Nr 1 (1 − z − 1 ) S c 1 + T s 1 � � = K 1 + r 1 z − 1 R c τ i 8 Digital Control Kannan M. Moudgalya, Autumn 2007

PID Controller with Filtering and T c = S c 9. 1 − z − 1 − Nr 1 (1 − z − 1 ) S c 1 + T s 1 � � = K 1 + r 1 z − 1 R c τ i = K (1 − z − 1 )(1 + r 1 z − 1 ) + T s /τ i (1 + r 1 z − 1 ) − Nr 1 (1 − z − 1 ) 2 (1 − z − 1 )(1 + r 1 z − 1 ) Comparing the denominator and the numerator, we obtain S c ( z ) = s 0 + s 1 z − 1 + s 2 z − 2 R c ( z ) = (1 − z − 1 )(1 + r 1 z − 1 ) with 1 + T s � � s 0 = K − Nr 1 τ i 1 + T s � � � � s 1 = K r 1 + 2 N − 1 τ i s 2 = − Kr 1 (1 + N ) 9 Digital Control Kannan M. Moudgalya, Autumn 2007

PID Controller with Filtering and T c = S c 10. Recall S c ( z ) = s 0 + s 1 z − 1 + s 2 z − 2 R c ( z ) = (1 − z − 1 )(1 + r 1 z − 1 ) PID control law is given by s 0 + s 1 z − 1 + s 2 z − 2 u ( n ) = S c e ( n ) = (1 − z − 1 )(1 + r 1 z − 1 ) e ( n ) R c Cross multiplying, we obtain the control law: (1 − z − 1 )(1 + r 1 z − 1 ) u ( n ) = ( s 0 + s 1 z − 1 + s 2 z − 2 ) e ( n ) On introduction of filtering action in the derivative mode, we lose the useful property of bumpless control action. 10 Digital Control Kannan M. Moudgalya, Autumn 2007

PD Controller with Filtering and T c = S c 11. Recall 1 − z − 1 − Nr 1 (1 − z − 1 ) S c 1 + T s 1 � � = K 1 + r 1 z − 1 R c τ i Do not want integral mode. Delete second term: 1 − Nr 1 (1 − z − 1 ) S c � � = K 1 + r 1 z − 1 R c = K (1 − Nr 1 ) + r 1 (1 + N ) z − 1 1 + r 1 z − 1 Discrete time PD control law is given by (1 + r 1 z − 1 ) u ( n ) = ( s 0 + s 1 z − 1 ) e ( n ) where, s 0 = K (1 − Nr 1 ) , s 1 = Kr 1 (1 + N ) . Not in incremental form. No integral action. 11 Digital Control Kannan M. Moudgalya, Autumn 2007

Setpoint, Derivative, Proportional Kicks 12. • The standard PID control law has a shortcoming. • If there is a sudden change in the setpoint, – both proportional and derivative modes will introduce large jumps in the control effort, known as setpoint kick. – The large change introduced by the derivative mode is known as derivative kick. – Proportional mode could also produce a large control ef- fort, known as proportional kick. – Both derivative and proportional kicks are generally not acceptable. • Solved in a feedback configuration, to be given in the next slide. 12 Digital Control Kannan M. Moudgalya, Autumn 2007

2-DOF PID Controller with no Setpoint Kicks 13. Recall PID control law with filtered derivative action: � � 1 + 1 τ d s u ( t ) = K τ i s + e ( t ) 1 + τ d s N With e ( t ) = r ( t ) − y ( t ) , it becomes, � � 1 + 1 τ d s u ( t ) = K τ i s + ( r ( t ) − y ( t )) 1 + τ d s N To remove setpoint kick, propose the following control law: � � K τ d s u ( t ) = � ( r ( t ) − y ( t )) − K 1 + y ( t ) 1 + τ d s 1 + τ d s � τ i s N N Setpoint kick removed. The exact structure to be explained. 13 Digital Control Kannan M. Moudgalya, Autumn 2007

2-DOF PID Controller with no Setpoint Kicks 14. Recall the control law from previous slide: � � K τ d s u ( t ) = � ( r ( t ) − y ( t )) − K 1 + y ( t ) 1 + τ d s 1 + τ d s � τ i s N N Grouping the terms involving y ( t ) , we obtain � � K τ d s 1 � r ( t ) − K u ( t ) = 1 + + y ( t ) 1 + τ d s 1 + τ d s 1 + τ d s � � � τ i s τ i s N N N This can be simplified as τ i τ d s 2 + 1 K � � u = τ i s (1 + τ d s/N ) r − K 1 + y τ i s (1 + τ d s/N ) In the form u = T c R c r − S c R c y , with T c (0) = S c (0) = K 14 Digital Control Kannan M. Moudgalya, Autumn 2007

2-DOF PID Controller with no Setpoint Kicks 15. Recall the control law that avoids setpoint kicks: � � K τ d s u ( t ) = � ( r ( t ) − y ( t )) − K 1 + y ( t ) 1 + τ d s 1 + τ d s � τ i s N N This can be implemented as, v r K u y G τ i s (1 + τ d s /N ) − − τ d s � � K 1 + 1 + τ d s/N Please read the rest of Chapter 8. 15 Digital Control Kannan M. Moudgalya, Autumn 2007