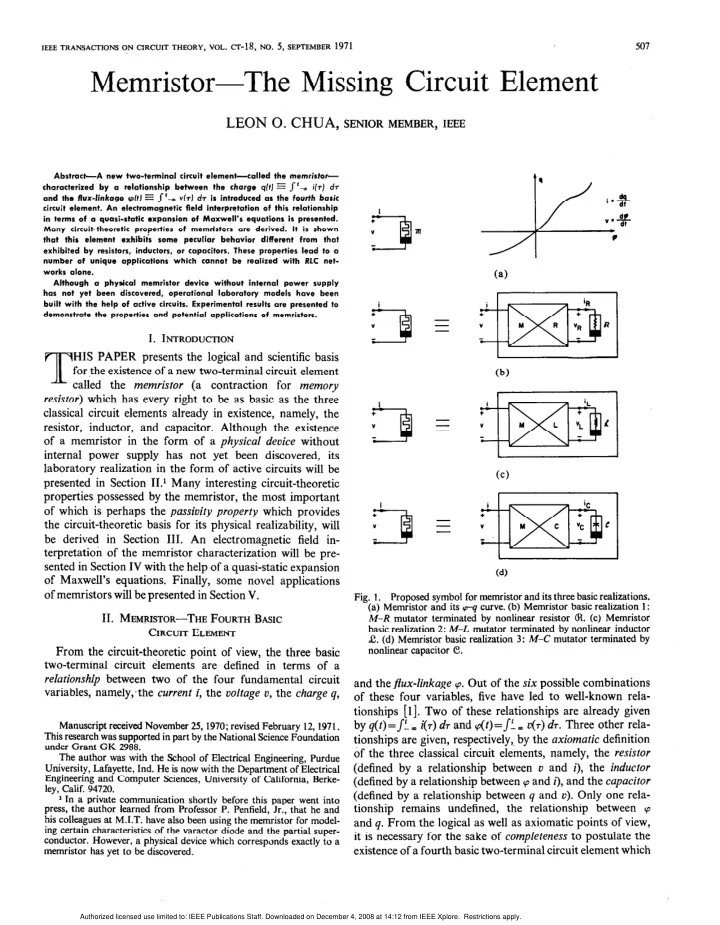

507 NO. 5, SEPTEMBER 1971 ,EEE TRANSACTIONS ON CIRCUIT THEORY, VOL. CT-18, Memristor-The Missing Circuit Element LEON 0. CHUA, SENIOR MEMBER, IEEE Abstract-A new two-terminal circuit element-called the memrirtor- i(7J d7 characterized by a relationship between the charge q(t) s St% flux-linkage (p(t) = J-‘-m vfrj d T is introduced os the fourth boric and the I circuit element. An electromagnetic field interpretation of this relationship + in terms of a quasi-static expansion of Maxwell’s equations is presented. ” nl Many circuit~theoretic properties of memdstorr are derived. It is shown -3 that this element exhibiis some peculiar behavior different from that exhibited by resistors, inductors, or capacitors. These properties lead to a RLC net- number of unique applications which cannot be realized with works alone. (a) Although a physical memristor device without internal power supply has not yet been discovered, operational laboratory models have been I + built with the help of active circuits. Experimental results ore presented to demonstrate the properties and potential applications of memristors. Y -3 I. 1NTR00~cnoN HIS PAPER presents the logical and scientific basis for the existence of a new two-terminal circuit element (b) T called the memristor (a contraction for memory 1 resistor) which has every right to be as basic as the three + classical circuit elements already in existence, namely, the ” resistor, inductor, and capacitor. Although the existence - -3 of a memristor in the form of a physical device without internal power supply has not yet been discovered, its laboratory realization in the form of active circuits will be (cl presented in Section II.’ Many interesting circuit-theoretic properties possessed by the memristor, the most important I + of which is perhaps the passivity property which provides ” - the circuit-theoretic basis for its physical realizability, will -3 be derived in Section III. An electromagnetic field in- terpretation of the memristor characterization will be pre- sented in Section IV with the help of a quasi-static expansion Cd) of Maxwell’s equations. Finally, some novel applications of memristors will be presented in Section V. Fig. 1. Proposed symbol for memristor and its three basic realizations. (a) Memristor and its q-q curve. (b) Memristor basic realization 1: II. MEMRISTOR-THE FOURTH BASIC M-R mutator terminated by nonlinear resistor &t. (c) Memristor basic realization 2: M-L mutator terminated by nonlinear inductor CIRCUIT ELEMENT C. (d) Memristor basic realization 3: M-C mutator terminated by From the circuit-theoretic point of view, the three basic nonlinear capacitor e. two-terminal circuit elements are defined in terms of a relationship between two of the four fundamental circuit and theflux-linkage cp. Out of the six possible combinations variables, namely;the current i, the voltage v, the charge q, of these four variables, five have led to well-known rela- tionships [l]. Two of these relationships are already given Manuscript received November 25, 1970; revised February 12,197l. by q(t)=JL w i(T) d 7 and cp(t)=sf. m D(T) d7. Three other rela- This research was supported in part by the National Science Foundation tionships are given, respectively,. by the axiomatic definition under Grant GK 2988. of the three classical circuit elements, namely, the resistor The author was with the School of Electrical Engineering, Purdue University, Lafayette, Ind. He is now with the Department of Electrical (defined by a relationship between v and i), the inductor Engineering and Computer Sciences, University of California, Berke- (defined by a relationship between cp and i), and the capacitor ley, Calif. 94720. (defined by a relationship between q and v). Only one rela- r In a private communication shortly before this paper went into tionship remains undefined, the relationship between 9 press, the author learned from Professor P. Penfield, Jr., that he and his colleagues at M.I.T. have also been using the memristor for model- and q. From the logical as well as axiomatic points of view, ing certain characteristics of the varactor diode and the partial super- it is necessary for the sake of completeness to postulate the conductor. However, a physical device which corresponds exactly to a existence of a fourth basic two-terminal circuit element which memristor has yet to be discovered. Authorized licensed use limited to: IEEE Publications Staff. Downloaded on December 4, 2008 at 14:12 from IEEE Xplore. Restrictions apply.
IEEE TRANSACTIONS ON CIRCUIT THEORY, SEPTEMBER 1971 508 TABLE I OF M-R, M-L, AND M-C CHARACTERIZATION AND REALIZATION MUTATORS = RANSMISSION MATRIX SYMBOL BASH: REALIZATIONS AND ‘PE USING CONTROLLED SOURCES ::I = [T(P’][J CHARACTERIZATION I w+gf REALIZATION 2 REALIZATION I il D---a il F--a- ‘2 + = [ 1 (2) + i2+ +(!%) -i2+ R “2 P 0 + + “2 I ~R,b) “2 I c 0 P (li,dt) Uqdt) - dVp “I= dt dip iI = -7 Y-R - REALIZATION 2 MUTATOR (q.Vl -RvR,iR) REALIZATION I 2 di2 VI’ -7 I REALIZATION I REALIZATION 2 gq-: I REALIZATtON 4 REALIZATION 3 Identical to TcR,(p) I +(!!k) i2+ “I = “2 f c Type I C-R MUTATOR 1 ’ : + di2 !TDq-y iI=- M-L v (VI)- “! MUTATOR i 1 L - (q,# -WL, iL) REALIZATION 2 REALIZATION I [ 1 0 P m : TML2fP” ) o Y 2 di, (Identical to TLR2(p) v, = - dt !I mf c Type 2 L-R MUTATOA i, =v2 .I I REALIZATlON I REALIZATION 2 , :f-pq* [ 1 P 0 I ; l k,(P) o ( I REALIZATlON 4 REALlZATlON 3 (I&tical 10 TLRltp) '1 d a Type I L-R MUTATOR i, = - i2 M-C MUTATOR REALIZATION 2 REALIZATION I + r. ,- -D---a 3pq blcp= p o il i2 1 + (ipI + x 2 ; “2 “I t/ildt)- I v, =-i (Idtmticdl t0 TCR2 ( p) 2 f) ,f a Type 2 C-R WTATOF d”2 il =r(t - Authorized licensed use limited to: IEEE Publications Staff. Downloaded on December 4, 2008 at 14:12 from IEEE Xplore. Restrictions apply.
Recommend
More recommend