In general, the lead block of the transfer function speeds up the - PowerPoint PPT Presentation

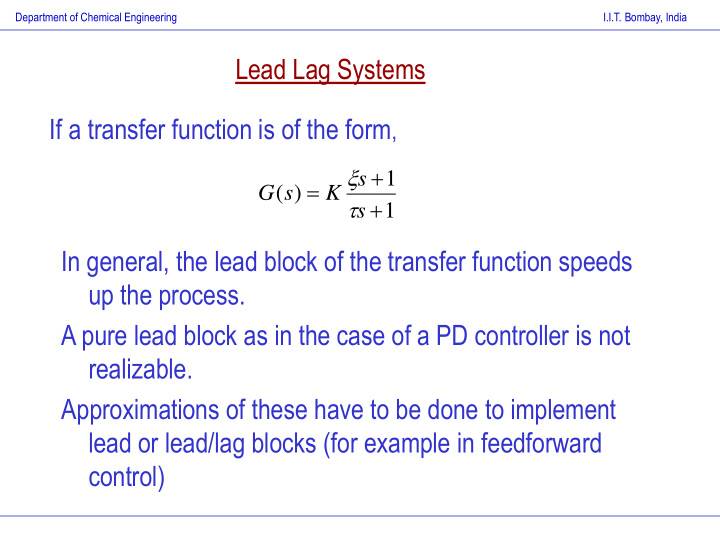
Department of Chemical Engineering I.I.T. Bombay, India Lead Lag Systems If a transfer function is of the form ,   s 1  G ( s ) K   s 1 In general, the lead block of the transfer function speeds up the process. A pure lead block as in the case of a PD controller is not realizable. Approximations of these have to be done to implement lead or lead/lag blocks (for example in feedforward control)

Department of Chemical Engineering I.I.T. Bombay, India Dynamic Response of a Lead Lag System   s 1  G ( s ) K   s 1 Using partial fraction expansion,   s 1 A    1 G ( s ) K K [ A ]     0 s 1 s 1 where A 0 =  /  and A 1 =1-A 0 = 1-    K       y ( s ) g ( s ) u ( s ) K ( 1 ) u ( s )       s 1

Department of Chemical Engineering I.I.T. Bombay, India Step Response of a Lead Lag System Depending on the value of  , an effective lead or lag action is seen

Department of Chemical Engineering I.I.T. Bombay, India Lectures 7: Dynamics of higher order systems

Department of Chemical Engineering I.I.T. Bombay, India Dynamic behaviour of second order systems Number of dynamic elements are 2. F h 1 h 2 Second order systems can arise because of two first order systems in interacting or noninteracting setups Interaction between the Inherent dynamics in the system is of higher order holdups could give an U-tube manometer. oscillatory response. Presence of a controller in a closed loop even if the process is first order (such as PI or PID controllers in a closed loop) can give a second order system.

Department of Chemical Engineering I.I.T. Bombay, India IIlustrative example: Interacting system of tanks in series F 0 h 2 h 1 First Principles Model dh    1 A F c ( h h ); 1 o 1 1 2 dt dh    2 A c ( h h ) c h 2 1 1 2 2 2 dt

Department of Chemical Engineering I.I.T. Bombay, India First Principles Model A A 1 c       1 2 1 If ; ; K ; K 1 2 1 2 c c c c 1 2 1 2 K K  1 2 y ( s ) u ( s )          2 2 s ( K ) s 1 1 2 1 2 2 1 The roots of the denominator (poles of the transfer function) could be complex. They could give rise to oscillatory behaviour for different u.

Department of Chemical Engineering I.I.T. Bombay, India Second Illustrative example: Control of a first order process + y d y Plant controller u -   t 1  dy     and      u ( t ) K ( y y ) ( y y ) dt y Ku  c d d   dt I 0   t dy 1        Therefore,   y KK ( y y ) ( y y ) dt  c d d dt   I 0 2 d y dy KK      c ( 1 KK ) y y  1 c d 2 dt dt I This is a second order system and will give rise to a second order transfer function.

Department of Chemical Engineering I.I.T. Bombay, India U-Tube Manometer  2 L d h 4 L dh 1    D P 1 P 2 h P   2 2 2 g dt g R dt 2 g L = length of fluid in the plane of h manometer tube initial rest h when D P = 0 ,  = density and viscosity of manometer fluid R = radius of manometer tube D P = P 1 - P 2 g = gravitation constant