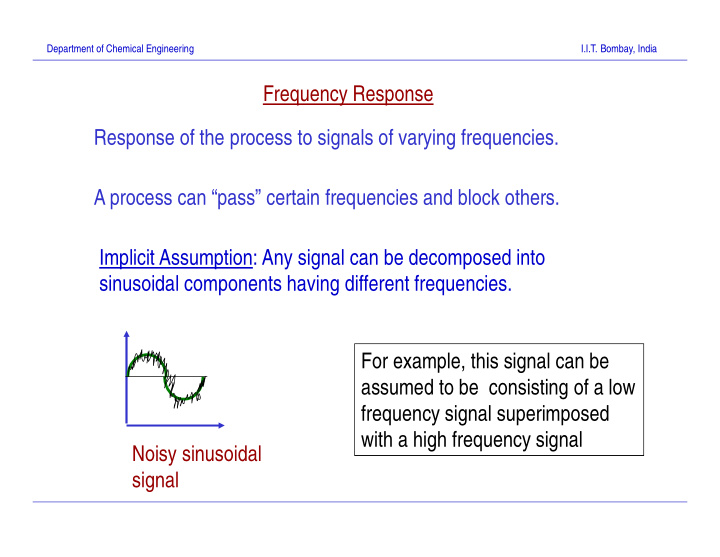



Department of Chemical Engineering I.I.T. Bombay, India Frequency Response Response of the process to signals of varying frequencies. A process can “pass” certain frequencies and block others. Implicit Assumption: Any signal can be decomposed into sinusoidal components having different frequencies. For example, this signal can be assumed to be consisting of a low frequency signal superimposed with a high frequency signal Noisy sinusoidal signal
Department of Chemical Engineering I.I.T. Bombay, India Frequency Response The inherent reluctance of a process and its natural dynamics determines its response to various frequencies. The reluctance is mostly a hindrance but sometime it can help ! Q c F,z D,x d A surge tank before the feed can dampen out the disturbances Q h and stabilize column operation B,x b
Department of Chemical Engineering I.I.T. Bombay, India Analysis of Dynamics via frequency response W=0.1 rad/sec W=1 rad/sec W=5 rad/sec
Department of Chemical Engineering I.I.T. Bombay, India Frequency Response Generation Process Transfer function y ( s ) G ( s ) ( ) u s Input signal w s u(t) = sin ( w t) u ( s ) 2 2 w y ( s ) G ( s ) 2 2 s w And then invert this in the time domain to get the frequency response.
Department of Chemical Engineering I.I.T. Bombay, India Basic Complex Algebra Given a complex number z=a+ j b, it can be represented in the complex plane as shown below. Im. axis b Real axis a Magnitude of z denoted by |z|= (a 2 +b 2 ) 0.5 and angle is tan -1 (b/a) and z can be more compactly represented as z=|z| e j
Department of Chemical Engineering I.I.T. Bombay, India Short cut Method for Frequency Response Generation Step 1: s=jw in G(s) to obtain G(jw) Step 2: Rationalize G(jw) to obtain Real and imaginary parts Step 3: The output sine wave will have an amplitude A out =A (Real 2 + Imag 2 ) and the phase angle is =tan -1 ( Imag/ Real). Illustrative example 1 jw 1 G ( jw ) s ( ) G s 1 1
Department of Chemical Engineering I.I.T. Bombay, India Short cut Method for Frequency Response Generation jw 1 1 jw G ( jw ) 2 2 2 2 2 2 1 1 1 w w w 1 w Re( jw ) and Im( jw ) 2 2 2 2 w 1 w 1 Output Amplitude is therefore 1 2 2 1 w 2 2 2 2 2 2 w 1 1 1 w w tan 1 Phase is therefore ( ) w
Department of Chemical Engineering I.I.T. Bombay, India Representation of Frequency Response Information Bode Plots: Plot the amplitude ratio (AR) and the phase lag on two separate plots called the Magnitude and the phase plots. For the illustrative example, Magnitude plots are typically logarithmic but phase plots are semi-logarithmic.
Department of Chemical Engineering I.I.T. Bombay, India Representation of Frequency Response Information Nyquist Plots: Plot the amplitude ratio (AR) and the phase lag on the same plot in the imaginary plane.Treat G(jw) as a complex number and plot the Real(G) and Imag(G) on the diagram. Note: Unlike the bode plot, the phase angle is implicit in the plot here.
Department of Chemical Engineering I.I.T. Bombay, India Frequency Response of several systems in series If g(s) = g 1 (s)g 2 (s)g 3 (s) … g N (s), then they can all be expressed in the frequency domain as g(jw)=g 1 (jw)g 2 (jw)g 3 (jw) … g N (jw) Using the polar form of representation g(jw)=|g 1 ||g 2 ||g 3 ||g 4 | …. |g N | e j , where , is the summation of the individual phase angles. Thus, the bode plots of higher order systems can be constructed from the local building blocks.
Department of Chemical Engineering I.I.T. Bombay, India General behaviour of the frequency response plots The amplitude ratio plots generally decay at higher frequencies, more so for chemical processes. The rate of decay is a function of the order of the process. The phase plot asymptotically approaches (p-q) times –90 o at high frequencies, where p is the number of poles and q is the number of zeros. Can you guess why ? Also, what is the frequency response of a pure delay process ?
Department of Chemical Engineering I.I.T. Bombay, India Interpretations from the frequency response K jw First Order Processes G ( jw ) 1 K tan 1 ( ) w AR and Phase are: 2 2 w 1 It is convenient to work in terms of a magnitude ratio: AR 1 2 2 log( ) log( w 1 ) 2 K As 0, AR/K 1 and log (AR/K) log(1); gives the low frequency asymptote As , and log (AR/K) -log( ) ; gives the high frequency asymptote (The two asymptotes meet at =1), I.e. at =1/ . Thus the natural time constant has a role in the frequency response.)
Department of Chemical Engineering I.I.T. Bombay, India Pure Capacity System: Frequency Response K G ( s ) , s K G ( jw ) jw K , AR w 1 o tan ( ) 90 AR log( ) log( ) w K
Department of Chemical Engineering I.I.T. Bombay, India Pure Lead System: Frequency Response ( ) ( 1 ) , G s K s ( ) ( 1 ) G jw K jw 2 2 1 , AR K w 1 tan ( ) w
Department of Chemical Engineering I.I.T. Bombay, India Lead - Lag System: Frequency Response Effective Lag ( 1 ) s G ( s ) K , 1 s ( jw 1 ) G ( jw ) K jw 1 Effective Lead 2 2 1 w AR K , 2 2 1 w 1 1 tan ( w ) tan ( w )
Department of Chemical Engineering I.I.T. Bombay, India Second-order Systems: Frequency Response Amplitude ( 1 ) G ( s ) K , ( s 1 )( s 1 ) 1 2 ( 1 ) Phase G ( jw ) K ( jw 1 )( jw 1 ) 1 2 1 o , 180 at higher frequencie s AR K 2 2 2 2 1 w 1 w o 1 2 0 at lower frequencie s AR 1 1 2 2 2 2 log( ) ( 1 w ) ( 1 w ) 1 2 K 2 2 AR log( ) log( 1 ), at low frequencie s K and to - 2log( w ) at higher frequencie s
Department of Chemical Engineering I.I.T. Bombay, India General Second-order Systems: Frequency Response Effect of damping factor Transfer Function ( K ) ( ) , G s 2 2 ( s 2 s 1 ) 1 AR , K 2 2 2 2 ( 1 w ) ( 2 w ) 2 w 1 tan 2 2 1 w It can be shown that the AR curve shows a hump for all values of less than 0.707 and the maxima occurs at 1 2 1 2 r
Department of Chemical Engineering I.I.T. Bombay, India Other higher order systems: Frequency Response Lead time constant > lag time constants Lead time constant < lag time constants
Department of Chemical Engineering I.I.T. Bombay, India Time Delay Systems s jw G ( s ) e , G ( jw ) e AR 1 , K w
Recommend
More recommend