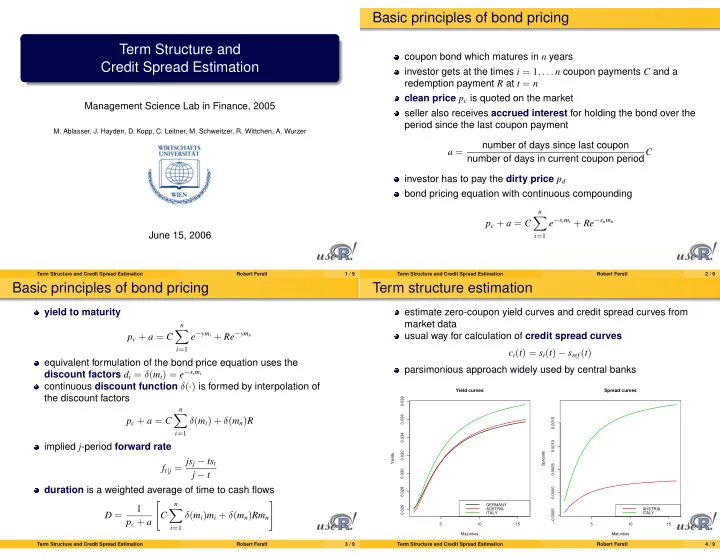

Basic principles of bond pricing Term Structure and coupon bond which matures in n years Credit Spread Estimation investor gets at the times i = 1 , . . . n coupon payments C and a redemption payment R at t = n clean price p c is quoted on the market Management Science Lab in Finance, 2005 seller also receives accrued interest for holding the bond over the period since the last coupon payment M. Ablasser, J. Hayden, D. Kopp, C. Leitner, M. Schweitzer, R. Wittchen, A. Wurzer number of days since last coupon a = number of days in current coupon period C investor has to pay the dirty price p d bond pricing equation with continuous compounding n e − s i m i + Re − s n m n � p c + a = C June 15, 2006 i = 1 Term Structure and Credit Spread Estimation Robert Ferstl 1 / 9 Term Structure and Credit Spread Estimation Robert Ferstl 2 / 9 Basic principles of bond pricing Term structure estimation yield to maturity estimate zero-coupon yield curves and credit spread curves from market data n � e − ym i + Re − ym n usual way for calculation of credit spread curves p c + a = C i = 1 c i ( t ) = s i ( t ) − s ref ( t ) equivalent formulation of the bond price equation uses the parsimonious approach widely used by central banks discount factors d i = δ ( m i ) = e − s i m i continuous discount function δ ( · ) is formed by interpolation of Yield curves Spread curves the discount factors 0.038 n � 0.036 p c + a = C δ ( m i ) + δ ( m n ) R 0.0015 i = 1 0.034 0.0010 implied j -period forward rate 0.032 Spreads Yields f t | j = js j − ts t 0.0005 0.030 j − t duration is a weighted average of time to cash flows 0.028 0.0000 � n � GERMANY 1 0.026 AUSTRIA AUSTRIA � −0.0005 D = C δ ( m i ) m i + δ ( m n ) Rm n ITALY ITALY p c + a 5 10 15 5 10 15 i = 1 Maturities Maturities Term Structure and Credit Spread Estimation Robert Ferstl 3 / 9 Term Structure and Credit Spread Estimation Robert Ferstl 4 / 9
Nelson and Siegel (1987) approach Nelson and Siegel (1987) approach Instantaneous forward rates f ( m , b ) = β 0 + β 1 exp ( − m m exp ( − m ) + β 2 ) Spot rates τ 1 τ 1 τ 1 1.0 1 − exp ( − m � 1 − exp ( − m � τ 1 ) τ 1 ) − exp ( − m s ( m , b ) = β 0 + β 1 + β 2 ) m m τ 1 τ 1 τ 1 0.8 β 1 ( m τ 1 ) β 2 ( m τ 1 ) exp ( − m τ 1 ) β 0 Objective function Model curves 0.6 n � 2 � � ˆ b opt = min ω i P i − P i weighted price errors 0.4 b i = 1 n 0.2 � y i − y i ) 2 b opt = min (ˆ yield errors b i = 1 0.0 0 2 4 6 8 10 Time to maturity Term Structure and Credit Spread Estimation Robert Ferstl 5 / 9 Term Structure and Credit Spread Estimation Robert Ferstl 6 / 9 Extensions References I Svensson (1994) extended the functional form by two additional Bank for International Settlements parameters which allows for a second hump-shape Zero-coupon yield curves: technical documentation BIS Papers , No. 25, October 2005 Instantaneous forward rates f ( m , b ) = β 0 + β 1 exp ( − m m exp ( − m m exp ( − m David Bolder, David Streliski ) + β 2 ) + β 3 ) τ 1 τ 1 τ 1 τ 2 τ 2 Yield Curve Modelling at the Bank of Canada Bank of Canada, Technical Report , No. 84, 1999 simple calculation method of credit spread curves could lead to Alois Geyer, Richard Mader twisting curves Estimation of the Term Structure of Interest Rates - A Parametric Jankowitsch and Pichler (2004) proposed a joint estimation Approach method , which leads to smoother and more realistic credit spread OeNB, Working Paper , No. 37, 1999 curves Term Structure and Credit Spread Estimation Robert Ferstl 7 / 9 Term Structure and Credit Spread Estimation Robert Ferstl 8 / 9
References II Rainer Jankowitsch, Stefan Pichler Parsimonious Estimation of Credit Spreads The Journal of Fixed Income , 14(3):49–63, 2004 Charles R. Nelson, Andrew F . Siegel Parsimonious Modeling of Yield Curves The Journal of Business , 60(4):473–489, 1987 Lars E.O. Svensson Estimating and Interpreting Forward Interest Rates: Sweden 1992 -1994 National Bureau of Economic Research, Technical Report , No. 4871, 1994 Term Structure and Credit Spread Estimation Robert Ferstl 9 / 9
Recommend
More recommend