


A new window on primordial non-Gaussianity based on 1201.5375 and 1206.4479 with M. Zaldarriaga Enrico Pajer Princeton University 2.0 � R � k � 2 � 10 9 1.5 Μ CMB � LSS 1.0 10 � 4 0.01 1 100 10 4 k Mpc
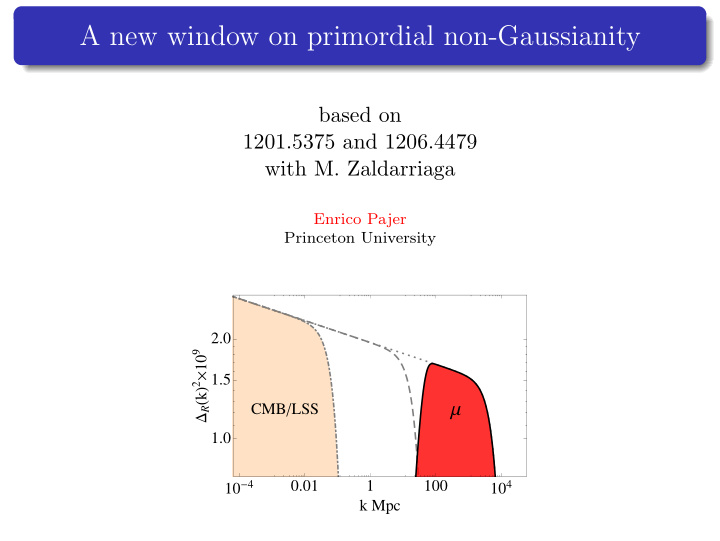
Summary We know little about primordial perturbations outside the range 10 − 4 � k Mpc � 1 µ -type spectral distortion of the CMB is a unique probe of small scales 50 � k Mpc � 10 4 [Sunyaev, Zel’dovich, Silk, Peebles, Hu, Danese, de Zotti, Chluba, . . . ] The monopole � µ (ˆ n ) � probes the small-scale power spectrum µT cross correlation probes the primordial bispectrum in the squeezed limit f loc NL Fisher forecast with current technology ∆ f loc NL � 10 3 Beat cosmic variance with an enormous number of modes Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 2 / 38
Outline 1 Motivations 2 Review of µ -distortion 3 A new window on primordial non-Gaussianity 4 Remarks Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 3 / 38
The golden age of cosmology We are living in the golden age of observational cosmology: COBE goes to Stockholm, WMAP, ACT & SPT measured the CMB with 1% accuracy. now Planck and a horde of ground and ballon based experiments Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 4 / 38
Primordial perturbations 1 H ? radiation matter dark E Log a Primordial superhorizon perturbations seed the structures in our universe They teach us about the earlier stage Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 5 / 38
The non-linear regime of structure formation Small superhorizon primordial perturbations → linear evolution Homogeneous background → no mix of different scales Perturbations re-enter the horizon → grow with time Large perturbations evolve in a complicated non-linear way → erase primordial information Linear regime: Large scale structures k < O (Mpc − 1 ) or look back in time Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 6 / 38
The cosmic microwave background A snapshot of the universe at 370 , 000 years, z ≃ 1100 Small perturbations δρ/ρ ∼ 10 − 5 → linearly related to primordial perturbations For l � 2000 or k > O (0 . 15 Mpc − 1 ) erased by diffusing damping! Can we access smaller scales? Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 7 / 38
The CMB/LSS window 2.00 1.00 0.50 outside CMB � LSS � R � k � 2 � 10 9 0.20 ? horizon Gaussian 0.10 0.05 Scale � inv 0.02 10 � 7 10 � 5 0.001 0.1 10 1000 k Mpc k � 10 − 4 Mpc − 1 are still outside our horizon k � 0 . 15 Mpc − 1 ( l � 2000) have been erased by Silk damping k � O (1) Mpc − 1 are now contaminated by gravitational non-linearities Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 8 / 38
Primordial perturbations: What do we know? Within the CMB/LSS window we know to the 1% precision [Hlozek et al. ’11] Random variable Amplitude 10 − 5 → can measure only few cumulants Almost scale invariant Adiabatic Gaussian Do these properties hold on smaller scales? Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 9 / 38
Outline 1 Motivations 2 Review of µ -distortion 3 A new window on primordial non-Gaussianity 4 Remarks Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 10 / 38
Photon thermodynamics: early times Early universe: hot photon-baryon-electron plasma Before z i ≃ 2 × 10 6 double Compton scattering ( e − + γ → e − + 2 γ ) and Bremsstrahlung are very efficient Perfect thermodynamical equilibrium. Any perturbations to the system is thermalized n � Ν � Photons can be created at low ν and re-scattered to high ν Planck spectrum ν 2 n ( ν ) = e ν/k B T − 1 5.0 10.0 Ν � kT 0.1 0.2 0.5 1.0 2.0 Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 11 / 38
Photon thermodynamics: µ -era Between z i and z f ∼ 5 × 10 4 double Compton scattering and bremsstrahlung are not efficient enough to create photons Elastic Compton scattering ( e − + γ → e − + γ ) maintains kinetic equilibrium Photon number is effectively frozen (except low ν ) n � Ν � A perturbation distort the spectrum Bose-Einstein spectrum with chemical potential µ ν 2 n ( ν ) = e ν/k B T + µ − 1 Ν � kT 0.5 1.0 2.0 5.0 Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 12 / 38
Photon thermodynamics: y-era After z f ∼ 5 × 10 4 also elastic Compton scattering is not efficient enough No kinetic equilibrium Photon number is still effectively frozen A perturbation deforms the spectrum → y-type distortion Mixing of black bodies with different T Different ν dependence. Observationally distinguishable [Khatri Chluba Sunyaev ’11] Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 13 / 38
Photon thermodynamics: decoupling After z L ≃ 10 3 photons travel without further interactions Electrons and protons combine into neutral H (and He, . . . ) Several other astrophysical foregrounds create y-distortion (mixing of black bodies) [Sunyaev Zel’dovich ’70] µ -distortion requires re-scattering of photons (kinetic equilibrium) → few contaminations! We have now a linear probe of scales 50 < k Mpc − 1 < 10 4 Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 14 / 38
Perturbations during the µ -era Perturbations during the µ -era [Khatri, Chluba Sunyaev ’11,’12] 5 × 10 4 � z � 2 × 10 6 Dissipation of acoustic modes due to diffusion damping [Silk ’72] . Scale invariant power spectrum n s ∼ 1 µ ≃ 2 . 4 × 10 − 8 Adiabatic cooling → Bose-Eistein condensation µ BE ≃ − 3 × 10 − 9 Non-standard physics e.g. decays of massive particles, . . . Standard scenario: µ probes the primordial power on small scales Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 15 / 38
Dissipation from random walk Photon mean free path λ mfp = ( n e σ T ) − 1 ⇒ random walk � √ ∆ t λ D ≃ λ mfp N ≃ λ mfp λ mfp Using ∆ t ∼ ∆ z/ ( zH ), n e ∼ z 3 and during radiation H ∼ z 2 � 1 ∆ z k ∼ a zH ∼ z − 5 / 2 λ ∼ z 3 / 2 λ ∼ ⇒ n e σ T Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 16 / 38
Dissipation of acoustic modes Perturbations of the adiabatic mode R re-enter the horizon, oscillate and dissipate δ γ ∼ R k cos( kt ) e − k 2 /k 2 D Shear viscosity and heat conduction damp the oscillations [Silk, Kaiser, Weinberg] k D ≃ z 3 / 2 4 × 10 − 6 Mpc − 1 Now we can only see k < k D ( z L ) ≃ 0 . 2 × Mpc − 1 � R � k � 2 � 10 9 z L z f µ -distortion can see 1 z i 1 50 < k Mpc − 1 < 10 4 measuring only large angles! (non-linear effect) 10 � 4 0.01 1 100 10 4 k Mpc Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 17 / 38
µ -era: hydrodynamical derivation For a Bose-Einstein spectrum Entropy density s ∼ T 3 (1 + const µ ) and photon number density n γ ∼ T 3 (1 + const µ ) Entropy per photon � s � � � µ 2 �� = 3 . 6 1 + 0 . 5 µ + O ⇒ ∂ t µ ∝ ∂ t ( s/n γ ) n γ Photon number conservation and generation of entropy for dissipative fluids ∂ µ ( u µ s ) = viscosity + conduction , ∂ µ ( u µ n γ ) = conduction One finds µ with the whole spatial dependence [EP & Zaldarriaga ’12b] Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 18 / 38
µ -era: heuristic derivation Analytical estimate of µ [Hu et al. 94’, Khatri et al. ’11, EP Zaldarriaga ’12b] Energy in acoustic waves with relativistic correction � i c 2 � s ρ � δ 2 � δE ∼ 1 + w ¯ γ � � f Bose-Einstein spectrum with E → E + δE and fixed N δµ ≃ − 1 . 4 δE ρ > 0 ¯ Integral probe of the power z i � R � k � 2 � 10 9 spectrum z L z f 1 1 � Μ d log k ∆ 2 µ ∝ R ( k ) 10 � 4 0.01 1 100 10 4 k Mpc Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 19 / 38
Tight coupling and free streaming Evolution of µ between z f ≃ 5 × 10 4 and us [EP & Zaldarriaga ’12b] Tight-coupling regime z f → z LS : only monopole µ 0 . Dissipation erases small-scale inhomogenities µ 0 ( q, z LS ) = µ 0 ( q, z f ) e − q 2 /q 2 D,µ ( z LS ) Free streaming z LS → 1: for l ≪ 1000 simple geometric projection µ l ( τ, q ) ≃ µ 0 ( τ f , q ) j l [ q ( τ − τ LS )] For l > 1000 there is damping from diffusion and the finite thickness of the last scattering surface � q D,µ . Relevant only for � µµ � Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 20 / 38
Relating µ to R 2 at small scales Dissipation is smeared over a volume k − 3 s Nature takes the average for us Small cosmic variance! [EP & Zaldarriaga ’12] Final analytical estimate � k + � � d 3 k 1 d 3 k 2 9 k 2 ) e i� R ( � k 1 ) R ( � k + · � x W µ ( x ) ≃ (2 π ) 6 2 k s � � i e − ( k 2 1 + k 2 2 ) /k 2 ×� cos ( k 1 t ) cos ( k 2 t ) � p D f Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 21 / 38
COBE-FIRAS bounds CMB compatible with a black body so far COBE-FIRAS puts a bound | µ | < 9 × 10 − 5 TRIS + COBE-FIRAS | µ | < 6 × 10 − 5 PIXIE could achieve ∆ µ ≃ 10 − 8 at 1- σ Enrico Pajer (Princeton) New window on primordial NG CWR Oct 2012 22 / 38
Recommend
More recommend