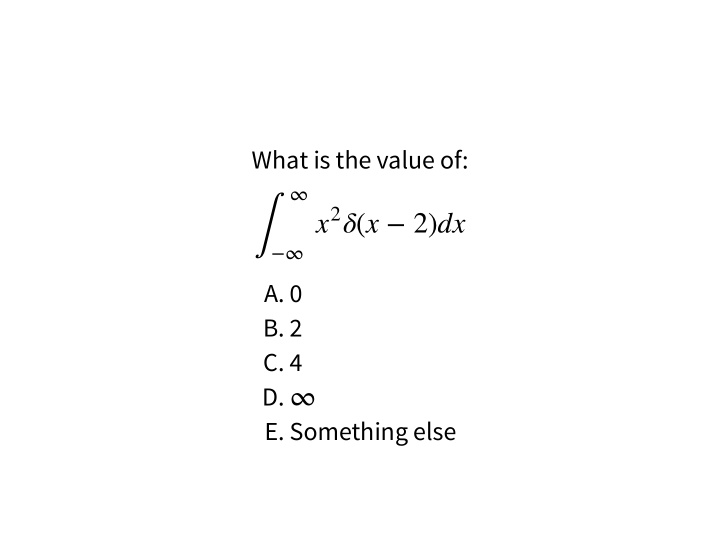



What is the value of: ∞ ∫ x 2 δ ( x − 2) dx −∞ A. 0 B. 2 C. 4 D. ∞ E. Something else
A point charge ( ) is located at position , as shown. What is q R , the charge density in all space? ρ ( r ) A. δ 3 ρ ( r ) = q ( R ) B. δ 3 ρ ( r ) = q ( r ) C. δ 3 ρ ( r ) = q ( R − r ) D. δ 3 ρ ( r ) = q ( r − R ) E. Something else??
An electric dipole ( and , small + q – q distance apart) sits centered in a d Gaussian sphere. What can you say about the flux of E through the sphere, and on the | E | sphere? A. Flux = 0, E = 0 everywhere on sphere surface B. Flux = 0, E need not be zero everywhere on sphere C. Flux is not zero, E = 0 everywhere on sphere D. Flux is not zero, E need not be zero...
Which of the following two fields has zero curl? I II A. Both do. B. Only I is zero C. Only II is zero D. Neither is zero E. ???
Can superposition be applied to electric potential, ? V ? ∑ V tot = V i V 1 V 2 V 3 = + + + … i A. Yes B. No C. Sometimes
Could this be a plot of ? Or ? (for SOME physical | E ( r ) | V ( r ) situation?) A. Could be , or E ( r ) V ( r ) B. Could be , but can't be E ( r ) V ( r ) C. Can't be , could be E ( r ) V ( r ) D. Can't be either E. ???
sits outside a solid neutral conducting A point charge + q copper sphere of radius . The charge q is a distance A r > A from the center, on the right side. What is the E-field at the center of the sphere? (Assume equilibrium situation). A. | E | = kq / r 2 , to le fu B. r 2 , to le fu kq / > | E | > 0 C. , to right | E | > 0 D. E = 0 E. None of these
A neutral copper sphere has a spherical hollow in the center. A charge is placed in the center of the hollow. What is the + q total charge on the outside surface of the copper sphere? (Assume Electrostatic equilibrium.) A. Zero B. − q C. + q D. 0 < q outer < + q E. − q < q outer < 0
True or False: The electric field, , in some region of E(r) space is zero, thus the electric potential, , in that same V ( r ) region of space is zero. A. True B. False
True or False: The electric potential, , in some region of V ( r ) space is zero, thus the electric field, , in that same E(r) region of space is zero. A. True B. False
The general solution for the electric potential in spherical coordinates with azimuthal symmetry (no dependence) is: ϕ ∞ B l ( ) ∑ A l r l V ( r , θ ) = P l (cos θ ) + r l +1 l =0 Consider a metal sphere (constant potential in and on the sphere, remember). Which terms in the sum vanish outside the sphere? (Recall: as ) V → 0 r → ∞ A. All the 's A l B. All the 's except A l A 0 C. All the 's B l D. All the 's except B l B 0 E. Something else
p = ∑ q i r i i What is the dipole moment of this system? (BTW, it is NOT overall neutral!) A. q d B. 2 q d 3 C. q d 2 D. 3 q d E. Someting else (or not defined)
You have a physical dipole, and a finite distance + q − q d apart. When can you use the expression: p ⋅ r ̂ 1 V ( r ) = r 2 4 πε 0 A. This is an exact expression everywhere. B. It's valid for large r C. It's valid for small r D. No idea...
You have a physical dipole, and a finite distance + q − q d apart. When can you use the expression: 1 q i 4 πε 0 ∑ V ( r ) = ℜ i i A. This is an exact expression everywhere. B. It's valid for large r C. It's valid for small r D. No idea...
Which charge distributions below produce a potential that C looks like when you are far away? r 2 E) None of these, or more than one of these! (For any which you did not select, how DO they behave at large r?)
Recommend
More recommend