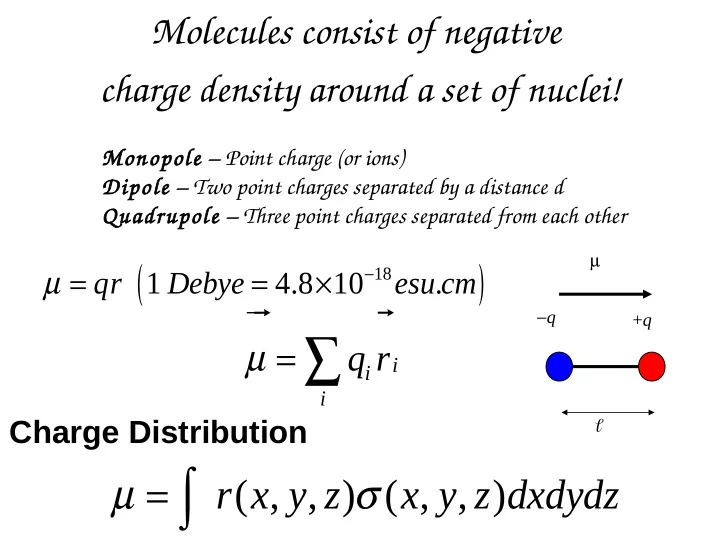

Molecules consist of negative charge density around a set of nuclei! Monopole – Point charge (or ions) Dipole – Two point charges separated by a distance d Quadrupole – Three point charges separated from each other u r r ( ) µ µ = = × − 18 qr 1 Debye 4.8 10 esu cm . u r r − q + q µ = ∑ q r i i i Charge Distribution u r r = ∫ µ σ r x y z ( , , ) ( , , ) x y z dxdydz
Dipole moment – Vector! For polymatomic molecules? H Cl µ b1 µ O µ b2 + q + H Cl l - - q µ b ( ) µ = ql θ µ µ = µ 2 b cos 2 θ µ b Note: the direction of The arrows should be reversed: Cl Cl O C O In chemistry, dipole moment Direction Is from positive to negative!
Induced Dipole in presence of an electric field: Polarizability u r u r µ = α α → E ; Polarizability molecular property ( ) u u r u r u r u r u r u r u r µ = 2 3 0 1 2 α = α + β + γ α + β + γ ( ) E E E E 0 E E E 0 β Hyperpolarizability; γ 2 nd order hyperpolarizability Induced dipole moment in a atom or molecule Induced dipole moment due to the polarization of charge distribution α Measure of the extent to which a charge distribution around an atom or a molecule can be distorted in presence on an uniform electric field (due to ion, another dipole) Loosely relate polarizability to volume units of volume!
Nature of intermolecular potential Lennard-Jones Potential 12 6 r r = ε − V e 2 e ÷ ÷ LJ r r ε What are the inter- r molecular interactions e that contribute to the total potential energy between two species? Sum of the pairwise potential energy of interaction Sum of the pairwise potential energy of interaction
Magnitudes of force vary depending on the type of interaction Ions in Electrolyte – Charges Liquid Helium- involve strong force! Weakest interactions Energy spectrum of intermolecular interactions Energy spectrum of intermolecular interactions
Various categories of intermolecular interactions (forces)
Ion-Ion Interaction for Charged molecules: Coulomb Potential ( q e q e )( ) Interaction via Coulomb potential = V r ( ) 1 2 πε 4 r q 1 e Z 2 e r 0
Monopole (Ion)-dipole interaction u r u r u r µ θ q Cos ( ) r θ = µ = j i V r , . E j − 2 ion dipole r
Monopole-dipole interaction u r u r u r µ θ q Cos ( ) θ = µ = j i V r , . E j − 2 ion dipole r π π ∫ ∫ θ θ θ θ = θ θ = Simple average over all : Cos Sin d Cos d Cos ( ) 0 0 0 ( ) θ = V r , 0 !!! − ion dipole Are all orientations equally probable? No! Orientations with lower energy are slightly favored! Dictated by Boltzmann Distribution ( ) θ V r , ( ) − P Orientation ~ exp ÷ k T B Need to calculate the average over all oreintations weighted by Boltzmann factor Boltzman Avgerage!
Boltzmann Average over all orientations u r u r ( ) ( ) µ θ µ θ θ q Cos V r , q V r , ( ) θ = j i − = j i θ − V r , .exp . Cos exp ÷ ÷ − 2 2 ion dip r k T r k T BA B B Analytically difficult, numerically easy! ( ) θ << → Assume V r : , k T interaction energy between monopole B and dipole is small compared to the thermal energy ( k T ) B x 2 = + − ≈ − expand exponential function exp(- ) x 1- x .. 1 x 2! ( ) ( ) θ θ V r , V r , θ − ≈ θ − Cos exp Cos 1 ÷ ÷ k T k T B B ( ) ( ) θ θ θ V r , V r , Cos = θ − θ = − Cos Cos k T k T B B
Orientation-Averaged potential for ion-dipole interactions u r µ q θ Cos ( ) ( ) θ = j i × − θ × V r , V r , − 2 ion dipole r k T Boltzman B u r u r µ µ θ q q Cos θ Cos = − j i × j i × 2 2 r r k T B u r 2 µ q 1 ( ) θ = − j i × × θ ÷ 2 V r , Cos ÷ − 2 ion dipole r k T Boltzman B π θ 3 Cos 2 π π [ ] ∫ ∫ θ = θ θ θ = θ − θ = − = ≠ 2 2 2 Cos Cos Sin d Cos d Cos ( ) 0 3 3 0 0 0 uu r ( ) 2 µ q 1 const . ( ) j i − − V r : : 4 − 4 r ion dipole 3 k T r Boltzman B
Interactions between two dipoles! Depending on the relative Orientation – magnitude and Sign of force will vary
Dipole-dipole interaction potential V ( r ) = µ 1 µ 2 f ( θ ) + q 2 - q 2 4 πε 0 r 3 r θ + q 1 - q 1 f ( θ ) = 1 − 3cos 2 θ Consider parallel dipoles (simplified) Orientation–averaged potential energy of two dipoles V ( r ) = µ 1 µ 2 f ( θ ) 4 πε 0 r 3 Take a Boltzmann average !! µ µ 2 2 2 1 = − V ( ) r 1 2 ~ Temperature attractive − ( ) d d 2 6 r πε 6 3 k T 4 r Dependent! B 0
Interaction of Ion and Induced-Dipole Presence of a nearby Ion Induced Dipole moment is (charge) will affect the generated, magnitude of Electronic distribution of Which depends on the An atom or a neutral Polarizability ( α )! (non-polar) molecule 2 α α q ( ) = − V r i ~ − ion ind _ dipole 4 4 2 r r
Dipole Induced-Dipole Interaction Not a thermal µ α α 2 ( ) = − Or Boltzman V r ~ 1 − dipole ind dipole _ π ε 2 2 6 6 4 r r Average: No 0 temperature dependence
Instantaneous electronic fluctuations – transient dipoles!
Induced Dipole-Induced Dipole Interaction (Dispersion force) Transient induced-dipole moments – electronic polarizabilies has to be involved - Due to correlated motion of electrons α α Independent of Orientation and ( ) i j V r ~ Temperature: No (KT) terms! − id id 6 r µ = 0 Termed London Forces, or dispersion! Termed London Forces, or dispersion! core of a quantum-mechanical effect! core of a quantum-mechanical effect! Can be extremely strong force if a large Can be extremely strong force if a large number of molecules are involved – number of molecules are involved – higher alkanes are solid at 300K higher alkanes are solid at 300K
Comparison of Various Interactions
Recommend
More recommend