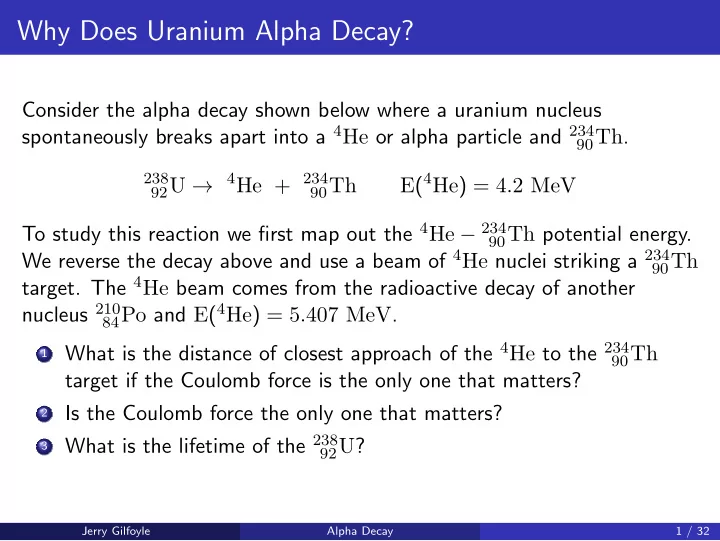
Why Does Uranium Alpha Decay? Consider the alpha decay shown below - PowerPoint PPT Presentation

Why Does Uranium Alpha Decay? Consider the alpha decay shown below where a uranium nucleus spontaneously breaks apart into a 4 He or alpha particle and 234 90 Th . 238 4 He + 234 E ( 4 He ) = 4 . 2 MeV 92 U → 90 Th To study this reaction we first map out the 4 He − 234 90 Th potential energy. We reverse the decay above and use a beam of 4 He nuclei striking a 234 90 Th target. The 4 He beam comes from the radioactive decay of another nucleus 210 84 Po and E ( 4 He ) = 5 . 407 MeV . 1 What is the distance of closest approach of the 4 He to the 234 90 Th target if the Coulomb force is the only one that matters? 2 Is the Coulomb force the only one that matters? 3 What is the lifetime of the 238 92 U ? Jerry Gilfoyle Alpha Decay 1 / 32

What Do We Know? 234 90 Th − α Potential The V = Z 1 Z 2 e 2 r V ( r ) ( MeV ) attractive nuclear part r ( fm ) Jerry Gilfoyle Alpha Decay 2 / 32

Mapping the Potential Energy Rutherford Scattering What is the distance of closest approach of the 4 He to the 234 90 Th target if only the Coulomb force is active? Is the Coulomb force the only one active? The energy of the 4 He emitted by the 210 84 Po to make the beam is E ( 4 He ) = 5 . 407 MeV . ZnS Microscope Collimator Alpha source Scattered helium Alpha beam Thorium target 210 4 206 Po He + Pb 84 2 82 Demo Jerry Gilfoyle Alpha Decay 3 / 32

Mapping the Potential Energy Rutherford Scattering What is the distance of closest approach of the 4 He to the 234 90 Th target if only the Coulomb force is active? Is the Coulomb force the only one active? The energy of the 4 He emitted by the 210 84 Po to make the beam is E ( 4 He ) = 5 . 407 MeV . ZnS Microscope Collimator Alpha source Scattered helium Alpha beam Thorium target 210 4 206 Po He + Pb 84 2 82 Demo Jerry Gilfoyle Alpha Decay 3 / 32

Mapping the Potential Energy Rutherford Scattering What is the distance of closest approach of the 4 He to the 234 90 Th target if only the Coulomb force is active? Is the Coulomb force the only one active? The energy of the 4 He emitted by the 210 84 Po to make the beam is E ( 4 He ) = 5 . 407 MeV . ZnS Microscope Collimator Alpha source Scattered helium Alpha beam Thorium target 210 4 206 Po He + Pb 84 2 82 Demo Jerry Gilfoyle Alpha Decay 3 / 32

Rutherford Trajectories Rutherford trajectories for different impact parameters y / b x Jerry Gilfoyle Alpha Decay 4 / 32

Mapping the Potential Energy The Differential Cross Section 4 He trajectory Th target Jerry Gilfoyle Alpha Decay 5 / 32

Mapping the Potential Energy The Differential Cross Section 4 He trajectory Th target � Z 1 Z 2 e 2 � 2 d σ 1 d Ω = sin 4 � θ � 4 E cm 2 Jerry Gilfoyle Alpha Decay 5 / 32

The Differential Cross Section areal particle rate incident angular = dN s ∝ × target × scattered into beam detector dt density dA of detector rate size dN s dN inc ∝ × n tgt × d Ω dt dt dN s = d σ d Ω × dN inc × n tgt × d Ω dt dt I beam - beam current dN inc = ∆ N inc = I beam Z - beam charge dt ∆ t Ze ρ tgt - target density n tgt = ρ tgt 1 = ρ tgt A tgt - molar mass N A V hit N A L tgt A tgt a beam A tgt V hit - beam-target overlap L tgt - target thickness Jerry Gilfoyle Alpha Decay 6 / 32

Areal or Surface Density of Nuclear Targets Jerry Gilfoyle Alpha Decay 7 / 32

The Differential Cross Section areal particle rate incident angular = dN s target scattered into beam detector ∝ × × dt density dA of detector rate size dN s dN inc × n tgt d Ω ∝ × dt dt = d σ dN s d Ω × dN inc × n tgt × d Ω dt dt I beam - beam current = ∆ N inc dN inc = I beam Z - beam charge dt ∆ t Ze ρ tgt - target density n tgt = ρ tgt a beam = ρ tgt 1 A tgt - molar mass A tgt N A V hit A tgt N A L tgt V hit - beam-target overlap L tgt - target thickness d Ω = dA det = ∆ A det dA det - detector area = sin θ d θ d φ r 2 r 2 r det - target-detector distance det det Jerry Gilfoyle Alpha Decay 8 / 32

Actual Rutherford Scattering Results 50 /2) θ ( 4 45 sin × Counts 40 35 30 25 20 15 H.Geiger and E.Marsden, Phil. Mag., 25, p. 604 (1913) alphas on gold 10 5 0 0 20 40 60 80 100 120 140 160 180 θ (deg) Jerry Gilfoyle Alpha Decay 9 / 32

Actual Rutherford Scattering Results 50 /2) θ � 2 � Z 1 Z 2 e 2 ( d σ 1 4 45 sin d Ω = sin 4 � θ � × 4 E cm Counts 40 2 35 30 25 20 15 H.Geiger and E.Marsden, Phil. Mag., 25, p. 604 (1913) alphas on gold 10 5 0 0 20 40 60 80 100 120 140 160 180 θ (deg) Jerry Gilfoyle Alpha Decay 9 / 32

Interpretation of Rutherford Scattering Results 1.2 1.0 Measured / Predicted 2 He + 234 4 90 Th → 4 2 He + 234 90 Th 0.8 � � 2 Z 1 Z 2 e 2 d σ 1 d Ω = 0.6 4 E cm sin 4 ( θ 2 ) Original decay 0.4 DOCA = 48 fm 238 92 U → 4 He + 234 90 Th E α ( U ) = 4 . 2 MeV 0.2 E α ( Po ) = 5 . 407 MeV 0.0 0 50 100 150 Scattering Angle ( deg ) What does this say about the 4 2 He − 234 90 Th potential energy? Jerry Gilfoyle Alpha Decay 10 / 32

Measuring the Size of the Nucleus θ cm ( deg ) Jerry Gilfoyle Alpha Decay 11 / 32

Measuring the Size of the Nucleus PRL 109 , 262701 (2012) E cm = 23 . 1 MeV E cm = 28 . 3 MeV θ cm ( deg ) Jerry Gilfoyle Alpha Decay 11 / 32

The 4 He − 234 90 Th Potential 40 α - Th Potential Blue - known Red - a guess 20 V ( MeV ) 0 - 20 - 40 0 10 20 30 40 50 60 70 r ( fm ) Jerry Gilfoyle Alpha Decay 12 / 32

The Paradox of Alpha Decay 1 We have probed the 248 90 Th potential into an internuclear distance of r DOCA = 48 fm using a 4 He beam of E ( 4 He ) = 5 . 407 MeV . 2 The data are consistent with the Coulomb force and no others. 3 The radioactive decay 238 92 U → 248 90 Th + 4 He emits an α (or 4 He ) with energy E α = 4 . 2 MeV . 4 For a classical ‘decay’ the emitted α should have an energy of at least E min = 5 . 407 MeV . 5 It appears the ‘decay’ α starts out at a distance r emit = 62 fm . 6 How do we explain this? Jerry Gilfoyle Alpha Decay 13 / 32

The Paradox of Alpha Decay 1 We have probed the 248 90 Th potential into an internuclear distance of r DOCA = 48 fm using a 4 He beam of E ( 4 He ) = 5 . 407 MeV . 2 The data are consistent with the Coulomb force and no others. 3 The radioactive decay 238 92 U → 248 90 Th + 4 He emits an α (or 4 He ) with energy E α = 4 . 2 MeV . 4 For a classical ‘decay’ the emitted α should have an energy of at least E min = 5 . 407 MeV . 5 It appears the ‘decay’ α starts out at a distance r emit = 62 fm . 6 How do we explain this? Quantum Tunneling! Jerry Gilfoyle Alpha Decay 13 / 32

The Paradox of Alpha Decay 1 We have probed the 248 90 Th potential into an internuclear distance of r DOCA = 48 fm using a 4 He beam of E ( 4 He ) = 5 . 407 MeV . 2 The data are consistent with the Coulomb force and no others. 3 The radioactive decay 238 92 U → 248 90 Th + 4 He emits an α (or 4 He ) with energy E α = 4 . 2 MeV . 4 For a classical ‘decay’ the emitted α should have an energy of at least E min = 5 . 407 MeV . 5 It appears the ‘decay’ α starts out at a distance r emit = 62 fm . 6 How do we explain this? Quantum Tunneling! 7 What do we measure? Jerry Gilfoyle Alpha Decay 13 / 32

The Paradox of Alpha Decay 1 We have probed the 248 90 Th potential into an internuclear distance of r DOCA = 48 fm using a 4 He beam of E ( 4 He ) = 5 . 407 MeV . 2 The data are consistent with the Coulomb force and no others. 3 The radioactive decay 238 92 U → 248 90 Th + 4 He emits an α (or 4 He ) with energy E α = 4 . 2 MeV . 4 For a classical ‘decay’ the emitted α should have an energy of at least E min = 5 . 407 MeV . 5 It appears the ‘decay’ α starts out at a distance r emit = 62 fm . 6 How do we explain this? Quantum Tunneling! 7 What do we measure? t 1 / 2 ( 238 U ) = 4 . 5 × 10 9 yr Lifetimes Jerry Gilfoyle Alpha Decay 13 / 32

The Plan For Calculating Nuclear Lifetimes 1 The α particle ( 4 He ) is confined by the nuclear potential and ‘bounces’ back and forth between the walls of the nucleus. Assume � 2 E α its energy is the same as the emitted nucleon so v = m . 2 Each time it ‘bounces’ off the nuclear wall it has a finite probability of tunneling through the barrier equal to the transmission coefficient T . 3 The decay rate will the product of the rate of collisions with a wall v and the probability of transmission equal to 2 R × T . vT = 2 R � m 4 The lifetime is the inverse of the decay rate 2 R 1 T . 2 E 5 The radius of a nucleus has been found to be described by r nuke = 1 . 2 A 1 / 3 where A is the mass number of the nucleus. 6 We are liberally copying the work of Gamow, Condon, and Gurney. Like them we will assume V = 0 inside the nucleus and V = 0 from the classical turning point to infinity. Jerry Gilfoyle Alpha Decay 14 / 32

The 4 He − 234 90 Th Potential Gamow, Condon and Gurney 30 20 V ( MeV ) 10 4.2 MeV 0 0 20 40 60 80 r ( fm ) Jerry Gilfoyle Alpha Decay 15 / 32

The Transfer-Matrix Solution 30 20 V ( MeV ) 10 4.2 MeV 0 0 20 40 60 80 r ( fm ) Jerry Gilfoyle Alpha Decay 16 / 32