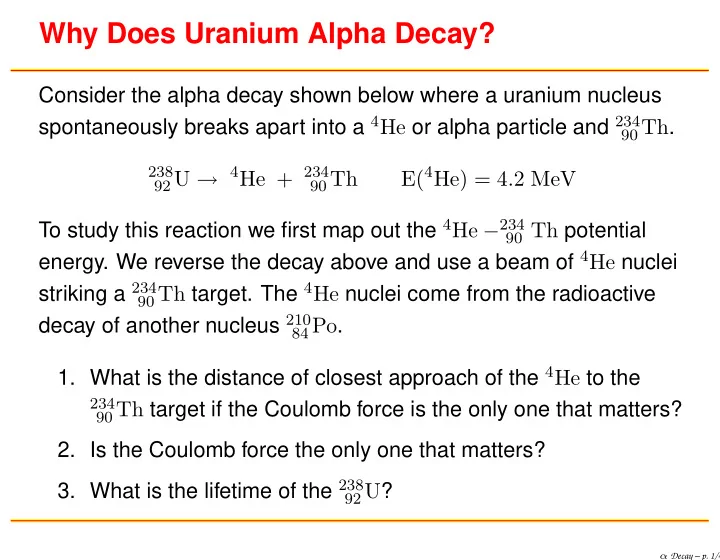
Why Does Uranium Alpha Decay? Consider the alpha decay shown below - PowerPoint PPT Presentation

Why Does Uranium Alpha Decay? Consider the alpha decay shown below where a uranium nucleus spontaneously breaks apart into a 4 He or alpha particle and 234 90 Th . 238 92 U → 4 He + 234 E( 4 He) = 4 . 2 MeV 90 Th To study this reaction we first map out the 4 He − 234 90 Th potential energy. We reverse the decay above and use a beam of 4 He nuclei striking a 234 90 Th target. The 4 He nuclei come from the radioactive decay of another nucleus 210 84 Po . 1. What is the distance of closest approach of the 4 He to the 234 90 Th target if the Coulomb force is the only one that matters? 2. Is the Coulomb force the only one that matters? 3. What is the lifetime of the 238 92 U ? α Decay – p. 1/4

What Do We Know? The 234 90 Th − α Potential V = Z 1 Z 2 e 2 r V � r � � MeV � attractive nuclear part r � fm � α Decay – p. 2/4

Mapping the Potential Energy Rutherford Scattering What is the distance of closest approach of the 4 He to the 234 90 Th target if only the Coulomb force is active? Is the Coulomb force the only one active? The energy of the 4 He emitted by the 210 84 Po is E( 4 He) = 5 . 407 MeV . ZnS Microscope Collimator Alpha source Scattered helium Alpha beam Thorium target 210 4 206 Po He + Pb 84 2 82 α Decay – p. 3/4

Mapping the Potential Energy The Total Cross Section dA α Decay – p. 4/4

Areal or Surface Density of Nuclear Targets α Decay – p. 5/4

Mapping the Potential Energy The Differential Cross Section α Decay – p. 6/4

Solid Angle α Decay – p. 7/4

Solid Angle α Decay – p. 8/4

Solid Angle α Decay – p. 9/4

Solid Angle α Decay – p. 10/4

Solid Angle α Decay – p. 11/4

Solid Angle α Decay – p. 12/4

Rutherford Scattering Results 1 ������������������������ Measured Predicted 0.5 0 0 90 180 Scattering Angle in degrees What does this say about the 4 2 He − 234 90 Th potential energy? α Decay – p. 13/4

Actual Rutherford Scattering Results 50 /2) θ 45 ( 4 Counts/sin 40 35 30 25 20 15 H.Geiger and E.Marsden, Phil. Mag., 25, p. 604 (1913) 10 alphas on gold 5 0 0 20 40 60 80 100 120 140 160 180 θ (deg) α Decay – p. 14/4

The 4 He − 234 90 Th Potential 40 Α� Th Potential Blue � known Red � a guess 20 V � MeV � 0 � 20 � 40 0 10 20 30 40 60 70 50 r � fm � α Decay – p. 15/4

Measuring the Size of the Nucleus θ cm ( deg ) α Decay – p. 16/4

Measuring the Size of the Nucleus PRL 109 , 262701 (2012) E cm = 23 . 1 MeV E cm = 28 . 3 MeV θ cm ( deg ) α Decay – p. 16/4

Rutherford Trajectories y x α Decay – p. 17/4

The 4 He − 234 90 Th Potential Gamow, Condon and Gurney 30 20 V � MeV � 10 4.2 MeV 0 0 20 40 60 80 r � fm � α Decay – p. 18/4

The α -Decay Puzzle 1. α -decay of uranium 238 U → 4 He + 234 90 Th ejects a 4.2-MeV 4 He . 2. Used a 5 . 4 − MeV 4 He beam 40 to probe the 4 He+ 234 90 Th force. Α� Th Potential Blue � known Red � a guess 20 3. It was all Coulomb down to V � MeV � 48 fm. 0 4 He 4. The decay with en- � 20 ergy 4.2 MeV is, apparently, � 40 ejected at a distance of 62 fm. 0 10 20 30 40 60 70 50 r � fm � 5. How can that happen? α Decay – p. 19/4

The α -Decay Puzzle 1. α -decay of uranium 238 U → 4 He + 234 90 Th ejects a 4.2-MeV 4 He . 2. Used a 5 . 4 − MeV 4 He beam 40 to probe the 4 He+ 234 90 Th force. Α� Th Potential Blue � known Red � a guess 20 3. It was all Coulomb down to V � MeV � 48 fm. 0 4 He 4. The decay with en- � 20 ergy 4.2 MeV is, apparently, � 40 ejected at a distance of 62 fm. 0 10 20 30 40 60 70 50 r � fm � 5. How can that happen? QUANTUM TUNNELING! α Decay – p. 19/4

The Plan for Solving the Alpha Decay Puzzle 1. Develop the notion of particle flux or flow. 2. Solve the Schroedinger equation for the rectangular barrier potential. 3. Determine the flux penetrating the barrier. 4. Develop the transfer-matrix method using the rectangular barrier results as the starting point. 5. Build a model of what happens in a uranium nucleus and predict the lifetime for α -decay. 6. Compare with data! α Decay – p. 20/4

The Rectangular Barrier Region 1 Region 2 Region 3 V 0 Incident Transmitted Wave and V � x � Reflected Transmitted Waves Wave Reflected Wave 0 2a x α Decay – p. 21/4

Particle Flux in a Beam Observation Point Particles of 0 ∆ average v t Cross−sectional velocity v 0 area of A α Decay – p. 22/4

The Rectangular Barrier Region 1 Region 2 Region 3 V 0 Incident Transmitted Wave and V � x � Reflected Transmitted Waves Wave Reflected Wave 0 2a x α Decay – p. 23/4

The Postulates 1. The state of a particle is represented by a wave function | ψ ( t ) � in a Hilbert space. The independent variables x and p are represented by Hermitian operators ˆ 2. X and P with the following matrix elements in the eigenbasis of ˆ ˆ X � x | ˆ � x | ˆ X | x ′ � = xδ ( x − x ′ ) P | x ′ � = xδ ′ ( x − x ′ ) The operators corresponding to dependent variables ω ( x, p ) are given Hermitian operators Ω( ˆ X , ˆ P ) = ω ( x → ˆ X , p → ˆ P ) . 3. If the particle is in a state | ψ � measurement of the variable Ω will yield one of the eigenvalues ω with probability P ( ω ) = |� ω | ψ �| 2 . The state of the system will change from | ψ � to | ω � . 4. The state vector | ψ ( t ) � obeys the Schroedinger equation i � d dtψ ( t ) = ˆ H | ψ ( t ) � where ˆ H ( ˆ X , ˆ P ) = H ( x → ˆ X , p → ˆ P ) is the quantum Hamiltonian operator and H is the corresponding classical problem. α Decay – p. 24/4

Quantum Tunneling α Decay – p. 25/4

Quantum Tunneling α Decay – p. 26/4

Quantum Tunneling 1� - 1 � � = 1� MeV a = 1� fm 1� - 4 T Red Curve - Quantum Result 1� - 7 Green Curve - Classical Result 5 1� 15 2� 25 3� Energy ( MeV ) α Decay – p. 27/4

Hint for Shankar 5.4.2.a The fundamental property of the Dirac delta function is the following. � ∞ � a + ǫ f ( x ) δ ( x − a ) dx = f ( a ) = f ( x ) δ ( x − a ) dx ǫ > 0 −∞ a − ǫ The Dirac delta function can be ‘represented’ by test functions that have the property defined above in the appropriate limit. 1 e − x 2 / 2 σ 2 δ ( x ) = lim √ 2 πσ σ → 0 α Decay – p. 28/4

The Transfer-Matrix Solution 30 20 V � MeV � 10 4.2 MeV 0 0 20 40 60 80 r � fm � α Decay – p. 29/4

The Transfer-Matrix Solution 30 20 V � MeV � 10 4.2 MeV 0 0 20 40 60 80 r � fm � α Decay – p. 30/4

The Transfer-Matrix Solution 30 20 V � MeV � 10 4.2 MeV 0 0 20 40 60 80 r � fm � α Decay – p. 31/4

Results Points �� Data, Line �� Theory 10 17 10 12 Lifetime � s � 10 7 100 0.001 10 � 8 6 7 8 5 E Α � MeV � α Decay – p. 32/4

Coordinates 40 Α� Th Potential Blue � known Red � a guess 20 V � MeV � 0 � 20 � 40 0 10 20 30 40 60 70 50 r � fm � α Decay – p. 33/4

Choosing the Sign Negative Initial Target y Position Positive x α Decay – p. 34/4

Center-of-Mass Rutherford Trajectories ’ v i1 Angle Scattering y CM ’ v i2 x α Decay – p. 35/4

The Inverse Cosine 3 x of 2 Cosine Inverse 1 � 1 0 1 x α Decay – p. 36/4

The Differential Cross Section α Decay – p. 37/4

Predicted Differential Cross Section for 4 He − Au 10 6 fm 2 in Section 10 4 Cross 10 2 90 180 Scattering Angle in degrees α Decay – p. 38/4

Measured Differential Cross Section for 4 He − Au 1 ������������������������ Measured Predicted 0.5 0 0 90 180 Scattering Angle in degrees α Decay – p. 39/4

The Evidence α Decay – p. 40/4

The Evidence α Decay – p. 41/4