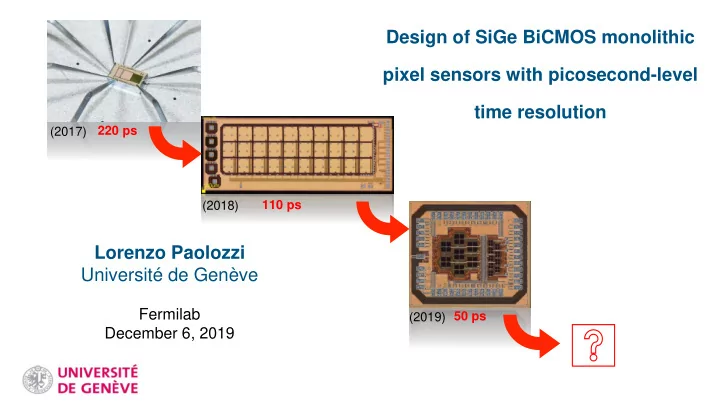

Design of SiGe BiCMOS monolithic pixel sensors with picosecond-level time resolution 220 ps (2017) 110 ps (2018) Lorenzo Paolozzi Université de Genève Fermilab 50 ps (2019) December 6, 2019 1
• Back in 2014 G. Iacobucci, R. Cardarelli and M. Nessi proposed a strategy to use SiGe HBTs for ultra-fast, low noise signal amplification in particle detectors. • The goal was to produce a monolithic pixelated silicon detector with 100 ps time resolution. ‣ L. Paolozzi and P. Valerio joined shortly later as chip designers. Today collaboration of: Funded by: Five years of (hard) R&D 2 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 2
Timing for high-energy physics experiments Hartmut F-W Sadrozinski et al 2018 Rep. Prog. Phys. 81 026101 Advanced track reconstruction 3 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 3
Timing for high-energy physics experiments Hartmut F-W Sadrozinski et al 2018 Rep. Prog. Phys. 81 026101 Advanced track reconstruction With timing 4 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 3
Timing for high-energy physics experiments Hartmut F-W Sadrozinski et al 2018 Rep. Prog. Phys. 81 026101 Pile-up suppression 5 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 4
Timing for high-energy physics experiments Hartmut F-W Sadrozinski et al 2018 Rep. Prog. Phys. 81 026101 Pile-up suppression 6 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 4
Situation today: technologies in HEP experiments NA62 GigaTracKer: hybrid pixels 300x300 µ m 2 no internal gain 130 ps time resolution G. Aglieri Rinella et al., JINST 14 (2019) P07010 7 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 5
Situation today: technologies in HEP experiments NA62 GigaTracKer: Low Gain Avalanche Detectors: hybrid pixels 300x300 µ m 2 hybrid pads 1x1 mm 2 no internal gain internal gain (10-100) 130 ps time resolution 30 ps time resolution G. Aglieri Rinella et al., JINST 14 (2019) P07010 N. Cartiglia et al., NIM A 924 (2019) 350-354 Resolution [ps] Gain 8 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 5
Situation today: technologies in HEP experiments NA62 GigaTracKer: Low Gain Avalanche Detectors: hybrid pixels 300x300 µ m 2 hybrid pads 1x1 mm 2 no internal gain internal gain (10-100) 130 ps time resolution 30 ps time resolution G. Aglieri Rinella et al., JINST 14 (2019) P07010 N. Cartiglia et al., NIM A 924 (2019) 350-354 Resolution [ps] Gain Excellent results. Is timing performance of silicon fully exploited ? How far are we from producing a monolithic 4D sensor with small pixels ? 9 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 5
Timing with silicon detectors 10 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 6
Time resolution of silicon pixel detectors (Recommended reading W. Riegler and G. Aglieri Rinella, Time resolution of silicon pixel sensors, JINST 12 (2017) P11017) What are the main parameters that control the time resolution of semiconductor detectors? 1. Geometry & fields 2. Charge collection (or Landau) noise 3. Electronics noise 𝐽 𝑗𝑜𝑒 𝑊 𝑝𝑣𝑢 𝒇 − 𝒊 + 11 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 7
1. Geometry and fields Sensor optimization for time measurement means: sensor time response independent from the particle trajectory GND ⟹ “Parallel plate” read out: wide pixel w.r.t. depletion depth 12 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 8
1. Geometry and fields Sensor optimization for time measurement means: sensor time response independent from the particle trajectory GND ⟹ “Parallel plate” read out: wide pixel w.r.t. depletion depth Induced current for 𝑤 𝑒𝑠𝑗𝑔𝑢,𝑗 ∙ ത 𝐽 𝑗𝑜𝑒 = 𝑟 𝑗 ҧ 𝐹 𝑥,𝑗 a parallel plate readout from Shockley- Ramo’s theorem: 𝑗 13 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 8
1. Geometry and fields Sensor optimization for time measurement means: sensor time response independent from the particle trajectory GND ⟹ “Parallel plate” read out: wide pixel w.r.t. depletion depth Induced current for 𝑤 𝑒𝑠𝑗𝑔𝑢,𝑗 ∙ ത 𝐽 𝑗𝑜𝑒 = 𝑟 𝑗 ҧ 𝐹 𝑥,𝑗 a parallel plate readout from Shockley- Ramo’s theorem: 𝑗 • Uniform weighting field (signal induction) • Uniform electric field (charge transport) Desired features: • Saturated charge drift velocity 14 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 8
1. Geometry and fields Sensor optimization for time measurement means: sensor time response independent from the particle trajectory GND ⟹ “Parallel plate” read out: wide pixel w.r.t. depletion depth 1 Induced current for 𝑤 𝑒𝑠𝑗𝑔𝑢,𝑗 ∙ ത 𝐽 𝑗𝑜𝑒 = 𝑟 𝑗 ҧ 𝐹 𝑥,𝑗 ≅ 𝑤 𝑒𝑠𝑗𝑔𝑢 𝐸 𝑟 𝑗 a parallel plate readout from Shockley- Ramo’s theorem: 𝑗 𝑗 Scalar, saturated Scalar, uniform • Uniform weighting field (signal induction) • Uniform electric field (charge transport) Desired features: • Saturated charge drift velocity 15 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 8
2. Charge-collection (or Landau) noise +HV 𝒇 − 𝒊 + GND Ionizing particle 1 is produced by the non uniformity of 𝐽 𝑗𝑜𝑒 ≅ 𝑤 𝑒𝑠𝑗𝑔𝑢 𝐸 𝑟 𝑗 the charge deposition in the sensor: 𝑗 When large clusters are absorbed at the electrodes, their contribution is removed from the induced current. The statistical origin of this variability of I ind makes this effect irreducible in PN-junction sensors. 16 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 9
2. Charge-collection (or Landau) noise Resolution [ps] N. Cartiglia et al., NIM A 924 (2019) 350-354 Gain Charge collection noise represents an intrinsic limit to the time resolution for a semiconductor PN-junction detector. ~30 ps reached by present LGAD sensors . Lower contribution from sensors without internal gain 17 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 10
3. Electronics noise Once the geometry has been fixed, the time resolution depends mostly on the amplifier performance. Threshold Time Pulse time 𝜏 𝑢 = 𝜏 𝑊 ≅ 𝐹𝑂𝐷 𝑒𝑊 𝐽 𝑗𝑜𝑒 𝑒𝑢 18 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 11
3. Electronics noise Once the geometry has been fixed, the time resolution depends mostly on the amplifier performance. Threshold Time Pulse time 𝜏 𝑢 = 𝜏 𝑊 ≅ 𝐹𝑂𝐷 𝑒𝑊 𝐽 𝑗𝑜𝑒 𝑒𝑢 Need an ultra-fast, low noise, low power-consumption electronics with fast rise time and small capacitance. Our solution: High 𝑔 𝑢 , single transistor preamplifier. 19 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 11
Equivalent Noise Charge: device comparison 𝑏 𝑋 𝑚𝑜2 𝐹𝑂𝐷 2 = 𝐵 1 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 2 𝜌 𝑑 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 3 𝑐 1 + 𝑐 2 𝜐 𝑁 𝜐 𝑁 20 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 12
Equivalent Noise Charge: device comparison 𝑏 𝑋 𝑚𝑜2 𝐹𝑂𝐷 2 = 𝐵 1 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 2 𝜌 𝑑 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 3 𝑐 1 + 𝑐 2 𝜐 𝑁 𝜐 𝑁 𝝊 𝑵 ~ 𝟐 𝒐𝒕 21 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 12
Equivalent Noise Charge: device comparison 𝑏 𝑋 𝑚𝑜2 𝐹𝑂𝐷 2 = 𝐵 1 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 2 𝜌 𝑑 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 3 𝑐 1 + 𝑐 2 𝜐 𝑁 𝜐 𝑁 𝝊 𝑵 ~ 𝟐 𝒐𝒕 How do MOS-FET and BJT compare in terms of noise? 22 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 12
Equivalent Noise Charge: device comparison 𝑏 𝑋 𝑚𝑜2 𝐹𝑂𝐷 2 = 𝐵 1 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 2 𝜌 𝑑 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 3 𝑐 1 + 𝑐 2 𝜐 𝑁 𝜐 𝑁 CMOS based amplifier 23 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 13
Equivalent Noise Charge: device comparison 𝑏 𝑋 𝑚𝑜2 𝐹𝑂𝐷 2 = 𝐵 1 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 2 𝜌 𝑑 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 3 𝑐 1 + 𝑐 2 𝜐 𝑁 𝜐 𝑁 2𝑙𝑈 ℎ CMOS based 1 𝑔 contribution Large ൗ amplifier 𝑛 24 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 13
Equivalent Noise Charge: device comparison 𝑏 𝑋 𝑚𝑜2 𝐹𝑂𝐷 2 = 𝐵 1 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 2 𝜌 𝑑 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 3 𝑐 1 + 𝑐 2 𝜐 𝑁 𝜐 𝑁 BJT based amplifier 25 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 14
Equivalent Noise Charge: device comparison 𝑏 𝑋 𝑚𝑜2 𝐹𝑂𝐷 2 = 𝐵 1 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 2 𝜌 𝑑 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 3 𝑐 1 + 𝑐 2 𝜐 𝑁 𝜐 𝑁 BJT based amplifier 26 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 14
Equivalent Noise Charge: device comparison 𝑏 𝑋 𝑚𝑜2 𝐹𝑂𝐷 2 = 𝐵 1 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 2 𝜌 𝑑 𝐷 𝑒𝑓𝑢 + 𝐷 𝑗𝑜 2 + 𝐵 3 𝑐 1 + 𝑐 2 𝜐 𝑁 𝜐 𝑁 2 𝑙 1 ⋅ 𝐷 𝑢𝑝𝑢 BJT based 2 𝐹𝑂𝐷 series noise ∝ 𝛾 + 𝑙 2 ⋅ 𝑆 𝑐 𝐷 𝑢𝑝𝑢 amplifier 27 /58 Lorenzo Paolozzi 06/12/2019 - Fermilab 14
Recommend
More recommend