TDDC17 Schemas: ( B x,y (P x,y+1 P x,y-1 P x+1,y P x-1,y )) # - PowerPoint PPT Presentation

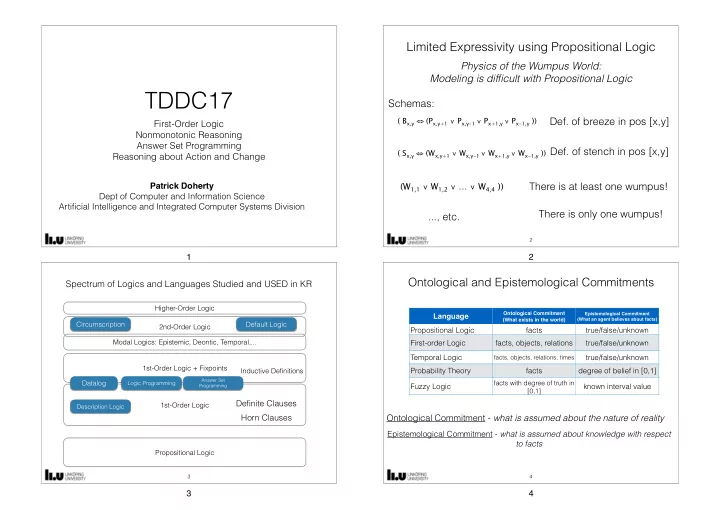
Limited Expressivity using Propositional Logic Physics of the Wumpus World: Modeling is difficult with Propositional Logic TDDC17 Schemas: ( B x,y ⇔ (P x,y+1 ∨ P x,y-1 ∨ P x+1,y ∨ P x-1,y )) # Def. of breeze in pos [x,y] First-Order Logic Nonmonotonic Reasoning Answer Set Programming ( S x,y ⇔ (W x,y+1 ∨ W x,y-1 ∨ W x+1,y ∨ W x-1,y )) # Def. of stench in pos [x,y] Reasoning about Action and Change (W 1,1 ∨ W 1,2 ∨ … ∨ W 4,4 )) " Patrick Doherty There is at least one wumpus! Dept of Computer and Information Science Artificial Intelligence and Integrated Computer Systems Division There is only one wumpus! ..., etc. 2 1 2 Ontological and Epistemological Commitments Spectrum of Logics and Languages Studied and USED in KR Higher-Order Logic Ontological Commitment Epistemological Commitment Language (What an agent believes about facts) (What exists in the world) Circumscription Default Logic 2nd-Order Logic Propositional Logic facts true/false/unknown Modal Logics: Epistemic, Deontic, Temporal,... First-order Logic facts, objects, relations true/false/unknown Temporal Logic true/false/unknown facts, objects, relations, times 1st-Order Logic + Fixpoints Probability Theory facts degree of belief in [0,1] Inductive Definitions Answer Set Datalog facts with degree of truth in Logic Programming Programming Fuzzy Logic known interval value [0,1] Definite Clauses 1st-Order Logic Description Logic Horn Clauses Ontological Commitment - what is assumed about the nature of reality Epistemological Commitment - what is assumed about knowledge with respect to facts Propositional Logic 3 4 3 4

The Syntax of First-Order Logic Ontological Commitments of First-order Logic Different applications have different first-order languages, Facts but certain components are common to all languages Objects Relations Propositional Connectives: ^ , _ , ! , ¬ , $ Propositional Constants: ? , > • Model with: Quantifiers: • 5 objects • 2 binary relations 8 (for all, the universal quantifier) • brother • on-head 9 (there exists, the existential quantifier) • 3 unary relations • person Punctuation: ”)”, ”(”, ” , ”, . . . • crown • king • 1 unary function Variables: v 1 , v 2 , . . . (which we write informally as x, y, z, . . . ) • left-leg() 5 6 5 6 First-Order Language First-Order Language Terms name individuals Definition 1. A first-order language , L ( R , F , C ), is determined by specifying: (1) A finite or countable set R of relation symbols , or predicate symbols , each of which Definition 2. The family of terms of L ( R , F , C ) is the smallest set meeting the conditions: has a positive integer associated with it denoting its arity. (2) A finite or countable set F of function symbols , each of which has a positive integer (1) Any variable is a term of L ( R , F , C ). associated with it denoting its arity. (2) Any constant symbol (member of C ) is a term of L ( R , F , C ). (3) A finite or countable set C of constant symbols . (3) If f is an n -place function symbol (member of F ) and t 1 , . . . , t n are terms of L ( R , F , C ), then f ( t 1 , . . . , t n ) is a term of L ( R , F , C ). Example 1. Let L ( R , F , C ) be the first-order language where, (1) R = { h Brother, 2 i , h OnHead, 2 i , h Person, 1 i , h Crown, 1 i , h King, 1 i } . Examples: Richard , John , leftleg ( x ) , leftleg ( John ) , Crown (2) F = { h leftleg, 1 i } . (3) C = { John, Richard, Crown } . 7 8 7 8

First-Order Language Restricted use of a First-Order Language We will use a function free first-order language and assume Definition 3. An atomic formula of L ( R , F , C ) is any string of the form R ( t 1 , . . . , t n ) where R 2 R is an n -place relation symbol and t 1 , . . . , t n are terms of L ( R , F , C ); also ? a one-to-one mapping between constants and objects. Additionally, and > are taken to be atomic formulas of L ( R , F , C ). we will assume our object domain is finite. Examples: Definition 1 A signature is a three tuple Σ = h O , P , V i of (disjoint) sets, where: Brother ( Richard , John ) , King ( John ) , OnHead ( Crown , x ) • O is a set of object constants, > • P is a set of predicate constants, and Definition 4. The family of formulas of L ( R , F , C ) is the smallest set meeting the following conditions: • V is a set of variables. • V (1) Any atomic formula of L ( R , F , C ) is a formula of L ( R , F , C ). (2) If A is a formula of L ( R , F , C ), so is ¬ A . Example 1 (3) For a binary connective � , if A and B are formulas of L ( R , F , C ), so is ( A � B ). (4) If A is a formula of L ( R , F , C ) and x is a variable, then ( 8 x ) A and ( 9 x ) A are Logic Programming Convention: • O = { arad, sibiu, fagaras, bucharest } formulas of L ( R , F , C ). Predicates are lower case • • P = { in, goto } • Variables are upper case ( ∀ x )( ∀ y ) Brother ( x, y ) → Brother ( y, x ) Object constants are lower case • • V = { X, Y } Examples: Brother ( Richard , John ) ∧ King ( John ) ( ∃ x ) King ( x ) ∧ OnHead ( Crown , x ) 9 10 9 10 Database Semantics Restricted 1st-Order Language cont • V { } R J Assume a language with 2 constant symbols, and Definition 2 Terms (over signature Σ ) are defined as follows: And one binary relation symbol • Variables and object constants are terms. Two constants can refer Some individuals can to the same individual be unnamed Definition 3 An atomic statement , or simply an atom , is an expression of the form Constants p ( t 1 , . . . , t n ) where p is a predicate symbol of arity n , and t 1 , . . . , t n are terms. Addition- ally, > , ? , representing True and False , respectively, are also atomic statements. Other statements in the language can be built from atomic statements using the logical Model connectives. Domain Definition 4 A grounded atomic statement , or simply a grounded atom , is an expression of the form p ( t 1 , . . . , t n ) where p is a predicate symbol of arity n , and t 1 , . . . , t n are terms 1st-order logic semantics: Some members of the set of all models that do not contain variables. A grounded statement is built up from grounded atomic statements using the logical connectives. Constants Unique Names Assumption Atomic Statements Domain Closure Assumption Grounded Atomic Statements goto ( X , Y ) goto ( sibiu , fagaras ) Model goto ( arad , Y ) in ( fagaras ) Domain in ( fagaras ) Database semantics: Some members of the set of all models goto ( X , sibiu ) ∧ goto ( sibiu , Z ) → in ( Z ) Statement: Grounded Statement: goto ( arad , sibiu ) ∧ goto ( sibiu , fagaras ) → in ( fagaras ) Database semantics is also used in logic programming 11 12 11 12

What about Quantifiers? Grounded Instantiations = Propositional Logic ! Example 4 Existential formulas are disjunctions Given the following signature Σ 1 : Given a signature , and set of formulas , we view each Σ 1 Δ of grounded formulas formula as the set of its grounded instantiations. • O = { a, b } • P = { p, q } Example 3 • V = { X } Given the following signature Σ 1 : and the the theory ∆ = { 9 X ( q ( X ) ! p ( X )) } , the grounding of ∆ is: • O = { a, b } ( q ( a ) ! p ( a )) _ ( q ( b ) ! p ( b )) • P = { p, q } • V = { X } Universal formulas are conjunctions and the the theory ∆ = { q ( X ) → p ( X ) } , the grounding of ∆ is: of grounded formulas q ( a ) → p ( a ) q ( b ) → p ( b ) Consequently, our theories are propositional where each grounded atomic statement can be viewed as a propositional variable, and each statement as a propositional formula, where the standard propositional semantics applies. 13 14 13 14 Action, Change and Common Sense • One of the most important problems in KR is to be able to represent action, dynamics, time, space, belief, knowledge and causality. • Intelligent Agents : Observe, Plan and Act. • Agents with general intelligence operate in Common Sense Informatic situations Reasoning about Action and Change • Late 60’s and 70’s: difficulties in modeling and understanding Nonmonotonic Reasoning • Invention of Nonmonotonic Logics in early 70’s • Used for modeling normative common sense behavior Common Sense Reasoning • Used for modeling inertial and other assumptions about dynamic environments • Great progress in modeling in 80’s and 90’s • Trend toward pragmatic, scalable reasoning tools after 2000 • Common sense reasoning and understanding of the world is still one of the “ Holy Grails ” of Artificial Intelligence. 15 16 15 16