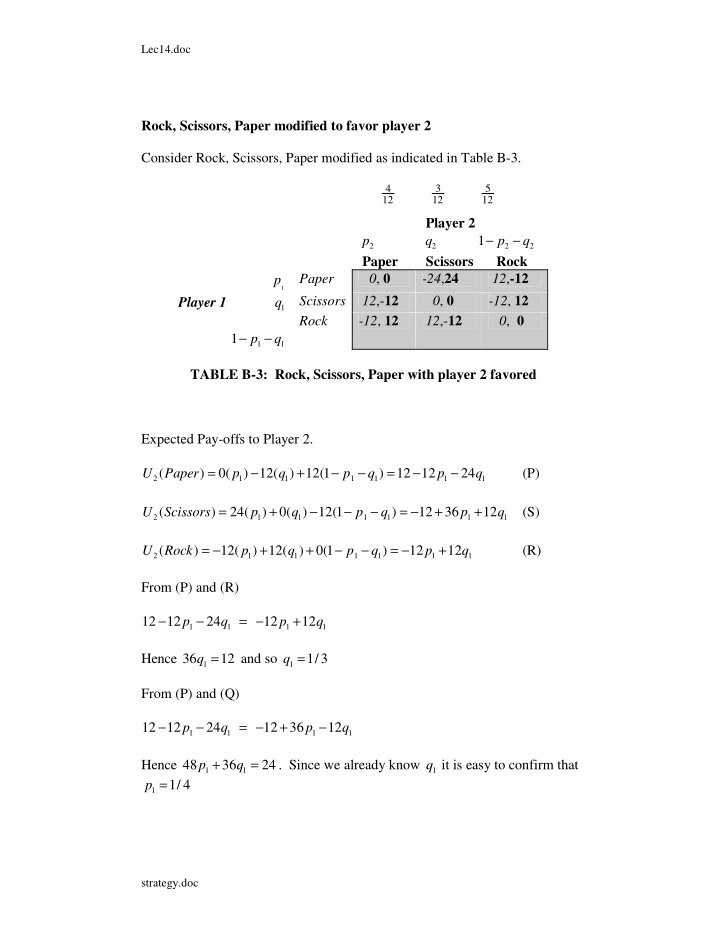



Lec14.doc Rock, Scissors, Paper modified to favor player 2 Consider Rock, Scissors, Paper modified as indicated in Table B-3. 4 3 5 12 12 12 Player 2 − − p q 1 p q 2 2 2 2 Paper Scissors Rock p Paper 0 , 0 -24 , 24 12 , -12 1 q Scissors 12 ,- 12 0 , 0 -12 , 12 Player 1 1 Rock -12 , 12 12 ,- 12 0 , 0 − − 1 p q 1 1 TABLE B-3: Rock, Scissors, Paper with player 2 favored Expected Pay-offs to Player 2. = − + − − = − − U ( Paper ) 0( p ) 12( q ) 12(1 p q ) 12 12 p 24 q (P) 2 1 1 1 1 1 1 = + − − − = − + + U Scissors p q p q p q (S) ( ) 24( ) 0( ) 12(1 ) 12 36 12 2 1 1 1 1 1 1 = − + + − − = − + U ( Rock ) 12( p ) 12( q ) 0(1 p q ) 12 p 12 q (R) 2 1 1 1 1 1 1 From (P) and (R) − − = − + 12 12 p 24 q 12 p 12 q 1 1 1 1 q = q = Hence 36 12 and so 1/3 1 1 From (P) and (Q) − − = − + − 12 12 p 24 q 12 36 p 12 q 1 1 1 1 + = Hence 48 p 36 q 24 . Since we already know q it is easy to confirm that 1 1 1 p = 1/ 4 1 strategy.doc
C. SOLUTION CONCEPTS Dominant strategy equilibrium Consider the following pricing game between Best Buy and Circuit City. Each can announce one of three prices High, Middle or Low. The payoffs are as shown in the table below. As a first step in trying to predict which strategies would be chosen, we ask whether there is a strategy, which is (weakly) dominated by another strategy. Circuit City High Middle Low High 100, 100 30 , 150 -40 , 90 Best Buy Middle 150, 30 50, 50 -10 , 60 Low 90 ,- 40 60,- 10 0 , 0 TABLE C-1: Pricing Game Dominated Strategy: Player i ’s strategy is s is weakly dominated by i s ′ if, for all possible responses by other agents, player i ’s another strategy i s ′ and strictly higher for some payoff is never lower using strategy i responses. In the Pricing Game above, Best Buy is strictly better off playing Middle than playing High. That is, for each price chosen by Circuit City, Best Buy is strictly better off playing Middle than playing High. Since the payoff matrix is symmetric, the same is true for Circuit City. 1
If we delete the dominated strategy, the payoff matrix reduces to the one depicted below. Circuit City Middle Low Best Buy Middle 50, 50 -10 , 60 Low 60,- 10 0 , 0 TABLE C-2: Pricing Game with dominated strategy deleted Now the strategy Middle is dominated by Low for Circuit City and Best Buy leaving only the strategy pair (Low, Low) Price competition is great for consumers! 2
Nash Equilibrium Unfortunately, it is only in special cases that there is a dominant strategy equilibrium. Typically, what is best for one player depends on what he believes his opponents will choose as their strategies. The key idea is that each player wants to maximize his expected payoff. Then, if he knew what his opponents were doing, he would choose his best response. Each player then tries to figure out what would happen if all players are sophisticated and are playing best responses. This leads to the following definition NASH EQUILIBRIUM A choice of strategies (possibly mixed strategies) by the n players is a strategic Nash equilibrium if, for each player, his chosen strategy is a best response to the strategies chosen by the other players. If players are choosing strategic equilibrium strategies, then no player would gain if he were to find out his opponents’ strategies. (Of course in a game like Rock, Scissors, Paper it would be very helpful to know what your opponent will actually play. But knowing only what kind of randomizing that he is doing does not help if both are using equilibrium strategies.) Theorem: John Nash (1951) For games in which each player has a finite number of feasible strategies, there is at least one strategic equilibrium. (In fact, except in some “knife edge” cases, the number of equilibria is odd.) It is because of this theorem (for which Nash was awarded the Nobel prize) that economists usually refer to a strategic equilibrium as a Nash Equilibrium. 3
D. CLASSES OF GAMES 1. Constant Sum Games Rock, Scissors, Paper is an example of a class of games in which wealth is simply transferred among players, so that the sum of the payoffs is zero. In such games equilibrium is unique and equilibrium strategies are mixed. We now consider some other classes of games and some of the additional issues that arise. One problem is that there may be more than one equilibrium. 2. Matching Games You have a chance to make 6 million dollars. All you do is have to meet someone at noon on October 30. Unfortunately all you know is that you must meet somewhere on the Campus. You write down a list of places where you can imagine meeting. To keep things simple, suppose your list has only three places on it. The payoff matrix is then as shown below. Player 2 Northern Inverted Bruin Walk Lights Fountain Northern Lights 6 , 6 0 , 0 0 , 0 Player 1 Inverted Fountain 0 , 0 6 , 6 0 , 0 Bruin Walk 0 , 0 0 , 0 6 , 6 TABLE D-1: Matching Game Exercise D-1 : Having identified 4 equilibria, can you find any more? How many equilibria are there for this game? Communication makes the game easy 4
3. The Dating Game (Battle of the Sexes) Player 2 (He) Movie Football 30 , 6 0 , 0 Player 1 (She) Movie Football 0 , 0 6 , 30 TABLE D-3: Dating Game Is is not difficult to check that the mixed strategy equilibrium is for players to choose their desired strategies with probability 56. Communication? Player 2 (He) Movie Football Player 1 (She) Movie 30 , 2 0 , 0 Football 0 , 0 6 , 20 TABLE D-4: Another dating game 5
4. Game of “Chicken” Suppose two males are set to play a game of “chicken.” They will drive towards each other in the middle of the road. If one is aggressive and goes straight while the other swerves(to his left), the aggressive player gets a payoff of 40 (the admiration of all those watching.) The “chicken” gets nothing. If they both swerve, they are equally admired. If neither swerve they crash and have to pay for their cars to be fixed. Player 2 Aggressive Chicken Player 1 Aggressive -5 , - 5 40 , 0 0 , 40 20 , 20 Chicken TABLE D-5: “Chicken” 6
5. Prisoners’ Dilemma One of the most famous (and important) classes of games is the prisoners’ dilemma. Both are in jail awaiting trial. If they keep quiet that will be found guilty of one crime and each get a year in prison. If one “squeals” and agrees to testify he will go free and the other will get a 10-year sentence. If both talk, enough mud will stick that each will end up with a three-year term. Since the two are kept in separate jail cells, communication is impossible. Player 2 Not Confess Confess Player 1 Not Confess -1 , -1 -10 , 0 Confess 0 , - 10 -3 , -3 TABLE D-6: Prisoners’ Dilemma It is easy to see that the only strategic equilibrium is for both players to confess. In fact the strategies not chosen are dominated strategies. That is, for each strategy of player 2, player 1 is better off choosing Confess than Not Confess. The same is true for player 2. Thus, after eliminated strictly dominated strategies, only one possibility remains - - both confess. 7
CHANGING THE STRATEGY SET Circuit City $120 $100 $80 $120 100, 100 30 , 150 -40 , 90 Best Buy $100 150, 30 50, 50 -10 , 60 90 ,- 40 60,- 10 0 , 0 $80 TABLE C-1: Pricing Game Circuit City $120 $100 $80 $60 $120 100, 100 30 , 150 -40 , 90 -60, 40 Best Buy $100 150, 30 50, 50 -10 , 60 -30, 30 $80 90 ,- 40 60,- 10 0 , 0 -20 ,-30 40 ,-60 -30,- 20 -50 ,-50 $60 30,-30 TABLE: Pricing Game with added price Circuit City $120 $100 $80 $60 100, 100 30 , 150 -40 , 90 -60, 40 $120 Best Buy $100 150, 30 50, 50 -10 , 60 -30, 30 $80 90 ,- 40 60,- 10 0 , 0 -20 ,-30 $60 40 ,-60 30,-30 -30,- 20 -50 ,-50 120 or 100, 100 60, -10 -30,-20 -, - CC-20 TABLE: Pricing Game with “super competitive” offer 8
Circuit City 120 or $120 $100 $80 $60 BB-20 $120 100, 100 30 , 150 -40 , 90 -60, 40 100,100 150, 30 50, 50 -10 , 60 -30, 30 Best Buy $100 -10,60 $80 90 ,- 40 60,- 10 0 , 0 -20 ,-30 -30,-20 $60 40 ,-60 30,-30 -30,- 20 -50 ,-50 -,- 120 or 100, 100 60, -10 -30,-20 -, - -,- CC-20 TABLE: Pricing Game with “super competitive” offer 9
Recommend
More recommend