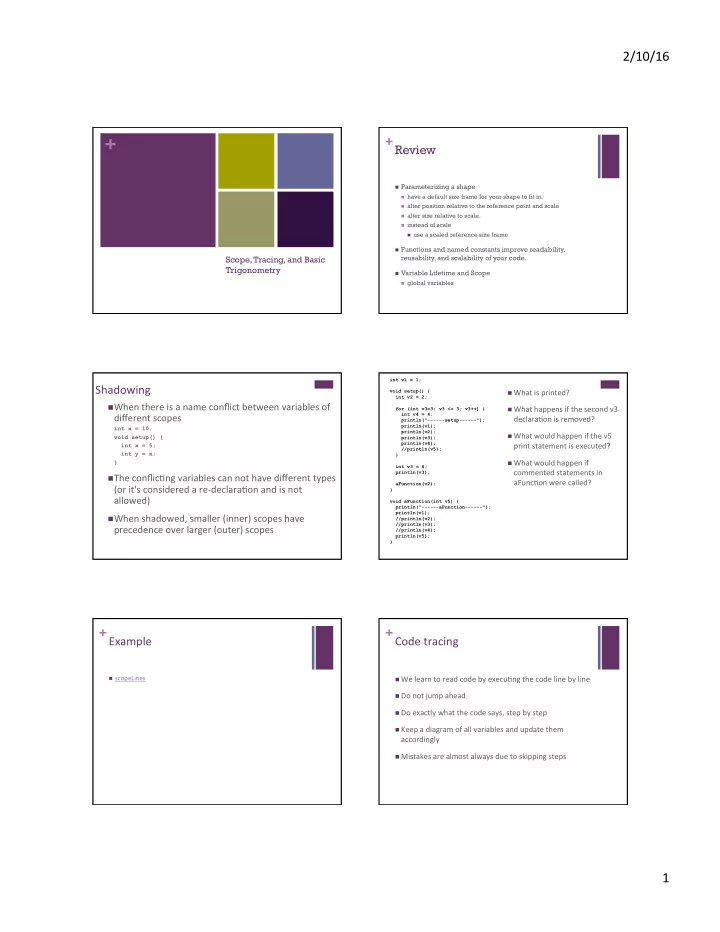

2/10/16 ¡ + + Review n Parameterizing a shape n have a default size frame for your shape to fit in. n alter position relative to the reference point and scale n alter size relative to scale. n instead of scale n use a scaled reference size frame n Functions and named constants improve readability, reusability, and scalability of your code. Scope, Tracing, and Basic Trigonometry n Variable Lifetime and Scope n global variables int v1 = 1; Shadowing ¡ n What ¡is ¡printed? ¡ void setup() { int v2 = 2; n When ¡there ¡is ¡a ¡name ¡conflict ¡between ¡variables ¡of ¡ n What ¡happens ¡if ¡the ¡second ¡v3 ¡ for (int v3=3; v3 <= 3; v3++) { different ¡scopes ¡ int v4 = 4; declara?on ¡is ¡removed? ¡ println("------setup------"); println(v1); int x = 10; println(v2); n What ¡would ¡happen ¡if ¡the ¡v5 ¡ void setup() { println(v3); println(v4); print ¡statement ¡is ¡executed? ¡ int x = 5; //println(v5); int y = x; } n What ¡would ¡happen ¡if ¡ } int v3 = 6; commented ¡statements ¡in ¡ println(v3); n The ¡conflic?ng ¡variables ¡can ¡not ¡have ¡different ¡types ¡ aFunc?on ¡were ¡called? ¡ aFunction(v2); (or ¡it's ¡considered ¡a ¡re-‑declara?on ¡and ¡is ¡not ¡ } allowed) ¡ void aFunction(int v5) { println("------aFunction------"); println(v1); n When ¡shadowed, ¡smaller ¡(inner) ¡scopes ¡have ¡ //println(v2); //println(v3); precedence ¡over ¡larger ¡(outer) ¡scopes ¡ //println(v4); println(v5); } + Example ¡ + Code ¡tracing ¡ n scopeLines ¡ n We ¡learn ¡to ¡read ¡code ¡by ¡execu?ng ¡the ¡code ¡line ¡by ¡line ¡ n Do ¡not ¡jump ¡ahead ¡ n Do ¡exactly ¡what ¡the ¡code ¡says, ¡step ¡by ¡step ¡ n Keep ¡a ¡diagram ¡of ¡all ¡variables ¡and ¡update ¡them ¡ accordingly ¡ n Mistakes ¡are ¡almost ¡always ¡due ¡to ¡skipping ¡steps ¡ 1 ¡
2/10/16 ¡ + Trace ¡this ¡ + Nested ¡loops ¡ 1 int n = 365; n for(...){ n You ¡can ¡put ¡a ¡loop ¡within ¡a ¡ for(...){ 2 int sum = 0; loop ¡ } 3 int digit; n Nes?ng ¡levels ¡are ¡unlimited, ¡ } but ¡in ¡prac?ce ¡programmers ¡ n while(...){ while(...){ rarely ¡go ¡beyond ¡3 ¡ 4 while(n>0) { } n Two ¡loops ¡nested ¡is ¡very ¡ 5 digit = n%10; } common, ¡especially ¡when ¡ n for(...){ 6 sum += digit; dealing ¡with ¡naturally ¡2-‑ while(...){ 7 n /= 10; dimensional ¡structures ¡(grids) ¡ } 8 } } n while(...){ for(...){ 9 println(sum); } } 7 ¡ + Nested ¡ for ¡ + Nested ¡ for ¡ int i, j, end = 10; int i, j, end = 10; for (i = 1; i <= end; i++) { for (i = 1; i <= end; i++) { for (j = i; j <= end; j++) { for (j = 1; j <= i; j++) { print("*"); print("*"); } } println(); println(); } } 9 ¡ 10 ¡ + Examples ¡ + Basics ¡of ¡Trigonometry ¡assuming ¡ ¡ right/up ¡axes ¡ n indexTile ¡(one ¡loop) ¡ h n indexTile ¡(loop ¡with ¡nested ¡Loop) ¡ (hypotenuse) o (opposite) q a (adjacent) 2 ¡
� � � 2/10/16 ¡ + Basics ¡of ¡Trigonometry ¡assuming ¡ ¡ + Basics ¡of ¡Trigonometry ¡assuming ¡ ¡ right/up ¡axes ¡ right/up ¡axes ¡ h h (hypotenuse) (hypotenuse) o o = h * sin(q) (opposite) (opposite) Recall: Recall: a^2 + o^2 = h^2 a^2 + o^2 = h^2 sin (q) = o/h q q a a = h * cos(q) cos (q) = a/h (adjacent) (adjacent) + Defini?on ¡ + Trigonometry ¡on ¡a ¡unit ¡circle ¡ 90 ° n sin (q) = o/h Recall: � x^2 + y^2 = r^2 � n o = h*sin (q) p r = 1 � (1*cos(q))^2 + � r (1*sin(q))^2 � = 1^2 � y n cos (q) = a/h or � q 0 ° n a = h*cos (q) x origin cos^2(q) + � sin^2(q) = � 1 � n tangent (q) = o/a = sin (q)/ cos (q) + Trigonometry ¡on ¡Processing ¡unit ¡circle ¡ + Trigonometry ¡on ¡a ¡unit ¡circle ¡ 90 ° p r origin x 0 ° q q 0 ° y r p 90 ° 3 ¡
2/10/16 ¡ + Trigonometry ¡on ¡a ¡unit ¡circle ¡ Drawing ¡points ¡along ¡a ¡circle ¡ ¡ 90 ° int steps = 8; int radius = 20; float angle = 2*PI/steps; for (int i=0; i<steps; i++) { float x = cos(angle*i)*radius; float y = sin(angle*i)*radius; q 0 ° // draw a point every 1/8th of a circle ellipse(x, y, 10, 10); } ¡ + Examples + Example: cyclical change n points on a circle n Drawing a sine wave n overlapping ellipses on a circle n Using sine to manipulate height of an object n spokes n Using cosine to manipulate width of an object n polygon n nested version (star) 4 ¡
Recommend
More recommend