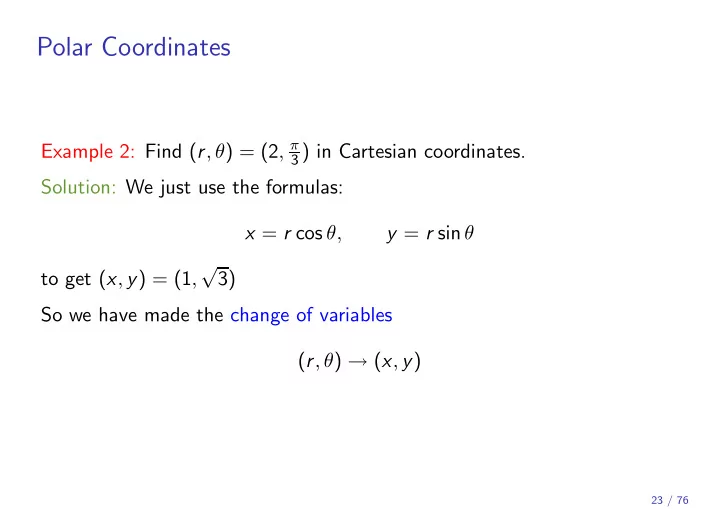
Polar Coordinates Example 2: Find ( r , ) = (2 , 3 ) in Cartesian - PowerPoint PPT Presentation

Polar Coordinates Example 2: Find ( r , θ ) = (2 , π 3 ) in Cartesian coordinates. Solution: We just use the formulas: x = r cos θ, y = r sin θ √ to get ( x , y ) = (1 , 3) So we have made the change of variables ( r , θ ) → ( x , y ) 23 / 76

Polar Coordinates Example 1: The circle of centre (0 , 0) and radius 1 can be written using polar coordinates as r = 1 BIG IDEA: Polar coordinates are suitable when the problems have circular symmetry. 24 / 76

Polar Coordinates Example 2: Valentine’s day is approaching ... In this spirit, consider the function r = 1 − cos θ 1 0.5 0 –2 –1.5 –1 –0.5 –0.5 –1 Figure: Cardioid 25 / 76

Polar Coordinates In general, let x = r cos θ, y = r sin θ with 0 ≤ θ < 2 π. Then a function g ( r , θ ) is a composition of f ( x , y ), with x ( r , θ ) = r cos θ and y ( r , θ ) = r sin θ , i.e., g ( r , θ ) = f ( x ( r , θ ) , y ( r , θ )) Useful formulas: � y � x 2 + y 2 , � r = θ = arctan x 26 / 76

Polar Coordinates Example 2: Let f ( x , y ) = x 2 + y 2 . 18 16 14 12 10 8 6 –3 –3 4 –2 –2 2 –1 –1 0 y 1 x 1 2 2 3 3 What is g ( r , θ ) = f ( r cos θ, r sin θ )? 27 / 76

Cylindrical Coordinates We can extend the idea of polar coordinates in 3 dimensions. Consider the chance of coordinates: ( x , y , z ) → ( r , θ, z ) with x = r cos θ, y = r sin θ, z = z with 0 ≤ θ < 2 π Then a cylinder with axis of symmetry the z -axis and radius 1 can be represented as r = 1 BIG IDEA: Suitable coordinates for problems with cylindrical symmetry! 28 / 76

Cylindrical Coordinates A function f ( x , y , z ) can be written as a function g ( r , θ, z ) = f ( r cos θ, r sin θ, z ) in cylindrical coordinates. Example: Let √ zx x 2 + y 2 − f ( x , y , z ) = e x 2 + y 2 � In cylindrical coordinates this would become g ( r , θ, z ) = f ( r cos θ, r sin θ, z ) = e r − z cos θ Much simpler to manipulate! 29 / 76

Spherical Coordinates We can extend the idea of polar coordinates in 3 dimensions, in yet another way than cylindrical coordinates. Consider the chance of coordinates: ( x , y , z ) → ( r , θ, φ ) with x = r cos θ sin φ, y = r sin θ sin φ, z = r cos φ with 0 ≤ θ < 2 π AND 0 ≤ φ ≤ π Example: Write ( x , y , z ) = (1 , 1 , 0) in spherical coordinates. √ Answer: ( r , θ, φ ) = ( 2 , π 2 ) 4 , π 30 / 76

Spherical Coordinates A sphere with centre (0 , 0) and radius 1 can be represented as r = 1 BIG IDEA: Suitable coordinates for problems with spherical symmetry! 31 / 76

Spherical Coordinates A function f ( x , y , z ) can be written as a function g ( r , θ, φ ) = f ( r cos θ sin φ, r sin θ sin φ, r cos φ ) in spherical coordinates. Example: Let f ( x , y , z ) = x 2 + y 2 + z 2 − z In spherical coordinates this would become g ( r , θ, φ ) = f ( r cos θ sin φ, r sin θ sin φ, r cos φ ) = r 2 − r cos φ Much simpler to manipulate! 32 / 76