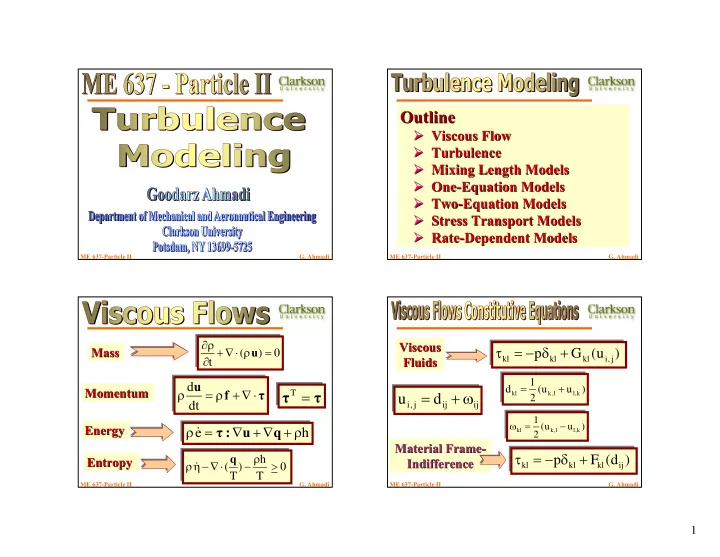
Outline Outline Viscous Flow Viscous Flow Turbulence - PowerPoint PPT Presentation

Outline Outline � Viscous Flow � Viscous Flow � Turbulence Turbulence � � Mixing Length Models Mixing Length Models � � � One One- -Equation Models Equation Models � Two Two- -Equation Models Equation Models � � Stress Transport Models Stress Transport Models � � � Rate Rate- -Dependent Models Dependent Models ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi ∂ ρ Viscous Viscous τ = − δ + + ∇ ⋅ ρ = Mass Mass u p G ( u ) ( ) 0 ∂ Fluids kl kl kl i , j Fluids t 1 u d = + d ( u u ) Momentum ρ dt = ρ + ∇ ⋅ Momentum τ = f τ = + ω T τ kl k , l l , k u d 2 i , j ij ij 1 ω = − ρ = ∇ + ∇ + ρ ( u u ) Energy Energy τ : u q & e h kl k , l l , k 2 Material Frame- Material Frame - τ = − δ + ρ q p F ( d ) h Entropy Entropy Indifference Indifference ρ η − ∇ ⋅ − > & ( ) 0 kl kl kl ij T T ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi 1

τ = − + λ δ + µ µ > ′ = + u = ′ u i = 0 ( p u ) 2 d u U u U 0 i i i i i kl i , i kl kl ′ = + λ + µ > p = p = ′ p P p 3 2 0 P 0 Navier Navier- - Stokes Stokes Reynolds Equation Reynolds Equation ∂ ∂ ∂ ∂ 2 u u p u ρ + = − + µ i i i ( u ) ′ ′ ∂ ∂ ∂ ∂ ∂ ∂ j ∂ ∂ ∂ ∂ 2 u u U U 1 P U . t x x x x + = − + ν − i j i i i U j i j j ∂ j ∂ ρ ∂ ∂ ∂ t x x x x x j i j j j ∂ u i = 0 τ = − ρ ′ ′ Turbulent Stress Turbulent Stress T u u ∂ x ij i j i ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi u = Eddy Eddy Eddy dU Eddy Velocity Scale ′ ′ ν T ≈ τ = − ρ = ρν T u v l c u 21 T Viscosity Viscosity Viscosity Viscosity dy = l Length Scale τ ∂ T ∂ U U 1 Kinematic Kinematic c = ′ ′ ′ ′ Speed of Sound ij = − = ν + j − δ u u ( i ) u u ν ∝ λ c ρ ∂ ∂ i j T k k ij x x 3 Viscosity Viscosity j i λ = Mean Free Path Mixing Mixing ∂ ∂ ν ∂ U U T ′ ′ Free Shear Flows Free Shear Flows τ = ρ − = T l 2 | | T v T l ~ l c σ ∂ 21 m ∂ ∂ Length Length y y y m 0 T Near Wall Flows ∂ Near Wall Flows = κ U l y ν = l 2 | | m ∂ T m y ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi 2

∂ U = γ T = Mixing ν T = Mixing 0 0 Local Equilibrium Mixing length When When 0 Local Equilibrium Mixing length Local Equilibrium Mixing length ∂ Length Length y Production = Dissipation Hypothesis Production = Dissipation Production = Dissipation Hypothesis Hypothesis ν ≈ 0 ν Experiment Experiment . 8 | Short Comings of Mixing Length Short Comings of Mixing Length T T max ν Cold U T ν = γ = 0 � � Eddy viscosity vanishes when velocity Eddy viscosity vanishes when velocity T T gradient is zero gradient is zero Hot � Lack of transport of turbulence scales Lack of transport of turbulence scales � � Estimating the mixing length Estimating the mixing length � ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi Eddy Viscosity Eddy Viscosity ν = 1 / 2 l c µ k T Exact k- Exact k -equation equation ′ ′ ∂ ′ ′ ′ ∂ d u u u u P U = − ′ + − ′ ′ i i i i i u ( ) u u ∂ ρ ∂ k i j dt 2 x 2 x 1 2 3 1 4 4 4 2 4 4 4 3 k j 1 4 2 4 3 Convective Turbulence Diffusion Pr oduction Transport Mixing Mixing γ T = ′ ′ ′ ′ ∂ ∂ ∂ 2 0 u u u u Reattachment Point Reattachment Point − ν + ν Length Length i i i i ∂ ∂ ∂ x x x x 2 Maximum Heat Maximum Heat j j j j 1 4 2 4 3 1 42 4 43 4 Experiment Experiment Transfer Transfer Dissipatio n Viscous Diffusion ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi 3

Modeled k- -equation equation Modeled k K- -equation equation K ∂ ν ∂ dk k ∂ τ ∂ 3 / 2 = dk U k T ( ) = + − Max ( Bk ) ak c ∂ σ ∂ dt x x ∂ ρ ∂ D 1 l dt y y 2 3 j k j 1 42 4 43 4 1 2 3 1 4 2 4 4 3 4 Dissipatio n Diffusion Pr oduction Diffusion ∂ ∂ ∂ 3 / 2 U U U k Short Comings of One- -Equation Models Equation Models + ν + − Short Comings of One j i i ( ) c T ∂ ∂ ∂ D 1 l x x x � Lack of transport of turbulence length scale � Lack of transport of turbulence length scale 2 3 j i j 1 4 4 4 2 4 4 4 3 � Estimating the length scale � Estimating the length scale Dissipatio n Pr oduction ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi z = ∂ ν ∂ ν ∂ l k dz z U = 2 l / k Time Scale = + 2 T T ( ) z [ c ( ) ∂ σ ∂ 1 ∂ dt y y k y 2 = 1 42 43 1 4 2 4 3 z l k / Frequency Scale Diffusion Pr oduction 2 = k − + l k / Vorticity Scale c ] S { ν 2 z 1 2 3 T ′ ′ ∂ ∂ Secondary u u ε = ν = Source i i Dissipatio n Rate Dissipatio n ∂ ∂ x x j j ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi 4

′ ′ ′ ε ∂ ∂ ∂ ∂ E(k) Universal Equilibrium Universal Equilibrium d u u u ′ = − ε − ν i i k ( u ' ) 2 , ∂ ∂ ∂ ∂ j dt x x x x 1 4 2 4 4 3 4 j k l l 1 4 2 4 3 , Generation by Diffusion vortex stretching ′ ′ ∂ ∂ 2 2 u u Inertia Inertia − ν i i 2 Subrang Subrang Kolmogorov Kolmogorov ∂ ∂ ∂ ∂ x x x x e e 1 4 4 4 2 4 4 4 3 k l k l k Viscous distructio n ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi ∂ k- k -equation equation U i = Mass Mass 0 ∂ x ∂ υ ∂ ∂ ∂ ∂ U dk k U U i = + ν + j − ε T i i ( ) ( ) ∂ σ ∂ ∂ ∂ ∂ 1 2 3 T dt x x x x x ∂ ∂ j k j j i j 1 4 2 4 4 3 4 1 4 4 4 2 4 4 4 3 dissipatio n dU 1 P Momentum Momentum ′ ′ = − − i u u Diffusion Pr oduction ρ ∂ ∂ i j dt x x ε - ε -equation equation i j 2 ∂ ε ∂ υ ∂ ε ε ∂ ∂ ε c k U 2 ∂ d U U ∂ U U 2 = + ν + − µ j ν = T i i ( ) c ( ) c ′ ′ − = ν + j − δ i ε ε u u ( ) k ∂ σ ∂ ∂ ∂ ∂ 1 T 2 dt x x k x x x k ∂ ∂ 1 2 3 ε i j T ij T ε x x 3 j j j i j 1 42 4 43 4 1 4 4 4 4 2 4 4 4 4 3 j i Distructio n Diffusion Generation ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi 5

- ε ε Model k- k Model ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi ε Model ε Model - ε - ε k- Model k- Model k k ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi 6

Algebraic Algebraic Stress Model Stress Model Algebraic Algebraic Stress Model Stress Model ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi � Eddy viscosity assumption Eddy viscosity assumption � � Isotropic eddy viscosity Isotropic eddy viscosity � � Negligible convection and diffusion � Negligible convection and diffusion of turbulent shear stress of turbulent shear stress i ′ ′ u u ~ k j � Absence of normal stress effects Absence of normal stress effects � ME 637-Particle II G. Ahmadi ME 637-Particle II G. Ahmadi 7