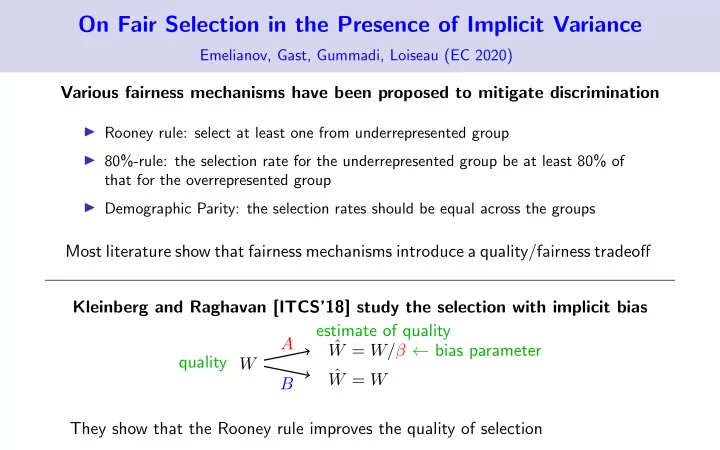
On Fair Selection in the Presence of Implicit Variance Emelianov, - PowerPoint PPT Presentation

On Fair Selection in the Presence of Implicit Variance Emelianov, Gast, Gummadi, Loiseau (EC 2020) Various fairness mechanisms have been proposed to mitigate discrimination ◮ Rooney rule: select at least one from underrepresented group ◮ 80%-rule: the selection rate for the underrepresented group be at least 80% of that for the overrepresented group ◮ Demographic Parity: the selection rates should be equal across the groups Most literature show that fairness mechanisms introduce a quality/fairness tradeoff Kleinberg and Raghavan [ITCS’18] study the selection with implicit bias estimate of quality A ˆ W = W/β ← bias parameter quality W ˆ W = W B They show that the Rooney rule improves the quality of selection

Selection with Implicit Variance Selection Problem Setup Our Model budget estimate of quality ˆ A W i ˆ W = W + ε · σ A n candidates select αn quality W ˆ W = W + ε · σ B B n A + n B x A n A + x B n B We consider two natural selection algorithms W ∼ N ( µ, σ 2 ) W ∼ N ( µ, σ 2 ) ˆ ˆ ˆ W B W B W B W W φ ˆ φ ˆ ˆ ˆ ˆ W A W A W A x B x A x B x A w ˆ w ˆ Group-Oblivious : select best Group-Fair : select best from each group ( x A ≥ γx B and x B ≥ γx A ) irrespective of their group

Our main result is that fairness mechanisms improve the quality Theorem Assume that the quality distribution is group-independent W ∼ N ( µ, σ 2 ) . For any α and γ < 1 : U d . p . > U γ -fair ≥ U g . obl . Proof Sketch ˆ ˆ ˆ W B W B W B ˆ ˆ ˆ W A W A W A w ˆ w ˆ w ˆ x A > x B x A = x B x A < x B Group-Oblivious Demographic Parity Bayesian-Optimal

We also study the cases when our assumptions are not valid Non-Gaussian Quality Distribution 3 U d . p . − U g . obl . , % Pareto(1,3) 10 2 pdf 5 1 0 0 α 1 W 0 . 2 0 . 4 0 . 6 0 . 8 1 1 1 . 2 1 . 4 1 . 6 1 . 8 2 Two-Stage Selection ˆ W i W i budget at 1st stage budget at 2nd stage n select α 1 n select α 2 n 1 stage 2 stage α 1 = α 2 4 4 2 2 U d . p . − U g . obl . U d . p . − U g . obl . 1 1 , % 2 2 , % U g . obl . U g . obl . 1 2 0 0 α 1 α 1 0 0 . 5 1 0 0 . 5 1