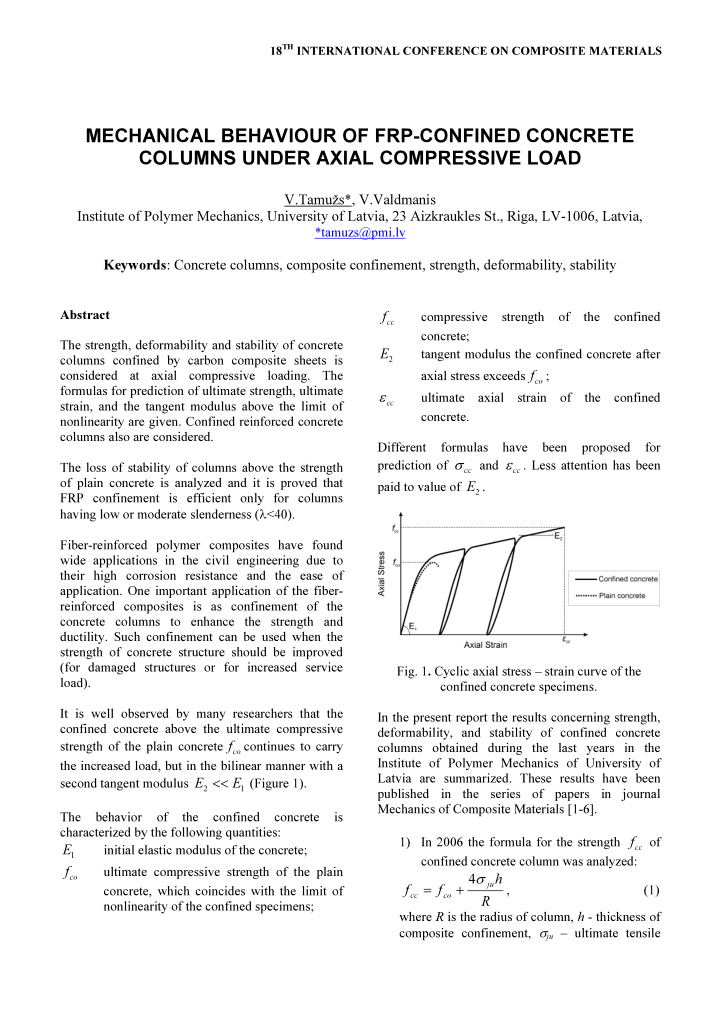



18 TH INTERNATIONAL CONFERENCE ON COMPOSITE MATERIALS MECHANICAL BEHAVIOUR OF FRP-CONFINED CONCRETE COLUMNS UNDER AXIAL COMPRESSIVE LOAD V.Tamužs*, V.Valdmanis Institute of Polymer Mechanics, University of Latvia, 23 Aizkraukles St., Riga, LV-1006, Latvia, *tamuzs@pmi.lv Keywords : Concrete columns, composite confinement, strength, deformability, stability Abstract f compressive strength of the confined cc concrete; The strength, deformability and stability of concrete E tangent modulus the confined concrete after 2 columns confined by carbon composite sheets is f considered at axial compressive loading. The axial stress exceeds ; co formulas for prediction of ultimate strength, ultimate ε cc ultimate axial strain of the confined strain, and the tangent modulus above the limit of concrete. nonlinearity are given. Confined reinforced concrete columns also are considered. Different formulas have been proposed for prediction of and . Less attention has been σ ε The loss of stability of columns above the strength cc cc of plain concrete is analyzed and it is proved that E . paid to value of 2 FRP confinement is efficient only for columns having low or moderate slenderness ( λ <40). Fiber-reinforced polymer composites have found wide applications in the civil engineering due to their high corrosion resistance and the ease of application. One important application of the fiber- reinforced composites is as confinement of the concrete columns to enhance the strength and ductility. Such confinement can be used when the strength of concrete structure should be improved (for damaged structures or for increased service Fig. 1 . Cyclic axial stress – strain curve of the load). confined concrete specimens. It is well observed by many researchers that the In the present report the results concerning strength, confined concrete above the ultimate compressive deformability, and stability of confined concrete strength of the plain concrete f continues to carry columns obtained during the last years in the co Institute of Polymer Mechanics of University of the increased load, but in the bilinear manner with a Latvia are summarized. These results have been E << E second tangent modulus (Figure 1). 2 1 published in the series of papers in journal Mechanics of Composite Materials [1-6]. The behavior of the confined concrete is characterized by the following quantities: 1) In 2006 the formula for the strength f of cc E initial elastic modulus of the concrete; 1 confined concrete column was analyzed: f ultimate compressive strength of the plain co h 4 σ ju f f , (1) concrete, which coincides with the limit of = + cc co R nonlinearity of the confined specimens; where R is the radius of column, h - thickness of composite confinement, σ ju – ultimate tensile
3) Immediately from (2) or (2 1 ) and Figure 1 strength of composite, "jacket" which should be determined very carefully [2]. follows the formula for ultimate axial strain f f − cc co , ε = ε + 2) The formula for the modulus E 2 (Figure 1) cc co E 2 was obtained in [3] which can be rewritten for carbon and basalt 0 . 65 f confinement: co = ⋅ ⋅ E 2 24 E (2) lat , 0.65 E E lat lat = + ⋅ ( − ) 0.17 . (3) ε ε ε ν ε cc co ju o co h f where E E is the "lateral modulus", but co lat = j R 0 . 44 E E j – modulus of composite jacket. lat (3 1 ) = + − 0 . 65 ( ) ε ε ε ν ε cc co ju o co f co The formula (2) was derived analyzing the expression for nondimensional differential 4) The tensile behavior of FRP material is ∆ ε lat characterized by linearly elastic stress-strain Poisson’s coefficient = as function of ν d relationship until failure. The ultimate lateral ∆ ε z stress in concrete is: the only nondimensional parameter of strength E σ = − ε f lu lat ju co of confined concrete column . The function E where - ultimate hoop strain of the FRP ε ju lat jacket. The realization of the fiber strength in a f co = is independent on scale and FRP highly depends on the fiber volume ν ν d d E lat fraction, the mean fiber strength (fixed at a concrete strength, but depends on confinement certain length), and the scatter of fiber strength properties (Figure 2). [7] in composite. However, the manufacturer does not give the information on the strength of fibers and its scatter (the Weibull parameters). Therefore, the data given by the manufacturer cannot be used without correction and the ultimate hoop strain must be determined from special tests. The most suitable test was found to be the split-disk test to estimate the hoop properties of the FRP jacket. For the carbon FRP confinement the average ultimate hoop strain d value from the split-disk test was found to ε ju be only about 0.6 of the value given by the manufacturer ε m ju [1]. However, experimental results show that the ultimate hoop strain ε ju measured directly in the confined concrete specimen test is even less than Fig. 2. Asymptotic DPR and Poisson ratio plotted in the ultimate hoop strain ε d ju determined from one graph for carbon and basalt confinements. Open symbols – DPR, closed symbols – Poisson ratio. the split-disk test. This reduction can be Solid line – approximation. explained by the following reasons: The quality of the FRP jacket – improperly • From data of basalt confinement the formula (2) aligned fibers, presence of the voids in the resin. is modified: Existence of localized deformations in the • 0 . 44 cracked concrete, which leads to a non-uniform f co (2 1 ) = ⋅ ⋅ E 6 . 19 E stress distribution in the FRP jacket and hence 2 lat E lat premature failure of the jacket.
It was found in [1] that the ultimate hoop strain values from the confined specimen tests is significantly lower than the values obtained from the split disk test. The coefficient of reduction depends on the quality of the column surface and quality of wrapping of carbon composite. According numerous data the reduction lies between 0.9 and 0.6. Therefore the ultimate hoop strain of the carbon FRP jacket will be no less than: m m 0 . 6 0 . 6 0 . 36 . (4) ε = ⋅ ε = ε ju ju ju Hereafter will be the conservative value of the ε ju Fig. 4 . Buckling of steel bar reinforcement ultimate hoop strain of the FRP jacket which accounts for the above mentioned effects. (It should E be noted, that reduction coefficient is only valid for 2 << 5) It is seen from Figure 1 that 1 . Therefore CFRP confinement. The reduction factors for the E 1 aramid (AFRP) and glass (GFRP) confinement must the danger of the confined column instability be determined separately.) f . In [5] appears when the axial stress exceeds cc the analysis of instability of confined columns In praxis only the columns reinforced by steel bars was carried out and it was found that are used. But the steel bars start to yield when the confinement is effective only for columns of stress level reaches the plain concrete strength. moderate slenderness ( λ < 40). During yielding the elastic modulus of the steel bars is almost zero and they no more contribute to the 100 stiffness of the column. The FRP jacket prevents the Column length 300mm 1 2 Column length 600mm Column length 1200mm buckling of the yielding steel bars until the failure of Column length 1500mm 80 Column length 2500mm the specimens. Critical stress σ cr [MPa] σ cr =f cc 60 40 σ cr =f co 20 0 0 20 40 60 80 100 Slenderness λ Fig. 5 . Predicted and experimentally obtained values of the critical stress as a function of the slenderness. Closed symbols – results from the confined specimen tests, Solid line – prediction of the critical Fig. 3 . Compressive behavior of confined concrete stress. Line 1 - Euler’s hyperbola, where E t =E 2 . Line columns with (1) and without (2) steel bar 2 - Euler’s hyperbola, where E t =E 1 . reinforcement. Consequently the formula for the strength of References confined reinforced columns f looks as: cc 2 [1] V. Tamužs, R. Tepfers, Chi-Sang You, T. Rousakis, 4 h σ r ju = + + f f n f , (4) I. Repelis, V. Skruls and U. Vilks "Behavior of ccr co s y R R concrete cylinders confined by carbon-composite tapes and prestressed yarns. 1. Experimental data". where r is the radius of steel bars, n – the Mechanics of Composite Materials , Vol. 42, No. 1, number of steel bars, f y – yield stress of steel [4]. pp. 13-32, 2006a.
Recommend
More recommend