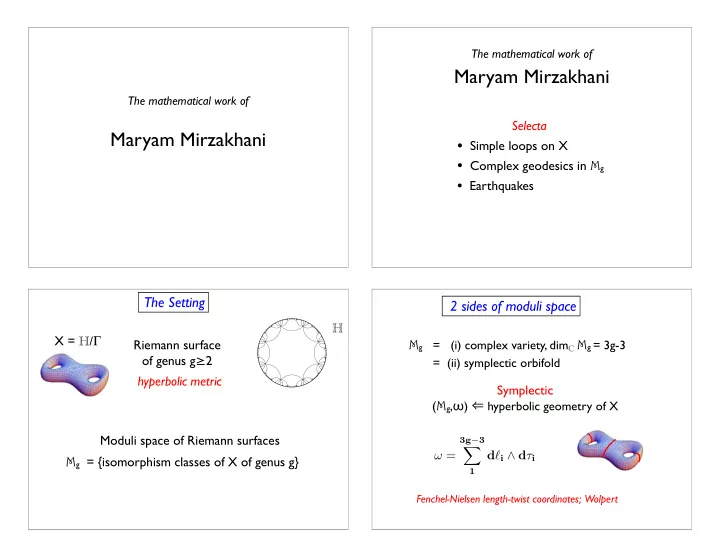

The mathematical work of Maryam Mirzakhani The mathematical work of Selecta Maryam Mirzakhani • Simple loops on X • Complex geodesics in M g • Earthquakes The Setting 2 sides of moduli space H X = H / Γ Riemann surface M g = (i) complex variety, dim C M g = 3g-3 of genus g ≥ 2 = (ii) symplectic orbifold hyperbolic metric Symplectic ( M g , ω ) ⇐ hyperbolic geometry of X Moduli space of Riemann surfaces 3g − 3 X ! = d ` i ∧ d ⌧ i M g = {isomorphism classes of X of genus g} 1 Fenchel-Nielsen length-twist coordinates; Wolpert
M g is totally inhomogeneous Complex structure on M g : inherited from X M g = T g /Mod g M g T g * T X M g = Q(X) = {holomorphic quadratic differentials q = q(z) dz 2 on X } Y Mod g X Complex structure ⇒ Teichmüller metric on M g • Aut( T g ) = Mod g ||q|| = ∫ |q(z)| |dz| 2 = area(X,|q|) • Q(X) ≃ Q(Y) ⇒ X ≃ Y X unlike S n or H n or K\G/ Γ = Kobayashi metric (Royden) ⇒ New proof of Witten conjecture Work of Mirzakhani: I Intersection numbers on moduli space: Simple loops in X Z c 1 ( L 1 ) d 1 · · · c 1 ( L n ) d n h τ d 1 , . . . , τ d n i = M g,n ⇒ solution of KdV equations / Virasoro algebra. Kontsevich, 1992 (2004) Classical ⇒ Topological statistics Theorem - Mirzakhani #Closed(X,L) ∼ e L /L E.g., probability a random simple loop in #Simple(X,L) ∼ C X L 6g-6 (prime number theorem, 1940s) genus 2 separates is 1/7. Proof: Integration over M g and hyperbolic dissection
Work of Mirzakhani: II Mirzakhani’s volume formulas Complex geodesics in M g Moduli of surfaces with geodesic boundary: Vol M g,n (L 1,..., L n ) = ∫ω N Real geodesic: (local) isometry f : R → M g P g,n (L 1,..., L n ) = = polynomial with coefficients in Q [ π ]. Complex geodesic: holomorphic isometry F : H → M g ex: P 1,1 (L) = (1/24)(L 2 + 4 π 2 ) Abundance: Previously only P g,n (0,...,0) was known. There exists complex geodesics through every p ∈ M g , in every possible direction. [Coefficients ⇒ statistics and characteristic numbers] (Teichmüller disks) Behavior of a real geodesic Behavior of a complex geodesic * Usually f( R ) is dense in M g ; ---- 2D cobweb? * But sometimes, f( R ) can be a fractal cobweb.... ....defying classification. Theorem - Mirzakhani & coworkers V = F( H ) is always an algebraic subvariety of M g. (E.g. genus 2, V = Teichmüller curve, Hilbert modular surface or whole space M 2. )
� SL 2 ( R ) Q M g Proof that V = F( H ) is an algebraic subvariety of M g. Dynamics over moduli space ↓ I. Eskin & Mirzakhani : complex geodesic = M g projection of orbit SL 2 ( R ) · (X,q) All ergodic SL 2 ( R )-invariant measures in Q M g come from special analytic varieties A ⊂ Q M g. X = P/~ A ⋅ (X,q) = (A(P)/~, dz 2 ) II. E & M & Mohammadi: q = dz 2 A All SL 2 ( R ) orbit closures come from such A. in SL 2 ( R ) III. Filip: A(P) ⊂ C Any such A ⊂ Q M g is an algebraic subvariety defined P ⊂ C over a number field. (and A projects to V.) Ramifications: Work of Mirzakhani: III Beyond Homogeneous Spaces Earthquakes Classical: Margulis, U � G / Γ G Lie group simple loop α , t ∈ R ⇒ twist( λ ,X), λ in ML g Ratner, et al Γ lattice X t = twist ( t α , X) U ⊂ G subgroup SL 2 ( R )x = Hx ⊂ G / Γ t X t ⤻ Mirzakhani SL 2 ( R ) � Q M g = Q T g /Mod g SL 2 ( R )x = A ⊂ Q T g /Mod g ⤻ α (Thurston) Rich theory of homogeneous dynamics resonates in = Hamiltonian flows on M g generated by highly inhomogeneous world of moduli spaces function X → length(X, λ )
Earthquake dynamics over M g Proof: Symplectic - Holomorphic Bridge � earthquake flow { unit length (X, λ )} = L 1 M g ↓ ( 1 1 ) t horocycle flow M g earthquake flow 0 � � Q 1 M g L 1 M g Theorem - Mirzakhani measurable ↓ ↓ isomorphism M g M g Thurston’s earthquake flow is ergodic and mixing. complex symplectic Previously, not even one dense earthquake path was known! ( ⇒ earthquake flow is ergodic) Work of Mirzakhani: Scope and perspectives ML (Dumas) breadth of methods integrated into a transformative research program many developments still unfolding
Recommend
More recommend