Lesson 1.2 Definition of Trig Functions: Let t be a real number and - PowerPoint PPT Presentation

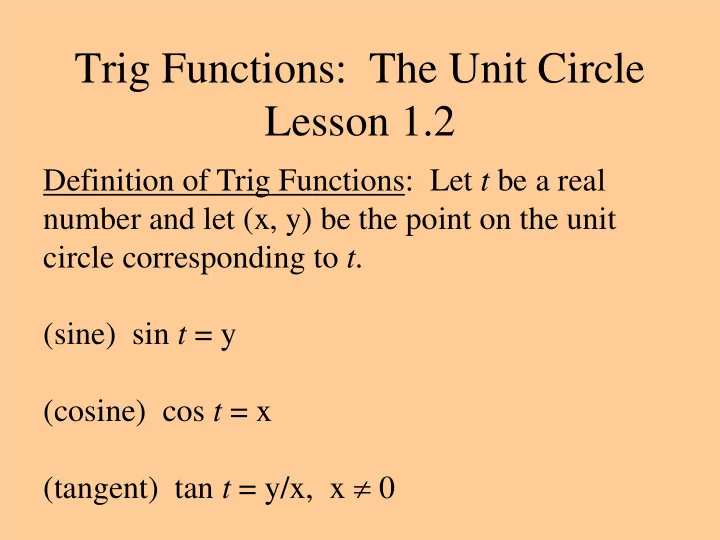
Trig Functions: The Unit Circle Lesson 1.2 Definition of Trig Functions: Let t be a real number and let (x, y) be the point on the unit circle corresponding to t . (sine) sin t = y (cosine) cos t = x (tangent) tan t = y/x, x  0

Ex 1: Find the point (x, y) on the unit circle that corresponds to the real number t . A. t =  /4 B. t = 7  /6 C. t = 4  /3 2 , − 1 3 2 2 − 1 3 2 , − 2 , − 2 2 2 Ex 2: Evaluate (if possible) the sine, cosine, and tangent of the real number. A. t =  /4 2 2 2 sin (  /4) = cos (  /4) = tan (  /4) =  1 2 2 2 2 2

Ex 2 (cont’d) : Evaluate (if possible) the sine, cosine, and tangent of the real number. −1,0 B. t =  0 sin (  ) =  1 cos (  ) = 0   0 tan (  ) = 1

Ex 2 (cont’d) : Evaluate (if possible) the sine, cosine, and tangent of the real number. − 1 3 2 , C. t = - 4  /3 2 3 2 tan (-4  /3) = 1 3  sin (-4  /3) = 2 2 3 2     1 2 1 cos (-4  /3) = 2 3   3   1

Definition of Reciprocal Trig Functions: Let t be a real number and let (x, y) be the point on the unit circle corresponding to t . (cosecant) csc t = 1/sin t = 1/y, y  0 (secant) sec t = 1/cos t = 1/x, x  0 (cotangent) cot t = 1/tan t = x/y, y  0

Ex 3: Evaluate (if possible) the six trig functions of the real number. 2 2 − 2 , A. t = 3  /4 2 2 2  2 2 sin (3  /4) = csc (3  /4) = 2  2 2 cos (3  /4) = sec (3  /4) =  2 2   2 1  1 tan (3  /4) = cot (3  /4) =    1 1

Ex 3 (cont’d) : Evaluate (if possible) the six trig functions of the real number. 0, −1 B. t = -  /2 1   1 csc (-  /2) = sin (-  /2) =  1  1 1 Undefined sec (-  /2) = cos (-  /2) = 0 0  1 0  0 cot (-  /2) = tan (-  /2) =  1 0 Undefined Homework: p.147 #6-28 even