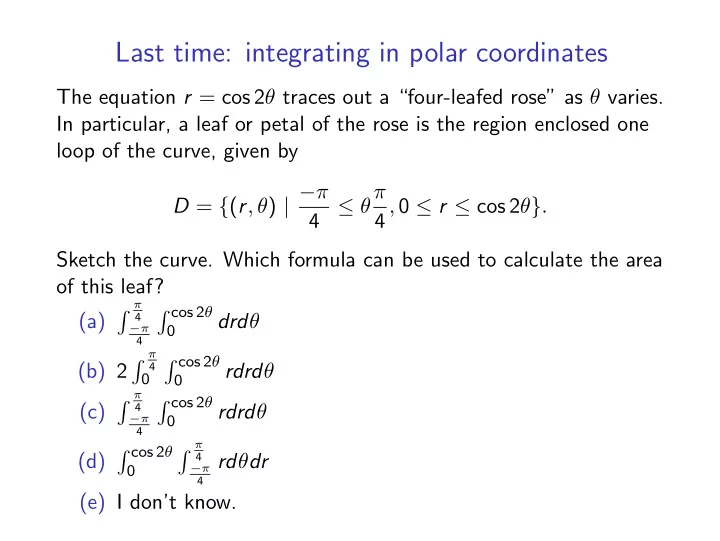
Last time: integrating in polar coordinates The equation r = cos 2 - PowerPoint PPT Presentation

Last time: integrating in polar coordinates The equation r = cos 2 θ traces out a “four-leafed rose” as θ varies. In particular, a leaf or petal of the rose is the region enclosed one loop of the curve, given by D = { ( r , θ ) | − π ≤ θπ 4 , 0 ≤ r ≤ cos 2 θ } . 4 Sketch the curve. Which formula can be used to calculate the area of this leaf? ∫︁ cos 2 θ ∫︁ π (a) 4 drd θ 0 − π 4 ∫︁ cos 2 θ ∫︁ π (b) 2 4 rdrd θ 0 0 ∫︁ cos 2 θ ∫︁ π (c) 4 rdrd θ 0 − π 4 ∫︁ cos 2 θ ∫︁ π (d) 4 4 rd θ dr 0 − π (e) I don’t know.

Practice with triple integrals We wrote E = { ( x , y , z ) | ( x , z ) ∈ D 2 , x 2 + z 2 ≤ y ≤ 4 } , where D 2 = { ( x , z ) | x 2 + z 2 ≤ 4 } . so ∫︂∫︂∫︂ V ( E ) = dV E ∫︂ 4 ∫︂∫︂ = x 2 + z 2 dy dA D 2 ∫︂∫︂ [ y ] 4 = x 2 + z 2 dA D 2 ∫︂∫︂ 4 − x 2 − z 2 dA . = D 2

Practice with triple integrals D 2 4 − x 2 − z 2 dA . We could write D 2 as ∫︁∫︁ So we need to calculate a region of type I or II, but it is easier to use polar coordinates (in the xz -plane): x = r cos θ, z = r sin θ ; ∫︂ 2 π ∫︂ 2 ∫︂∫︂ 4 − x 2 − z 2 dA = (4 − r 2 ) r drd θ D 2 0 0 ∫︂ 2 π ]︃ 2 [︃ 2 r 2 − 1 4 r 4 d θ 0 0 ∫︂ 2 π 4 d θ = 8 π. 0

Practice with triple integrals E is the region bounded by the planes x = 2 , y = 2 , z = 0 and x + y − 2 z = 2, and D is its triangular shadow in the xy -plane. Fill in the blanks, and discuss with your neighbour: D = { ( x , y ) | ? ≤ x ≤ ? , ?? ≤ y ≤ ?? } . (a) We’re working on it. (b) We’re stuck. (c) We have answers, but they’re different and we don’t know who is right. (d) We have the same answer.

Practice with triple integrals Now find u 1 ( x , y ) and u 2 ( x , y ) such that E = { ( x , y , z ) | ( x , y ) ∈ D , u 1 ( x , y ) ≤ z ≤ u 2 ( x , y ) } . (a) We’re working on it. (b) We’re stuck. (c) We have answers, but they’re different and we don’t know who is right. (d) We have the same answer.