intermediate exam ii problem 1 spring 05
play

Intermediate Exam II: Problem #1 (Spring 05) The circuit of - PowerPoint PPT Presentation

Aug 03, 2023 •44 likes •1.24k views

Intermediate Exam II: Problem #1 (Spring 05) The circuit of capacitors connected to a battery is at equilibrium. (a) Find the equivalent capacitance C eq . (b) Find the voltage V 3 across capacitor C 3 . (c) Find the the charge Q 2 on capacitor

  1. Unit Exam II: Problem #1 (Spring ’08) The circuit of capacitors is at equilibrium. (a) Find the charge Q 1 on capacitor 1 and the charge Q 2 on capacitor 2. (b) Find the voltage V 1 across capacitor 1 and the voltage V 2 across capacitor 2. (c) Find the charge Q 3 and the energy U 3 on capacitor 3. C 3 = 5 µ F Solution: « − 1 „ 1 1 C 1 = 6 µ F = 12 µ F (a) C 12 = 6 µ F + = 4 µ F , C 2 12 µ F Q 1 = Q 2 = Q 12 = (4 µ F)(12V) = 48 µ C . (b) V 1 = Q 1 = 48 µ C 12V 6 µ F = 8V , C 1 V 2 = Q 2 = 48 µ C 12 µ F = 4V . C 2 (c) Q 3 = (5 µ F)(12V) = 60 µ C , U 3 = 1 2 (5 µ F)(12V) 2 = 360 µ J . 1/5/2019 [tsl377 – 12/60]

  2. Unit Exam II: Problem #2 (Spring ’08) Consider the electric circuit shown. Find the current I 1 through resistor 1 and the voltage V 1 across it (a) when the switch S is open, (b) when the switch S is closed. (c) Find the equivalent resistance R eq of the circuit and the total power P dissipated in it when the switch S is closed. = 4Ω R 3 S = 4Ω R 1 = 2Ω R 2 12V 1/5/2019 [tsl378 – 13/60]

  3. Unit Exam II: Problem #2 (Spring ’08) Consider the electric circuit shown. Find the current I 1 through resistor 1 and the voltage V 1 across it (a) when the switch S is open, (b) when the switch S is closed. (c) Find the equivalent resistance R eq of the circuit and the total power P dissipated in it when the switch S is closed. Solution: 12V = 4Ω (a) I 1 = 4Ω + 2Ω = 2A , V 1 = (4Ω)(2A) = 8V . R 3 S (b) I 1 = 1 12V 2Ω + 2Ω = 1 . 5A , V 1 = (4Ω)(1 . 5A) = 6V . 2 „ 1 « − 1 = 4Ω 4Ω + 1 R 1 (c) R eq = + 2Ω = 4Ω , 4Ω = 2Ω R 2 P = (12V) 2 = 36W . 4Ω 12V 1/5/2019 [tsl378 – 13/60]

  4. Unit Exam II: Problem #3 (Spring ’08) Consider the electric circuit shown. Find the currents I 1 , I 2 , and I 3 (a) with the switch S open, (b) with the switch S closed. I 1 8V 2Ω 2Ω I 2 12V 2Ω S I 3 6V 1/5/2019 [tsl379 – 14/60]

  5. Unit Exam II: Problem #3 (Spring ’08) Consider the electric circuit shown. Find the currents I 1 , I 2 , and I 3 (a) with the switch S open, (b) with the switch S closed. I 1 8V 2Ω Solution: 2Ω (a) I 1 = 8V − 12V = − 1A , 4Ω I 2 12V I 2 = − I 1 = +1A . I 3 = 0 . (b) I 1 = 8V − 12V 2Ω = − 1A , S 4Ω I 3 = 6V − 12V = − 3A . I 2Ω 3 6V I 2 = − I 1 − I 3 = +4A . 1/5/2019 [tsl379 – 14/60]

  6. Unit Exam II: Problem #1 (Spring ’09) Both capacitor circuits are at equilibrium. (a) In the circuit on the left, the voltage across capacitor 1 is V 1 = 8V . Find the charge Q 1 on capacitor 1, the charge Q 2 on capacitor 2, and the voltage V 2 across capacitor 2. Find the emf E A supplied by the battery. (b) In the circuit on the right, the charge on capacitor 3 is Q 3 = 6 µ C . Find the voltage V 3 across capacitor 3, the voltage V 4 across capacitor 4, and the charge Q 4 on capacitor 4. Find the emf E B supplied by the battery. µ µ µ C = 1 F C = 2 F C = 3 F 2 1 3 µ F C = 4 4 ε ε B A 1/5/2019 [tsl392 – 15/60]

  7. Unit Exam II: Problem #1 (Spring ’09) Both capacitor circuits are at equilibrium. (a) In the circuit on the left, the voltage across capacitor 1 is V 1 = 8V . Find the charge Q 1 on capacitor 1, the charge Q 2 on capacitor 2, and the voltage V 2 across capacitor 2. Find the emf E A supplied by the battery. (b) In the circuit on the right, the charge on capacitor 3 is Q 3 = 6 µ C . Find the voltage V 3 across capacitor 3, the voltage V 4 across capacitor 4, and the charge Q 4 on capacitor 4. Find the emf E B supplied by the battery. µ µ µ C = 1 F C = 2 F C = 3 F 2 1 3 µ F C = 4 4 ε ε Solution: B A (a) Q 1 = (1 µ F)(8V) = 8 µ C , Q 2 = Q 1 = 8 µ C , V 2 = 8 µ C 2 µ F = 4V , E A = 8V + 4V = 12V . (b) V 3 = 6 µ C 3 µ F = 2V , V 4 = V 3 = 2V , Q 4 = (2V)(4 µ F) = 8 µ C , E B = V 3 = V 4 = 2V . 1/5/2019 [tsl392 – 15/60]

  8. Unit Exam II: Problem #2 (Spring ’09) Consider the resistor circuit shown. (a) Find the equivalent resistance R eq . (b) Find the power P supplied by the battery. 2Ω (c) Find the current I 4 through the 4Ω -resistor. 1Ω 1Ω (d) Find the voltage V 2 across the 2Ω -resistor. 4Ω 3Ω 3Ω 24V 1/5/2019 [tsl393 – 16/60]

  9. Unit Exam II: Problem #2 (Spring ’09) Consider the resistor circuit shown. (a) Find the equivalent resistance R eq . (b) Find the power P supplied by the battery. 2Ω (c) Find the current I 4 through the 4Ω -resistor. 1Ω 1Ω (d) Find the voltage V 2 across the 2Ω -resistor. Solution: 4Ω 3Ω 3Ω (a) R eq = 8Ω . (b) P = (24V) 2 = 72W . 8Ω 24V (c) I 4 = 1 24V 8Ω = 1 . 5A . 2 (d) V 2 = (1 . 5A)(2Ω) = 3V . 1/5/2019 [tsl393 – 16/60]

  10. Unit Exam II: Problem #3 (Spring ’09) Consider the electric circuit shown. Find the currents I 1 , I 2 , I 3 , and I 4 . 1Ω 1Ω I I 1 4 1V 3V 5V I I 2V 2 3 1Ω 1Ω 1/5/2019 [tsl394 – 17/60]

  11. Unit Exam II: Problem #3 (Spring ’09) Consider the electric circuit shown. Find the currents I 1 , I 2 , I 3 , and I 4 . 1Ω 1Ω I I 1 4 1V Solution: 3V Use loops along quadrants in assumed current directions. Start at center. 5V +3V − I 1 (1Ω) − 1V = 0 ⇒ I 1 = 2A . I I 2V 2 3 +3V − I 2 (1Ω) + 2V = 0 ⇒ I 2 = 5A . − 2V − I 3 (1Ω) + 5V = 0 ⇒ I 3 = 3A . 1Ω 1Ω +1V − I 4 (1Ω) + 5V = 0 ⇒ I 4 = 6A . 1/5/2019 [tsl394 – 17/60]

  12. Unit Exam II: Problem #1 (Spring ’11) Both capacitor circuits are at equilibrium. (a) Find the charge Q 1 on capacitor 1. (b) Find the voltage V 3 across capacitor 3. (c) Find the charge Q 2 on capacitor 2. (d) Find the energy U 4 stored on capacitor 4. C C 1 = 1pF = 4pF 4 C = 2pF 24V 2 24V C 3 = 3pF 1/5/2019 [tsl404 – 18/60]

  13. Unit Exam II: Problem #1 (Spring ’11) Both capacitor circuits are at equilibrium. (a) Find the charge Q 1 on capacitor 1. (b) Find the voltage V 3 across capacitor 3. (c) Find the charge Q 2 on capacitor 2. (d) Find the energy U 4 stored on capacitor 4. C C 1 = 1pF = 4pF 4 C = 2pF 24V 2 24V Solution: C 3 = 3pF „ 1 « − 1 + 1 (a) C 13 = = 0 . 75pF , Q 1 = Q 3 = Q 13 = (24V)(0 . 75pF) = 18pC . C 1 C 3 (b) V 3 = Q 3 = 18pC 3pF = 6V . C 3 (c) Q 2 = (24V)(2pF) = 48pC . (d) U 4 = 1 4 = 1 2 (4pF)(24V) 2 = 1152pJ . 2 C 4 V 2 1/5/2019 [tsl404 – 18/60]

  14. Unit Exam II: Problem #2 (Spring ’11) Consider the resistor circuit shown. (a) Find the current I L on the left. (b) Find the current I R on the right. (c) Find the equivalent resistance R eq of all four resistors. (d) Find the power P 2 dissipated in resistor 2. = 1Ω = 4Ω R 1 R 4 I I 24V L R = 2Ω R 2 = 3Ω R 3 1/5/2019 [tsl405 – 19/60]

  15. Unit Exam II: Problem #2 (Spring ’11) Consider the resistor circuit shown. (a) Find the current I L on the left. (b) Find the current I R on the right. (c) Find the equivalent resistance R eq of all four resistors. (d) Find the power P 2 dissipated in resistor 2. = 1Ω = 4Ω R 1 R 4 Solution: I I 24V L R 24V (a) I L = 1Ω + 3Ω = 6A . = 2Ω R 2 (b) I R = 24V 4Ω = 6A . = 3Ω R 3 « − 1 „ 1Ω + 3Ω + 1 1 2Ω + 1 (c) R eq = = 1Ω . 4Ω (d) P 2 = (24V) 2 = 288W . 2Ω 1/5/2019 [tsl405 – 19/60]

  16. Unit Exam II: Problem #3 (Spring ’11) Consider the electric circuit shown. (a) Find the current I 1 . (b) Find the current I 2 . (c) Find the current I 3 . (d) Find the potential difference V a − V b . a 5Ω 3V I 10Ω 12V 2 I I 6V 1 3 b 1/5/2019 [tsl406 – 20/60]

  17. Unit Exam II: Problem #3 (Spring ’11) Consider the electric circuit shown. (a) Find the current I 1 . (b) Find the current I 2 . (c) Find the current I 3 . (d) Find the potential difference V a − V b . a 5Ω 3V I 10Ω 12V 2 I I 6V 1 3 b Solution: ⇒ I 1 = 15V (a) 12V + 3V − I 1 (10Ω) = 0 10Ω = 1 . 5A . ⇒ I 1 = 6V (b) − 6V + 12V − I 2 (5Ω) = 0 5Ω = 1 . 2A . (c) I 3 = I 1 + I 2 = 2 . 7A . (d) V a − V b = − 6V + 12V = 6V . 1/5/2019 [tsl406 – 20/60]

  18. Unit Exam II: Problem #1 (Spring ’12) Find the equivalent capacitances C eq of the two capacitor circuits. µ 3 n F 2 F 3 n F 3 n F µ 2 F 3 n F µ µ 2 F 2 F 1/5/2019 [tsl427 – 21/60]

  19. Unit Exam II: Problem #1 (Spring ’12) Find the equivalent capacitances C eq of the two capacitor circuits. µ 3 n F 2 F 3 n F 3 n F µ 2 F 3 n F µ µ 2 F 2 F Solution: „ 1 « − 1 1 1 • C eq = 3nF + 3nF + 3nF + = 4nF . 3nF « − 1 „ 1 1 1 = 4 • C eq = 2 µ F + 2 µ F + 2 µ F + 5 µ F . 2 µ F 1/5/2019 [tsl427 – 21/60]

  20. Unit Exam II: Problem #2 (Spring ’12) Consider a parallel-plate capacitor of capacitance C = 6 pF with plates separated a distance d = 1 mm and a potential difference V = V + − V − = 3 V between them. (a) Find the magnitude E of the electric field between the plates. (b) Find the amount Q of charge on each plate. (c) Find the energy U stored on the capacitor. −Q +Q (d) Find the area A of each plate. E V V − + d 1/5/2019 [tsl428 – 22/60]

  21. Unit Exam II: Problem #2 (Spring ’12) Consider a parallel-plate capacitor of capacitance C = 6 pF with plates separated a distance d = 1 mm and a potential difference V = V + − V − = 3 V between them. (a) Find the magnitude E of the electric field between the plates. (b) Find the amount Q of charge on each plate. (c) Find the energy U stored on the capacitor. −Q +Q (d) Find the area A of each plate. E Solution: (a) E = V 3V V V − + d = 1mm = 3000V / m . d (b) Q = CV = (6pF)(3V) = 18pC . (c) U = 1 2 QV = 0 . 5(18pC)(3V) = 27pJ . (d) A = Cd (6pF)(1mm) 8 . 85 × 10 − 12 C 2 N − 1 m − 2 = 6 . 78 × 10 − 4 m 2 . = ǫ 0 1/5/2019 [tsl428 – 22/60]

  22. Unit Exam II: Problem #3 (Spring ’12) Consider the electric circuit shown. Find the currents I 1 , I 2 , I 3 , and I 4 I 2Ω 3 I 2 I 2Ω 12V 1 4Ω I 4 1/5/2019 [tsl429 – 23/60]

  23. Unit Exam II: Problem #3 (Spring ’12) Consider the electric circuit shown. Find the currents I 1 , I 2 , I 3 , and I 4 I 2Ω 3 I 2 I 2Ω 12V 1 4Ω I 4 Solution: 12V • I 1 = 2Ω + 4Ω = 2A . • I 2 = 12V 2Ω = 6A . • I 3 = I 4 = I 1 + I 2 = 8A . 1/5/2019 [tsl429 – 23/60]

  24. Unit Exam II: Problem #4 (Spring ’12) Consider the electric circuit shown. Find the currents I 1 , I 2 , and I 3 8Ω I 1 12V I 3 6V I 3V 2 4Ω 1/5/2019 [tsl430 – 24/60]

  25. Unit Exam II: Problem #4 (Spring ’12) Consider the electric circuit shown. Find the currents I 1 , I 2 , and I 3 8Ω I 1 12V I 3 6V I 3V Solution: 2 4Ω ⇒ I 1 = 9 • 12V + 6V − (8Ω) I 1 = 0 4 A = 2 . 25A . ⇒ I 2 = 3 • 6V − 3V − (4Ω) I 2 = 0 4 A = 0 . 75A . • I 3 = I 1 + I 2 = 3 . 00A . 1/5/2019 [tsl430 – 24/60]

  26. Unit Exam II: Problem #1 (Spring ’13) Consider the capacitor circuit shown at equilibrium. (a) Find the equivalent capacitance C eq . (b) Find the total energy U stored in the four capacitors. (c) Find the voltage V ∗ across the capacitor marked by an asterisk. 5nF 6nF * 20V 4nF 5nF 1nF 10V 9nF 9nF 6nF * 1/5/2019 [tsl456 – 25/60]

  27. Unit Exam II: Problem #1 (Spring ’13) Consider the capacitor circuit shown at equilibrium. (a) Find the equivalent capacitance C eq . (b) Find the total energy U stored in the four capacitors. (c) Find the voltage V ∗ across the capacitor marked by an asterisk. 5nF 6nF * 20V 4nF 5nF 1nF 10V 9nF 9nF 6nF * Solution: « − 1 « − 1 „ 1 1 1 „ 1 1 1 C eq = 5nF + 1nF + 6nF + C eq = 4nF + 5nF + 9nF + 6nF 9nF = 2nF = 3nF U = 1 U = 1 2 (2nF)(10V) 2 = 100nJ 2 (3nF)(20V) 2 = 600nJ V ∗ = 10 V ∗ = 20 3 V = 3 . 33V 3 V = 6 . 67V 1/5/2019 [tsl456 – 25/60]

  28. Unit Exam II: Problem #2 (Spring ’13) Consider the resistor circuit shown. (a) Find the equivalent resistance R eq . (b) Find the current I flowing through the battery. (c) Find the voltage V ∗ across the resistor marked by an asterisk. 6Ω 1Ω * 8Ω 8Ω 20V * 6Ω 20V 3Ω 3Ω 1Ω 1/5/2019 [tsl457 – 26/60]

  29. Unit Exam II: Problem #2 (Spring ’13) Consider the resistor circuit shown. (a) Find the equivalent resistance R eq . (b) Find the current I flowing through the battery. (c) Find the voltage V ∗ across the resistor marked by an asterisk. 6Ω 1Ω * 8Ω 8Ω 20V * 6Ω 20V 3Ω 3Ω 1Ω Solution: „ 1 „ 1 « − 1 « − 1 8Ω + 1 6Ω + 1 R eq = + 3Ω + 3Ω = 10Ω R eq = + 1Ω + 1Ω = 5Ω 8Ω 6Ω I = 20V I = 20V 10Ω = 2A 5Ω = 4A V ∗ = (1A)(8Ω) = 8V V ∗ = (2A)(6Ω) = 12V 1/5/2019 [tsl457 – 26/60]

  30. Unit Exam II: Problem #3 (Spring ’13) Consider the RC circuit shown. The switch has been closed for a long time. (a) Find the current I B flowing through the battery. (b) Find the voltage V C across the capacitor. (c) Find the charge Q on the capacitor. (d) Find the current I 3 flowing through the 3Ω -resistor right after the switch has been opened. 4Ω 4Ω 10nF 2Ω 1Ω 12V 12V 10nF 3Ω 3Ω S S 1/5/2019 [tsl458 – 27/60]

  31. Unit Exam II: Problem #3 (Spring ’13) Consider the RC circuit shown. The switch has been closed for a long time. (a) Find the current I B flowing through the battery. (b) Find the voltage V C across the capacitor. (c) Find the charge Q on the capacitor. (d) Find the current I 3 flowing through the 3Ω -resistor right after the switch has been opened. 4Ω 4Ω 10nF 2Ω 1Ω 12V 12V 10nF 3Ω 3Ω S S Solution: 12V 12V I B = 2Ω + 4Ω = 2A I B = 3Ω + 1Ω + 4Ω = 1 . 5A V C = (2A)(2Ω) = 4V V C = (1 . 5A)(3Ω + 1Ω) = 6V Q = (4V)(10nF) = 40nC Q = (6V)(10nF) = 60nC 4V 6V I 3 = 2Ω + 3Ω = 0 . 8A I 3 = 3Ω + 1Ω = 1 . 5A 1/5/2019 [tsl458 – 27/60]

  32. Unit Exam II: Problem #1 (Spring ’14) Both capacitor circuits, charged up by batteries as shown, are now at equilibrium. The charge on capacitor C 1 = 6 pF [8pF] is Q 1 = 18 pC [16pF] and charge on capacitor C 4 = 8 pF [4pf] is Q 4 = 16 pC [12pF]. (a) Find the voltage V 2 across capacitor C 2 = 4 pF . (b) Find the emf E A supplied by the battery. (c) Find the charge Q 3 on capacitor C 3 = 3 pF . (d) Find the emf E B supplied by the battery. C 1 ε ε B C 3 C 4 A C 2 1/5/2019 [tsl472 – 28/60]

  33. Unit Exam II: Problem #1 (Spring ’14) Both capacitor circuits, charged up by batteries as shown, are now at equilibrium. The charge on capacitor C 1 = 6 pF [8pF] is Q 1 = 18 pC [16pF] and charge on capacitor C 4 = 8 pF [4pf] is Q 4 = 16 pC [12pF]. (a) Find the voltage V 2 across capacitor C 2 = 4 pF . (b) Find the emf E A supplied by the battery. (c) Find the charge Q 3 on capacitor C 3 = 3 pF . (d) Find the emf E B supplied by the battery. C 1 ε ε B C 3 C 4 A C 2 Solution: V 2 = Q 2 (a) Q 2 = Q 1 = 18pC , [16pC] , = 4 . 5V [4V] . C 2 (b) E A = V 1 + V 2 = 3V + 4 . 5V = 7 . 5V [2V + 4V = 6V] . (c) V 3 = V 4 = Q 4 = 2V [3V] , Q 3 = V 3 C 3 = 6pC [9pC] . C 4 (d) E B = V 3 = V 4 = 2V [3V] . 1/5/2019 [tsl472 – 28/60]

  34. Unit Exam II: Problem #2 (Spring ’14) Consider the resistor circuit shown with R 1 = 2Ω [ 3Ω ], R 2 = 3Ω [ 2Ω ], and R 3 = 1Ω . (a) Find the current I 2 through resistor R 2 . (b) Find the voltage V 3 across resitor R 3 . (c) Find the power P 1 dissipated in resistor R 1 . (d) Find the equivalent resistance R eq . R 12V 1 R 2 R 3 1/5/2019 [tsl473 – 29/60]

  35. Unit Exam II: Problem #2 (Spring ’14) Consider the resistor circuit shown with R 1 = 2Ω [ 3Ω ], R 2 = 3Ω [ 2Ω ], and R 3 = 1Ω . (a) Find the current I 2 through resistor R 2 . (b) Find the voltage V 3 across resitor R 3 . (c) Find the power P 1 dissipated in resistor R 1 . (d) Find the equivalent resistance R eq . R 12V 1 R 2 Solution: R 3 12V » 12V – (a) I 2 = 3Ω + 1Ω = 3A 2Ω + 1Ω = 4A . (b) V 3 = (3A)(1Ω) = 3V [(4A)(1Ω) = 4V] . » (12V) 2 (c) P 1 = (12V) 2 – = 72W = 48W . 2Ω 3Ω „ 1 "„ 1 # « − 1 « − 1 1 = 4 1 = 3 (d) R eq = 2Ω + 3 Ω 3Ω + 2 Ω . 3Ω + 1Ω 2Ω + 1Ω 1/5/2019 [tsl473 – 29/60]

  36. Unit Exam II: Problem #3 (Spring ’14) Consider the electric circuit shown. Find the currents I 1 , I 2 , I 3 , I 4 when ... (a) only switch S A is closed, (a) only switch S C is closed, (b) only switch S B is closed, (b) only switch S B is closed, (c) switches S A and S B are closed. (c) switches S B and S C are closed. S B S A S C 6V 3V 2V 4V I I I I 3 1 2 4 5Ω 5Ω 5Ω 1/5/2019 [tsl474 – 30/60]

  37. Unit Exam II: Problem #3 (Spring ’14) Consider the electric circuit shown. Find the currents I 1 , I 2 , I 3 , I 4 when ... (a) only switch S A is closed, (a) only switch S C is closed, (b) only switch S B is closed, (b) only switch S B is closed, (c) switches S A and S B are closed. (c) switches S B and S C are closed. S B S A S C 6V 3V 2V 4V I I I I 3 1 2 4 5Ω 5Ω 5Ω Solution: (a) I 1 = 0 . 6A , I 2 = − 0 . 6A , I 3 = 0 , I 4 = 0 . (a) I 1 = 0 , I 2 = 0 , I 3 = − 0 . 4A , I 4 = 0 . 4A . (b) I 1 = 0 , I 2 = 0 . 2A , I 3 = − 0 . 2A , I 4 = 0 . (b) I 1 = 0 , I 2 = 0 . 2A , I 3 = − 0 . 2A , I 4 = 0 . (c) I 1 = 0 . 6A , I 2 = − 0 . 4A , (c) I 1 = 0 , I 2 = 0 . 2A , I 3 = − 0 . 2A , I 4 = 0 . I 3 = − 0 . 6A , I 4 = 0 . 4A . 1/5/2019 [tsl474 – 30/60]

  38. Unit Exam II: Problem #1 (Fall ’14) Both capacitor circuits, charged up by batteries as shown, are now at equilibrium. Each of the six capacitors has a 2pF capacitance. (a) Find the equivalent capacitance of the circuit on the left. (b) Find the voltages V 1 , V 2 , V 3 across capacitors C 1 , C 2 , C 3 , respectively. (c) Find the equivalent capacitance of the circuit on the right. (d) Find the charges Q 4 , Q 5 , Q 6 on capacitors C 4 , C 5 , C 6 , respectively. C C 1 4 C C C 3 5 2 C 12V 6 12V 1/5/2019 [tsl482 – 31/60]

  39. Unit Exam II: Problem #1 (Fall ’14) Both capacitor circuits, charged up by batteries as shown, are now at equilibrium. Each of the six capacitors has a 2pF capacitance. (a) Find the equivalent capacitance of the circuit on the left. (b) Find the voltages V 1 , V 2 , V 3 across capacitors C 1 , C 2 , C 3 , respectively. (c) Find the equivalent capacitance of the circuit on the right. (d) Find the charges Q 4 , Q 5 , Q 6 on capacitors C 4 , C 5 , C 6 , respectively. C C 1 4 C C C 3 5 2 Solution: „ 1 « − 1 C 1 12V 6 12V (a) C eq = 2pF + 2pF + = 3pF . 2pF (b) V 1 = 12V , V 2 = V 3 = 6V « − 1 „ 1 1 = 4 (c) C eq = 2pF + 2pF + 3 pF . 2pF (d) Q 45 = Q 6 = C eq (12V) = 16pC ⇒ Q 4 = Q 5 = 8pC . 1/5/2019 [tsl482 – 31/60]

  40. Unit Exam II: Problem #2 (Fall ’14) Consider the resistor circuit shown with R 1 = 5Ω , R 2 = 1Ω , and R 3 = 3Ω . (a) Find the equivalent resistance R eq . (b) Find the currents I 1 , I 2 , I 3 through resistors R 1 , R 2 , R 3 , respectively. (c) Find the voltages V 1 , V 2 , V 3 across resistors R 1 , R 2 , R 3 , respectively. R 3 R 12V 1 R 2 1/5/2019 [tsl483 – 32/60]

  41. Unit Exam II: Problem #2 (Fall ’14) Consider the resistor circuit shown with R 1 = 5Ω , R 2 = 1Ω , and R 3 = 3Ω . (a) Find the equivalent resistance R eq . (b) Find the currents I 1 , I 2 , I 3 through resistors R 1 , R 2 , R 3 , respectively. (c) Find the voltages V 1 , V 2 , V 3 across resistors R 1 , R 2 , R 3 , respectively. R 3 R 12V 1 R 2 Solution: « − 1 „ 1Ω + 3Ω + 1 1 = 20 (a) R eq = 9 Ω = 2 . 22Ω . 5Ω (b) I 1 = 12V 12V 5Ω = 2 . 4A , I 2 = I 3 = 1Ω + 3Ω = 3A . (c) V 1 = R 1 I 1 = 12V , V 2 = R 2 I 2 = 3V , V 3 = R 3 I 3 = 9V . 1/5/2019 [tsl483 – 32/60]

  42. Unit Exam II: Problem #3 (Fall ’14) Consider the two-loop circuit shown. (a) Find the current I 1 . (b) Find the current I 2 . (c) Find the potential difference V a − V b . 4V 2V a 3Ω 5Ω 6V b I I 2 1 1/5/2019 [tsl484 – 33/60]

  43. Unit Exam II: Problem #3 (Fall ’14) Consider the two-loop circuit shown. (a) Find the current I 1 . (b) Find the current I 2 . (c) Find the potential difference V a − V b . 4V 2V a 3Ω 5Ω 6V b I I 2 1 Solution: (a) I 1 = 6V − 4V = 0 . 4A . 5Ω (b) I 2 = 6V + 2V = 2 . 67A . 3Ω (c) V a − V b = 6V + 2V = 8V . 1/5/2019 [tsl484 – 33/60]

  44. Unit Exam II: Problem #1 (Spring ’15) Both capacitor circuits are at equilibrium. (a) Find the charge Q 1 on capacitor 1. (b) Find the energy U 3 stored on capacitor 3. (c) Find the charge Q 2 on capacitor 2. 24V (d) Find the voltage V 4 across capacitor 4. C = 3pF 3 C 2 = 2pF C = 1pF 1 C = 4pF 4 24V 1/5/2019 [tsl491 – 34/60]

  45. Unit Exam II: Problem #1 (Spring ’15) Both capacitor circuits are at equilibrium. (a) Find the charge Q 1 on capacitor 1. (b) Find the energy U 3 stored on capacitor 3. (c) Find the charge Q 2 on capacitor 2. 24V (d) Find the voltage V 4 across capacitor 4. C = 3pF 3 C 2 = 2pF C = 1pF 1 Solution: C = 4pF 4 (a) Q 1 = C 1 V 1 = (1pF)(24V) = 24pC . 24V (b) U 3 = 1 3 = 1 2 (3pF)(24V) 2 = 864pJ . 2 C 3 V 2 „ 1 « − 1 + 1 = 4 (c) C 24 = 3 pF , C 2 C 4 „ 4 « Q 2 = Q 4 = Q 24 = C 24 V 24 = 3 pF (24V) = 32pC . (d) V 4 = Q 4 = 32pC 4pF = 8V . C 4 1/5/2019 [tsl491 – 34/60]

  46. Unit Exam II: Problem #2 (Spring ’15) In the two resistor circuits shown find the equivalent resistances R 123 (left) and R 456 (right). Then find the currents I 1 , I 2 , I 3 through the individual resistors on the left. and the currents I 4 , I 5 , I 6 through the individual resistors on the right. 2Ω 2Ω R = R = 4 1 2Ω R = 2Ω 2Ω 2Ω R = R = R = 2 3 5 6 14V 14V 1/5/2019 [tsl492 – 35/60]

  47. Unit Exam II: Problem #2 (Spring ’15) In the two resistor circuits shown find the equivalent resistances R 123 (left) and R 456 (right). Then find the currents I 1 , I 2 , I 3 through the individual resistors on the left. and the currents I 4 , I 5 , I 6 through the individual resistors on the right. 2Ω 2Ω R = R = 4 1 2Ω R = 2Ω 2Ω 2Ω R = R = R = 2 3 5 6 14V 14V Solution: „ 1 « − 1 2Ω + 1 = 4 • R 23 = 2Ω + 2Ω = 4Ω , R 123 = 3 Ω 4Ω „ 1 « − 1 2Ω + 1 • R 45 = = 1Ω , R 456 = R 45 + R 6 = 3Ω 2Ω • I 1 = 14V I 2 = I 3 = 14V 2Ω = 7A , 4Ω = 3 . 5A • I 6 = I 45 = 14V I 4 = I 5 = 1 3Ω = 4 . 67A , 2 I 6 = 2 . 33A 1/5/2019 [tsl492 – 35/60]

  48. Unit Exam II: Problem #3 (Spring ’15) In the circuit shown find the currents I 1 , I 2 , and the potential difference V b − V a (a) if the switch S is open, (b) if the switch S is closed. 8V 4V a S 3Ω 2Ω b I I 2 1 1/5/2019 [tsl493 – 36/60]

  49. Unit Exam II: Problem #3 (Spring ’15) In the circuit shown find the currents I 1 , I 2 , and the potential difference V b − V a (a) if the switch S is open, (b) if the switch S is closed. 8V 4V a S 3Ω 2Ω b I I 2 1 Solution: (a) I 1 = I 2 = 12V 5Ω = 2 . 4A V b − V a = 8V − (2 . 4A)(2Ω) = − 4V + (2 . 4A)(3Ω) = 3 . 2V . (b) I 1 = 8V I 2 = 4V 2Ω = 4A , 3Ω = 1 . 33A , V b − V a = 0 . 1/5/2019 [tsl493 – 36/60]

  50. Unit Exam II: Problem #1 (Fall ’15) Consider the capacitor circuit shown at equilibrium. (a) Find the equivalent capacitance C eq . (b) Find the total energy U stored in the three capacitors. (c) Find the voltage V ∗ across the capacitor marked by an asterisk. (d) Find the voltage V 1 across the 1nF-capacitor. 6V 8V 4nF * 2nF 3nF 3nF * 1nF 1nF 1/5/2019 [tsl515 – 37/60]

  51. Unit Exam II: Problem #1 (Fall ’15) Consider the capacitor circuit shown at equilibrium. (a) Find the equivalent capacitance C eq . (b) Find the total energy U stored in the three capacitors. (c) Find the voltage V ∗ across the capacitor marked by an asterisk. (d) Find the voltage V 1 across the 1nF-capacitor. 6V 8V 4nF * 2nF 3nF 3nF * 1nF 1nF Solution: « − 1 « − 1 „ 1 1 „ 1 1 (a) C eq = 1nF + 2nF + = 1 . 5nF (a) C eq = 3nF + 1nF + = 2nF 3nF 4nF (b) U = 1 (b) U = 1 2 (1 . 5nF)(6V) 2 = 27nJ 2 (2nF)(8V) 2 = 64nJ (c) V ∗ = 1 (c) V ∗ = 1 26V = 3V 2 8V = 4V (d) V 1 = 6V − 3V = 3V (d) V 1 = 8V − 4V = 4V 1/5/2019 [tsl515 – 37/60]

  52. Unit Exam II: Problem #2 (Fall ’15) Consider the resistor circuit shown. (a) Find the equivalent resistance R eq . (b) Find the currents I 1 and I 2 . (c) Find the power P supplied by the battery. I 6V I 8V 1 3Ω 1 4Ω 2Ω 3Ω 2Ω 4Ω I I 2 2 1/5/2019 [tsl516 – 38/60]

  53. Unit Exam II: Problem #2 (Fall ’15) Consider the resistor circuit shown. (a) Find the equivalent resistance R eq . (b) Find the currents I 1 and I 2 . (c) Find the power P supplied by the battery. I 6V I 8V 1 3Ω 1 4Ω 2Ω 3Ω 2Ω 4Ω I I 2 2 Solution: „ 1 „ 1 « − 1 « − 1 4Ω + 1 2Ω + 1 (a) R eq = + 3Ω = 5Ω (a) R eq = + 3Ω = 4Ω 4Ω 2Ω (b) I 1 = 6V I 2 = 1 (b) I 1 = 8V I 2 = 1 5Ω = 1 . 2A , 2 I 1 = 0 . 6A 4Ω = 2A , 2 I 1 = 1A (c) P = (1 . 2A)(6V) = 7 . 2W (c) P = (2A)(8V) = 16W 1/5/2019 [tsl516 – 38/60]

  54. Unit Exam II: Problem #3 (Fall ’15) Consider the electric circuit shown. Find the currents I 1 , I 2 , I 3 . 2Ω 3Ω 2Ω 3Ω 3V 3V 12V 3V 12V 3V I I I I I I 2 3 2 3 1 1 1/5/2019 [tsl517 – 39/60]

  55. Unit Exam II: Problem #3 (Fall ’15) Consider the electric circuit shown. Find the currents I 1 , I 2 , I 3 . 2Ω 3Ω 2Ω 3Ω 3V 3V 12V 3V 12V 3V I I I I I I 2 3 2 3 1 1 Solution: 12V − I 2 (2Ω) − 3V = 0 12V − I 2 (2Ω) + 3V = 0 ⇒ I 2 = 9V ⇒ I 2 = 15V 2Ω = 4 . 5A 2Ω = 7 . 5A . 12V − I 3 (3Ω) + 3V = 0 12V − I 3 (3Ω) − 3V = 0 ⇒ I 3 = 15V ⇒ I 3 = 9V 3Ω = 5A . 3Ω = 3A . I 1 = I 2 + I 3 = 9 . 5A I 1 = I 2 + I 3 = 10 . 5A 1/5/2019 [tsl517 – 39/60]

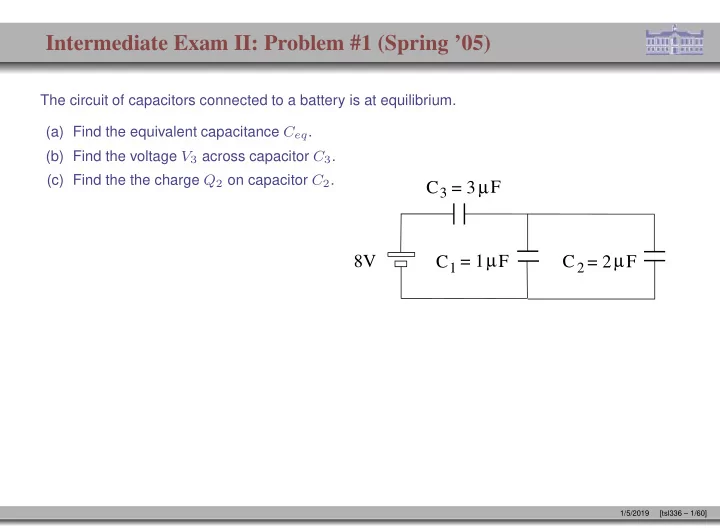
  56. Unit Exam II: Problem #1 (Spring ’16) The circuit of capacitors connected to a battery is at equilibrium. (a) Find the equivalent capacitance C eq . (b) Find the total energy U stored in the three capacitors. (c) Find the charge Q 6 on the capacitor on the left. (d) Find the the voltages V 2 and V 4 across the two capacitor on the right. 2 µ F 6 µ F 4 µ F 8V 1/5/2019 [tsl529 – 40/60]

  57. Unit Exam II: Problem #1 (Spring ’16) The circuit of capacitors connected to a battery is at equilibrium. (a) Find the equivalent capacitance C eq . (b) Find the total energy U stored in the three capacitors. (c) Find the charge Q 6 on the capacitor on the left. (d) Find the the voltages V 2 and V 4 across the two capacitor on the right. Solution: 2 µ F 6 µ F « − 1 „ 1 1 4 µ F (a) C eq = 2 µ F + 4 µ F + = 3 µ F . 6 µ F 8V (b) U = 1 2 (3 µ F)(8V) 2 = 96 µ J . (c) Q 6 = (8V)(3 µ F) = 24 µ C . (d) V 2 = V 4 = 1 2 (8V) = 4V . 1/5/2019 [tsl529 – 40/60]

  58. Unit Exam II: Problem #2 (Spring ’16) Consider the electrical circuit shown. (a) Find the current I 1 when the switch S is open. (b) Find the currents I 1 and I 2 when the switch S is closed. 2Ω 3Ω 4V 5Ω S I 2 4Ω I 1 6V 1/5/2019 [tsl530 – 41/60]

  59. Unit Exam II: Problem #2 (Spring ’16) Consider the electrical circuit shown. (a) Find the current I 1 when the switch S is open. (b) Find the currents I 1 and I 2 when the switch S is closed. 2Ω 3Ω 4V 5Ω S Solution: I 2 6V − 4V (a) I 1 = 4Ω + 5Ω + 3Ω + 2Ω = 0 . 143A . 4Ω I 1 6V 6V 4V (b) I 1 = 4Ω + 5Ω = 0 . 667A , I 2 = 3Ω + 2Ω = 0 . 8A . 1/5/2019 [tsl530 – 41/60]

  60. Unit Exam II: Problem #3 (Spring ’15) This RC circuit has been running for a long time with the switch open. (a) Find the current I while the switch is still open. (b) Find the current I right after the switch has been closed. (c) Find the current I a long time later. (d) Find the charge Q on the capacitor also a long time later. 2Ω 4Ω S 12V 7nF I 1/5/2019 [tsl531 – 42/60]

  61. Unit Exam II: Problem #3 (Spring ’15) This RC circuit has been running for a long time with the switch open. (a) Find the current I while the switch is still open. (b) Find the current I right after the switch has been closed. (c) Find the current I a long time later. (d) Find the charge Q on the capacitor also a long time later. 2Ω Solution: 4Ω S 12V 12V (a) I = 2Ω + 4Ω = 2A . 7nF I (b) I = 12V 2Ω = 6A . 12V (c) I = 2Ω + 4Ω = 2A . (d) Q = (8V)(7nF) = 56nC . 1/5/2019 [tsl531 – 42/60]

  62. Unit Exam II: Problem #1 (Fall ’16) The capacitors (initially discharged) have been connected to the battery. The circuit is now at equilibrium. Find ... (a) the voltage V 2 across capacitor C 2 , (a) the voltage V 4 across capacitor C 4 , (b) the energy U 5 on capacitor C 5 , (b) the energy U 7 on capacitor C 7 , (c) the charge Q 3 on capacitor C 3 , (c) the charge Q 6 on capacitor C 6 , (d) the equivalent capacitance C eq . (d) the equivalent capacitance C eq . C 4 = 4 µ F 12V C 3 = 3 µ F C 5 = 5 µ F µ C 6 = 6 µ F C = 6 F 6 C 7 = 7 µ F C 3 = 3 µ F C 2 = 2 µ F 18V 1/5/2019 [tsl538 – 43/60]

  63. Unit Exam II: Problem #1 (Fall ’16) The capacitors (initially discharged) have been connected to the battery. The circuit is now at equilibrium. Find ... (a) the voltage V 2 across capacitor C 2 , (a) the voltage V 4 across capacitor C 4 , (b) the energy U 5 on capacitor C 5 , (b) the energy U 7 on capacitor C 7 , (c) the charge Q 3 on capacitor C 3 , (c) the charge Q 6 on capacitor C 6 , (d) the equivalent capacitance C eq . (d) the equivalent capacitance C eq . Solution: (a) V 2 = 12V . (a) V 4 = 18V . (b) U 5 = 1 (b) U 7 = 1 2 (5 µ F)(12V) 2 = 360 µ J . 2 (7 µ F)(18V) 2 = 1134 µ J . (c) C 36 = 2 µ F (c) C 36 = 2 µ F ⇒ Q 3 = Q 36 = (12V)(2 µ F) = 24 µ C . ⇒ Q 6 = Q 36 = (18V)(2 µ F) = 36 µ C . (d) C eq = C 5 + C 36 + C 2 = 9 µ F . (d) C eq = C 4 + C 36 + C 7 = 13 µ F . 1/5/2019 [tsl538 – 43/60]

  64. Unit Exam II: Problem #2 (Fall ’16) This resistor circuit is in a state of steady currents. Find ... (a) the voltage V 2 across resistor R 2 , (a) the voltage V 3 across resistor R 3 , (b) the power P 4 dissipated in resistor R 4 , (b) the power P 6 dissipated in resistor R 6 , (c) the current I 3 flowing through resistor R 3 (c) the current I 4 flowing through resistor R 4 , (d) the equivalent resistance R eq . (d) the equivalent resistance R eq . 3Ω 18V R = 3 4Ω R = 2Ω R = 4 2 3Ω 4Ω R = R = 3 4 1Ω 6Ω R = R = 1 6 2Ω R = 12V 2 1/5/2019 [tsl539 – 44/60]

  65. Unit Exam II: Problem #2 (Fall ’16) This resistor circuit is in a state of steady currents. Find ... (a) the voltage V 2 across resistor R 2 , (a) the voltage V 3 across resistor R 3 , (b) the power P 4 dissipated in resistor R 4 , (b) the power P 6 dissipated in resistor R 6 , (c) the current I 3 flowing through resistor R 3 (c) the current I 4 flowing through resistor R 4 , (d) the equivalent resistance R eq . (d) the equivalent resistance R eq . Solution: (a) V 2 = 18V . (a) V 3 = 12V (b) P 4 = 18V 2 (b) P 6 = 12V 2 = 81W . = 24W . 4Ω 6Ω 18V 12V (c) I 3 = 3Ω + 1Ω = 4 . 5A . (c) I 4 = 2Ω + 4Ω = 2A . „ 1 „ 1 « − 1 « − 1 1Ω + 3Ω + 1 1 2Ω + 4Ω + 1 1 (d) R eq = 4Ω + = 1Ω . (d) R eq = 3Ω + = 1 . 5Ω 2Ω 6Ω 1/5/2019 [tsl539 – 44/60]

  66. Unit Exam II: Problem #3 (Fall ’16) This two-loop resistor circuit is in a state of steady currents. Find ... (a) the current I 1 , (b) the current I 2 , (c) the potential difference V a − V b . 7V 11V 7V 11V a a I I I I 5V 5V 1 2 1 2 b b 6Ω 8Ω 8Ω 6Ω 1/5/2019 [tsl540 – 45/60]

  67. Unit Exam II: Problem #3 (Fall ’16) This two-loop resistor circuit is in a state of steady currents. Find ... (a) the current I 1 , (b) the current I 2 , (c) the potential difference V a − V b . 7V 11V 7V 11V a a I I I I 5V 5V 1 2 1 2 b b 6Ω 8Ω 8Ω 6Ω Solution: (a) I 1 = 5V + 7V (a) I 1 = 7V − 5V = +1 . 5A . = +0 . 333A . 8Ω 6Ω (b) I 2 = 5V + 11V (b) I 2 = 5V + 11V = +2 . 67A . = +2A . 6Ω 8Ω (c) V a − V b = − 7V + 11V = +4V . (c) V a − V b = 7V + 11V = +18V . 1/5/2019 [tsl540 – 45/60]

  68. Unit Exam II: Problem #1 (Spring ’17) The capacitors (initially discharged) have been connected to the battery. The circuit is now at equilibrium. Find ... (a) the charge Q 4 on the 4pF-capacitor, (a) the charge Q 3 on the 3pF-capacitor, (b) the energy U 7 on the 7pF-capacitor, (b) the energy U 5 on the 5pF-capacitor, (c) the voltage V 10 across the upper (c) the voltage V 8 across the lower 10pF-capacitor, 8pF-capacitor, (d) the equivalent capacitance C eq . (d) the equivalent capacitance C eq . 4pF 10pF 8pF 9V 7pF 5pF 6V 10pF 8pF 3pF 1/5/2019 [tsl548 – 46/60]

  69. Unit Exam II: Problem #1 (Spring ’17) The capacitors (initially discharged) have been connected to the battery. The circuit is now at equilibrium. Find ... (a) the charge Q 4 on the 4pF-capacitor, (a) the charge Q 3 on the 3pF-capacitor, (b) the energy U 7 on the 7pF-capacitor, (b) the energy U 5 on the 5pF-capacitor, (c) the voltage V 10 across the upper (c) the voltage V 8 across the lower 10pF-capacitor, 8pF-capacitor, (d) the equivalent capacitance C eq . (d) the equivalent capacitance C eq . Solution: (a) Q 4 = (6V)(4pF) = 24pC . (a) Q 3 = (9V)(3pF) = 27pC . (b) U 7 = 1 (b) U 5 = 1 2 (7pF)(6V) 2 = 126pJ . 2 (5pF)(9V) 2 = 202 . 5pJ . (c) V 10 = 1 (c) V 8 = 1 2 6V = 3V . 2 9V = 4 . 5V . (d) C eq = 4pF + 7pF + 5pF = 16pF . (d) C eq = 3pF + 5pF + 4pF = 12pF . 1/5/2019 [tsl548 – 46/60]

  70. Unit Exam II: Problem #2 (Spring ’17) Consider this circuit with two terminals, four resistors, and one switch. (a) Find the equivalent resistance R (open) when the switch is open. eq (b) Find the equivalent resistance R (closed) when the switch is closed. eq 2Ω 1Ω 3Ω 1Ω S S 1Ω 2Ω 1Ω 3Ω 1/5/2019 [tsl549 – 47/60]

  71. Unit Exam II: Problem #2 (Spring ’17) Consider this circuit with two terminals, four resistors, and one switch. (a) Find the equivalent resistance R (open) when the switch is open. eq (b) Find the equivalent resistance R (closed) when the switch is closed. eq 2Ω 1Ω 3Ω 1Ω S S 1Ω 2Ω 1Ω 3Ω Solution: « − 1 « − 1 „ 1 1 = 3 „ 1 1 R (open) R (open) = 1Ω + 2Ω + 2 Ω . = 1Ω + 3Ω + = 2Ω . eq eq 1Ω + 2Ω 1Ω + 3Ω „ 1 „ 1 „ 1 „ 1 « − 1 « − 1 « − 1 « − 1 1Ω + 1 1Ω + 1 1Ω + 1 1Ω + 1 R (closed) R (closed) = + = + eq eq 2Ω 2Ω 3Ω 3Ω = 4 = 3 3 Ω . 2 Ω . 1/5/2019 [tsl549 – 47/60]

  72. Unit Exam II: Problem #3 (Spring ’17) Consider this circuit with two batteries, two resistors, and one switch. (a) Find the current I when the switch is open. (b) Find the current I when the switch is closed. (c) Find the potential difference V a − V b when the switch is open. (d) Find the potential difference V a − V b when the switch is closed. 15V 16V 15V 12V a a S S I I b b 5Ω 6Ω 5Ω 2Ω 1/5/2019 [tsl550 – 48/60]

  73. Unit Exam II: Problem #3 (Spring ’17) Consider this circuit with two batteries, two resistors, and one switch. (a) Find the current I when the switch is open. (b) Find the current I when the switch is closed. (c) Find the potential difference V a − V b when the switch is open. (d) Find the potential difference V a − V b when the switch is closed. Solution: (a) I = 15V (a) I = 16V 5Ω = 3A . 2Ω = 8A . (b) I = 15V 5Ω + 12V (b) I = 16V 2Ω + 15V 6Ω = 3A + 2A = 5A . 5Ω = 8A + 3A = 11A . (c) V a − V b = 12V . (c) V a − V b = 15V . (d) V a − V b = 0 . (d) V a − V b = 0 . 1/5/2019 [tsl550 – 48/60]

  74. Unit Exam II: Problem #1 (Fall ’17) This circuit is at equilibrium. • Find the charge Q 7 on capacitor C 7 [ Q 5 on C 5 ]. • Find the energy U 5 on capacitor C 5 [ U 7 on C 7 ]. • Find the voltages V 2 , V 4 across capacitors C 2 , C 4 [ V 3 , V 6 across C 3 , C 6 ]. C 5 = 5 µ F µ F C = 2 C 3 = 3 µ F 2 24V C 4 = 4 µ F = 6 µ F C 6 C 7 = 7 µ F 1/5/2019 [tsl557 – 49/60]

  75. Unit Exam II: Problem #1 (Fall ’17) This circuit is at equilibrium. • Find the charge Q 7 on capacitor C 7 [ Q 5 on C 5 ]. • Find the energy U 5 on capacitor C 5 [ U 7 on C 7 ]. • Find the voltages V 2 , V 4 across capacitors C 2 , C 4 [ V 3 , V 6 across C 3 , C 6 ]. C 5 = 5 µ F µ F C = 2 C 3 = 3 µ F 2 24V C 4 = 4 µ F = 6 µ F C 6 C 7 = 7 µ F Solution: • Q 7 = (24V)(7 µ F) = 168 µ C [ Q 5 = (24V)(5 µ F) = 120 µ C] • » – • U 5 = 1 U 7 = 1 2 (5 µ F)(24V) 2 = 1440 µ J 2 (7 µ F)(24V) 2 = 2016 µ J • V 2 + V 4 = 24V , V 2 C 2 = V 4 C 4 ⇒ V 2 = 16V , V 4 = 8V [ V 3 + V 6 = 24V , V 3 C 3 = V 6 C 6 ⇒ V 3 = 16V , V 6 = 8V] 1/5/2019 [tsl557 – 49/60]

  76. Unit Exam II: Problem #2 (Fall ’17) Consider the resistor circuit on the left [right]. Find the currents I 1 , I 2 [ I 3 , I 4 ] and the potential difference V a − V b [ V c − V d ] (a) when the switch S w [ S y ] is open, (b) when the switch S w [ S y ] is closed 3Ω 5Ω 6Ω 4Ω b a d c S w S y 3V 6V 2V 5V I I I 3 I 1 2 4 1/5/2019 [tsl558 – 50/60]

  77. Unit Exam II: Problem #2 (Fall ’17) Consider the resistor circuit on the left [right]. Find the currents I 1 , I 2 [ I 3 , I 4 ] and the potential difference V a − V b [ V c − V d ] (a) when the switch S w [ S y ] is open, (b) when the switch S w [ S y ] is closed 3Ω 5Ω 6Ω 4Ω b a d c S w S y 3V 6V 2V 5V I I I 3 I 1 2 4 Solution: (a) I 1 = I 2 = 3V + 6V 5Ω + 3Ω = 1 . 125A , V a − V b = 9 V. » I 3 = I 4 = 2V + 5V – 6Ω + 4Ω = 0 . 7A , V c − V d = 7 V. (b) I 1 = 3V I 2 = 6V 5Ω = 0 . 6A , 3Ω = 2A , V a − V b = 9 V. » I 3 = 5V I 4 = 2V – 4Ω = 1 . 25A , 6Ω = 0 . 333A , V c − V d = 7 V. 1/5/2019 [tsl558 – 50/60]

Recommend

y 0.4m x B 2/5/2019 [tsl342 1/69] Intermediate Exam III: Problem #1 (Spring 05) An

y 0.4m x B 2/5/2019 [tsl342 1/69] Intermediate Exam III: Problem #1 (Spring 05) An

Intermediate Exam III: Problem #1 (Spring 05) An infinitely long straight current of magnitude I = 6 A is directed into the plane ( ) and located a distance d = 0 . 4 m from the coordinate origin (somewhere on the dashed circle). The magnetic

1.92k views • 142 slides
Intermediate Exam I: Problem #1 (Spring 05) The electric field E generated by the two point

Intermediate Exam I: Problem #1 (Spring 05) The electric field E generated by the two point

Intermediate Exam I: Problem #1 (Spring 05) The electric field E generated by the two point charges, 3nC and q 1 (unknown), has the direction shown. (a) Find the magnitude of E . y (b) Find the value of q 1 . E 45 o x 4m q 1 2m 3nC

1.55k views • 122 slides
Question: 1 2 3 4 5 Total Points: 12 13 12 4 9 50 SOC 301 - Spring 2017 Midterm Exam

Question: 1 2 3 4 5 Total Points: 12 13 12 4 9 50 SOC 301 - Spring 2017 Midterm Exam

SOC 301 Name: KEY Social Statistics April 11, 2017 Professor Chester Ismay Midterm Exam Read carefully through each problem. Take your time and relax. Please write your name at the top of each page of the exam. You are to work on

172 views • 5 slides
Page 2 NAME: Practice Exam 2 Problem 1 (4 points) Use the axioms of probability to prove that P

Page 2 NAME: Practice Exam 2 Problem 1 (4 points) Use the axioms of probability to prove that P

UNIVERSITY OF ILLINOIS AT URBANA-CHAMPAIGN Department of Electrical and Computer Engineering CS 440/ECE 448 Artificial Intelligence Spring 2020 PRACTICE EXAM 2 Actual Exam will be held on Compass, Monday, March 30, 2020 This is will be an

229 views • 21 slides
FRUITLAND INTERMEDIATE SPRING INTERNSHIP BY: RYAN DILLON CLIENT Fruitland Intermediate

FRUITLAND INTERMEDIATE SPRING INTERNSHIP BY: RYAN DILLON CLIENT Fruitland Intermediate

FRUITLAND INTERMEDIATE SPRING INTERNSHIP BY: RYAN DILLON CLIENT Fruitland Intermediate School Ms. White, a 4 th grade mathematics teacher WHAT WAS NEEDED Each week Ms. White would test her students by giving them weekly math facts

446 views • 16 slides
Page 2 NAME: Exam 1 Problem 1 (4 points) Give one reason why a test of intelligence should

Page 2 NAME: Exam 1 Problem 1 (4 points) Give one reason why a test of intelligence should

UNIVERSITY OF ILLINOIS AT URBANA-CHAMPAIGN Department of Electrical and Computer Engineering CS 440/ECE 448 Artificial Intelligence Spring 2020 PRACTICE EXAM 1 Monday, February 24, 2020 This is will be a CLOSED BOOK exam. Book and notes are

374 views • 16 slides
Comp 212 - Intermediate Programming EXAM #1 February 12, 2003 Rice University - Instructors: Cox

Comp 212 - Intermediate Programming EXAM #1 February 12, 2003 Rice University - Instructors: Cox

Comp 212 - Intermediate Programming EXAM #1 February 12, 2003 Rice University - Instructors: Cox & Nguyen NAME: ______________________ Instructions 1. This exam is conducted under the Rice Honor Code. It is a closed-notes, closed-book

332 views • 8 slides
CS 4410 Spring 2016 Prelim 2 April 28, 2016 Full Name: NetID: Problem Points Score 1 10 2

CS 4410 Spring 2016 Prelim 2 April 28, 2016 Full Name: NetID: Problem Points Score 1 10 2

CS 4410 Spring 2016 Prelim 2 April 28, 2016 Full Name: NetID: Problem Points Score 1 10 2 20 3 16 4 15 5 12 6 12 7 15 Total 100 The exam is 11 pages long. There are 7 problems in the exam. You will Instructions. have 120

480 views • 11 slides
Exam Review 1 logistical note post-exam stack smashing assignment due two weeks after spring

Exam Review 1 logistical note post-exam stack smashing assignment due two weeks after spring

Exam Review 1 logistical note post-exam stack smashing assignment due two weeks after spring break (was one on schedule, but) likely harder than tricky will count for more 2 exam format around 20 question parts mostly multiple choice

634 views • 41 slides
CSC 151 Spring 2020 Topic: Project Introduction April 17, 2020 Day 32 Exam 3 Reminder Exam 3

CSC 151 Spring 2020 Topic: Project Introduction April 17, 2020 Day 32 Exam 3 Reminder Exam 3

CSC 151 Spring 2020 Topic: Project Introduction April 17, 2020 Day 32 Exam 3 Reminder Exam 3 epilogue and cover sheet are due tonight (Friday) at 10:30 pm Central Time! CSC 151 Project Project Overview Find and use a new data set Do some

224 views • 8 slides
Prelim 2 Review Spring 2019 Exam Info 4/21/19 Prelim 2 Review 2 What is on the Exam?

Prelim 2 Review Spring 2019 Exam Info 4/21/19 Prelim 2 Review 2 What is on the Exam?

CS 1110 Prelim 2 Review Spring 2019 Exam Info 4/21/19 Prelim 2 Review 2 What is on the Exam? Questions from the following topics: Iteration and Lists, Dictionaries, Tuples Nested lists, nested loops Recursion Classes &

765 views • 39 slides
Drowsiness problem The estimation of Drowsiness is an intermediate condition between

Drowsiness problem The estimation of Drowsiness is an intermediate condition between

Drowsiness problem The estimation of Drowsiness is an intermediate condition between alertness and sleep which reduces the consciousness level and hinder a person drowsiness under different to respond quickly (Awais, Badruddin, &

62 views • 4 slides
SI232 Computer Architecture PRACTICE Final Exam Name ______________________________

SI232 Computer Architecture PRACTICE Final Exam Name ______________________________

Notes for Spring 2006 Below is part of an old final exam. The emphasis with this course was somewhat different, so irrelevant material was removed. Expect additional material on: 1. As described before the 6 week exam (see calendar for Feb

255 views • 12 slides
Why study algorithms? Their impact is broad and far-reaching. Internet. Web search, packet

Why study algorithms? Their impact is broad and far-reaching. Internet. Web search, packet

COS 226, SPRING 2014 A LGORITHMS AND D ATA S TRUCTURES K EVIN W AYNE http://www.princeton.edu/~cos226 COS 226 course overview What is COS 226? Intermediate-level survey course. Programming and problem solving, with applications.

370 views • 25 slides
Duke University CompSci 514 Quiz 2 Spring 2015 Name (Print): , (Family name) (Given name)

Duke University CompSci 514 Quiz 2 Spring 2015 Name (Print): , (Family name) (Given name)

Duke University CompSci 514 Quiz 2 Spring 2015 Name (Print): , (Family name) (Given name) Student ID Number: Date of Exam: March 31, 2015 Time Period: 6:30pm-7:50pm Number of Exam Pages: 9 (including this cover sheet) Exam Type: open

325 views • 9 slides
CMSC 430 Introduction to Compilers Spring 2015 Intermediate Representations and Bytecode

CMSC 430 Introduction to Compilers Spring 2015 Intermediate Representations and Bytecode

CMSC 430 Introduction to Compilers Spring 2015 Intermediate Representations and Bytecode Formats Introduction Front end Source AST/IR Lexer Parser Types code IR2 IRn IRn .s Middle end Back end Front end syntax recognition,

379 views • 24 slides
CMSC 430 Introduction to Compilers Spring 2016 Intermediate Representations and Bytecode

CMSC 430 Introduction to Compilers Spring 2016 Intermediate Representations and Bytecode

CMSC 430 Introduction to Compilers Spring 2016 Intermediate Representations and Bytecode Formats Introduction Front end Source AST/IR Lexer Parser Types code IR2 IRn IRn .s Middle end Back end Front end syntax recognition,

278 views • 24 slides
Lecture 15 Economics UN3213 Intermediate Macroeconomics Professor Mart n Uribe Spring

Lecture 15 Economics UN3213 Intermediate Macroeconomics Professor Mart n Uribe Spring

Columbia University Department of Economics Lecture 15 Economics UN3213 Intermediate Macroeconomics Professor Mart n Uribe Spring 2019 Announcements: Welcome back! HWK 4 will be returned on wednesday. Recitations: Review of

355 views • 22 slides
Applied Algorithm Design: Exam Prof. Pietro Michiardi SPRING 2013 Rules and suggestions The

Applied Algorithm Design: Exam Prof. Pietro Michiardi SPRING 2013 Rules and suggestions The

Applied Algorithm Design: Exam Prof. Pietro Michiardi SPRING 2013 Rules and suggestions The exam is composed solely of questions related to the course contents The following evaluation scheme is used: A correct answer: +2 points

328 views • 3 slides
Custer Baker Intermediate School Welcome to Custer Baker Intermediate School Intermediate

Custer Baker Intermediate School Welcome to Custer Baker Intermediate School Intermediate

Custer Baker Intermediate School Welcome to Custer Baker Intermediate School Intermediate Characteristics Ages range from 10-14 years Unique developmental characteristics Emotional Social Intellectual Moral Physical

542 views • 17 slides
Review Final exam Final exam will be 11-12 problems, drop any 2 Cumulative up to and including

Review Final exam Final exam will be 11-12 problems, drop any 2 Cumulative up to and including

Review Final exam Final exam will be 11-12 problems, drop any 2 Cumulative up to and including week 13 (emphasis on weeks 10-13: classes & pointers) 2 hours exam time, so 12 min per problem (midterm 2 had 8-ish) Review: Overview

769 views • 39 slides
INF5110 Compiler Construction Spring 2017 1 / 97 Outline 1. Intermediate code generation

INF5110 Compiler Construction Spring 2017 1 / 97 Outline 1. Intermediate code generation

INF5110 Compiler Construction Spring 2017 1 / 97 Outline 1. Intermediate code generation Intro Intermediate code Three-address code P-code Generating P-code Generation of three address code Basic: From P-code to TA-Code and back:

1.18k views • 97 slides
INF5110 Compiler Construction Spring 2016 1 / 98 Outline 1. Intermediate code generation

INF5110 Compiler Construction Spring 2016 1 / 98 Outline 1. Intermediate code generation

INF5110 Compiler Construction Spring 2016 1 / 98 Outline 1. Intermediate code generation Intro Intermediate code Three-address code P-code Generating P-code Generation of three address code Basic: From P-code to TA-Code and back:

1.41k views • 98 slides
CS160 Midterm Exam Spring 2007, version 1 User Interface Design, Prototyping, and Evaluation Total

CS160 Midterm Exam Spring 2007, version 1 User Interface Design, Prototyping, and Evaluation Total

CS160 Midterm Exam Spring 2007, version 1 User Interface Design, Prototyping, and Evaluation Total number of points on exam: 100 Instructions This is a closed book, individual test. You are not allowed to use your notes, texts, or laptop

485 views • 6 slides

More recommend