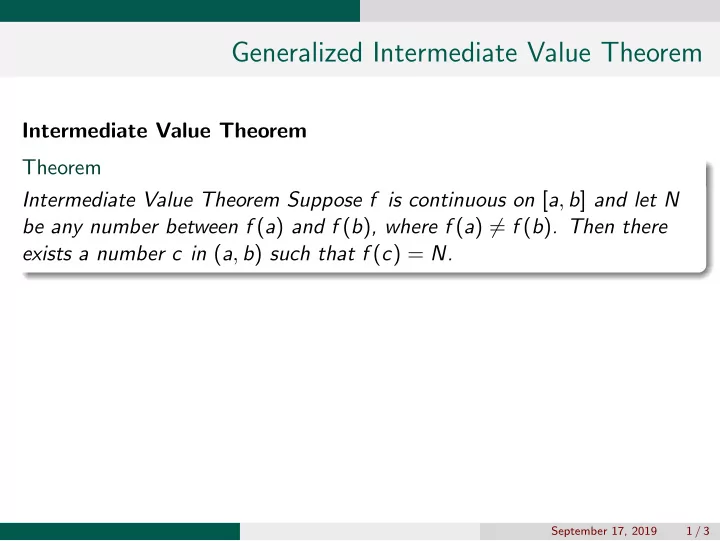
Generalized Intermediate Value Theorem Intermediate Value Theorem - PowerPoint PPT Presentation

Generalized Intermediate Value Theorem Intermediate Value Theorem Theorem Intermediate Value Theorem Suppose f is continuous on [ a , b ] and let N be any number between f ( a ) and f ( b ) , where f ( a ) � = f ( b ) . Then there exists a number c in ( a , b ) such that f ( c ) = N. September 17, 2019 1 / 3

Generalized Intermediate Value Theorem Intermediate Value Theorem Theorem Intermediate Value Theorem Suppose f is continuous on [ a , b ] and let N be any number between f ( a ) and f ( b ) , where f ( a ) � = f ( b ) . Then there exists a number c in ( a , b ) such that f ( c ) = N. Generalized Intermediate Value Theorem Theorem Let f be continuous on [ a , b ] . Let x 0 , x 1 , . . . , x n be points in [ a , b ] and a 1 , a 2 , . . . , a n > 0 . There exists a number c between a and b such that ( a 1 + · · · + a n ) f ( c ) = a 1 f ( x 1 ) + · · · + a n f ( x n ) September 17, 2019 1 / 3

Generalized IVT applied to error estimates Recall − h 2 f ′ ( x ) = f ( x + h ) − f ( x − h ) � � f ′′′ ( c 1 ) + f ′′′ ( c 2 ) 2 h 12 for c 1 ∈ ( x , x + h ) and c 2 ∈ ( x − h , x ). September 17, 2019 2 / 3

Generalized IVT applied to error estimates Theorem Let f be continuous on [ a , b ] . Let x 0 , x 1 , . . . , x n be points in [ a , b ] and a 1 , a 2 , . . . , a n > 0 . There exists a number c between a and b such that ( a 1 + · · · + a n ) f ( c ) = a 1 f ( x 1 ) + · · · + a n f ( x n ) We can combine the error terms of the central difference formula as 12) f ( c ) = h 2 ( 1 12 + 1 � � f ′′′ ( c 1 ) + f ′′′ ( c 2 ) 12 for c ∈ ( x − h , x + h ) to obtain a nicer looking estimate: − h 2 f ′ ( x ) = f ( x + h ) − f ( x − h ) 6 f ′′′ ( c ) c ∈ ( x − h , x + h ) 2 h September 17, 2019 3 / 3