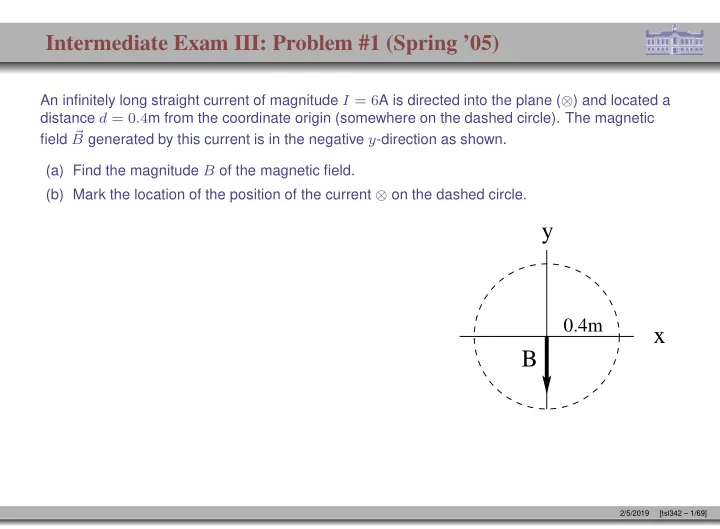

Intermediate Exam III: Problem #2 (Spring ’07) Consider two very long, straight wires with currents I 1 = 6 A at x = 1 m and I 3 = 3 A at x = 3 m in the directions shown. Find magnitude and direction (up/down) of the magnetic field (a) B 0 at x = 0 , I = 6A I = 3A 1 3 (b) B 2 at x = 2 m, B 0 B 2 B 4 (c) B 4 at x = 4 m. x [m] 0 1 2 3 4 Solution: (a) B 0 = − µ 0 (6A) 2 π (1m) + µ 0 (3A) 2 π (3m) = − 1 . 2 µ T + 0 . 2 µ T = − 1 . 0 µ T (down), (b) B 2 = µ 0 (6A) 2 π (1m) + µ 0 (3A) 2 π (1m) = 1 . 2 µ T + 0 . 6 µ T = 1 . 8 µ T (up), (c) B 4 = µ 0 (6A) 2 π (3m) − µ 0 (3A) 2 π (1m) = 0 . 4 µ T − 0 . 6 µ T = − 0 . 2 µ T (down). 2/5/2019 [tsl366 – 10/69]
Intermediate Exam III: Problem #3 (Spring ’07) A conducting frame with a moving conducting rod is located in a uniform magnetic field as shown. (a) Find the magnetic flux Φ B through the frame at the instant shown. (b) Find the induced emf E at the instant shown. (c) Find the direction (cw/ccw) of the induced current. v = 4m/s 2m B = 5T 2m 4m 2m 2/5/2019 [tsl367 – 11/69]
Intermediate Exam III: Problem #3 (Spring ’07) A conducting frame with a moving conducting rod is located in a uniform magnetic field as shown. (a) Find the magnetic flux Φ B through the frame at the instant shown. (b) Find the induced emf E at the instant shown. (c) Find the direction (cw/ccw) of the induced current. v = 4m/s 2m B = 5T 2m Solution: 4m 2m (a) Φ B = � A · � B = ± (20m 2 )(5T) = ± 100Wb . (b) E = − d Φ B = ± (5T)(2m)(4m / s) = ± 40V . dt (c) clockwise. 2/5/2019 [tsl367 – 11/69]
Unit Exam III: Problem #4 (Spring ’07) A proton ( m = 1 . 67 × 10 − 27 kg, q = 1 . 60 × 10 − 19 C) with velocity v = 3 . 7 × 10 4 m/s enters a region of magnetic field B directed perpendicular to the plane of the sheet. The field bends the path of the proton into a semicircle of radius r = 19 cm as shown. (a) Find the force necessary to keep the proton moving on the circle (b) Find the direction ( ⊙ or ⊗ ) and the magnitude of the magnetic field B that provides this force. (c) Find the time t it takes the proton to complete the semicircular motion. B r 2/5/2019 [tsl433 – 12/69]
Unit Exam III: Problem #4 (Spring ’07) A proton ( m = 1 . 67 × 10 − 27 kg, q = 1 . 60 × 10 − 19 C) with velocity v = 3 . 7 × 10 4 m/s enters a region of magnetic field B directed perpendicular to the plane of the sheet. The field bends the path of the proton into a semicircle of radius r = 19 cm as shown. (a) Find the force necessary to keep the proton moving on the circle (b) Find the direction ( ⊙ or ⊗ ) and the magnitude of the magnetic field B that provides this force. (c) Find the time t it takes the proton to complete the semicircular motion. B Solution: r (a) F = mv 2 = 1 . 20 × 10 − 17 N . r ⇒ B = F qv = 2 . 03 × 10 − 3 T . (b) F = qvB ⊗ ⇒ t = πr v = 1 . 61 × 10 − 5 s . (c) vt = πr 2/5/2019 [tsl433 – 12/69]
Unit Exam III: Problem #1 (Spring ’08) Consider two circular currents I 1 = 3 A at radius r 1 = 2 m and I 2 = 5 A at radius r 2 = 4 m in the directions shown. (a) Find magnitude B and direction ( ⊙ , ⊗ ) of the resultant magnetic field at the center. (b) Find magnitude µ and direction ( ⊙ , ⊗ ) of the magnetic dipole moment generated by the two currents. I 2 I 1 r 2 r 1 2/5/2019 [tsl381 – 13/69]
Unit Exam III: Problem #1 (Spring ’08) Consider two circular currents I 1 = 3 A at radius r 1 = 2 m and I 2 = 5 A at radius r 2 = 4 m in the directions shown. (a) Find magnitude B and direction ( ⊙ , ⊗ ) of the resultant magnetic field at the center. (b) Find magnitude µ and direction ( ⊙ , ⊗ ) of the magnetic dipole moment generated by the two currents. I 2 Solution: I (a) B = µ 0 (3A) 2(2m) − µ 0 (5A) 1 2(4m) = (9 . 42 − 7 . 85) × 10 − 7 T r ⇒ B = 1 . 57 × 10 − 7 T ⊗ 2 (b) µ = π (4m) 2 (5A) − π (2m) 2 (3A) = (251 − 38)Am 2 r 1 ⇒ µ = 213Am 2 ⊙ 2/5/2019 [tsl381 – 13/69]
Unit Exam III: Problem #2 (Spring ’08) (a) Consider a solid wire of radius R = 3 mm. Find magnitude I and direction (in/out) that produces a magnetic field B = 7 µ T at radius r = 8 mm. (b) Consider a hollow cable with inner radius R int = 3 mm and outer radius R ext = 5 mm. A current I out = 0 . 9 A is directed out of the plane. Find direction (up/down) and magnitude B 2 , B 6 of the magnetic field at radius r 2 = 2 mm and r 6 = 6 mm, respectively. (a) (b) I out I r 8mm 0mm 2mm 6mm B r 2/5/2019 [tsl382 – 14/69]
Unit Exam III: Problem #2 (Spring ’08) (a) Consider a solid wire of radius R = 3 mm. Find magnitude I and direction (in/out) that produces a magnetic field B = 7 µ T at radius r = 8 mm. (b) Consider a hollow cable with inner radius R int = 3 mm and outer radius R ext = 5 mm. A current I out = 0 . 9 A is directed out of the plane. Find direction (up/down) and magnitude B 2 , B 6 of the magnetic field at radius r 2 = 2 mm and r 6 = 6 mm, respectively. (a) (b) Solution: I out I µ 0 I (a) 7 µ T = ⇒ I = 0 . 28A (out). 2 π (8mm) r 8mm 0mm 2mm 6mm B 6 = µ 0 (0 . 9A) B (b) B 2 = 0 , 2 π (6mm) = 30 µ T (up). r 2/5/2019 [tsl382 – 14/69]
Unit Exam III: Problem #3 (Spring ’08) A circular wire of radius r = 2 . 5 m and resistance R = 4 . 8Ω is placed in the yz -plane as shown. A time-dependent magnetic field B = B x ˆ i is present. The dependence of B x on time is shown graphically. (a) Find the magnitude | Φ (1) B | and | Φ (3) B | of the magnetic flux through the cicle at times t = 1 s and t = 3 s, respectively. (b) Find magnitude I 1 , I 3 and direction (cw/ccw) of the induced current at times t = 1 s and t = 3 s, respectively. z B [T] x 3 2 r 1 y t [s] 0 0 2 4 x 2/5/2019 [tsl383 – 15/69]
Unit Exam III: Problem #3 (Spring ’08) A circular wire of radius r = 2 . 5 m and resistance R = 4 . 8Ω is placed in the yz -plane as shown. A time-dependent magnetic field B = B x ˆ i is present. The dependence of B x on time is shown graphically. (a) Find the magnitude | Φ (1) B | and | Φ (3) B | of the magnetic flux through the cicle at times t = 1 s and t = 3 s, respectively. (b) Find magnitude I 1 , I 3 and direction (cw/ccw) of the induced current at times t = 1 s and t = 3 s, respectively. z B [T] x Solution: 3 2 r (a) | Φ (1) B | = π (2 . 5m) 2 (2T) = 39 . 3 Wb , 1 y t [s] 0 | Φ (3) B | = π (2 . 5m) 2 (1T) = 19 . 6 Wb . 0 2 4 x d Φ (1) ˛ ˛ ˛ ˛ B (b) ˛ = 0 ⇒ I 1 = 0 , ˛ ˛ ˛ dt ˛ ˛ d Φ (3) ˛ ˛ ⇒ I 3 = 19 . 6V ˛ ˛ B ˛ = | π (2 . 5m) 2 ( − 1T / s) | = 19 . 6V 4 . 8Ω = 4 . 1A (ccw). ˛ ˛ ˛ dt ˛ ˛ 2/5/2019 [tsl383 – 15/69]
Unit Exam III: Problem #3 (Spring ’08) A proton ( m = 1 . 67 × 10 − 27 kg, q = 1 . 60 × 10 − 19 C) with velocity v = 3 . 7 × 10 4 m/s moves on a circle of radius r = 0 . 49 m in a counterclockwise direction. (a) Find the centripetal force F needed to keep the proton on the circle. (b) Find direction ( ⊙ or ⊗ ) and magnitude of the field B that provides the centripetal force F . (c) Find the electric current I produced by the rotating proton. v r 2/5/2019 [tsl434 – 16/69]
Unit Exam III: Problem #3 (Spring ’08) A proton ( m = 1 . 67 × 10 − 27 kg, q = 1 . 60 × 10 − 19 C) with velocity v = 3 . 7 × 10 4 m/s moves on a circle of radius r = 0 . 49 m in a counterclockwise direction. (a) Find the centripetal force F needed to keep the proton on the circle. (b) Find direction ( ⊙ or ⊗ ) and magnitude of the field B that provides the centripetal force F . (c) Find the electric current I produced by the rotating proton. v r Solution: (a) F = mv 2 = (1 . 67 × 10 − 27 kg)(3 . 7 × 10 4 m / s) 2 = 4 . 67 × 10 − 18 N . r 0 . 49m 4 . 67 × 10 − 18 N ⇒ B = F (b) F = qvB qv = (1 . 60 × 10 − 19 C)(3 . 7 × 10 4 m / s) = 0 . 788mT ⊗ (in). (c) I = q τ = 2 πr ⇒ I = qv 2 πr = 1 . 92 × 10 − 15 A . τ , v 2/5/2019 [tsl434 – 16/69]
Unit Exam III: Problem #1 (Spring ’09) A triangular conducting loop in the yz -plane with a counterclockwise current I = 3 A is free to rotate about the axis PQ . A uniform magnetic field � B = 0 . 5Tˆ k is present. (a) Find the magnetic moment � µ (magnitude and direction) of the triangle. (b) Find the magnetic torque � τ (magnitude and direction) acting on the triangle. (c) Find the magnetic force � F H (magnitude and direction) acting on the long side (hypotenuse) of the triangle. (d) Find the force � F R (magnitude and direction) that must be applied to the corner R to keep the triangle from rotating. z 8m P Q I 8m B R y x 2/5/2019 [tsl395 – 17/69]
Unit Exam III: Problem #1 (Spring ’09) A triangular conducting loop in the yz -plane with a counterclockwise current I = 3 A is free to rotate about the axis PQ . A uniform magnetic field � B = 0 . 5Tˆ k is present. (a) Find the magnetic moment � µ (magnitude and direction) of the triangle. (b) Find the magnetic torque � τ (magnitude and direction) acting on the triangle. (c) Find the magnetic force � F H (magnitude and direction) acting on the long side (hypotenuse) of the triangle. (d) Find the force � F R (magnitude and direction) that must be applied to the corner R to keep the triangle from rotating. z 8m P Q I 8m B Solution: R y µ = (3A)(32m 2 )ˆ i = 96Am 2 ˆ (a) � i. x µ × � i ) × (0 . 5Tˆ B = (96Am 2 ˆ k ) = − 48Nmˆ (b) � τ = � j . √ 2m)(0 . 5T)(sin 45 ◦ ) = 12N (c) F H = (3A)(8 ⊙ . (d) ( − 8mˆ k ) × � τ = 48Nmˆ ⇒ � F R = − 6Nˆ F R = − � j i . 2/5/2019 [tsl395 – 17/69]
Unit Exam III: Problem #2 (Spring ’09) Two semi-infinite straight wires are connected to a curved wire in the form of a full circle, quarter circle, or half circle of radius R = 1 m in four different configurations. A current I = 1 A flows in the directions shown. Find magnitude B a , B b , B c , B d and direction ( ⊙ / ⊗ ) of the magnetic field thus generated at the points a, b, c, d . I I c a I I b d 2/5/2019 [tsl396 – 18/69]
Unit Exam III: Problem #2 (Spring ’09) Two semi-infinite straight wires are connected to a curved wire in the form of a full circle, quarter circle, or half circle of radius R = 1 m in four different configurations. A current I = 1 A flows in the directions shown. Find magnitude B a , B b , B c , B d and direction ( ⊙ / ⊗ ) of the magnetic field thus generated at the points a, b, c, d . I I c a I I Solution: b d ˛ ˛ 4 πR + µ 0 I µ 0 I 2 R + µ 0 I ˛ ˛ B a = ˛ = | 100nT + 628nT + 100nT | = 828nT ⊗ ˛ ˛ 4 πR ˛ ˛ 4 πR + µ 0 I µ 0 I 4 R − µ 0 I ˛ ˛ ˛ B b = ˛ = | 100nT + 314nT − 100nT | = 314nT ⊗ ˛ ˛ 4 πR ˛ ˛ ˛ 4 πR + µ 0 I µ 0 I ˛ ˛ B c = 8 R + 0 ˛ = | 100nT + 157nT | = 257nT ⊗ ˛ ˛ ˛ ˛ ˛ 4 πR − µ 0 I µ 0 I 2 R + µ 0 I ˛ ˛ B d = ˛ = | 100nT − 628nT + 100nT | = 428nT ⊙ ˛ ˛ 4 πR ˛ 2/5/2019 [tsl396 – 18/69]
Unit Exam III: Problem #3 (Spring ’09) A pair of rails are connected by two mobile rods. A uniform magnetic field B directed into the plane is present. In the situations (a), (b), (c), (d), one or both rods move at constant velocity as shown. The resistance of the conducting loop is R = 0 . 2Ω in each case. Find magnitude I and direction (cw/ccw) of the induced current in each case. (a) (c) 4m 4m v = 3m/s v = 5m/s v = 3m/s B = 0.7T B = 0.7T (b) (d) 4m 4m v = 5m/s v = 5m/s v = 3m/s B = 0.7T B = 0.7T 2/5/2019 [tsl397 – 19/69]
Unit Exam III: Problem #3 (Spring ’09) A pair of rails are connected by two mobile rods. A uniform magnetic field B directed into the plane is present. In the situations (a), (b), (c), (d), one or both rods move at constant velocity as shown. The resistance of the conducting loop is R = 0 . 2Ω in each case. Find magnitude I and direction (cw/ccw) of the induced current in each case. (a) (c) 4m 4m v = 3m/s v = 5m/s v = 3m/s B = 0.7T B = 0.7T (b) (d) 4m 4m v = 5m/s v = 5m/s v = 3m/s Solution: B = 0.7T B = 0.7T I = 8 . 4V (a) |E| = (3m / s)(0 . 7T)(4m) = 8 . 4V , 0 . 2Ω = 42A ccw I = 14V (b) |E| = (5m / s)(0 . 7T)(4m) = 14V , 0 . 2Ω = 70A cw I = 5 . 6V (c) |E| = (5m / s − 3m / s)(0 . 7T)(4m) = 5 . 6V , 0 . 2Ω = 28A cw I = 22 . 4V (d) |E| = (5m / s + 3m / s)(0 . 7T)(4m) = 22 . 4V , 0 . 2Ω = 112A ccw 2/5/2019 [tsl397 – 19/69]
Unit Exam III: Problem #1 (Spring ’11) (a) Two very long straight wires carry currents as shown. A cube with edges of length 8cm serves as scaffold. Find the magnetic field at point P in the form B = B x ˆ i + B y ˆ j + B z ˆ k with B x , B y , B z in SI units. (b) Two circular currents of radius 5cm, one in the xy -lane and the other in the yz -plane, carry currents as shown. Both circles are centered at point O . Find the magnetic field at point O in the form B = B x ˆ i + B y ˆ j + B z ˆ k with B x , B y , B z in SI units. z z 3A P O y y 3A x 2A x 2A 2/5/2019 [tsl415 – 20/69]
Unit Exam III: Problem #1 (Spring ’11) (a) Two very long straight wires carry currents as shown. A cube with edges of length 8cm serves as scaffold. Find the magnetic field at point P in the form B = B x ˆ i + B y ˆ j + B z ˆ k with B x , B y , B z in SI units. (b) Two circular currents of radius 5cm, one in the xy -lane and the other in the yz -plane, carry currents as shown. Both circles are centered at point O . Find the magnetic field at point O in the form B = B x ˆ i + B y ˆ j + B z ˆ k with B x , B y , B z in SI units. z z 3A P O y y 3A x 2A x Solution: 2A µ 0 (2A) µ 0 (3A) (a) B x = 0 , B y = 2 π (0 . 08m) = 5 µ T , B z = 2 π (0 . 08m) = 7 . 5 µ T . µ 0 (2A) B z = − µ 0 (3A) (b) B x = 2(0 . 05m) = 25 . 1 µ T , B y = 0 , 2(0 . 05m) = − 37 . 7 µ T . 2/5/2019 [tsl415 – 20/69]
Unit Exam III: Problem #2 (Spring ’11) The coaxial cable shown has surfaces at radii 1mm, 3mm, and 5mm. The magnetic field is the same at radii 2mm and 6mm, namely B = 7 µ T in the direction shown. (a) Find magnitude (in SI units) and direction (in/out) of the current I int flowing through the inner conductor. (b) Find magnitude (in SI units) and direction (in/out) of the current I ext flowing through the outer conductor. I ext B B r I int 2mm 6mm 2/5/2019 [tsl416 – 21/69]
Unit Exam III: Problem #2 (Spring ’11) The coaxial cable shown has surfaces at radii 1mm, 3mm, and 5mm. The magnetic field is the same at radii 2mm and 6mm, namely B = 7 µ T in the direction shown. (a) Find magnitude (in SI units) and direction (in/out) of the current I int flowing through the inner conductor. (b) Find magnitude (in SI units) and direction (in/out) of the current I ext flowing through the outer conductor. I ext B B r I int 2mm 6mm Solution: (a) (7 µ T)(2 π )(0 . 002m) = µ 0 I int ⇒ I int = 0 . 07A (out) (b) (7 µ T)(2 π )(0 . 006m) = µ 0 ( I int + I ext ) ⇒ I int + I ext = 0 . 21A (out) ⇒ I ext = 0 . 14A (out) 2/5/2019 [tsl416 – 21/69]
Unit Exam III: Problem #3 (Spring ’11) A conducting frame with a moving conducting rod is located in a uniform magnetic field as shown. The rod moves at constant velocity. (a) Find the magnetic flux Φ B through the frame and the induced emf E around the frame at the instant shown. (b) Find the magnetic flux Φ B through the frame and the induced emf E around the frame two seconds later. Write magnitudes only (in SI units), no directions. v = 2m/s 2m B = 3T 2m 4m 2m 2/5/2019 [tsl417 – 22/69]
Unit Exam III: Problem #3 (Spring ’11) A conducting frame with a moving conducting rod is located in a uniform magnetic field as shown. The rod moves at constant velocity. (a) Find the magnetic flux Φ B through the frame and the induced emf E around the frame at the instant shown. (b) Find the magnetic flux Φ B through the frame and the induced emf E around the frame two seconds later. Write magnitudes only (in SI units), no directions. v = 2m/s 2m B = 3T 2m Solution: 4m 2m (a) Φ B = (20m 2 )(3T) = 60Wb , E = (2m / s)(3T)(2m) = 12V . (b) Φ B = (8m 2 )(3T) = 24Wb , E = (2m / s)(3T)(4m) = 24V . 2/5/2019 [tsl417 – 22/69]
Unit Exam III: Problem #1 (Spring ’12) In a region of uniform magnetic field B = 5mTˆ i , a proton ( m = 1 . 67 × 10 − 27 kg , q = 1 . 60 × 10 − 19 C) is launched with velocity v 0 = 4000m / sˆ k . (a) Calculate the magnitude F of the magnetic force that keeps the proton on a circular path. (b) Calculate the radius r of the circular path. (c) Calculate the time T it takes the proton to go around that circle once. (d) Sketch the circular path of the proton in the graph. z v 0 m, q y B x 2/5/2019 [tsl435 – 23/69]
Unit Exam III: Problem #1 (Spring ’12) In a region of uniform magnetic field B = 5mTˆ i , a proton ( m = 1 . 67 × 10 − 27 kg , q = 1 . 60 × 10 − 19 C) is launched with velocity v 0 = 4000m / sˆ k . (a) Calculate the magnitude F of the magnetic force that keeps the proton on a circular path. (b) Calculate the radius r of the circular path. (c) Calculate the time T it takes the proton to go around that circle once. (d) Sketch the circular path of the proton in the graph. z v 0 Solution: m, q (a) F = qv 0 B = 3 . 2 × 10 − 18 N . y (b) mv 2 ⇒ r = mv 0 0 = qv 0 B = 8 . 35mm . r qB B x (c) T = 2 πr = 2 πm = 13 . 1 µ s . v 0 qB (d) Center of circle to the right of proton’s initial position (cw motion). 2/5/2019 [tsl435 – 23/69]
Unit Exam III: Problem #2 (Spring ’12) (a) Two very long straight wires positioned in the xy -plane carry electric currents I 1 , I 2 as shown. Calculate magnitude ( B 1 , B 2 ) and direction ( ⊙ , ⊗ ) of the magnetic field produced by each current at the origin of the coordinate system. (b) A conducting loop of radius r = 3 cm placed in the xy -plane carries a current I 3 = 0 . 7 A in the direction shown. Find direction and magnitude of the torque � τ acting on the loop if it is placed in a magnetic field B = 5mTˆ i . y (a) y (b) I = 0.7A 3 2cm I = 5A 2 x x 2cm 2cm I = 3A 1 B = 5mT 2/5/2019 [tsl436 – 24/69]
Unit Exam III: Problem #2 (Spring ’12) (a) Two very long straight wires positioned in the xy -plane carry electric currents I 1 , I 2 as shown. Calculate magnitude ( B 1 , B 2 ) and direction ( ⊙ , ⊗ ) of the magnetic field produced by each current at the origin of the coordinate system. (b) A conducting loop of radius r = 3 cm placed in the xy -plane carries a current I 3 = 0 . 7 A in the direction shown. Find direction and magnitude of the torque � τ acting on the loop if it is placed in a magnetic field B = 5mTˆ i . y (a) y (b) I = 0.7A 3 2cm I = 5A 2 x x 2cm 2cm I = 3A Solution: 1 B = 5mT (a) B 1 = µ 0 (3A) µ 0 (5A) 2 π (2cm) = 30 µ T . ⊙ B 2 = 2 π (1 . 41cm) = 70 . 9 µ T . ⊙ µ = π (3cm) 2 (0 . 7A)ˆ k = 1 . 98 × 10 − 3 Am 2 ˆ µ × B = 9 . 90 × 10 − 6 Nmˆ (b) � ⇒ � τ = � j . k 2/5/2019 [tsl436 – 24/69]
Unit Exam III: Problem #3 (Spring ’12) The coaxial cable shown in cross section has surfaces at radii 1mm, 3mm, and 5mm. Equal currents flow through both conductors: I int = I ext = 0 . 03 A ⊙ (out). Find direction ( ↑ , ↓ ) and magnitude ( B 1 , B 3 , B 5 , B 7 ) of the magnetic field at the four radii indicated ( • ) . I ext 7mm 3mm r I int 5mm 1mm 2/5/2019 [tsl437 – 25/69]
Unit Exam III: Problem #3 (Spring ’12) The coaxial cable shown in cross section has surfaces at radii 1mm, 3mm, and 5mm. Equal currents flow through both conductors: I int = I ext = 0 . 03 A ⊙ (out). Find direction ( ↑ , ↓ ) and magnitude ( B 1 , B 3 , B 5 , B 7 ) of the magnetic field at the four radii indicated ( • ) . I ext 7mm 3mm r I int 5mm 1mm Solution: 2 π (1mm) B 1 = µ 0 (0 . 03A) ⇒ B 1 = 6 µ T ↑ 2 π (3mm) B 1 = µ 0 (0 . 03A) ⇒ B 1 = 2 µ T ↑ 2 π (5mm) B 1 = µ 0 (0 . 06A) ⇒ B 1 = 2 . 4 µ T ↑ 2 π (7mm) B 1 = µ 0 (0 . 06A) ⇒ B 1 = 1 . 71 µ T ↑ 2/5/2019 [tsl437 – 25/69]
Unit Exam IV: Problem #1 (Spring ’12) In the circuit shown we close the switch S at time t = 0 . Find the current I L through the inductor and the voltage V 6 across the 6Ω -resistor (a) immediately after the switch has been closed, (b) a very long time later. 7H Ω 6 Ω Ω 3 1 12V S 2/5/2019 [tsl441 – 26/69]
Unit Exam IV: Problem #1 (Spring ’12) In the circuit shown we close the switch S at time t = 0 . Find the current I L through the inductor and the voltage V 6 across the 6Ω -resistor (a) immediately after the switch has been closed, (b) a very long time later. 7H Ω 6 Solution: Ω Ω 3 1 I 6 = 12V 12V (a) I L = 0 , 10Ω = 1 . 2A , V 6 = (6Ω)(1 . 2A) = 7 . 2V . (b) I L = 12V S 4Ω = 3A , V 6 = 0 . 2/5/2019 [tsl441 – 26/69]
Unit Exam IV: Problem #2 (Spring ’12) At time t = 0 the capacitor is charged to Q max = 4 µ C and the switch is being closed. The charge on the capacitor begins to decrease and the current through the inductor begins to increase. (a) At what time t 1 is the capacitor discharged for the first time? (b) At what time t 2 has the current through the inductor returned to zero for the first time? (c) What is the maximum energy stored in the capacitor at any time? (d) What is the maximum energy stored in the inductor at any time? L = 30mH C = 5 µ F S 2/5/2019 [tsl442 – 27/69]
Unit Exam IV: Problem #2 (Spring ’12) At time t = 0 the capacitor is charged to Q max = 4 µ C and the switch is being closed. The charge on the capacitor begins to decrease and the current through the inductor begins to increase. (a) At what time t 1 is the capacitor discharged for the first time? (b) At what time t 2 has the current through the inductor returned to zero for the first time? (c) What is the maximum energy stored in the capacitor at any time? (d) What is the maximum energy stored in the inductor at any time? L = 30mH Solution: C = 5 µ F S √ (a) T = 2 π t 1 = T ω = 2 π LC = 2 . 43ms , 4 = 0 . 608ms . (b) t 2 = T 2 = 1 . 22ms . = Q 2 max (c) U max = 1 . 6 µ J . C 2 C (d) U max = U max = 1 . 6 µ J (energy conservation.) L C 2/5/2019 [tsl442 – 27/69]
Unit Exam IV: Problem #3 (Spring ’12) The ac voltage supplied in the circuit shown is E = E max cos( ωt ) with E max = 170 V and ω = 377 rad/s. (a) What is the maximum value I max of the current? (b) What is the emf E ( t ) at t = 5 ms? (c) What is the current I ( t ) at t = 5 ms? (d) What is the power transfer P ( t ) between ac source and device at t = 5 ms? ~ L = 40mH 2/5/2019 [tsl443 – 28/69]
Unit Exam IV: Problem #3 (Spring ’12) The ac voltage supplied in the circuit shown is E = E max cos( ωt ) with E max = 170 V and ω = 377 rad/s. (a) What is the maximum value I max of the current? (b) What is the emf E ( t ) at t = 5 ms? (c) What is the current I ( t ) at t = 5 ms? (d) What is the power transfer P ( t ) between ac source and device at t = 5 ms? ~ L = 40mH Solution: (a) I max = E max 170V = (377rad / s)(40mH) = 11 . 3A . ωL (b) E = (170V) cos(1 . 885rad) = (170V)( − 0 . 309) = − 52 . 5V . (c) I = (11 . 3A) cos(1 . 885rad − π/ 2) = (11 . 3A) cos(0 . 314) = (11 . 3A)(0 . 951) = 10 . 7A . (d) P = E I = ( − 52 . 5V)(10 . 7A) = − 562W . 2/5/2019 [tsl443 – 28/69]
Unit Exam III: Problem #1 (Spring ’13) In a region of uniform magnetic field B a proton ( m = 1 . 67 × 10 − 27 kg , q = 1 . 60 × 10 − 19 C) experiences a force F = 9 . 0 × 10 − 19 Nˆ i as it passes through point P with velocity v 0 = 3000m / sˆ j on a circular path. (a) Find the magnetic field B (magnitude and direction). (b) Calculate the radius r of the circular path. (c) Locate the center C of the circular path in the coordinate system on the page. z [cm] 5 4 3 2 1 4 5 1 2 3 y [cm] 1 2 3 P 4 5 x [cm] 2/5/2019 [tsl461 – 29/69]
Unit Exam III: Problem #1 (Spring ’13) In a region of uniform magnetic field B a proton ( m = 1 . 67 × 10 − 27 kg , q = 1 . 60 × 10 − 19 C) experiences a force F = 9 . 0 × 10 − 19 Nˆ i as it passes through point P with velocity v 0 = 3000m / sˆ j on a circular path. (a) Find the magnetic field B (magnitude and direction). (b) Calculate the radius r of the circular path. (c) Locate the center C of the circular path in the coordinate system on the page. z [cm] Solution: 5 4 F ˆ i = ˆ j × ˆ = 1 . 88 × 10 − 3 T , (a) B = k 3 qv 0 2 ⇒ B = 1 . 88 × 10 − 3 T ˆ k . 1 4 5 (b) F = mv 2 1 2 3 0 y [cm] = qv 0 B 1 r 2 ⇒ r = mv 2 = mv 0 3 0 = 1 . 67cm . P 4 F qB 5 (c) C = 4 . 67cmˆ i + 3 . 00cmˆ x [cm] j . 2/5/2019 [tsl461 – 29/69]
Unit Exam III: Problem #1 (Spring ’13) In a region of uniform magnetic field B a proton ( m = 1 . 67 × 10 − 27 kg , q = 1 . 60 × 10 − 19 C) experiences a force F = 8 . 0 × 10 − 19 Nˆ i as it passes through point P with velocity v 0 = 2000m / s ˆ k on a circular path. (a) Find the magnetic field B (magnitude and direction). (b) Calculate the radius r of the circular path. (c) Locate the center C of the circular path in the coordinate system on the page. z [cm] 5 4 3 2 P 1 4 5 1 2 3 y [cm] 1 2 3 4 5 x [cm] 2/5/2019 [tsl462 – 30/69]
Unit Exam III: Problem #1 (Spring ’13) In a region of uniform magnetic field B a proton ( m = 1 . 67 × 10 − 27 kg , q = 1 . 60 × 10 − 19 C) experiences a force F = 8 . 0 × 10 − 19 Nˆ i as it passes through point P with velocity v 0 = 2000m / s ˆ k on a circular path. (a) Find the magnetic field B (magnitude and direction). (b) Calculate the radius r of the circular path. (c) Locate the center C of the circular path in the coordinate system on the page. z [cm] Solution: 5 4 F ˆ i = ˆ k × ( − ˆ = 2 . 50 × 10 − 3 T , (a) B = j ) 3 qv 0 2 ⇒ B = − 2 . 50 × 10 − 3 Tˆ j . P 1 4 5 1 2 3 (b) F = mv 2 0 y [cm] = qv 0 B 1 r 2 ⇒ r = mv 2 = mv 0 3 0 = 0 . 835cm . 4 F qB 5 (c) C = 3 . 84cmˆ i + 3 . 00cm ˆ x [cm] k . 2/5/2019 [tsl462 – 30/69]
Unit Exam III: Problem #2 (Spring ’13) A very long, straight wire is positioned along the x -axis and a circular wire of 1 . 5 cm radius in the yz plane with its center P on the z -axis as shown. Both wires carry a current I = 0 . 6 A in the directions shown. (a) Find the magnetic field B c (magnitude and direction) generated at point P by the current in the circular wire. (b) Find the magnetic field B s (magnitude and direction) generated at point P by the current in the straight wire. (c) Find the magnetic moment � µ (magnitude and direction) of the circular current. z [cm] 5 I 4 P 3 2 1 4 5 1 2 3 y [cm] I 1 2 3 4 5 x [cm] 2/5/2019 [tsl463 – 31/69]
Unit Exam III: Problem #2 (Spring ’13) A very long, straight wire is positioned along the x -axis and a circular wire of 1 . 5 cm radius in the yz plane with its center P on the z -axis as shown. Both wires carry a current I = 0 . 6 A in the directions shown. (a) Find the magnetic field B c (magnitude and direction) generated at point P by the current in the circular wire. (b) Find the magnetic field B s (magnitude and direction) generated at point P by the current in the straight wire. (c) Find the magnetic moment � µ (magnitude and direction) of the circular current. z [cm] Solution: 5 I 4 (a) B c = µ 0 (0 . 6A) 2(0 . 015m) ( − ˆ i ) = − 2 . 51 × 10 − 5 Tˆ i . P 3 2 (b) B s = µ 0 (0 . 6A) 2 π (0 . 03m) ( − ˆ j ) = − 4 . 00 × 10 − 6 Tˆ j . 1 4 5 1 2 3 y [cm] µ = π (0 . 015mm) 2 (0 . 6A)( − ˆ i ) = − 4 . 24 × 10 − 4 Am 2 ˆ (c) � i . I 1 2 3 4 5 x [cm] 2/5/2019 [tsl463 – 31/69]
Unit Exam III: Problem #2 (Spring ’13) A very long straight wire is positioned along the x -axis and a circular wire of 2 . 0 cm radius in the yz plane with its center P on the y -axis as shown. Both wires carry a current I = 0 . 5 A in the directions shown. (a) Find the magnetic field B c (magnitude and direction) generated at point P by the current in the circular wire. (b) Find the magnetic field B s (magnitude and direction) generated at point P by the current in the straight wire. (c) Find the magnetic moment � µ (magnitude and direction) of the circular current. z [cm] 5 4 3 2 1 4 1 2 3 5 6 y [cm] I 1 P 2 I 3 4 5 x [cm] 2/5/2019 [tsl464 – 32/69]
Unit Exam III: Problem #2 (Spring ’13) A very long straight wire is positioned along the x -axis and a circular wire of 2 . 0 cm radius in the yz plane with its center P on the y -axis as shown. Both wires carry a current I = 0 . 5 A in the directions shown. (a) Find the magnetic field B c (magnitude and direction) generated at point P by the current in the circular wire. (b) Find the magnetic field B s (magnitude and direction) generated at point P by the current in the straight wire. (c) Find the magnetic moment � µ (magnitude and direction) of the circular current. z [cm] Solution: 5 4 (a) B c = µ 0 (0 . 5A) ˆ i = 1 . 57 × 10 − 5 Tˆ 3 i . 2(0 . 02m) 2 µ 0 (0 . 5A) 1 2 π (0 . 035m) ( − ˆ k ) = − 2 . 86 × 10 − 6 T ˆ (b) B s = k . 4 1 2 3 5 6 y [cm] I 1 P µ = π (0 . 02m) 2 (0 . 5A)ˆ i = 6 . 28 × 10 − 4 Am 2 ˆ (c) � i . 2 I 3 4 5 x [cm] 2/5/2019 [tsl464 – 32/69]
Unit Exam III: Problem #3 (Spring ’13) Consider a wire with a resistance per unit length of 1 Ω /cm bent into a rectangular loop and placed into the yz -plane as shown. The magnetic field in the entire region is uniform and increases from zero as follows: B = (2ˆ i + 1ˆ j + 3ˆ k ) t T/s, where t is the time in seconds. (a) Find the magnetic flux Φ B through the rectangle at time t = 2 s. (b) Find magnitude and direction (cw/ccw) of the induced EMF E around the rectangle at time t = 2 s. (c) Infer the induced current I from the induced EMF . z [cm] 5 4 3 2 1 4 1 2 3 5 6 y [cm] 1 2 3 4 5 x [cm] 2/5/2019 [tsl465 – 33/69]
Unit Exam III: Problem #3 (Spring ’13) Consider a wire with a resistance per unit length of 1 Ω /cm bent into a rectangular loop and placed into the yz -plane as shown. The magnetic field in the entire region is uniform and increases from zero as follows: B = (2ˆ i + 1ˆ j + 3ˆ k ) t T/s, where t is the time in seconds. (a) Find the magnetic flux Φ B through the rectangle at time t = 2 s. (b) Find magnitude and direction (cw/ccw) of the induced EMF E around the rectangle at time t = 2 s. (c) Infer the induced current I from the induced EMF . z [cm] Solution: 5 (a) Φ B = ± (4cm)(3cm)(2T / s)(2s) = ± 4 . 8 × 10 − 3 Wb 4 (b) E = ∓ (4cm)(3cm)(2T / s) = ∓ 2 . 4mV (cw) 3 2 . 4mV 2 (c) I = (1Ω / cm)(14cm) = 0 . 171mA 1 4 1 2 3 5 6 y [cm] 1 2 3 4 5 x [cm] 2/5/2019 [tsl465 – 33/69]
Unit Exam III: Problem #3 (Spring ’13) Consider a wire with a resistance per unit length of 1 Ω /cm bent into a rectangular loop and placed into the yz -plane as shown. The magnetic field in the entire region is uniform and increases from zero as follows: B = (3ˆ i + 1ˆ j + 2ˆ k ) t T/s, where t is the time in seconds. (a) Find the magnetic flux Φ B through the rectangle at time t = 2 s. (b) Find magnitude and direction (cw/ccw) of the induced EMF E around the rectangle at time t = 2 s. (c) Infer the induced current I from the induced EMF . z [cm] 5 4 3 2 1 4 1 2 3 5 6 y [cm] 1 2 3 4 5 x [cm] 2/5/2019 [tsl466 – 34/69]
Unit Exam III: Problem #3 (Spring ’13) Consider a wire with a resistance per unit length of 1 Ω /cm bent into a rectangular loop and placed into the yz -plane as shown. The magnetic field in the entire region is uniform and increases from zero as follows: B = (3ˆ i + 1ˆ j + 2ˆ k ) t T/s, where t is the time in seconds. (a) Find the magnetic flux Φ B through the rectangle at time t = 2 s. (b) Find magnitude and direction (cw/ccw) of the induced EMF E around the rectangle at time t = 2 s. (c) Infer the induced current I from the induced EMF . z [cm] Solution: 5 (a) Φ B = ± (5cm)(3cm)(3T / s)(2s) = ± 9 . 0 × 10 − 3 Wb 4 (b) E = ∓ (5cm)(3cm)(3T / s) = ∓ 4 . 5mV (cw) 3 4 . 5mV 2 (c) I = (1Ω / cm)(16cm) = 0 . 281mA 1 4 1 2 3 5 6 y [cm] 1 2 3 4 5 x [cm] 2/5/2019 [tsl466 – 34/69]
Unit Exam III: Problem #1 (Spring ’14) A counterclockwise current I = 1 . 7 A [ I = 1 . 3 A] is flowing through the conducting rectangular frame shown in a region of magnetic field B = 6 mT ˆ j [ B = 6 mT ˆ k ]. (a) Find the force F bc [ F ab ] (magnitude and direction) acting on side bc [ ab ] of the rectangle. (b) Find the magnetic moment � µ (magnitude and direction) of the current loop. (c) Find the torque � τ (magnitude and direction) acting on the current loop. z [cm] d c 5 4 3 2 a b 1 4 1 2 3 5 y [cm] 1 2 3 4 5 x [cm] 2/5/2019 [tsl476 – 35/69]
Unit Exam III: Problem #1 (Spring ’14) A counterclockwise current I = 1 . 7 A [ I = 1 . 3 A] is flowing through the conducting rectangular frame shown in a region of magnetic field B = 6 mT ˆ j [ B = 6 mT ˆ k ]. (a) Find the force F bc [ F ab ] (magnitude and direction) acting on side bc [ ab ] of the rectangle. (b) Find the magnetic moment � µ (magnitude and direction) of the current loop. (c) Find the torque � τ (magnitude and direction) acting on the current loop. z [cm] d c 5 4 3 2 a b 1 Solution: 4 1 2 3 5 y [cm] 1 (a) F bc = (1 . 7A)(3cm ˆ k ) × (6mT ˆ j ) = − 3 . 06 × 10 − 4 N ˆ i 2 3 [ F ab = (1 . 3A)(2cm ˆ j ) × (6mT ˆ k ) = 1 . 56 × 10 − 4 N ˆ i ] 4 5 i ](1 . 7A) = 1 . 02 × 10 − 3 Am 2 ˆ µ = [(2cm)(3cm) ˆ (b) � i x [cm] i ](1 . 3A) = 7 . 8 × 10 − 4 Am 2 ˆ µ = [(2cm)(3cm) ˆ [ � i ] τ = (1 . 02 × 10 − 3 Am 2 ˆ i ) × (6mT ˆ j ) = 6 . 12 × 10 − 6 Nm ˆ (c) � k τ = (7 . 8 × 10 − 4 Am 2 ˆ i ) × (6mT ˆ k ) = − 4 . 68 × 10 − 6 Nm ˆ [ � j ] 2/5/2019 [tsl476 – 35/69]
Unit Exam III: Problem #2 (Spring ’14) (a) Find the magnetic field B a (magnitude and direction) generated by the three long, straight currents I 1 = I 2 = I 3 = 1 . 8 mA [ 2 . 7 mA]] in the directions shown. (b) Find the magnetic field B b (magnitude and direction) generated by the two circular currents I 5 = I 6 = 1 . 5 mA [ 2 . 5 mA] in the directions shown. I (a) (b) 5 I 2 I 6 8cm B 9cm b I 1 I 4cm 3 B 9cm 9cm a 2/5/2019 [tsl477 – 36/69]
Unit Exam III: Problem #2 (Spring ’14) (a) Find the magnetic field B a (magnitude and direction) generated by the three long, straight currents I 1 = I 2 = I 3 = 1 . 8 mA [ 2 . 7 mA]] in the directions shown. (b) Find the magnetic field B b (magnitude and direction) generated by the two circular currents I 5 = I 6 = 1 . 5 mA [ 2 . 5 mA] in the directions shown. I (a) (b) 5 I 2 I 6 8cm B 9cm b I 1 I 4cm 3 B Solution: 9cm 9cm a (a) B a = µ 0 (1 . 8mA) = 4 × 10 − 9 T (directed ← ) 2 π (9cm) [ B a = µ 0 (2 . 7mA) = 6 × 10 − 9 T (directed ← )] 2 π (9cm) (b) B b = µ 0 (1 . 5mA) − µ 0 (1 . 5mA) = 1 . 18 × 10 − 8 T (directed ⊗ ) 2(4cm) 2(8cm) [ B b = µ 0 (2 . 5mA) − µ 0 (2 . 5mA) = 1 . 96 × 10 − 8 T (directed ⊗ )] 2(4cm) 2(8cm) 2/5/2019 [tsl477 – 36/69]
Unit Exam III: Problem #3 (Spring ’14) Consider a region of uniform magnetic field B = (3 ˆ i + 2 ˆ j + 1 ˆ k ) mT [ B = (2 ˆ i + 3 ˆ j + 1 ˆ k ) mT]. A conducting rod slides along conducting rails in the yz -plane as shown. The rails are connected on the right. The clock is set to t = 0 at the instant shown. (a) Find the magnetic flux Φ B through the conducting loop at t = 0 . (b) Find the magnetic flux Φ B through the conducting loop at t = 1 s. (c) Find the induced EMF . (d) Find the direction (cw/ccw) of the induced current. z [cm] v = 1cm/s 5 4 3 2 1 4 5 6 1 2 3 y [cm] 1 2 3 4 5 x [cm] 2/5/2019 [tsl478 – 37/69]
Unit Exam III: Problem #3 (Spring ’14) Consider a region of uniform magnetic field B = (3 ˆ i + 2 ˆ j + 1 ˆ k ) mT [ B = (2 ˆ i + 3 ˆ j + 1 ˆ k ) mT]. A conducting rod slides along conducting rails in the yz -plane as shown. The rails are connected on the right. The clock is set to t = 0 at the instant shown. (a) Find the magnetic flux Φ B through the conducting loop at t = 0 . (b) Find the magnetic flux Φ B through the conducting loop at t = 1 s. (c) Find the induced EMF . (d) Find the direction (cw/ccw) of the induced current. z [cm] v = 1cm/s 5 4 Solution: 3 (a) Φ B = (3cm)(2cm)(3mT) = 1 . 8 × 10 − 6 Wb 2 [Φ B = (3cm)(2cm)(2mT) = 1 . 2 × 10 − 6 Wb] 1 4 5 6 1 2 3 y [cm] (b) Φ B = (4cm)(2cm)(3mT) = 2 . 4 × 10 − 6 Wb 1 2 [Φ B = (4cm)(2cm)(2mT) = 1 . 6 × 10 − 6 Wb] 3 4 (c) E = (1cm / s)(3mT)(2cm) = 6 × 10 − 7 V 5 x [cm] [ E = (1cm / s)(2mT)(2cm) = 4 × 10 − 7 V] (d) cw [cw] 2/5/2019 [tsl478 – 37/69]
Unit Exam III: Problem #1 (Fall ’14) Consider two infinitely long, straight wires with currents I a = 7 A, I b = 9 A in the directions shown. Find direction (in/out) and magnitude of the magnetic fields B 1 , B 2 , B 3 at the points marked in the graph. I a B 3 3m B 2 B 1 3m 3m 3m I b 2/5/2019 [tsl485 – 38/69]
Unit Exam III: Problem #1 (Fall ’14) Consider two infinitely long, straight wires with currents I a = 7 A, I b = 9 A in the directions shown. Find direction (in/out) and magnitude of the magnetic fields B 1 , B 2 , B 3 at the points marked in the graph. I a B 3 3m B 2 B 1 3m Solution: 3m 3m I b • Convention used: out = positive, in = negative „ 7A • B 1 = µ 0 6m − 9A « = − 0 . 367 µ T (in). 2 π 3m „ 7A • B 2 = µ 0 3m − 9A « = − 0 . 133 µ T (in). 2 π 3m „ 7A • B 3 = µ 0 3m − 9A « = +0 . 167 µ T (out). 2 π 6m 2/5/2019 [tsl485 – 38/69]
Unit Exam III: Problem #2 (Fall ’14) Consider the (piecewise rectangular) conducting loop in the xy -plane as shown with a counterclockwise current I = 4 A in a uniform magnetic field � B = 2Tˆ j . (a) Find the magnetic moment � µ (magnitude and direction) of the loop. (b) Find the force � F (magnitude and direction) acting on the side ab of the rectangle. (c) Find the torque � τ (magnitude and direction) acting on the loop. 5m y 5m 10m I B 10m a b x z 2/5/2019 [tsl486 – 39/69]
Unit Exam III: Problem #2 (Fall ’14) Consider the (piecewise rectangular) conducting loop in the xy -plane as shown with a counterclockwise current I = 4 A in a uniform magnetic field � B = 2Tˆ j . (a) Find the magnetic moment � µ (magnitude and direction) of the loop. (b) Find the force � F (magnitude and direction) acting on the side ab of the rectangle. (c) Find the torque � τ (magnitude and direction) acting on the loop. 5m y 5m 10m I B 10m a b Solution: x µ = (4A)(75m 2 )ˆ k = 300Am 2 ˆ (a) � k. z (b) � F = I� L × � j ) = 80Nˆ B = (4A)(10mˆ i ) × (2Tˆ k. B = (300Am 2 ˆ µ × � k ) × (2Tˆ j ) = − 600Nmˆ (c) � τ = � i 2/5/2019 [tsl486 – 39/69]
Unit Exam III: Problem #3 (Fall ’14) A conducting frame with a moving conducting rod is located in a uniform magnetic field directed out of the plane as shown. The rod moves at constant velocity. (a) Find the magnetic flux Φ B through the frame and the induced emf E around the frame at the instant shown. (b) Find the magnetic flux Φ B through the frame and the induced emf E around the frame two seconds later. Write magnitudes only (in SI units), no directions. B = 5T v = 2m/s 2m 2m 4m 2m 2/5/2019 [tsl487 – 40/69]
Unit Exam III: Problem #3 (Fall ’14) A conducting frame with a moving conducting rod is located in a uniform magnetic field directed out of the plane as shown. The rod moves at constant velocity. (a) Find the magnetic flux Φ B through the frame and the induced emf E around the frame at the instant shown. (b) Find the magnetic flux Φ B through the frame and the induced emf E around the frame two seconds later. Write magnitudes only (in SI units), no directions. B = 5T v = 2m/s 2m 2m 4m 2m Solution: (a) Φ B = (16m 2 )(5T) = 80Wb , E = (2m / s)(5T)(4m) = 40V . (b) Φ B = (4m 2 )(5T) = 20Wb , E = (2m / s)(5T)(2m) = 20V . 2/5/2019 [tsl487 – 40/69]
Unit Exam III: Problem #1 (Spring ’15) A clockwise current I = 2 . 1 A is flowing around the conducting triangular frame shown in a region of uniform magnetic field � B = − 3 mT ˆ j . (a) Find the force � F ab acting on side ab of the triangle. (b) Find the force � F bc acting on side bc of the triangle. (c) Find the magnetic moment � µ of the current loop. (d) Find the torque � τ acting on the current loop. Remember that vectors have components or magnitude and direction. z [cm] 5 a 4 3 2 c b 1 4 1 2 3 5 y [cm] 1 2 3 B 4 5 x [cm] 2/5/2019 [tsl494 – 41/69]
Unit Exam III: Problem #1 (Spring ’15) A clockwise current I = 2 . 1 A is flowing around the conducting triangular frame shown in a region of uniform magnetic field � B = − 3 mT ˆ j . (a) Find the force � F ab acting on side ab of the triangle. (b) Find the force � F bc acting on side bc of the triangle. (c) Find the magnetic moment � µ of the current loop. (d) Find the torque � τ acting on the current loop. Remember that vectors have components or magnitude and direction. Solution: z [cm] (a) � F ab = (2 . 1A)( − 2cm ˆ k ) × ( − 3mT ˆ j ) = − 1 . 26 × 10 − 4 N ˆ i . 5 a (b) � 4 F bc = 0 . 3 » − 1 – (2 . 1A) = − 4 . 2 × 10 − 4 Am 2 ˆ 2 (2cm)(2cm) ˆ (c) � µ = i . 2 i c b 1 4 1 2 3 5 τ = ( − 4 . 2 × 10 − 4 Am 2 ˆ j ) = 1 . 26 × 10 − 6 Nm ˆ i ) × ( − 3mT ˆ y [cm] (d) � k . 1 2 3 B 4 5 x [cm] 2/5/2019 [tsl494 – 41/69]
Unit Exam III: Problem #2 (Spring ’15) Consider four long, straight currents in four different configurations. All currents are I = 4 mA in the directions shown ( ⊗ = in, ⊙ = out). Find the magnitude (in SI units) and the direction ( ← , → , ↑ , ↓ ) of the magnetic fields B 1 , B 2 , B 3 , B 4 generated at the points 1 , . . . , 4 , respectively. 2cm 1 2 2cm 3cm 3cm 3cm 3cm 2cm 3 4 2cm 2/5/2019 [tsl495 – 42/69]
Unit Exam III: Problem #2 (Spring ’15) Consider four long, straight currents in four different configurations. All currents are I = 4 mA in the directions shown ( ⊗ = in, ⊙ = out). Find the magnitude (in SI units) and the direction ( ← , → , ↑ , ↓ ) of the magnetic fields B 1 , B 2 , B 3 , B 4 generated at the points 1 , . . . , 4 , respectively. 2cm 1 2 2cm 3cm 3cm 3cm 3cm 2cm 3 4 Solution: 2cm • B 1 = 2 µ 0 (4mA) 2 π (3cm) = 5 . 33 × 10 − 8 T (directed ↓ ). • B 2 = 0 (no direction). • B 3 = 2 µ 0 (4mA) 2 π (2cm) = 8 . 00 × 10 − 8 T (directed → ). • B 4 = 0 (no direction). 2/5/2019 [tsl495 – 42/69]
Unit Exam III: Problem #3 (Spring ’15) A wire shaped into a triangle has resistance R = 3 . 5Ω and is placed in the yz -plane as shown. A uniform time-dependent magnetic field B = B x ( t )ˆ i is present. The dependence of B x on time is shown graphically. (a) Find magnitude | Φ (1) B | and | Φ (4) B | of the magnetic flux through the triangle at times t = 1 s and t = 4 s, respectively. (b) Find magnitude I 1 , I 4 and direction (cw/ccw) of the induced current at times t = 1 s and t = 4 s, respectively. z [m] 5 B [T] x 4 2 3 2 1 t [s] 1 0 4 1 2 3 5 2 3 4 1 5 6 y [m] −1 1 2 −2 3 B 4 5 x [m] 2/5/2019 [tsl496 – 43/69]
Unit Exam III: Problem #3 (Spring ’15) A wire shaped into a triangle has resistance R = 3 . 5Ω and is placed in the yz -plane as shown. A uniform time-dependent magnetic field B = B x ( t )ˆ i is present. The dependence of B x on time is shown graphically. (a) Find magnitude | Φ (1) B | and | Φ (4) B | of the magnetic flux through the triangle at times t = 1 s and t = 4 s, respectively. (b) Find magnitude I 1 , I 4 and direction (cw/ccw) of the induced current at times t = 1 s and t = 4 s, respectively. z [m] Solution: 5 B [T] x 4 2 (a) | Φ (1) 3 B | = | (2m 2 )( − 2T) | = 4 . 0 Wb , 2 1 t [s] | Φ (4) 1 0 B | = | (2m 2 )(0T) = 0 . 4 1 2 3 5 2 3 4 1 5 6 y [m] −1 1 2 −2 d Φ (1) ˛ ˛ ˛ ˛ ˛ A dB 3 ˛ ˛ B B ˛ = | (2m 2 )(0T / s) = 0 ˛ ˛ 4 ˛ = (b) ˛ ˛ 5 ˛ ˛ ˛ dt ˛ dt ˛ x [m] ⇒ I 1 = 0 , d Φ (4) ˛ ˛ ˛ ˛ ˛ A dB ˛ ˛ ˛ = | (2m 2 )(1T / s) | = 2 . 0V B ˛ ˛ ˛ = ˛ ˛ ˛ ˛ ˛ dt ˛ dt ˛ ⇒ I 4 = 2 . 0V 3 . 5Ω = 0 . 571A (cw). 2/5/2019 [tsl496 – 43/69]
Unit Exam III: Problem #1 (Fall ’15) Consider a region with uniform magnetic field (i) � B = 5Tˆ j , (ii) � B = − 6Tˆ i . A conducting loop in the xy -plane has the shape of a quarter circle with a clockwise current (i) I = 4 A, (ii) I = 3 A. (a) Find the magnetic moment � µ (magnitude and direction) of the loop. (b) Find the force � F (magnitude and direction) acting on the side (i) ab , (ii) bc of the loop. (c) Find the torque � τ (magnitude and direction) acting on the loop. y c I 2m b a 2m x z 2/5/2019 [tsl523 – 44/69]
Unit Exam III: Problem #1 (Fall ’15) Consider a region with uniform magnetic field (i) � B = 5Tˆ j , (ii) � B = − 6Tˆ i . A conducting loop in the xy -plane has the shape of a quarter circle with a clockwise current (i) I = 4 A, (ii) I = 3 A. (a) Find the magnetic moment � µ (magnitude and direction) of the loop. (b) Find the force � F (magnitude and direction) acting on the side (i) ab , (ii) bc of the loop. (c) Find the torque � τ (magnitude and direction) acting on the loop. y Solution: c I µ = (4A)(3 . 14m 2 )( − ˆ k ) = − 12 . 6Am 2 ˆ (ia) � k. 2m (ib) � j ) = − 40Nˆ F ab = (4A)( − 2mˆ i ) × (5Tˆ k. τ = ( − 12 . 6Am 2 ˆ k ) × (5Tˆ j ) = 63 . 0Nmˆ (ic) � i b a 2m x µ = (3A)(3 . 14m 2 )( − ˆ k ) = − 9 . 42Am 2 ˆ (iia) � k. z (iib) � F bc = (3A)(2mˆ j ) × ( − 6Tˆ i ) = 36Nˆ k. τ = ( − 9 . 42Am 2 ˆ k ) × ( − 6Tˆ i ) = 56 . 5Nmˆ (iic) � j 2/5/2019 [tsl523 – 44/69]
Unit Exam III: Problem #2 (Fall ’15) Consider two infinitely long, straight wires with currents of equal magnitude I a = I b = 6 A in the directions shown. Find direction (in/out) and magnitude of the magnetic fields B 1 , ..., B 6 at the points marked in the graph. I a B 1 B 2 B 3 2m B B 5 B 2m 6 4 2m 2m 2m 2m I b 2/5/2019 [tsl524 – 45/69]
Unit Exam III: Problem #2 (Fall ’15) Consider two infinitely long, straight wires with currents of equal magnitude I a = I b = 6 A in the directions shown. Find direction (in/out) and magnitude of the magnetic fields B 1 , ..., B 6 at the points marked in the graph. I a Solution: „ 6A • B 1 = µ 0 4m − 6A « B 1 B 2 B 3 = 0 (no direction). 2m 2 π 4m „ 6A • B 2 = µ 0 4m − 6A « B B 5 B 2m = − 0 . 3 µ T (into plane). 6 4 2 π 2m „ 6A 2m 2m 2m 2m • B 3 = µ 0 4m + 6A « = +0 . 6 µ T (out of plane). I b 2 π 4m „ 6A • B 4 = µ 0 2m + 6A « = 0 . 9 µ T (out of plane). 2 π 4m „ 6A • B 5 = µ 0 2m + 6A « = 1 . 2 µ T (out of plane). 2 π 2m „ 6A • B 6 = µ 0 2m − 6A « = 0 (no direction). 2 π 2m 2/5/2019 [tsl524 – 45/69]
Unit Exam III: Problem #3 (Fall ’15) A conducting wire bent into a square of side (i) 1.2m, (ii) 1.3m is placed in the yz -plane. The time-dependence of the magnetic field B ( t ) = B x ( t )ˆ i is shown graphically. (a) Find the magnitude | Φ B | of the magnetic flux through the square at times (i) t = 1 s, t = 3 s, and t = 4 s, (ii) t = 4 s, t = 5 s, and t = 7 s . (b) Find the magnitude |E| of the induced EMF at the above times. (c) Find the direction (cw, ccw, zero) of the induced current at the above times. B [T] x z 4 Solution: 2 t [s] 0 1 2 3 4 5 6 7 8 y −2 −4 x 2/5/2019 [tsl525 – 46/69]
Unit Exam III: Problem #3 (Fall ’15) A conducting wire bent into a square of side (i) 1.2m, (ii) 1.3m is placed in the yz -plane. The time-dependence of the magnetic field B ( t ) = B x ( t )ˆ i is shown graphically. (a) Find the magnitude | Φ B | of the magnetic flux through the square at times (i) t = 1 s, t = 3 s, and t = 4 s, (ii) t = 4 s, t = 5 s, and t = 7 s . (b) Find the magnitude |E| of the induced EMF at the above times. (c) Find the direction (cw, ccw, zero) of the induced current at the above times. B [T] x z 4 Solution: 2 (ia) | Φ (1) t [s] B | = (1 . 44m 2 )(4T) = 5 . 76 Wb 0 1 2 3 4 5 6 7 8 y −2 | Φ (3) B | = (1 . 44m 2 )(2T) = 2 . 88 Wb −4 x | Φ (4) B | = (1 . 44m 2 )(0T) = 0 (ib) E (1) = (1 . 44m 2 )(0T / s) = 0 E (3) = (1 . 44m 2 )(2T / s) = 2 . 88V E (4) = (1 . 44m 2 )(2T / s) = 2 . 88V (ic) zero, cw, cw 2/5/2019 [tsl525 – 46/69]
Unit Exam III: Problem #3 (Fall ’15) A conducting wire bent into a square of side (i) 1.2m, (ii) 1.3m is placed in the yz -plane. The time-dependence of the magnetic field B ( t ) = B x ( t )ˆ i is shown graphically. (a) Find the magnitude | Φ B | of the magnetic flux through the square at times (i) t = 1 s, t = 3 s, and t = 4 s, (ii) t = 4 s, t = 5 s, and t = 7 s . (b) Find the magnitude |E| of the induced EMF at the above times. (c) Find the direction (cw, ccw, zero) of the induced current at the above times. B [T] x z 4 Solution: 2 (iia) | Φ (4) B | = (1 . 69m 2 )(0T) = 0 t [s] 0 1 2 3 4 5 6 7 8 y −2 | Φ (5) B | = (1 . 69m 2 )(2T) = 3 . 38 Wb −4 x | Φ (7) B | = (1 . 69m 2 )(4T) = 6 . 76 Wb (iib) E (4) = (1 . 69m 2 )(2T / s) = 3 . 38V E (5) = (1 . 69m 2 )(2T / s) = 3 . 38V E (7) = (1 . 69m 2 )(0T / s) = 0 (iic) cw, cw, zero 2/5/2019 [tsl525 – 46/69]
Unit Exam III: Problem #1 (Spring ’16) Conducting squares 1 and 2, each of side 2cm, are positioned as shown. A current I = 3 A is flowing around each square in the direction shown. A uniform magnetic field � B = 5 mT ˆ k exists in the entire region. (a) Find the forces � F ab and � F cd acting on sides ab and cd , respectively. (b) Find the magnetic moments � µ 1 and � µ 2 of squares 1 and 2, respectively. (c) Find the torques � τ 1 and � τ 2 acting on squares 1 and 2, respectively. Remember that vectors have components or magnitude and direction. z [cm] 5 4 1 3 a 2 b B 1 4 1 2 3 5 y [cm] 1 d 2 2 3 4 c 5 x [cm] 2/5/2019 [tsl532 – 47/69]
Unit Exam III: Problem #1 (Spring ’16) Conducting squares 1 and 2, each of side 2cm, are positioned as shown. A current I = 3 A is flowing around each square in the direction shown. A uniform magnetic field � B = 5 mT ˆ k exists in the entire region. (a) Find the forces � F ab and � F cd acting on sides ab and cd , respectively. (b) Find the magnetic moments � µ 1 and � µ 2 of squares 1 and 2, respectively. (c) Find the torques � τ 1 and � τ 2 acting on squares 1 and 2, respectively. Remember that vectors have components or magnitude and direction. z [cm] Solution: 5 (a) � F ab = (3A)(2cm ˆ j ) × (5mT ˆ k ) = 3 × 10 − 4 N ˆ 4 i . 1 3 � F cd = (3A)( − 2cm ˆ i ) × (5mT ˆ k ) = 3 × 10 − 4 N ˆ j . a 2 b B µ 1 = (2cm) 2 (3A) ˆ i = 1 . 2 × 10 − 3 Am 2 ˆ (b) � i . 1 4 1 2 3 5 µ 2 = (2cm) 2 (3A) ˆ k = 1 . 2 × 10 − 3 Am 2 ˆ � k . y [cm] 1 d 2 τ 1 = (1 . 2 × 10 − 3 Am 2 ˆ k ) = − 6 × 10 − 6 Nm ˆ i ) × (5mT ˆ 2 (d) � j . 3 4 τ 2 = (1 . 2 × 10 − 3 Am 2 ˆ c k ) × (5mT ˆ 5 � k ) = 0 . x [cm] 2/5/2019 [tsl532 – 47/69]
Unit Exam III: Problem #2 (Spring ’16) (a) Consider two long, straight currents I = 3 mA in the directions shown. Find the magnitude of the magnetic field at point a . Find the directions ( ← , → , ↑ , ↓ ) of the magnetic field at points b and c . (b) Consider a circular current I = 3 mA in the direction shown. Find the magnitude of the magnetic field at point d . Find the directions ( ⊗ , ⊙ ) of the magnetic field at points e and f . I (a) (b) c I I d e f a 9cm 7cm 7cm b 2/5/2019 [tsl533 – 48/69]
Unit Exam III: Problem #2 (Spring ’16) (a) Consider two long, straight currents I = 3 mA in the directions shown. Find the magnitude of the magnetic field at point a . Find the directions ( ← , → , ↑ , ↓ ) of the magnetic field at points b and c . (b) Consider a circular current I = 3 mA in the direction shown. Find the magnitude of the magnetic field at point d . Find the directions ( ⊗ , ⊙ ) of the magnetic field at points e and f . I (a) (b) c I I d e f a 9cm 7cm 7cm b Solution: (a) B a = 2 µ 0 (3mA) 2 π (7cm) = 1 . 71 × 10 − 8 T B b ↑ , B c ↑ . (b) B d = µ 0 (3mA) = 2 . 09 × 10 − 8 T , B e ⊙ , B f ⊗ . 2(9cm) 2/5/2019 [tsl533 – 48/69]
Unit Exam III: Problem #3 (Spring ’16) A wire shaped into a rectangular loop as shown is placed in the yz -plane. A uniform time-dependent magnetic field B = B x ( t )ˆ i is present. The dependence of B x on time is shown graphically. (a) Find magnitude | Φ (2) B | of the magnetic flux through the loop at time t = 2 s. (b) Find magnitude | Φ (5) B | of the magnetic flux through the loop at time t = 5 s. (c) Find magnitude |E (2) | of the induced EMF at time t = 2 s. (d) Find magnitude |E (5) | of the induced EMF at time t = 5 s. (e) Find the direction (cw/ccw) and magnitude I of the induced current at time t = 2 s if the wire has resistance 1Ω per meter of length. z [m] 5 B [T] x 4 2 3 2 1 t [s] 1 0 4 1 2 3 5 2 3 4 1 5 6 y [m] −1 1 −2 2 3 B 4 5 x [m] 2/5/2019 [tsl534 – 49/69]
Unit Exam III: Problem #3 (Spring ’16) A wire shaped into a rectangular loop as shown is placed in the yz -plane. A uniform time-dependent magnetic field B = B x ( t )ˆ i is present. The dependence of B x on time is shown graphically. (a) Find magnitude | Φ (2) B | of the magnetic flux through the loop at time t = 2 s. (b) Find magnitude | Φ (5) B | of the magnetic flux through the loop at time t = 5 s. (c) Find magnitude |E (2) | of the induced EMF at time t = 2 s. (d) Find magnitude |E (5) | of the induced EMF at time t = 5 s. (e) Find the direction (cw/ccw) and magnitude I of the induced current at time t = 2 s if the wire has resistance 1Ω per meter of length. z [m] Solution: 5 (a) | Φ (2) B | = | (8m 2 )(0T) | = 0 , B [T] x 4 2 3 (b) | Φ (5) B | = | (8m 2 )(2T) | = 16 Wb , 2 1 t [s] 1 0 4 1 2 3 5 ˛ ˛ 2 3 4 ˛ A dB 1 5 6 y [m] −1 (c) |E (2) | = ˛ = | (8m 2 )(1T / s) = 8V ˛ ˛ 1 ˛ ˛ −2 2 dt 3 B 4 5 ˛ ˛ ˛ A dB (d) |E (5) | = ˛ = | (8m 2 )(0T / s) = 0 ˛ ˛ x [m] ˛ ˛ dt (e) I (2) = 8V 12Ω = 0 . 667A . (cw). 2/5/2019 [tsl534 – 49/69]
Unit Exam III: Problem #1 (Fall ’16) A current I is flowing around the conducting rectangular frame in the direction shown. The frame is located in a region of uniform magnetic field B . (a) Find the force F ab (magnitude and direction) acting on side ab . (b) Find the force F bc (magnitude and direction) acting on side bc . (c) Find the magnetic moment � µ (magnitude and direction) of the current loop. (d) Find the torque � τ (magnitude and direction) acting on the frame. z [cm] 5 c b 4 3 2 a 1 4 1 2 3 5 y [cm] 1 2 3 4 5 x [cm] 2/5/2019 [tsl541 – 50/69]
Recommend
More recommend