Hypergeometric Probability Distributions MDM4U: Mathematics of Data - PDF document

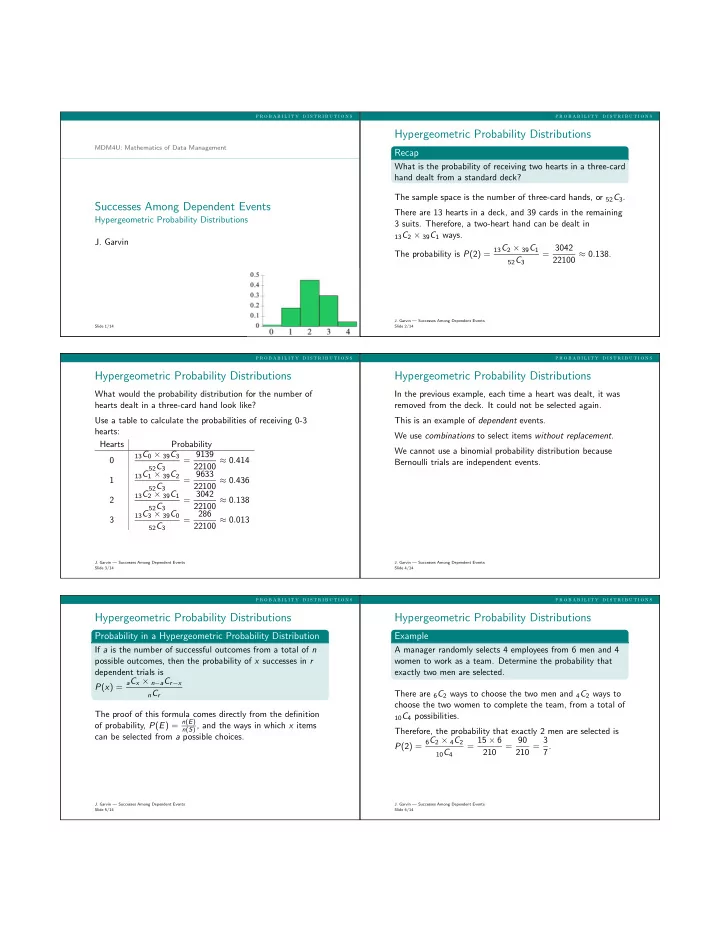
p r o b a b i l i t y d i s t r i b u t i o n s p r o b a b i l i t y d i s t r i b u t i o n s Hypergeometric Probability Distributions MDM4U: Mathematics of Data Management Recap What is the probability of receiving two hearts in a three-card hand dealt from a standard deck? The sample space is the number of three-card hands, or 52 C 3 . Successes Among Dependent Events There are 13 hearts in a deck, and 39 cards in the remaining Hypergeometric Probability Distributions 3 suits. Therefore, a two-heart hand can be dealt in 13 C 2 × 39 C 1 ways. J. Garvin The probability is P (2) = 13 C 2 × 39 C 1 = 3042 22100 ≈ 0 . 138. 52 C 3 J. Garvin — Successes Among Dependent Events Slide 1/14 Slide 2/14 p r o b a b i l i t y d i s t r i b u t i o n s p r o b a b i l i t y d i s t r i b u t i o n s Hypergeometric Probability Distributions Hypergeometric Probability Distributions What would the probability distribution for the number of In the previous example, each time a heart was dealt, it was hearts dealt in a three-card hand look like? removed from the deck. It could not be selected again. Use a table to calculate the probabilities of receiving 0-3 This is an example of dependent events. hearts: We use combinations to select items without replacement . Hearts Probability We cannot use a binomial probability distribution because = 9139 13 C 0 × 39 C 3 0 22100 ≈ 0 . 414 Bernoulli trials are independent events. 52 C 3 13 C 1 × 39 C 2 = 9633 1 22100 ≈ 0 . 436 52 C 3 13 C 2 × 39 C 1 = 3042 2 22100 ≈ 0 . 138 52 C 3 13 C 3 × 39 C 0 286 3 = 22100 ≈ 0 . 013 52 C 3 J. Garvin — Successes Among Dependent Events J. Garvin — Successes Among Dependent Events Slide 3/14 Slide 4/14 p r o b a b i l i t y d i s t r i b u t i o n s p r o b a b i l i t y d i s t r i b u t i o n s Hypergeometric Probability Distributions Hypergeometric Probability Distributions Probability in a Hypergeometric Probability Distribution Example If a is the number of successful outcomes from a total of n A manager randomly selects 4 employees from 6 men and 4 possible outcomes, then the probability of x successes in r women to work as a team. Determine the probability that dependent trials is exactly two men are selected. P ( x ) = a C x × n − a C r − x n C r There are 6 C 2 ways to choose the two men and 4 C 2 ways to choose the two women to complete the team, from a total of The proof of this formula comes directly from the definition 10 C 4 possibilities. of probability, P ( E ) = n ( E ) n ( S ) , and the ways in which x items Therefore, the probability that exactly 2 men are selected is can be selected from a possible choices. = 15 × 6 = 90 210 = 3 P (2) = 6 C 2 × 4 C 2 7. 10 C 4 210 J. Garvin — Successes Among Dependent Events J. Garvin — Successes Among Dependent Events Slide 5/14 Slide 6/14

p r o b a b i l i t y d i s t r i b u t i o n s p r o b a b i l i t y d i s t r i b u t i o n s Hypergeometric Probability Distributions Hypergeometric Probability Distributions Your Turn Example You shuffle a standard deck of cards, and deal a hand of five A quality control inspector at a popsicle factory knows that cards from atop the deck. What is the probability that the approximately 6% of all popsicles shipped by the factory are hand contains exactly three clubs? broken. He randomly pulls 10 popsicles from a batch of 500 for testing. What is the probability that at least 2 are broken? There are 13 C 3 ways to choose the clubs and 39 C 2 ways to choose the remaining two cards in the hand. If 6% of all popsicles are broken, there should be 500 × 0 . 06 = 30 broken popsicles in the batch. There are a total of 52 C 5 possible five-card hands. This means there are 30 broken ( a ) and 470 ( n − a ) Therefore, the probability of having exactly 3 clubs is non-broken popsicles, from which 10 ( x ) must be selected = 2 717 P (3) = 13 C 3 × 39 C 2 33 320 ≈ 0 . 08. from a total of 500 ( n ) popsicles. 52 C 5 J. Garvin — Successes Among Dependent Events J. Garvin — Successes Among Dependent Events Slide 7/14 Slide 8/14 p r o b a b i l i t y d i s t r i b u t i o n s p r o b a b i l i t y d i s t r i b u t i o n s Hypergeometric Probability Distributions Expected Value To find the probability that at least 2 are broken, use an Like the previous probability distributions, there is a formula indirect method, eliminating the cases where no popsicles or for expected value. one popsicle is broken. Example P ( x ≥ 2) = 1 − P (0) − P (1) In a hypergeometric probability distribution consisting of r trials, with a successful outcomes from a total of n possible = 1 − 30 C 0 × 470 C 10 − 30 C 1 × 470 C 9 outcomes, the expected value is 500 C 10 500 C 10 E ( X ) = ra ≈ 0 . 116 n Since the proof of this formula requires a great deal of algebraic manipulation, it is left as an exercise for the determined student. J. Garvin — Successes Among Dependent Events J. Garvin — Successes Among Dependent Events Slide 9/14 Slide 10/14 p r o b a b i l i t y d i s t r i b u t i o n s p r o b a b i l i t y d i s t r i b u t i o n s Expected Value Expected Value Your Turn Example 1000 fish from a small lake were caught and tagged. After A bag contains 15 red and 20 blue marbles. If 7 marbles are one year, a new catch of 500 fish contained 60 that were randomly drawn from the bag, what is the expected number tagged. What is the estimated fish population in the lake? of red marbles drawn? Hint: What do the three values given above represent in the There are r = 7 dependent trials, and a = 15 successful equation for expected value? outcomes from a total of n = 15 + 20 = 35 possible There are r = 500 trials (the new catch). outcomes. There are a = 1000 successful outcomes (catching a tagged So the expected number of red marbles drawn is E ( X ) = 7 × 15 = 105 fish). 35 = 3. 35 The expected value is E ( X ) = 60 (the number of tagged fish caught). Substituting into the expected value equation, we obtain 60 = 500 × 1000 , or n = 500 × 1000 ≈ 8333 fish. 60 n J. Garvin — Successes Among Dependent Events J. Garvin — Successes Among Dependent Events Slide 11/14 Slide 12/14

p r o b a b i l i t y d i s t r i b u t i o n s p r o b a b i l i t y d i s t r i b u t i o n s Expected Value Questions? A alternative method of solving is to assume that the proportion of tagged fish in the sample corresponds to that of the entire population. 60 tagged fish were caught in a sample of 500, for a proportion 60 500. 1000 fish from the entire population were originally tagged, for a proportion 1000 . n Therefore, 60 500 = 1000 . This can be rearranged as n n = 500 × 1000 ≈ 8333 fish, as obtained earlier. 60 J. Garvin — Successes Among Dependent Events J. Garvin — Successes Among Dependent Events Slide 13/14 Slide 14/14