five
play

five moments and http://www.physics.umd.edu same translation but - PowerPoint PPT Presentation

Aug 24, 2023 •25 likes •115 views

E LEMENTS OF A RCHITECTURAL S TRUCTURES : Moments F ORM, B EHAVIOR, AND D ESIGN forces have the tendency to make a ARCH 614 D R. A NNE N ICHOLS body rotate about an axis S PRING 2019 lecture five moments and http://www.physics.umd.edu

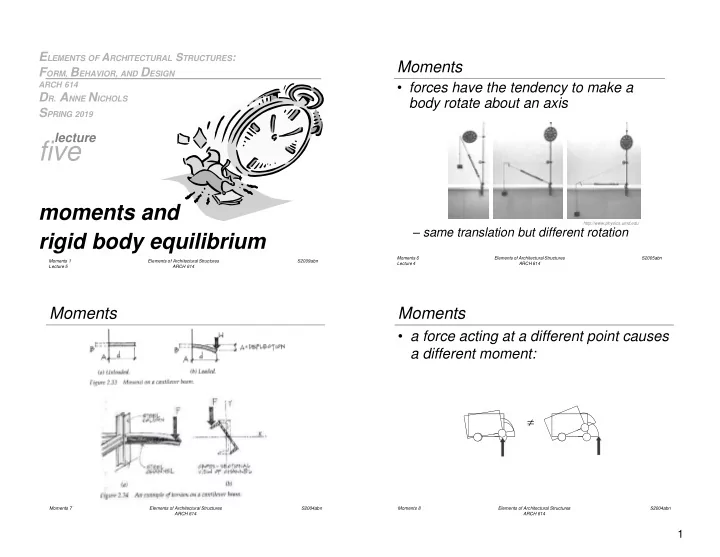
  1. E LEMENTS OF A RCHITECTURAL S TRUCTURES : Moments F ORM, B EHAVIOR, AND D ESIGN • forces have the tendency to make a ARCH 614 D R. A NNE N ICHOLS body rotate about an axis S PRING 2019 lecture five moments and http://www.physics.umd.edu – same translation but different rotation rigid body equilibrium Moments 6 Elements of Architectural Structures S2005abn Moments 1 Elements of Architectural Structures S2009abn Lecture 4 ARCH 614 Lecture 5 ARCH 614 Moments Moments • a force acting at a different point causes a different moment:  Moments 7 Elements of Architectural Structures S2004abn Moments 8 Elements of Architectural Structures S2004abn ARCH 614 ARCH 614 1

  2. Moments Moments • defined by magnitude and direction • with same F: • units: N  m, k  ft      M F d M F d • direction: A 1 A 2 F (bigger) + cw (!) C - ccw A B • value found from F d and  distance   M F d • d also called “ lever ” or “ moment ” arm Moments 9 Elements of Architectural Structures S2005abn Moments 10 Elements of Architectural Structures S2005abn Lecture 4 ARCH 614 Lecture 4 ARCH 614 Moments Moments • additive with sign convention • Varignon’s Theorem • can still move the force – resolve a force into components at a point and finding perpendicular distances along the line of action – calculate sum of moments • location of moment independent – equivalent to original moment B B • makes life easier! d  F d  A = A – geometry d d F – when component runs through point, d=0 M A = F  d M A = F  d - M B = F  d  M B = F  d  Moments 11 Elements of Architectural Structures S2004abn Moments 12 Elements of Architectural Structures S2004abn ARCH 614 ARCH 614 2

  3. Physics and Moments of a Force Moments of a Force • my Physics book (right hand rule): • moments of a force – introduced in Physics as “ Torque Acting on a Particle ” – and used to satisfy rotational equilibrium Moments 10 Elements of Architectural Structures S2006abn Moments 9 Elements of Architectural Structures S2006abn Lecture 4 ARCH 614 Lecture 4 ARCH 614 Moment Couples Moment Couples • 2 forces • equivalent couples – same size – same magnitude and direction F F – opposite direction – F & d may be different d 1 d 1 – distance d apart d d A A – cw or ccw d 2 d 2   300 N 300 N F F M F d 120 N 120 N 100 mm 100 mm 300 N 300 N 200 N 200 N 120 N 120 N 250 mm 250 mm 150 mm 150 mm – not dependant on point of application 200 N 200 N     M F d F d 1 2 TOPIC 13 Elements of Architectural Structures S2004abn Moments 14 Elements of Architectural Structures S2004abn ARCH 614 ARCH 614 3

  4. Moment Couples Moment Couples • added just like moments caused by one • moment couples in structures force • can replace two couples with a single couple 300 N 300 N 240 N 240 N 100 mm 100 mm 200 N 200 N 300 N 300 N + + 240 N 240 N = = 250 mm 250 mm 150 mm 150 mm 200 N 200 N Moments 15 Elements of Architectural Structures S2004abn Moments 14 Elements of Architectural Structures S2006abn ARCH 614 Lecture 4 ARCH 614 Equivalent Force Systems Force-Moment Systems • two forces at a point is equivalent to the • single force causing a moment can be resultant at a point replaced by the same force at a • resultant is equivalent to two different point by providing the moment components at a point that force caused • resultant of equal & opposite forces at a point is zero F F F F F F F F F F F F A  A  A  A  A  A  A  A  d d d d • put equal & opposite forces at a point -F -F -F -F A A A A A A A A (sum to 0) • transmission of a force along action line • moments are shown as arched arrows Moments 16 Elements of Architectural Structures S2005abn Moments 16 Elements of Architectural Structures S2004abn Lecture 4 ARCH 614 ARCH 614 4

  5. Parallel Force Systems Force-Moment Systems • forces are in the same direction • a force-moment pair can be replaced by a force at another point causing the • can find resultant force original moment • need to find location for equivalent moments     M=F  d M=F  d M=F  d M=F  d F F F F F F F F A  A  ( ( A A B B ) ) x x R=A+B R=A+B F F F F F F F F A  A  A  A  a a A  A  A  A  A  A  A  A  d d d d B B A A A A A A A A A A -F -F -F -F A A A A C C D D C C D D a a x x b b B  B  b b Moments 18 Elements of Architectural Structures S2004abn Moments 17 Elements of Architectural Structures S2004abn ARCH 614 ARCH 614 Free Body Diagram Equilibrium • rigid body • FBD (sketch) – doesn ’ t deform • tool to see all forces on a body or a C B – coplanar force systems point including A   • static: – external forces  R F 0 (  H) x x – weights   – force reactions  R F 0 y y (  V) – external moments   M  – moment reactions M 0 – internal forces Equilibrium 3 Elements of Architectural Structures S2006abn Equilibrium 10 Elements of Architectural Structures S2004abn Lecture 5 ARCH 614 ARCH 614 5

  6. Free Body Diagram Free Body Diagram • determine body • sketch FBD with relevant geometry • FREE it from: 100 lb • resolve each force into components – known & unknown angles – name them – ground + weight – known & unknown forces – name them – supports & connections – known & unknown moments – name them • draw all external forces • are any forces related to other forces? acting ON the body 100 lb • for the unknowns – reactions m  g • write only as many equilibrium equations as – applied forces needed – gravity • solve up to 3 equations Equilibrium 11 Elements of Architectural Structures S2004abn ARCH 614 Equilibrium 12 Elements of Architectural Structures S2004abn ARCH 614 Free Body Diagram Reactions on Rigid Bodies • solve equations • result of applying force – most times 1 unknown easily solved • unknown size – plug into other equation(s) • connection or support type – known direction • common to have unknowns of – related to motion prevented – force magnitudes – force angles – moment magnitudes no translation no vertical motion no rotation no translation Equilibrium 19 Elements of Architectural Structures S2004abn Equilibrium 10 Elements of Architectural Structures S2006abn ARCH 614 Lecture 5 ARCH 614 6

  7. Supports and Connections Supports and Connections Equilibrium 20 Elements of Architectural Structures S2004abn Equilibrium 21 Elements of Architectural Structures S2004abn ARCH 614 ARCH 614 Moment Equations Concentrated Loads • sum moments at intersection where the most forces intersect • multiple moment equations may not be useful • combos:    F x  F  M 1  0 0 0    F y  M 1  M 2  0 0 0    1  2  3  M 0 M 0 M 0 Equilibrium 21 Elements of Architectural Structures S2005abn Loads 15 Elements of Architectural Structures S2004abn Lecture 5 ARCH 614 ARCH 614 7

  8. Distributed Loads Beam Supports • statically determinate L L L simply supported overhang cantilever (most common) • statically indeterminate L L L L Propped Restrained continuous (most common case when L 1 =L 2 ) Loads 16 Elements of Architectural Structures S2004abn Internal Beam Forces 20 Elements of Architectural Structures S2004abn ARCH 614 Lecture 12 ARCH 614 Equivalent Force Systems Load Areas • replace forces by resultant • area is width x “ height ” of load • place resultant where M = 0 • w is load per unit length • using calculus and area centroids • W is total load L      W wdx dA A loading 0 loading  w x W 2w    w x W w 2 2 w w y 0 w(x) x x x W W W/2 W/2 dx x x x/2 x/2 2x/3 x/3 x/2 x/6 x/3 L x el dx Loads 17 Elements of Architectural Structures S2006abn Loads 19 Elements of Architectural Structures S2006abn Lecture 9 ARCH 614 Lecture 9 ARCH 614 8

  9. Method of Sections Method of Sections • relies on internal forces being in • joints on or off the section are good to equilibrium on a section sum moments • cut to expose 3 or less members • quick for few members • coplanar forces   M = 0 too • not always obvious where to cut or sum P P P P . C C AC AC D D F F A . A . E B E B B A AB AB A B y B y Steel Trusses 15 Elements of Architectural Structures S2007abn Steel Trusses 16 Elements of Architectural Structures S2007abn Lecture 17 ARCH 614 Lecture 17 ARCH 614 9

Recommend

More recommend