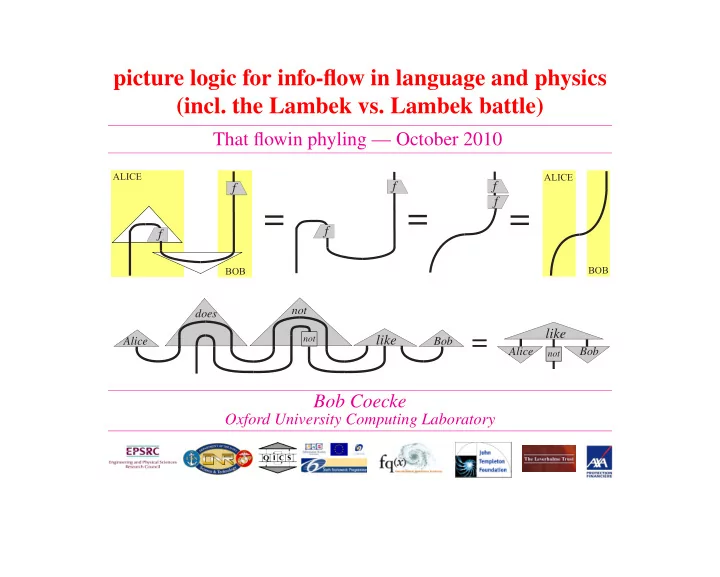

picture logic for info-flow in language and physics (incl. the Lambek vs. Lambek battle) That flowin phyling — October 2010 ALICE ALICE f f f f = = = f f BOB BOB not does like not like = Alice Bob Alice Bob not Bob Coecke Oxford University Computing Laboratory
Context: S. Abramsky and B. Coecke (2004) A categorical semantics of quantum protocols . In: Proc. 19th IEEE LiCS. IEEE Press.
Context: S. Abramsky and B. Coecke (2004) A categorical semantics of quantum protocols . In: Proc. 19th IEEE LiCS. IEEE Press. J. Lambek (2010; talk in 2006 at Cats, Kets, Cloisters) Compact monoidal categories from Linguistics to Physics . In: New Struc- tures for Physics, B. Coecke, Ed., Springer-Verlag.
Context: S. Abramsky and B. Coecke (2004) A categorical semantics of quantum protocols . In: Proc. 19th IEEE LiCS. IEEE Press. J. Lambek (2010; talk in 2006 at Cats, Kets, Cloisters) Compact monoidal categories from Linguistics to Physics . In: New Struc- tures for Physics, B. Coecke, Ed., Springer-Verlag. S. Clark and S. Pulman (2007) Combining symbolic and distribu- tional models of meaning . Proceedings of AAAI Spring Sympo- sium on Quantum Interaction.
Context: S. Abramsky and B. Coecke (2004) A categorical semantics of quantum protocols . In: Proc. 19th IEEE LiCS. IEEE Press. J. Lambek (2010; talk in 2006 at Cats, Kets, Cloisters) Compact monoidal categories from Linguistics to Physics . In: New Struc- tures for Physics, B. Coecke, Ed., Springer-Verlag. S. Clark and S. Pulman (2007) Combining symbolic and distribu- tional models of meaning . Proceedings of AAAI Spring Sympo- sium on Quantum Interaction. B. Coecke, M. Sadrzadeh and S. Clark (2008; 2010) Mathemat- ical Foundations for a Compositional Distributional Model of Meaning . Linguistic Analysis - Lambek Festschrift.
This talk: Survey of aspects of categories and graphical language: • What is a monoidal category • Connection to categories • Known completeness results
This talk: Survey of aspects of categories and graphical language: • What is a monoidal category • Connection to categories • Known completeness results Pictures of quantum state information flow Pictures of linguistic meaning information flow
This talk: Survey of aspects of categories and graphical language: • What is a monoidal category • Connection to categories • Known completeness results Pictures of quantum state information flow Pictures of linguistic meaning information flow Differences between these two Flexibility of these two
WHY ‘MONOIDAL’ CATEGORIES?
BECAUSE THEY ARE EVERYWHERE!
1. Let A be a raw potato.
1. Let A be a raw potato. A admits many states e.g. dirty, clean, skinned, ...
1. Let A be a raw potato. A admits many states e.g. dirty, clean, skinned, ... 2. We want to process A into cooked potato B . B admits many states e.g. boiled, fried, deep fried, baked with skin, baked without skin, ...
1. Let A be a raw potato. A admits many states e.g. dirty, clean, skinned, ... 2. We want to process A into cooked potato B . B admits many states e.g. boiled, fried, deep fried, baked with skin, baked without skin, ... Let f ′ f ′′ f ✲ B ✲ B ✲ B A A A be boiling, frying, baking.
1. Let A be a raw potato. A admits many states e.g. dirty, clean, skinned, ... 2. We want to process A into cooked potato B . B admits many states e.g. boiled, fried, deep fried, baked with skin, baked without skin, ... Let f ′ f ′′ f ✲ B ✲ B ✲ B A A A be boiling, frying, baking. States are processes ψ ✲ A. I := unspecified
3. Let g ◦ f ✲ C A f ✲ B and be the composite process of first boiling A g ✲ C . then salting B
3. Let g ◦ f ✲ C A f ✲ B and be the composite process of first boiling A g ✲ C . Let then salting B 1 X ✲ X X be doing nothing. We have 1 Y ◦ ξ = ξ ◦ 1 X = ξ .
4. Let A ⊗ D be potato A and carrot D and let
4. Let A ⊗ D be potato A and carrot D and let f ⊗ h ✲ B ⊗ E A ⊗ D be boiling potato while frying carrot.
4. Let A ⊗ D be potato A and carrot D and let f ⊗ h ✲ B ⊗ E A ⊗ D be boiling potato while frying carrot. Let x ✲ M C ⊗ F be mashing spice-cook-potato and spice-cook-carrot.
5. Total process : f ⊗ h g ⊗ k x ✲ M = A ⊗ D x ◦ ( g ⊗ k ) ◦ ( f ⊗ h ) ✲ B ⊗ E ✲ C ⊗ F ✲ M. A ⊗ D
5. Total process : f ⊗ h g ⊗ k x ✲ M = A ⊗ D x ◦ ( g ⊗ k ) ◦ ( f ⊗ h ) ✲ B ⊗ E ✲ C ⊗ F ✲ M. A ⊗ D 6. Recipe = composition structure on processes .
5. Total process : f ⊗ h g ⊗ k x ✲ M = A ⊗ D x ◦ ( g ⊗ k ) ◦ ( f ⊗ h ) ✲ B ⊗ E ✲ C ⊗ F ✲ M. A ⊗ D 6. Recipe = composition structure on processes . 7. Law governing recipes :
5. Total process : f ⊗ h g ⊗ k x ✲ M = A ⊗ D x ◦ ( g ⊗ k ) ◦ ( f ⊗ h ) ✲ B ⊗ E ✲ C ⊗ F ✲ M. A ⊗ D 6. Recipe = composition structure on processes . 7. Law governing recipes : ( 1 B ⊗ g ) ◦ ( f ⊗ 1 C ) = ( f ⊗ 1 D ) ◦ ( 1 A ⊗ g )
5. Total process : f ⊗ h g ⊗ k x ✲ M = A ⊗ D x ◦ ( g ⊗ k ) ◦ ( f ⊗ h ) ✲ B ⊗ E ✲ C ⊗ F ✲ M. A ⊗ D 6. Recipe = composition structure on processes . 7. Law governing recipes : ( 1 B ⊗ g ) ◦ ( f ⊗ 1 C ) = ( f ⊗ 1 D ) ◦ ( 1 A ⊗ g ) i.e. boil potato then fry carrot = fry carrot then boil potato
Very successful in proof theory and programming : proof theory programming Propositions Data Types Proofs Programs BLUE = systems Red = processes
Very successful in proof theory and programming : proof theory programming Propositions Data Types Proofs Programs BLUE = systems Red = processes but also applies to: biology physics language Biological syst. Physical syst. language syst. Biological proc Physical proc. language proc.
Very successful in proof theory and programming : proof theory programming Propositions Data Types Proofs Programs BLUE = systems Red = processes but also applies to: biology physics language Biological syst. Physical syst. language syst. Biological proc Physical proc. language proc.
A MINIMAL LANGUAGE FOR QUANTUM REASONING Abramsky & Coecke (2004) A categorical semantics for quantum protocols . arXiv:quant-ph/0402130 Coecke (2005) Kindergarten quantum mechanics . arXiv:quant-ph/0510032
— (physical) data in the language — Systems: A B C Processes: f g h ✲ A ✲ B ✲ C A A B Compound systems: f ⊗ g ✲ B ⊗ D A ⊗ B I A ⊗ C Temporal composition: h ◦ g g h 1 A ✲ A ✲ C := A ✲ B ✲ C A A
— graphical notation — g g f f g ◦ f ≡ f ⊗ g ≡ f Roger Penrose (1971) Applications of negative dimensional tensors . In: Combinatorial Mathematics and its Applications. Academic Press. Andr´ e Joyal & Ross Street (1991) The geometry of tensor calculus I. Advances in Mathematics 88 , 55–112.
— merely a new notation? — ( g ◦ f ) ⊗ ( k ◦ h ) = ( g ⊗ k ) ◦ ( f ⊗ h )
— merely a new notation? — ( g ◦ f ) ⊗ ( k ◦ h ) = ( g ⊗ k ) ◦ ( f ⊗ h ) g g k k = f h f h
— merely a new notation? — ( g ◦ f ) ⊗ ( k ◦ h ) = ( g ⊗ k ) ◦ ( f ⊗ h ) g g k k = f h f h peel potato and then fry it, peel potato while clean carrot, = while, and then, clean carrot and then boil it fry potato while boil carrot
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— graphical notation — ψ : I → A π : A → I π ◦ ψ : I → I π π A ψ ψ A
— adjoint — f : A → B B f A
— adjoint — f † : B → A A f B
— asserting (pure) entanglement — = quantum classical = =
— quantum-like — A A
— quantum-like — A A A A = A A
— quantum-like — A A A A = A A
— quantum-like — A A A A = A A
— quantum-like — A A A A = A A
— quantum-like — A A A A = A A
— quantum-like — = f f
— sliding — f = = f f f
— sliding — f = = f f f In QM: cups = Bell-states, caps =Bell-effects, π -rotations = transpose
classical data flow? f f f = f
classical data flow? f = f
classical data flow? f = f
classical data flow? ALICE ALICE f = f BOB BOB ⇒ quantum teleportation
Applying “decorated” normalization 3 f f f = f ⇒ Entanglement swapping
Recommend
More recommend