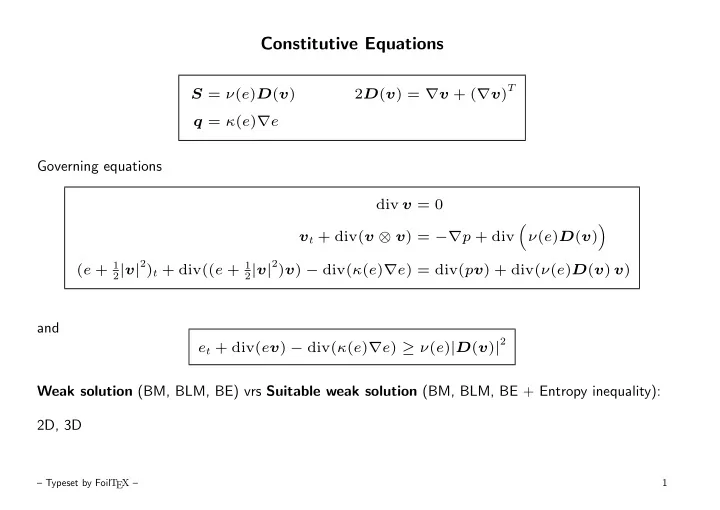

Constitutive Equations 2 D ( v ) = ∇ v + ( ∇ v ) T S = ν ( e ) D ( v ) q = κ ( e ) ∇ e Governing equations div v = 0 � � v t + div( v ⊗ v ) = −∇ p + div ν ( e ) D ( v ) 2 | v | 2 ) t + div(( e + 1 2 | v | 2 ) v ) − div( κ ( e ) ∇ e ) = div( p v ) + div( ν ( e ) D ( v ) v ) ( e + 1 and e t + div( e v ) − div( κ ( e ) ∇ e ) ≥ ν ( e ) | D ( v ) | 2 Weak solution (BM, BLM, BE) vrs Suitable weak solution (BM, BLM, BE + Entropy inequality): 2D, 3D – Typeset by Foil T EX – 1
Energy estimates and their consequences � � div v = 0 v t + div( v ⊗ v ) = −∇ p + div ν ( . . . ) D ( v ) e + | v | 2 e + | v | 2 � � �� � � � � t + div − div( κ ( . . . ) ∇ e ) = div( p v ) + div ν ( . . . ) D ( v ) v v 2 2 e t + div( e v ) − div( κ ( . . . ) ∇ e )( ≥ ) = ν ( . . . ) | D ( v ) | 2 e 0 + | v 0 | 2 e + | v | 2 e ∈ L ∞ ( L 1 ) v ∈ L ∞ ( L 2 ) � � � � � � • ( t, x ) dx ≤ dx = ⇒ Ω 2 Ω 2 � T 0 ν ( . . . ) | D ( v ) | 2 dx ≤ C ∇ v ∈ L 2 ( L 2 ) • = ⇒ e > C ∗ a.e. , e ∈ L m ( L m ) , ∇ ( e ) (1 − λ ) / 2 ∈ L 2 ( L 2 ) • ν ( . . . ) | D ( v ) | 2 ≥ 0 , = ⇒ p = ( − ∆) − 1 div div( v ⊗ v − ν ( . . . ) D ( v )) Equation for the pressure (Navier’s slip) v ∈ L 10 / 3 ( L 10 / 3 ) p ∈ L 5 / 3 ( L 5 / 3 ) • v ∈ L ∞ ( L 2 ) and ∇ v ∈ L 2 ( L 2 ) = ⇒ and – Typeset by Foil T EX – 2
Energy estimates and their consequences � � div v = 0 v t + div( v ⊗ v ) = −∇ p + div ν ( . . . ) D ( v ) e + | v | 2 e + | v | 2 � � �� � � � � t + div − div( κ ( . . . ) ∇ e ) = div( p v ) + div ν ( . . . ) D ( v ) v v 2 2 ν ( . . . ) | D ( v ) | 2 e t + div( e v ) − div( κ ( . . . ) ∇ e ) ( ≥ ) = � ∗ � L 5 / 2 ( W 1 , 5 / 2 ) = L − 5 / 3 ( W 1 , − 5 / 3 ) • v t ∈ • e t ∈ L 1 ( W − 1 ,q ′ ) with q > 10 • Aubin-Lions lemma and its generalization: v and e precompact in L m ( L m ) for m ∈ [1 , 5 3 ) • Trace theorem and Aubin-Lions lemma: pre-compactness of v on ∂ Ω • Vitali’s theorem Two steps in the proof of existence • Stability of the system w.r.t. weakly converging sequences • Constructions of approximations (several levels), derivation of uniform estimates, weak limits - candidates for the solutions, taking limits in nonlinearities – Typeset by Foil T EX – 3
Result #1 Theorem 1. ( M. Bul´ alek, ’06-’07 ) Assume that ıˇ cek, E. Feireisl , J. M´ ν 1 ≥ ν ( s ) ≥ ν 0 > 0 and κ 1 ≥ κ ( s ) ≥ κ 0 > 0 for all s ∈ R n ,div and e 0 ∈ L 1 , e 0 ≥ C ∗ > 0 a.a. in Ω . Let g ∈ L 1 (0 , T ) . Let ∂ Ω ∈ C 1 , 1 , v 0 ∈ L 2 Then for all T > 0 (and any α ∈ [0 , ∞ ) ) there exists (suitable) weak solution ( v , p ) to the system in consideration, completed by Navier’s slip boundary conditions, such that L 2 weak ) ∩ L 2 (0 , T ; W 1 , 2 � v ∈ C (0 , T ; n ,div ) tr v ∈ L 2 (0 , T ; L 2 ( ∂ Ω)) � 5 5 p ∈ L 3 (0 , T ; L 3 ) p ( t, x ) dx = g ( t ) Ω m ∈ [1 , 5 3) , n ∈ [1 , 5 e ∈ L ∞ (0 , T ; L 1 ) ∩ L m (0 , T ; L m ) ∩ L n (0 , T ; W 1 ,n ) 4) ( p + | v | 2 10 10 5 5 9 (0 , T ; L 9 ) 2 ) v ∈ L D ( v ) v ∈ L 4 ([0 , T ]; L 4 ) – Typeset by Foil T EX – 4
Fluids with shear rate dependent viscosities S = ν ( | D | 2 ) D ( v ) If v = ( u ( x 2 ) , 0 , 0) , then | D ( v ) | 2 = 1 / 2 | u ′ | 2 ... shear rate. • ν ( | D | 2 ) = | D | r − 2 • ν ( | D | 2 ) = ν 0 + ν 1 | D | r − 2 1 < r < ∞ r > 2 • power-law model • Ladyzhenskaya model (65) • ν ( | D | 2 ) ց as | D | 2 ր • (Smagorinskii turbulence model: r = 3 ) • shear thinning fluid ( r < 2 ) (A) given r ∈ (1 , ∞ ) there are C 1 > 0 and C 2 > 0 such that for all symmetric matrices B , D � � ( ν ( | D | 2 ) D ∂ r − 2 r − 2 2 | B | 2 ≤ C 1 ( K + | D | 2 ) · ( B ⊗ B ) ≤ C 2 ( K + | D | 2 ) 2 | B | 2 ∂ D K can be even 0 in many cases. – Typeset by Foil T EX – 5
Four approaches used in the analysis r ≥ 2 + 1 r > 2 − 1 • Higher regularity method = ⇒ regularity for 5 , but gives existence for 5 • Monotone operator theory - Test by u n − u r ≥ 2 + 1 k ∂ k v n ( v n − v ) ∈ L 1 ( Q ) ⇐ v n ⇒ O.A. Ladyzhenskaya ’65, J.L. Lions ’69 5 • L ∞ - truncation of Sobolev functions - Test by ( v n − v )(1 − min(1 , | v n − v | )) λ r > 2 − 2 k ∂ k v n ∈ L 1 ( Q ) ⇐ v n ⇒ J. Frehse , J. M´ alek, M. Steinhauer ’00, Wolf ’07 5 • W 1 , ∞ - truncation of Sobolev functions - Test by ( v n − v ) λ r ≥ 2 − 4 v n ⊗ v n ∈ L 1 ( Q ) ⇐ ⇒ Conjecture based on J. Frehse , J. M´ alek, M. Steinhauer ’03 5 L. Diening, M. R˚ uˇ ziˇ cka, J. W¨ olf ’07 – Typeset by Foil T EX – 6
Lemma on Lipschitz approximations of Sobolev functions Lemma for one function : Let Ω smooth, bounded and u ∈ W 1 , 1 (Ω) . 0 Then for every λ > 0 , θ > 0 there is u θ,λ ∈ W 1 , ∞ (Ω) : 0 • � u θ,λ � ∞ ≤ θ , • �∇ u θ,λ � ∞ ≤ cλ , • { u � = u θ,λ } ⊂ Ω ∩ ( { M ( u ) > θ } ∪ { M ( |∇ u | ) > λ ) } alek, Steinhauer ) Let Ω ∈ C 0 , 1 and u n → 0 in W 1 ,r (Ω) . Lemma ( Diening, M´ 0 Denote K := sup n � u n � 1 ,r and γ n := � u n � r → 0 and µ j := 2 2 j . Set θ n := √ γ n . Then there are λ n,j ∈ [ µ j , µ j +1 ] • � u n,j � ∞ ≤ θ n and �∇ u n,j � ∞ ≤ Cλ n,j • u n,j → 0 strongly in L ∞ (Ω) • u n,j ⇀ 0 weakly in W 1 ,s 0 (Ω) s ∈ � 1 , ∞ ) Evenmore, for all n, j • �∇ u n,j χ { un,j � = un } � r ≤ c � λ n,j χ { un,j � = un } � r ≤ c γn 1 θn µ j +1 + cK 2 j/r – Typeset by Foil T EX – 7
Fluids with pressure dependent viscosities S = ν ( p ) D ( v ) ν ( p ) = exp( γp ) Bridgman(31): ”The physics of high pressure” Cutler, McMickle, Webb and Schiessler(58) Johnson, Cameron(67), Johnson, Greenwood(77), Johnson, Tewaarwerk(80) Paluch et al. (99), Bendler et al. (01) elastohydrodynamics: Szeri(98) synovial fluids No global existence result. • Renardy(86), local, ( ν ( p ) → 0 as p → ∞ ) p • Gazzola(97), Gazzola, Secchi(98): local, severe restrictions – Typeset by Foil T EX – 8
∂ t v + P div( v ⊗ v ) − P div( ν ( p ) D ( v )) = 0 p = ( − ∆) − 1 div div( v ⊗ v − ν ( p ) D ( v )) F := ( − ∆) − 1 div div (a Fourier multiplier) Minimal requirement: v �→ p = p ( v ) is well defined.Let p 1 , p 2 be two solutions corresponding to v . p 1 − p 2 = F (( ν ( p 2 ) − ν ( p 1 )) D ( v )) = F ( ∂ p ν ( p 2 + θ 1 ( p 2 − p 1 )) D ( v ) ( p 2 − p 1 )) Not clear which side contains the leading operator. A very complex relation. ν ( p, | D ( v ) | 2 ) �� � ν ( p 1 , | D ( v ) | 2 ) − ν ( p 2 , | D ( v ) | 2 ) � p 1 − p 2 = −F D ( v ) = F ( ∂ p ν ( p 2 + θ 1 ( p 2 − p 1 ) , | D ( v ) | 2 ) D ( v ) ( p 2 − p 1 )) � p 1 − p 2 � q ≤ � ∂ p ν ( p 2 + θ 1 ( p 2 − p 1 ) , | D ( v ) | 2 ) D ( v ) ( p 1 − p 2 ) � q | ∂ p ν ( p, | D | 2 ) D | � p 1 − p 2 � q ≤ sup p, D ν ( p, | D | 2 ) = ln(1 + | p | + | D | ) – Typeset by Foil T EX – 9
Fluids with shear rate and pressure dependent viscosities S = ν ( p, | D | 2 ) D ( v ) η 0 − η ∞ ν ( p, | D | 2 ) = ( η ∞ + 1 + δ | D | 2 − r ) exp( γ p ) r = 1 . 56 Davies and Li(94), Gwynllyw, Davies and Phillips(96) p ν ( p, | D | 2 ) = c 0 r = 1 Schaeffer(87) - instabilities in granular materials | D | r − 2 ν ( p, | D | 2 ) = ( A + (1 + exp( α p )) − q + | D | 2 ) 2 0 ≤ q ≤ 1 r − 1 2 − rA (2 − r ) / 2 α > 0 , A > 0 1 ≤ r < 2 2 α elastohydrodynamics, synovial fluids, film flows, granular materials – Typeset by Foil T EX – 10
S = ν ( p, | D ( v ) | 2 ) D ( v ) Assumptions on (A1) given r ∈ (1 , 2) there are C 1 > 0 and C 2 > 0 such that for all symmetric matrices B , D and all p � � ν ( p, | D | 2 ) D ∂ r − 2 r − 2 2 | B | 2 ≤ C 1 (1 + | D | 2 ) · ( B ⊗ B ) ≤ C 2 (1 + | D | 2 ) 2 | B | 2 ∂ D (A2) for all symmetric matrices D and all p � ∂ [ ν ( p, | D | 2 ) D ] � r − 2 1 C 1 � � � ≤ γ 0 (1 + | D | 2 ) 4 ≤ γ 0 γ 0 < . � � � ∂p � C div, 2 C 1 + C 2 � – Typeset by Foil T EX – 11
Examples of ν ’s fulfilling (A1) and (A2) Consider r − 2 ν i ( p, | D | 2 ) = ( µ i ( p ) + | D | 2 ) 2 i = 1 , 2 , 3 − q µ 1 ( p ) = A + (1 + α 2 p 2 ) 2 µ 2 ( p ) = A + (1 + exp( αp )) − q � A + exp( − αq P )) if p > 0 , µ 3 ( p ) = A + 1 if p ≤ 0 . with α | q | (2 − r ) ≤ r − 1 α > 0 , A > 0 , q > 0 , r ∈ (9 / 5 , 2) and 4 ν i ( · , | D | 2 ) is increasing in the first variable for any fixed D These models are pressure thickening and shear thinning which is in agreement with experimental observations. These models fulfil the assumptions (A1) – (A2) . – Typeset by Foil T EX – 12
Result #2 Theorem 2. ( M. Bul´ ıˇ cek , J. M´ alek, K. R. Rajagopal ’07) Let (A1) – (A2) hold and r in (A1) satisfy � 8 � r ∈ 5 , 2 Assume that • ∂ Ω ∈ C 1 , 1 • v 0 ∈ L 2 n ,div • g ∈ L 1 (0 , T ) Then for all T > 0 (and any α ∈ (0 , 1] ) there exists at least one weak solution ( v , p ) of the system (*) completed by Navier’s slip boundary conditions such that v ∈ C (0 , T ; L 2 weak ) ∩ L r (0 , T ; W 1 ,r n ,div ) 5 r 5 r � 6 (0 , T ; L 6 ) p ∈ L and p ( t, x ) dx = g ( t ) Ω If r ∈ (9 / 5 , 2) , the existence of suitable weak solution can be established. – Typeset by Foil T EX – 13
Recommend
More recommend