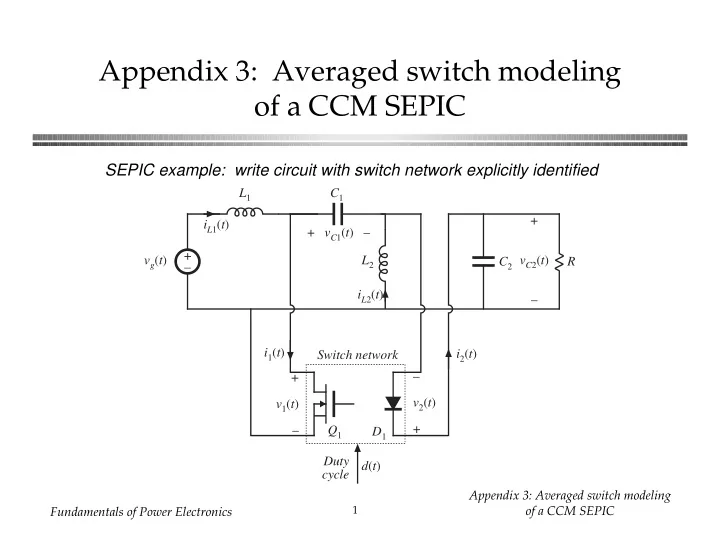
Appendix 3: Averaged switch modeling of a CCM SEPIC SEPIC example: - PowerPoint PPT Presentation

Appendix 3: Averaged switch modeling of a CCM SEPIC SEPIC example: write circuit with switch network explicitly identified L 1 C 1 + i L 1 ( t ) + v C 1 ( t ) – + v g ( t ) L 2 v C 2 ( t ) C 2 R – i L 2 ( t ) – i 1 ( t ) i 2 ( t ) Switch network – + v 2 ( t ) v 1 ( t ) + – Q 1 D 1 Duty d ( t ) cycle Appendix 3: Averaged switch modeling 1 of a CCM SEPIC Fundamentals of Power Electronics

A few points regarding averaged switch modeling • The switch network can be defined arbitrarily, as long as its terminal voltages and currents are independent, and the switch network contains no reactive elements. • It is not necessary that some of the switch network terminal quantities coincide with inductor currents or capacitor voltages of the converter, or be nonpulsating. • The object is simply to write the averaged equations of the switch network; i.e., to express the average values of half of the switch network terminal waveforms as functions of the average values of the remaining switch network terminal waveforms, and the control input. Appendix 3: Averaged switch modeling 2 of a CCM SEPIC Fundamentals of Power Electronics

SEPIC CCM waveforms Sketch terminal waveforms of switch network Port 1 Port 2 v 1 ( t ) v 2 ( t ) v C 1 + v C 2 v C 1 + v C 2 v 1 ( t ) T s v 2 ( t ) T 2 0 0 0 0 0 0 dT s T s dT s T s t t i 2 ( t ) i 1 ( t ) i L 1 + i L 2 i L 1 + i L 2 i 2 ( t ) T s i 1 ( t ) T 2 0 0 0 0 0 0 dT s T s t dT s T s t Appendix 3: Averaged switch modeling 3 of a CCM SEPIC Fundamentals of Power Electronics

Expressions for average values of switch network terminal waveforms Use small ripple approximation v 1 ( t ) T s = d '( t ) v C 1 ( t ) T s + v C 2 ( t ) T s i 1 ( t ) T s = d ( t ) i L 1 ( t ) T s + i L 2 ( t ) T s v 2 ( t ) T s = d ( t ) v C 1 ( t ) T s + v C 2 ( t ) T s i 2 ( t ) T s = d '( t ) i L 1 ( t ) T s + i L 2 ( t ) T s Need next to eliminate the capacitor voltages and inductor currents from these expressions, to write the equations of the switch network. Appendix 3: Averaged switch modeling 4 of a CCM SEPIC Fundamentals of Power Electronics

Derivation of switch network equations (Algebra steps) We can write Result i 1 ( t ) T s i L 1 ( t ) T s + i L 2 ( t ) T s = d ( t ) 〈 i 1 ( t ) 〉 T s + – v 2 ( t ) d '( t ) d '( t ) 〈 v 1 ( t ) 〉 T s + 〈 v 2 ( t ) 〉 T s T s v 2 ( t ) T s i 1 ( t ) T s v C 1 ( t ) T s + v C 2 ( t ) T s = d ( t ) – d ( t ) d ( t ) 〈 i 2 ( t ) 〉 T s – + Hence Averaged switch network T s = d '( t ) v 1 ( t ) v 2 ( t ) d ( t ) T s Modeling the switch network via averaged dependent sources T s = d '( t ) i 2 ( t ) i 1 ( t ) d ( t ) T s Appendix 3: Averaged switch modeling 5 of a CCM SEPIC Fundamentals of Power Electronics

Steady-state switch model: Dc transformer model I 1 D' : D + – V 1 V 2 I 2 – + Appendix 3: Averaged switch modeling 6 of a CCM SEPIC Fundamentals of Power Electronics

Steady-state CCM SEPIC model Replace switch network with dc transformer model L 1 C 1 + I L 1 + V C 1 – + L 2 V C 2 V g C 2 R – I L 2 – Can now let inductors I 1 D' : D become short circuits, + – capacitors become open circuits, and solve for dc V 1 V 2 conditions. I 2 – + Appendix 3: Averaged switch modeling 7 of a CCM SEPIC Fundamentals of Power Electronics

Small-signal model d ( t ) = D + d ( t ) Perturb and linearize the switch network averaged waveforms, v 1 ( t ) T s = V 1 + v 1 ( t ) as usual: i 1 ( t ) T s = I 1 + i 1 ( t ) v 2 ( t ) T s = V 2 + v 2 ( t ) i 2 ( t ) T s = I 2 + i 2 ( t ) Voltage equation becomes D + d V 1 + v 1 = D ' – d V 2 + v 2 D V 2 + v 2 – d V 1 + V 2 Eliminate nonlinear terms V 1 + v 1 = D ' D and solve for v 1 terms: V 1 = D ' D V 2 + v 2 – d DD ' Appendix 3: Averaged switch modeling 8 of a CCM SEPIC Fundamentals of Power Electronics

Linearization, continued Current equation becomes D + d I 2 + i 2 = D ' – d I 1 + i 1 Eliminate nonlinear terms and solve for i 2 terms: D I 1 + i 1 – d I 1 + I 2 I 2 + i 2 = D ' D I 2 = D ' D I 1 + i 1 – d DD ' Appendix 3: Averaged switch modeling 9 of a CCM SEPIC Fundamentals of Power Electronics

Switch network: Small-signal ac model Reconstruct equivalent circuit in the usual manner: I 1 + i 1 D' : D + – – + V 1 DD ' d I 2 V 1 + v 1 V 2 + v 2 DD ' d + – I 2 + i 2 Appendix 3: Averaged switch modeling 10 of a CCM SEPIC Fundamentals of Power Electronics

Small-signal ac model of the CCM SEPIC Replace switch network with small-signal ac model: L 1 C 1 + I L 1 + i L 1 V C 1 + v C 1 + L 2 C 2 V C 2 + v C 2 V g + v g R – I L 2 + i L 2 – D' : D + – Can now solve this V 1 model to determine DD ' d I 2 ac transfer functions DD ' d Appendix 3: Averaged switch modeling 11 of a CCM SEPIC Fundamentals of Power Electronics