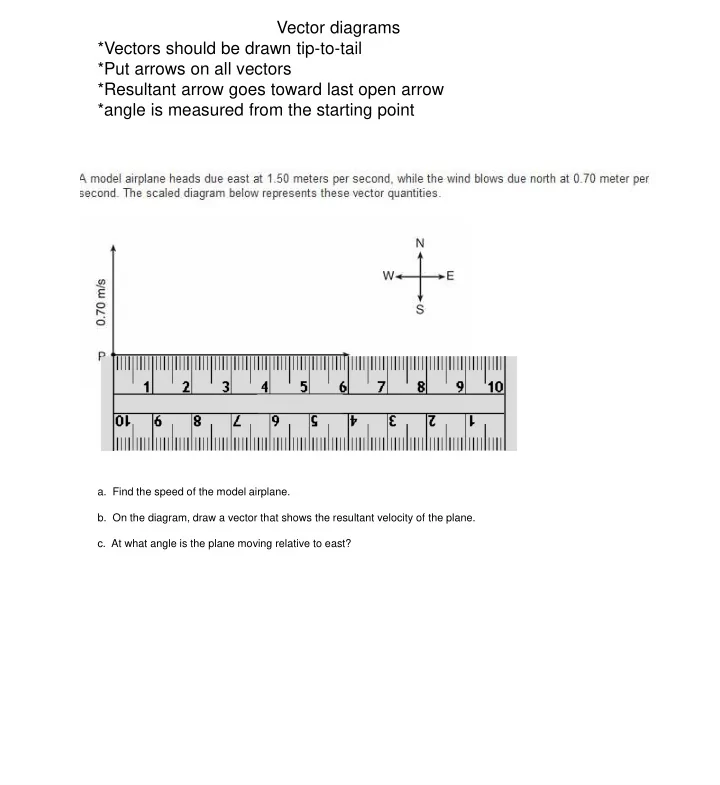
Vector diagrams *Vectors should be drawn tip-to-tail *Put arrows on - PDF document

Vector diagrams *Vectors should be drawn tip-to-tail *Put arrows on all vectors *Resultant arrow goes toward last open arrow *angle is measured from the starting point a. Find the speed of the model airplane. b. On the diagram, draw a vector that shows the resultant velocity of the plane. c. At what angle is the plane moving relative to east?

Vector diagrams *Vectors should be drawn tip-to-tail *Put arrows on all vectors *Resultant arrow goes toward last open arrow *angle is measured from the starting point 25 0 scale: 1.5 m/s = 6 cm so 1 cm =0.25 m/s resultant is ≈ 6.5 cm = 1.63 m/s Using pythagoreant theorem: (1.5 m/s) 2 + (.7 m/s) 2 = R 2 R = 1.66 m/s a. Find the speed of the model airplane. 1.6 m/s to 1.7 m/s b. On the diagram, draw a vector that shows the resultant velocity of the plane. 6.3 cm to 6.7 cm @ 23 to 27 degrees c. At what angle is the plane moving relative to east? 25 ± 20

Motion graphs slope a d v area a.Find speed at 1.0 sec. b. Find acceleration at 7 sec. c. Find distance traveled from 6 - 8 sec. uniform acceleration shapes Constant speed shapes

Motion graphs slope a d v area 5 m/s a.Find speed at 1.0 sec. 5 m b. Find acceleration at 7 sec. slope = 15-10 m/s =2.5 m/s2 20 m 8 - 6 s c. Find distance traveled from 6 - 8 sec. area = 20 + 5 = 25 m uniform acceleration shapes Constant speed shapes

Projectile Motion *The horizontal motion is constant speed *The vertical motion is accelerated at 9.8m/s2 *Complementary angles have same range *Most time and height-- closest to 900 *Farthest range -- closest to 450 a. Find the horizontal component of the initial velocity. b. Find the vertical component of the initial velocity. c. What is the acceleration of the ball at its highest point? d. What is the speed of the ball at its highest point? e. What other angle would go the same horizontal distance if projected at 25 m/s? f. Draw the trajectory of a soccer ball kicked at 25 m/s and an angle of 45 degrees on the diagram.

Projectile Motion *The horizontal motion is constant speed *The vertical motion is accelerated at 9.8m/s2 *Complementary angles have same range *Most time and height-- closest to 900 *Farthest range -- closest to 450 a. Find the horizontal component of the initial velocity. Ax = A cosθ = 25m/s(cos 40 0 ) = 19 m/s b. Find the vertical component of the initial velocity. Ay = A sin θ = 25 m/s(sin 40 0 ) = 16 m/s c. What is the acceleration of the ball at its highest point? 9.8 m/s 2 d. What is the speed of the ball at its highest point? 19 m/s e. What other angle would go the same horizontal distance if projected at 25 m/s? 50 degrees f. Draw the trajectory of a soccer ball kicked at 25 m/s and an angle of 45 degrees on the diagram.

Freebody Diagrams *Only draw forces on the diagram and NOT the net force *Weight is the only force that MUST be in FBD *Normal force if touching a solid surface, Friction if not frictionless a.Draw FBD. b . c . d. What is the net force acting on the sled?

Freebody Diagrams *Only draw forces on the diagram and NOT the net force *Weight is the only force that MUST be in FBD *Normal force if touching a solid surface, Friction if not frictionless F F N F f Fg a.Draw FBD. b . Ax = A cosθ = 60N(cos 30 0 ) = 52 N c . 52 N d. What is the net force acting on the sled? zero. There is no net force.

*Net forces cause masses to accelerate in the direction of the NET force. a = Fnet m An ice skater applies a horizontal force to a 20.-kilogram block on frictionless, level ice, causing the block to accelerate uniformly at 1.4 meters/second squared to the right. After the skater stops pushing the block, it slides onto a region of ice that is covered with a thin layer of sand. The coefficient of kinetic friction between the block and the sand-covered ice is 0.28. a. On the diagram below, starting at point A , draw a vector to represent the force applied to the block by the skater. Begin the vector at point A and use a scale of 1.0 centimeters = 5.0 newtons. b. Calculate the magnitude of the force of friction acting on the block as it slides over the sand-covered ice. [Show all work, including the equation and substitution with units.]

*Net forces cause masses to accelerate in the direction of the NET force. a = Fnet m An ice skater applies a horizontal force to a 20.-kilogram block on frictionless, level ice, causing the block to accelerate uniformly at 1.4 meters/second squared to the right. After the skater stops pushing the block, it slides onto a region of ice that is covered with a thin layer of sand. The coefficient of kinetic friction between the block and the sand-covered ice is 0.28. a. On the diagram below, starting at point A , draw a vector to represent the force applied to the block by the skater. Begin the vector at point A and use a scale of 1.0 centimeters = 5.0 newtons. Since there's no friction on the ice 28N/5N/cm =5.6 cm skater, then the net force will be the applied force acting on the skater. 28 N a = Fnet/m 1.4 m/s 2 = F/20kg F = 28 N b. Calculate the magnitude of the force of friction acting on the block as it slides over the sand-covered ice. [Show all work, including the equation and substitution with units.] Ff = μFN = .28(20kg x 9.8m/s 2 ) = 55 N

Inclined Plane *Force of gravity is broken into components *Normal force is perpendicular to surface a. Draw a FBD labeling all forces. b. Determine the force of friction. c. Determine the normal force.

Inclined Plane *Force of gravity is broken into components *Normal force is perpendicular to surface F F a. Draw a FBD labeling all forces. N f b. Determine the force of friction. F f = Fgsinθ= 98N sin20 0 = 33.5 N Fg c. Determine the normal force. F N = Fgcosθ= 98N cos20 0 = 92.1 N

Circular Motion *Net Force and acceleration toward center *velocity is tangent to circle a. Calculate the centripetal force acting on the car. b. How long does it take the car to go around the track? c. Draw acceleration and velocity vectors on the cart at the position shown.

Circular Motion *Net Force and acceleration toward center *velocity is tangent to circle a. Calculate the centripetal force acting on the car. v Fc = mac = mv 2 /r a = 1.5 kg(4m/s) 2 2.4 m = 10 N b. How long does it take the car to go around the track? v = d/t = 2 π r/t = 2(3.14)(2.4m) = 4 m/s t= 3.77 s t c. Draw acceleration and velocity vectors on the cart at the position shown.

Work and Energy *Work equals change in energy *Work requires force and motion *Work is never done in uniform circular motion a. b. How much total energy does the system have at A. c. Find the speed of the rollercoaster at B. d. How does the KE at C compare to the KE at B?

Work and Energy *Work equals change in energy *Work requires force and motion *Work is never done in uniform circular motion a. The total mechanical energy is the same. b. How much total energy does the system have at A. PE = mgh = (250kg +75 kg)(9.8m/s 2 )(20m) = 63,700 J = 6.4 x10 4 J c. Find the speed of the rollercoaster at B. PE top = KE bottom = 6.4 x104 J = 1/2mv 2 =1/2(325kg)v 2 v = 20. m/s d. How does the KE at C compare to the KE at B? The KE at C is less than at B.

Springs *Use F=kx when a force is given or a weight is hung on a spring. *Use PEs=1/2kx2 when speed or height is given since it implies conservation of energy is being used.

Springs *Use F=kx when a force is given or a weight is hung on a spring. *Use PEs=1/2kx2 when speed or height is given since it implies conservation of energy is being used. Fs= kx 6N = k(.04m) k =150 N/m

a . b .

a . PEs = 1/2 kx 2 PE = 0.19 J PE = 1/2(150 N/m)(.05 m) 2 b . PEs = PE g = mgh h = 0.96 m 0.19 J = 0.02 kg(9.8m/s 2 )(h)

Momentum *Momentum is a VECTOR *Impulse = Change in momentum *LAW of Conservation of Momentum 1. After Before 5 kg 5 kg 15 m/s 10 m/s 20 m/s 10 m/s B B A A 2. 3 .

Momentum *Momentum is a VECTOR *Impulse = Change in momentum *LAW of Conservation of Momentum 1. After p = mv Before 5 kg 5 kg 15 m/s 10 m/s 20 m/s 10 m/s B B A A + B15 kgm/s = 50 kgm/s + B10 kgm/s = 100 kgm/s B = 10 kg 100 + 10B = 50 + 15 B 2. = - 6 kgm/s - 18 kgm/s + 12 kgm/s magnitude = 6 kgm/s total p = 0 3 . -1.2kg v + 1.8kg(2m/s) =0 v = 3.0 m/s

Electrostatics *Only electrons can move to give a charge *Electric field lines point away from positive charges toward negative charges a. Find force between the charges. b. c.

Electrostatics *Only electrons can move to give a charge *Electric field lines point away from positive charges toward negative charges a. Find force between the charges. Fe = kq 1 q 2 /r 2 = 8.99 x 10 9 N-m 2 /kg 2 (8x10 -19 C)(4.8x10 -19 C/(1.2x10 -4 m) 2 Fe = 2.4 x 10 -19 N b. c. inverse square law

Series Circuits *Everything gets the same current *Total current depends on total resistance *One device goes out, all go out a. Find resistor, R. b.F ind potential difference across 50 ohm. c. Find total energy in one minute for the circuit. d. If another resistor is added in series, what happens to the ammeter reading?