Types of problems Given a combinatorial object what are the - PDF document

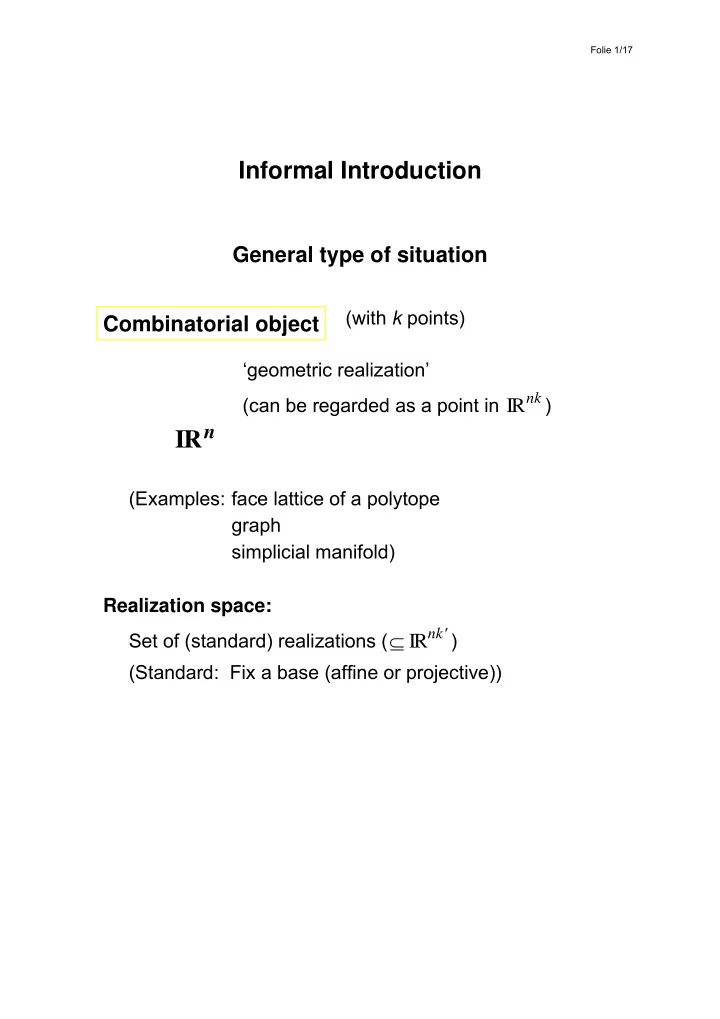
Folie 1/17 Informal Introduction General type of situation (with k points) Combinatorial object ‘geometric realization’ (can be regarded as a point in IR nk ) IR n (Examples: face lattice of a polytope graph simplicial manifold) Realization space: IR nk ) ′ Set of (standard) realizations ( ⊆ (Standard: Fix a base (affine or projective))

Folie 2/17 Types of problems – Given a combinatorial object what are the properties of the realization space? such as ≠ ∅ ? (existence), connected? (isotopy problem) – Characterize (up to stable equivalence, …) the subsets of IR nk ′ which occur as realization spaces of combinatorial objects of the type under consideration!

Folie 3/17 Example. 1 2 3 1 6 7 4 4 A map on the torus 8 5 5 9 1 3 1 2

Folie 4/17 Let f be an injective mapping of the set of vertices of K into IR 3 and P ∈ K a polygon The image of P is the closed polygon arising from connecting the images of the vertices by straight line segments corresponding to the edges of P . The relative interior of a simple planar polygon (i.e. without self-intersections) is the bounded open planar region bounded by the polygon. A polyhedral embedding of K is an injective mapping f from the set of vertices of K into IR 3 , such that 1. The image of each polygon P ∈ K is a strictly convex polygon; 2. the relative interiors of the images of the elements of K are pairwise disjoint. f a f ⊆ IR 3 denotes the union of these images. K

Folie 5/17 k p be the set of vertices of K , given in Let v … … , , , w , , w n v 1 5 1 a fixed order. A polyhedral embedding of K is called standard if = F G I = F G I = F G I 0 J , f ( J , J , f ( 1 0 H K H K f ( ) H K ) ) 0 0 1 v 1 v 2 v 3 0 0 0 = F G I = F G I 0 1 J , f ( J . f ( ) ) H 0 K H 1 K v 4 v 5 1 1 The realization space of K is k ( ( ∈ IR 3 n … f is a polyhedral standard f f w ), , ( w n )) 1 embedding of K. p

Folie 6/17 Semialgebraic sets Definition: A (primary) semialgebraic set P ⊆ IR n is a finite intersection of sets of the form k p IR n f ∈ = < 0 , x ( ) x 0 , g ( ) x ∈ = … … where f g I . , Q , , , ( , , ) x x x x x 1 n 1 n Structure of the realization space Proposition 1. The realization space of an abstract polyhedral complex is a semialgebraic set.

Folie 7/17 The Universality Theorem Theorem: ≥ 0 and G a graph with vertex set Let n k , k p . … … v , , , w , , w n v 1 5 1 ⊆ IR n 3 Let be a semialgebraic set. P Then there exists a map M which contains only triangles and quadrangles and which contains G as an induced graph, ⊆ such that for each subfield and each standard K IR : G → K 3 embedding the following are equivalent: f i) f can be extended to a polyhedral embedding M → K 3 . f : i') f can be extended to an nc-embedding M → K 3 . f : ∈ P … ii) . f f ( ( w ), , ( w )) 1 n

Folie 8/17 Corollaries 1. Let L be a strict subfield of the field of real algebraic numbers. Then there is a map M which can be polyhedrally embedded in IR 3 but not in L 3 . 2. The realizability problem for maps in IR 3 is polynomial- time equivalent to the ‘Existential Theory of the Reals’ and thus NP-hard.

Folie 9/17 Steps for the proof: 1. Encoding the semialgebraic set by collinearities and edges 2. Encoding the collinearities by standard handles 3. Encoding the semialgebraic set by an admissible polyhedral complex 4. Universal extension of the polyhedral complex to a map

Folie 10/17 Step 1 Encoding the semialgebraic set by collinearities and edges Proposition 2. ⊆ IR n 3 Let be a semialgebraic set. P Then there are sets { } = … … V , , , w , , w v v 1 5 1 n ⊇ U V ( ) U V \ ⊆ E 2 ( ) , U C ⊆ 3 such that for each subfield K ⊆ IR and each standard : V → K 3 embedding the following are equivalent: f i) f can be extended to an embedding f of the graph 3 U E into K such that f z are collinear ( , ) f f ( ), ( ), ( ) x y k p ∈ for each x y z . , , C i') Same as i) such that in addition the image of the graph U V E does not meet a given compact subset of ( \ , ) IR 3 . ∈ P … ii) . f f (( w ), , ( w )) 1 n

Folie 11/17 Step 2 Encoding the collinearities by standard handles Definition: A standard handle is a map with boundary which is isomorphic to H , where 1 1 2 3 6 7 H : = 4 4 The quadrangle 6 7 9 8 is removed. 5 5 8 9 1 1 2 3 Lemma 1: a) For each polyhedral embedding of H the quadrangle 6 7 9 8 is planar and strictly convex. b) Each embedding of 6 7 9 8 as a strictly convex quadrangle can be extended to a polyhedral embed- ding of H within any arbitrary pyramid having the quadrangle as its base.

Folie 12/17 Lemma 2: There exists an admissible polyhedral complex K 0 with three vertices x y z , , , such that for each injective mapping k p → IR 3 are equivalent: f : x y z , , i) f can be extended to a polyhedral embedding f of K 0 ii) f z are collinear. f f ( ), ( ), ( ) x y Moreover: 1. All polygons in K 0 are quadrangles; z are collinear then for each ε > 0 and 2. if f f f ( ), ( ), ( ) x y each extension of f to an embedding g of the graph , the extension f can be chosen such that K 3 3 K 0 ⊆ U f ( ) ( ( g K )). ε 3 3 , g w ( ) g ( ) v f ( ) f ( ) f ( ) y z x g u ( )

Folie 13/17 Proof of Lemma 2: K 1 is composed from 8 standard handles which intersect only in common boundary edges. The boundary quadrangles are z g b f g b e f b x e f b x a e x c d a d a c h e d c h e d h y f e d a b c g h z y x The planarity of these quadrangles for a polyhedral embedding of K 1 (Lemma 1) implies that all the vertices … are coplanar: a , , , , , h x y z x c d a ⎫ c d a c d a h ⎪ c d h ⎪ c d h e d h e ⎪ ⎪ d h e y ⎬ not collinear. x a e x a e b ⎪ x e b ⎪ x e b f e b f ⎪ e b f g ⎪ ⎭ b f g b f g z

Folie 14/17 z y e f a d y f e b g c h d z a b g c h x x

Folie 15/17 Construction of K 0 K 0 consists of 3 copies of K 1 , which are disjoint except for the common vertices x y z , , . Since the graph K 3 3 , is not planar, at least two of the three planes are different and thus x y z , , are collinear.

Folie 16/17 Step 3 Encoding the semialgebraic set by an admissible polyhedral complex We begin with the sets W of vertices, E of edges constructed in the first step and encode each collinearity in C according to Lemma 2. Then we add the edges of G and additional ∗ pairs of edges with additional vertices ∗ such that the resulting polyhedral complex K is connected. K is admissible and orientable and all its polygons are quadrangles . Using Proposition 2 and Lemma 2 we get: K has all properties required for M in the universality theorem, except for the property of being a map.

Folie 17/17 Step 4 Universal extension of an admissible polyhedral complex to a map Universal extension Definition: Let L be an abstract polyhedral complex and K L ⊆ a subcomplex. L is called a universal extension of K if for each ε > 0 and each polyhedral embedding f : K → IR 3 there exists an extension of f to f : L → IR 3 such that i) f is a polyhedral embedding, b g is contained in the ε -neighbourhood of ii) the image f L b g , f K iii) the vertices of L − K I 3 . are in Q Proposition 3: Let K be a connected admissible abstract polyhedral complex. a) Then there exists a universal extension of K to a map M (with boundary). b) If K is orientable then there even exists a universal extension of K to a map M (without boundary). Moreover M can be chosen such that all new polygons are triangles.