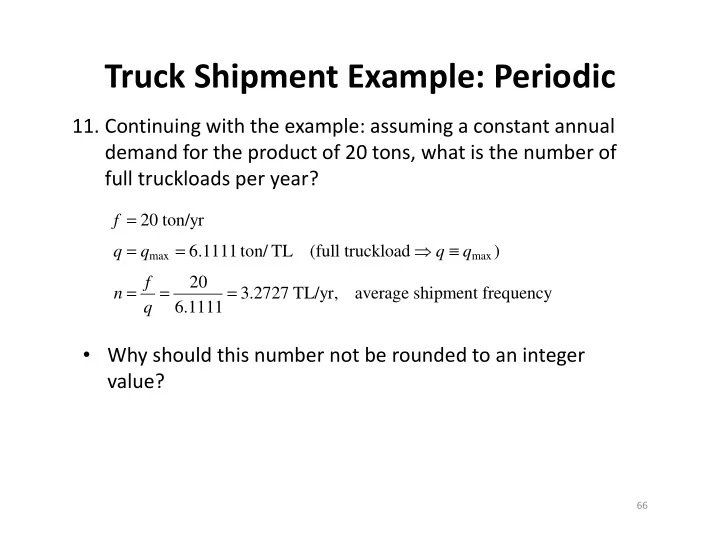
Truck Shipment Example: Periodic 11. Continuing with the example: - PowerPoint PPT Presentation

Truck Shipment Example: Periodic 11. Continuing with the example: assuming a constant annual demand for the product of 20 tons, what is the number of full truckloads per year? = f 20 ton/yr  = = ≡ q q 6.1111 ton/ TL (full truckload q q ) max max f 20 = = = 3.2727 TL/yr, average shipment frequency n 6.1111 q • Why should this number not be rounded to an integer value? 66

Truck Shipment Example: Periodic 12. What is the shipment interval? 1 6.1111 q = = = = 0.3056 yr/TL, average shipment interval t 20 n f • How many days are there between shipments? 365.25 day/yr 365.25 × = = t 365.25 111.6042 day/TL n 67

Truck Shipment Example: Periodic 13. What is the annual full-truckload transport cost? = = d 532 mi, r $2.5511/ mi TL 2.5511 r TL = = = r $0.4175 / ton-mi FTL 6.1111 q max ( ) = = = , = monetary weight in $/mi TC f r d nr d wd w FTL FTL TL = 3.2727(2.5511)532 = $4,441.73/yr • What would be the cost if the shipments were to be made at least every three months? 3 1 f   = yr/TL = = 4 TL/yr = t n q max min { } 12 max , t n n max min { } ′ = max , TC n n r d FTL min TL { } = max 3.2727, 4 2.5511(532) = $5,428.78/yr 68

Truck Shipment Example: Periodic • Independent and allocated full-truckload charges:  [ ] [ ] ≤ , = c ( ), q q UB LB q qr d max 0 FTL Transport Charge for a Shipment 3 TL 4072 2 TL 2714 1 TL 1357 Transport Charge ($) MC 87.51 150/2000 0.7960 6.11 12.22 69 Shipment Size (tons)

Truck Shipment Example: Periodic • Total Logistics Cost (TLC) includes all costs that could change as a result of a logistics-related decision = + + TLC TC IC PC = transport cost TC = inventory cost IC = + + IC IC IC cycle pipeline safety = PC purchase cost • Cycle inventory : held to allow cheaper large shipments • Pipeline inventory : goods in transit or awaiting transshipment • Safety stock : held due to transport uncertainty • Purchase cost : can be different for different suppliers 70

Truck Shipment Example: Periodic • Same units of inventory can serve multiple roles at each position in a production process • Working stock : held as part of production process • (in-process, pipeline, in-transit, presentation) • Economic stock: held to allow cheaper production • (cycle, anticipation) • Safety stock: held to buffer effects of uncertainty • (decoupling, MRO (maintenance, repair, and operations) ) 71

Truck Shipment Example: Periodic 14. Since demand is constant throughout the year, one half of a shipment is stored at the destination, on average. Assuming that the production rate is also constant, one half of a shipment will also be stored at the origin, on average. Assuming each ton of the product is valued at $25,000, what is a “reasonable estimate” for the total annual cost for this cycle inventory? = (annualcost of holding one ton)(average annual inventory level) IC cycle = ( )( α ) vh q = unit value of shipment ($/ton) v = inventory carrying rate, the cost per dollar of inventory per year (1/yr) h α = average int er-shipment inventory fraction at Origin and Destination q = shipment size (ton) 72

Truck Shipment Example: Periodic • Inv. Carrying Rate ( h ) = interest + warehousing + obsolescence • Interest: 5% per Total U.S. Logistics Costs • Warehousing: 6% per Total U.S. Logistics Costs • Obsolescence: default rate ( yr ) h = 0.3  h obs ≈ 0.2 (mfg product) – Low FGI cost ( yr ): h = h int + h wh + h obs – High FGI cost ( hr ): h ≈ h obs , can ignore interest & warehousing • ( h int + h wh )/ H = (0.05+0.06)/2000 = 0.000055 ( H = oper. hr/yr) – Estimate h obs using “percent-reduction interval” method: given time t h when product loses x h -percent of its original value v , find h obs x x   h h = = = , and = h t v x v h t x h t obs h h obs h h obs h t h obs h – Example: If a product loses 80% of its value after 2 hours 40 minutes: 40 0.8 x  h = + = = = = 2 2.67 hr 0.3 t h h 60 2.67 t h – Important: t h should be in same time units as production time, t CT 73

Truck Shipment Example: Periodic   1 1 q q  • Avg. annual cycle inventory level = + =  +  = (1) α = 1 q q   2 2 2 2 q q q q 2 2 0 0 • Note: Cycle inventory is FGI at Origin and RMI at Destination 74

Truck Shipment Example: Periodic • Inter-shipment inventory fraction alternatives: α = α + α O D q q q 2 2 2 ≈ 0 1 1 1 1 α = + = α = + = 1 0 2 2 2 2 q 2 ≈ 0 ≈ 0 ≈ 0 1 1 α = 0 + 0 = 0 α = 0 + = 2 2 75

Truck Shipment Example: Periodic • “Reasonable estimate” for the total annual cost for the cycle inventory: = α IC vhq cycle = (1)(25,000)(0.3)6.1111 = $45,833.33/ yr where 1 1 α = at Origin + at Destination = 1 2 2 = $25,000 = unit value of shipment ($/ton) v = 0.3 = estimated carrying rate for manufactured products (1/yr) h = = 6. 111 = FTL shipment size (ton) q q max 76

Truck Shipment Example: Periodic 15. What is the annual total logistics cost (TLC) for these (necessarily P2P) full-truckload TL shipments? = + TLC TC IC FTL FTL cycle = + α nr d vhq TL = 3.2727(2.5511)532 + (1)(25,000)(0.3)6.1111 = 4,441.73 + 45,833.33 = $50,275.06 / yr • Problem: FTL may not minimize TLC  Can assume, for any periodic shipment, q ≤ q max  Assuming P2P TL, what to find q , q * , that minimizes TLC   q  ( ) = = c q   r d r d TL TL TL   q max 77

Truck Shipment Example: Periodic 16. What is minimum possible annual total logistics cost for P2P TL shipments, where the shipment size can now be less than a full truckload? f f = + = + α = + α TLC ( ) q TC ( ) q IC q ( ) c ( ) q vhq rd vhq TL TL TL q q ( ) 20(2.5511)532 dTLC q f r d  TL * TL = 0 = = = 1.9024 ton q TL α (1)25000(0.3) dq vh f * * ( ) = + α TLC q r d vhq TL TL TL TL * q TL 20 = (2.5511)532 + (1)25000(0.3)1.8553 1.8553 = 14,268.12 14,268.12 + = $28,536.25 / yr 78

Truck Shipment Example: Periodic • Including the minimum charge and maximum payload restrictions:   { }   max , f r d MC f r d TL TL * TL   = min , ≈ q q max TL α α vh vh     • What is the TLC if this size shipment could be made as a (not-necessarily P2P) allocated full-truckload? f r ( ) * * * * TL ( ) = + α = + α TLC q q r d vhq f d vhq AllocFTL TL TL FTL TL TL * q q max TL 2.5511 = + 20 532 (1)25000(0.3)1.9024 6.1111 = 4,441.73 14,268.12 + ( ) = $18,709.85 / yr vs. $28,536.25 as independent P2P TL 79

Truck Shipment Example: Periodic 17. What is the optimal LTL shipment size? f ( ) = ( ) + ( ) = ( ) + α TLC q TC q IC q c q vhq LTL LTL LTL q * = arg min ( ) = 0.7622 ton q TLC q LTL LTL q • Must be careful in picking starting point for optimization since LTL formula only valid for limited range of values:  37 ≤ ≤ 3354 (dist) d   2 s    + 14 150 10,000 (wt)  ≤ ≤ q 8    = , r PPI 2,000 2,000   LTL LTL  1 15   7 ( )   2 q 7 29  − + 2 + 14  q d s s  3 2000 ≤ 650 ft (cube)     2  s   150 10,000 650 s    ≤ ≤ min , 0.075 ≤ ≤ 1.44 q q   2000 2,000 2000 80

Truck Shipment Example: Periodic 18. Should the product be shipped TL or LTL? * * * ( ) = ( ) + ( ) = 34,349.19 + 5,716.40 = $40,065.59 / yr TLC q TC q IC q LTL LTL LTL LTL LTL TLC TL TLC LTL 40066 TC LTL 28536 TC TL IC 0.76 1.90 Shipment weight (tons) 81