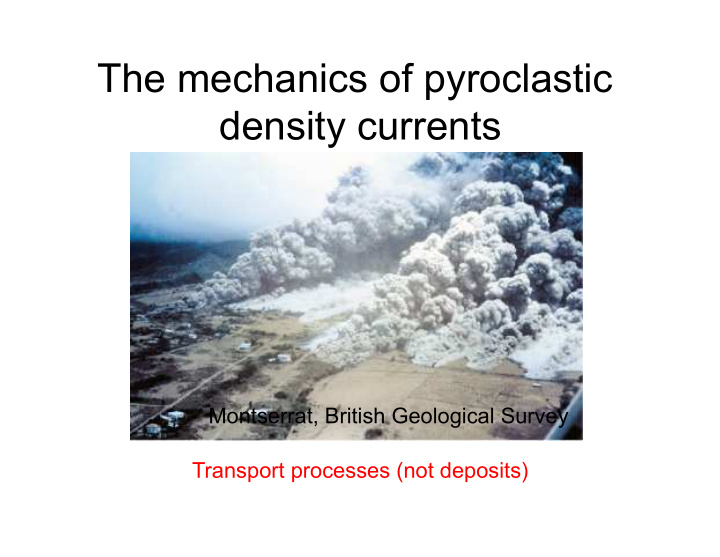
The mechanics of pyroclastic density currents Montserrat, British - PowerPoint PPT Presentation

The mechanics of pyroclastic density currents Montserrat, British Geological Survey Transport processes (not deposits)

The role of particle-scale processes on the large scale dynamics of pyroclastic density currents Michael Manga Interrupt and ask questions anytime

Mutiphase flows in explosive eruptions Plinian Column • � Buoyant plume • � Particle+Gas Flow Pyroclastic Flow • � Particulate gravity current • � Particle+Gas Flow • � Interaction with water Montserrat Univ West Indies St Helens D Swanson, USGS

Multiphase flows in explosive eruptions Montserrat, Univ West Indies • � Controls of particle size? • � How fast? • � How far? • � Internal structure? St Helens, USGS • � Connect processes to deposits

Challenge • � Wide range of length and time scales • � Many critical processes occur at the scale of particles • � How to integrate the micro- and macro-scale mass, momentum and heat transfer?

Challenge • � Wide range of length and time scales • � Many critical processes occur at the scale of particles • � How to integrate the micro- and macro-scale mass, momentum and heat transfer?

Multiple levels of coupling between discrete and continuous phases

Multiple levels of coupling between discrete and continuous phases Prolonged Frictional Contact

Multiple levels of coupling between discrete and continuous phases Instantaneous Collisions

Multiple levels of coupling between discrete and continuous phases St= � p / � f

Mean field multifluid equations Continuity � ) + � ( ( ) = 0 1 � 1 � 1 � 1 � 1 u i � t � x Momentum + � ( 1 � 1 � 1 u i 1 u j ) � ( 1 � 1 � 1 u i ) = � t � x i � � � � �� ( 1 P ) � � � � N ( � , e ) 1 � + 1 1 � � � 1 � ij � ( 1 u i � 2 u i ) + � � ˆ e g + � � � � � � p M 0 2 2 � x i � Re � � x i � St � Fr d � � � � Thermal Energy � 1 T � 1 T � 1 q � � 1 1 � � � � � t + 1 U i 2 T - 1 T ( ) 1 � 1 c p � = + � � � � � � 1 x i Th St � x i Pe � � � � � � Details of constitutive models, equations of state, turbulence models, in Dufek and Bergantz (2007) Key: determining closure models

Sub-grid scale thermo-mechanical processes 1) � Collisions between particles within pyroclastic flows (and in volcanic conduits in the afternoon) 2) � Role of boundary conditions (over water vs over land) 3) � Heat transfer from particles to water

1. How much of this ash is made WITHIN the flow? Augustine, 1986 Maurice and Katia Krafft Influence on flow mechanics, deposits?

Frictional ash production experiment 24 R = 1 � y � ( u ) Cagnoli and Manga, JGR (2004)

Collisional ash production experiment 2 � 3/ 2 � ( ) ( ) g 0 � 2 � 2 � 23 R = 24 � � � ( ) � 3/ 2 2 d � � � � Ash, not fractal size distribution (collision energy not large enough)

1. Generating ash within flows Continuity � � ) + � � t ( g � g ( g � g � g U i ) = 0 Thermal � x i � � ) + � � t ( 2 � 2 ( 2 � 2 � 2 U i ) = � 23 R � 24 R � g � g � = � g � T T � q � � g � g � g c p � t + g U i � H g 2 � H g 3 � H g 4 � x i � Mass loss due to Mass loss due to � x i � x i � � collisional ash frictional ash prodution prodution � � ) + � � t ( 3 � 3 ( 3 � 3 � 3 U i ) = + 23 R � 2 � 2 � = � 2 � T T � q � 2 � 2 � 2 c p � t + 2 U i + H g 2 � x i � Mass gain due to � x i � x i � � collisional ash prodution � � ) + � � t ( 4 � 4 ( 4 � 4 � 4 U i ) = + 24 R � 3 � 3 � = � 3 � T T � q � 3 � 3 � 3 c p � t + 3 U i � x i + H g 3 � Mass gain due to � x i � x i � � frictional ash prodution � 4 � 4 � = � 4 � T T � q 4 � 4 � 4 c p � t + 4 U i + H g 4 � � x i � x i � � Momentum � ij + � g g U j ) = � g P � ij � � U i ) + � � t ( g � g ( g � g � g U i + g I i + g � g � g i � x i � x i � x j � ij + � 2 2 U j ) = � 2 P � ij � � U i ) + � � t ( 2 � 2 ( 2 � 2 � 2 U i + 2 I i + 2 � 2 � g i � 23 R 2 U i � 24 R 2 U i � x i � x i � x j � ij + � 3 3 U j ) = � 3 P � ij � � U i ) + � � t ( 3 � 3 ( 3 � 3 � 3 U i + 3 I i + 3 � 3 � g i + 23 R 3 U i � x i � x i � x j � ij + � 4 4 U j ) = � 4 P � ij � � U i ) + � � t ( 4 � 4 ( 4 � 4 � 4 U i + 4 I i + 4 � 4 � g i + 24 R 4 U i � x i � x i � x j

Model problem grid 1m x 5 m; time step < 0.1s initial velocity 50 m/s initial concentration 0.025 initial size 1 cm temperature 650 C

Flow over level terrain 1 cm pumice Collisional ash Frictional ash Collisional and frictional ash are well mixed Travels far beyond pumice Total ash produced: 7%

1 cm pumice Downslope Collisional ash Accelerating Frictional ash

Ash production rate 1 cm pumice Ash generated in more energetic part of flow Collisional ash Frictional ash

Ash production rate 1 cm pumice Collisional ash Frictional ash

Conclusions • � A few to a few 10s of % of flow is converted to ash • � Ash generation increases runout distance • � Ash generated within flow separates from larger particles (travels faster, higher, farther) Lacroix 1902 • � Origin of rounding of larger rounded clasts St. Helens, USGS

2. Transport capacity of pyroclastic flows: substrate-flow interactions Kos Plateau tuff

Role of boundary condition

Particle-substrate interactions Air gun Measure velocity before and after collision; whether particle bounces Variables: angle � , velocity, mass, substrate

Example (water substrate) • � Extract quantitative information . . .

Water substrate sinkers Restitution coefficient: Fraction that sink:

Pumice substrate No effect of mass, impact velocity Restitution coefficient:

Model problem initial velocity 80 m/s Initial height 100 m initial concentration 0.025 or 0.40 density 1000 kg/m 3 size: 95% are 10 microns, 5% are 0.5 mm temperature 700 K; air 300 K

Effect of boundary type pumice 10 microns 0.5 mm 10 microns water 0.5 mm Over land flows develop a dense bed-load region because particles are not lost from the flow

Add Lagrangian tracers Interact with the flow, but do not affect the flow Introduced near inlet Size from 1 micron to 10 m

Over water > 1cm concentration 0.025 furthest transport of 1 cm clast < 1cm > 1cm concentration 0.40 < 1cm No concentrated bedload Concentration has little effect of large clast transport

Over land > 1cm concentration 0.025 furthest transport of 1 cm clast < 1cm > 1cm concentration 0.40 < 1cm With concentrated bedload, large clasts transported to the end of the flow! Flows travel further than over water

Over water Over land 0.025 0.025 > 1cm < 1cm 0.40 0.40 1) Boundary condition has a small effect for dilute flows 2) Dense flows over land develop a particle-rich bedload which transports large clasts (over water, particles sink and no particle-rich bedload forms)

Adding mass back into the current? Can settling particles “splash” mass back into the current?

Experiments

Experiments Fauria et al. (2016) Mass ejected can exceed mass of incident particle

Scaling Fauria et al. (2016) New and literature data, new scaling law

Density current model Compute concentration, velocity, temperature as a function of time (and distance) Assume turbulent gravity current (e.g., Dade and Huppert, 1995) Allow big particles to settle more details in Fauria et al. (2016)

Density current model Allow small particles to splash Conserve energy Currents travel until either all particles settle, or they become buoyant more details in Fauria et al. (2016)

Splash cools flow, increases runout Fauria et al. (2016) Fauria et al. (2016)

Splash cools flow, increases runout Fauria et al. (2016)

What we learned Large clast transport is . . . 1) � dominated by momentum exchange from smaller particles 2) � suppressed over water because a dense bedload region does not develop (boundary effect is indirect through the concentration of particles in bedload region) 3) � Resuspension can change runnout distance by an order-of-magnitude

3. Interaction with water Hot flows, when they enter water, generate stream Montserrat, Univ West Indies • � How much? • � How fast? • � Effects of steam generation?

Measurement of steam production rate Stroberg, Manga and Dufek, JVGR 2010 100-700 o C Pumice, glass beads 3 mm - 2 cm 1) � Measure mass of stream released 2) � Measure time clasts float (results in Dufek, Manga Staedter, J Geophys Res 2007)

Measurement of steam production rate ( ) � ( ) ( ) ( p T � w T ) ( ) � ( ) ( p T � w T ) ( ) p � ( ) p c p p � p c p 6 p � R v = = � m p [ w c p ( b T � w T ) + L ] �� d 3 [ w c p ( b T � w T ) + L ]