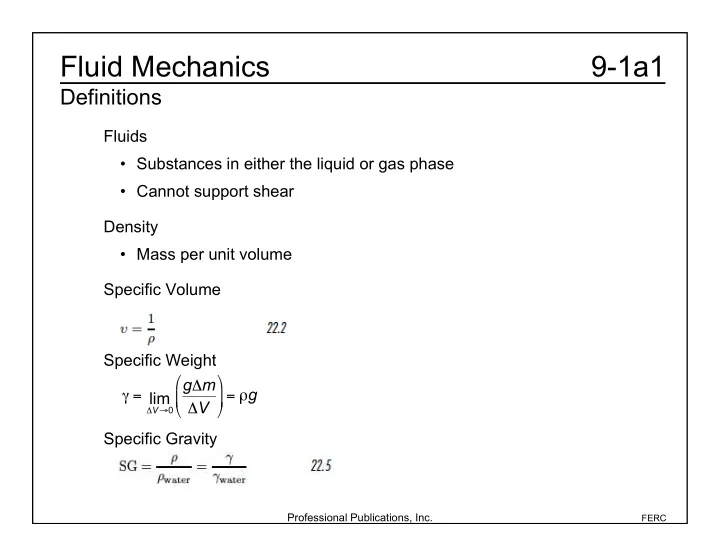

Fluid Mechanics 9-1a1 Definitions Fluids • Substances in either the liquid or gas phase • Cannot support shear Density • Mass per unit volume Specific Volume Specific Weight � � g � m � = � g � = lim � � V � � � V � 0 Specific Gravity Professional Publications, Inc. FERC
Fluid Mechanics 9-1a2 Definitions Example (FEIM): Determine the specific gravity of carbon dioxide gas (molecular weight = 44) at 66°C and 138 kPa compared to STP air. J 8314 kmol � K R carbon dioxide = = 189 J/kg � K 44 kg kmol J 8314 kmol � K R air = = 287 J/kg � K 29 kg kmol � � � � � � J 287 (273.16) � � � � 5 Pa � � kg � K = PR air T STP 1.38 � 10 � � � � � � � SG = = 1.67 = 5 Pa R CO 2 Tp STP � � 1.013 � 10 J � � � � � STP 189 (66 ° C + 273.16) � � � � � � kg � K � � � � � � Professional Publications, Inc. FERC
Fluid Mechanics 9-1b Definitions Shear Stress • Normal Component: • Tangential Component - For a Newtonian fluid: - For a pseudoplastic or dilatant fluid: Professional Publications, Inc. FERC
Fluid Mechanics 9-1c1 Definitions Absolute Viscosity • Ratio of shear stress to rate of shear deformation Surface Tension Capillary Rise Professional Publications, Inc. FERC
Fluid Mechanics 9-1c2 Definitions Example (FEIM): Find the height to which ethyl alcohol will rise in a glass capillary tube 0.127 mm in diameter. Density is 790 kg/m 3 , � = 0.0227 N/m, and � = 0 ° . � � (4) 0.0227 kg (1.0) � � s 2 h = 4 � cos � � � = 0.00923 m = � d � � � � 790 kg � 9.8 m (0.127 � 10 � 3 m) � � � m 3 s 2 � � � � Professional Publications, Inc. FERC
Fluid Mechanics 9-2a1 Fluid Statics Gage and Absolute Pressure Hydrostatic Pressure p absolute = p gage + p atmospheric p = � h + � gh p 2 � p 1 = �� ( z 2 � z 1 ) Example (FEIM): In which fluid is 700 kPa first achieved? (A) ethyl alcohol (B) oil (C) water (D) glyceri Professional Publications, Inc. FERC
Fluid Mechanics 9-2a2 Fluid Statics p 0 = 90 kPa p 1 = p 0 + � 1 h 1 = 90 kPa + 7.586kPa � � (60 m) = 545.16 kPa � � m � � � � p 2 = p 1 + � 2 h 2 = 545.16 kPa + 8.825kPa (10 m) = 633.41 kPa � � m � � � � p 3 = p 2 + � 3 h 3 = 633.41 kPa + 9.604kPa (5 m) = 681.43 kPa � � m � � � � p 4 = p 3 + � 4 h 4 = 681.43 kPa + 12.125 kPa (5 m) = 742 kPa � � m � � Therefore, (D) is correct. Professional Publications, Inc. FERC
Fluid Mechanics 9-2b1 Fluid Statics Manometers Professional Publications, Inc. FERC
Fluid Mechanics 9-2b2 Fluid Statics Example (FEIM): The pressure at the bottom of a tank of water is measured with a mercury manometer. The height of the water is 3.0 m and the height of the mercury is 0.43 m. What is the gage pressure at the bottom of the tank? From the table in the NCEES Handbook, � mercury = 13560 kg 3 � water = 997 kg/m 3 m � p = g � 2 h 2 � � 1 h 1 ( ) � � � � � � � � = 9.81m � 13560 kg (0.43 m) � 997 kg (3.0 m) � � � � � � � s 2 m 3 m 3 � � � � � � � � = 27858 Pa Professional Publications, Inc. FERC
Fluid Mechanics 9-2c Fluid Statics Barometer Atmospheric Pressure Professional Publications, Inc. FERC
Fluid Mechanics 9-2d Fluid Statics Forces on Submerged Surfaces The average pressure on the inclined section is: ( ) 997 kg � � 9.81m � � � ( ) p ave = � 3 m + 5 m 1 � � 2 3 2 m s � � � � = 39122 Pa Example (FEIM): The tank shown is filled with water. The resultant force is Find the force on 1 m width of the ( ) 2.31 m ( ) 1 m ( ) R = p ave A = 39122 Pa inclined portion. = 90372 N Professional Publications, Inc. FERC
Fluid Mechanics 9-2e Fluid Statics Center of Pressure If the surface is open to the atmosphere, then p 0 = 0 and Professional Publications, Inc. FERC
Fluid Mechanics 9-2f1 Fluid Statics Example 1 (FEIM): The tank shown is filled with water. At what depth does the resultant force act? A = bh I y c = b 3 h The surface under pressure is a 12 rectangle 1 m at the base and 4 m 2.31 m tall. Z c = sin60 ° = 4.618 m Professional Publications, Inc. FERC
Fluid Mechanics 9-2f2 Fluid Statics Using the moment of inertia for a rectangle given in the NCEES Handbook, b 3 h b 2 z * = I y c = = AZ c 12 bhZ c 12 Z c (2.31 m) 2 (12)(4.618 m) = 0.0963 m = R depth = ( Z c + z *) sin 60° = (4.618 m + 0.0963 m) sin 60° = 4.08 m Professional Publications, Inc. FERC
Fluid Mechanics 9-2g Fluid Statics Example 2 (FEIM): The rectangular gate shown is 3 m high and has a frictionless hinge at the bottom. The fluid has a density of 1600 kg/m 3 . The magnitude of the p ave = � gz ave (1600 kg 3 )(9.81m 2 )( 1 2 )(3 m) force F per meter of width to keep the m s gate closed is most nearly = 23544 Pa R w = p ave h = (23544 Pa)(3 m) = 70662 N/m F + F h = R R is one-third from the bottom (centroid of a triangle from the NCEES Handbook). Taking the moments about R, (A) 0 kN/m 2 F = F h 70,667 N (B) 24 kN/m � � � � w = 1 F � R (C) 71 kN/m m = 23.6 kN/m � = � � 3 w 3 (D) 370 kN/m � � � � Therefore, (B) is correct. Professional Publications, Inc. FERC
Fluid Mechanics 9-2h Fluid Statics Archimedes’ Principle and Buoyancy • The buoyant force on a submerged or floating object is equal to the weight of the displaced fluid. • A body floating at the interface between two fluids will have buoyant force equal to the weights of both fluids displaced. F buoyant = � water V displaced Professional Publications, Inc. FERC
Fluid Mechanics 9-3a Fluid Dynamics Hydraulic Radius for Pipes Example (FEIM): A pipe has diameter of 6 m and carries water to a depth of 2 m. What is the hydraulic radius? r = 3 m d = 2 m � = (2 m)(arccos(( r � d )/ r )) = (2 m)(arccos 1 3 ) = 2.46 radians (Careful! Degrees are very wrong here.) s = r � = (3 m)(2.46 radians) = 7.38 m A = 1 2 ( r 2 ( � � sin � )) = ( 1 2 )((3 m) 2 (2.46 radians � sin2.46)) = 8.235 m 2 R H = A s = 8.235 m 2 7.38 m = 1.12 m Professional Publications, Inc. FERC
Fluid Mechanics 9-3b Fluid Dynamics Continuity Equation � 1 = � 2 . If the fluid is incompressible, then Professional Publications, Inc. FERC
Fluid Mechanics 9-3c Fluid Dynamics Example (FEIM): The speed of an incompressible fluid is 4 m/s entering the 260 mm pipe. The speed in the 130 mm pipe is most nearly (A) 1 m/s (B) 2 m/s (C) 4 m/s (D) 16 m/s A 1 v 1 = A 2 v 2 A 1 = 4 A 2 � � ( ) 4m so v 2 = 4 v 1 = 4 � = 16 m/s � s � � Therefore, (D) is correct. Professional Publications, Inc. FERC
Fluid Mechanics 9-3d1 Fluid Dynamics Bernoulli Equation • In the form of energy per unit mass: p 1 + v 1 2 + gz 1 = p 2 2 + v 2 2 2 + gz 2 � 1 � 2 Professional Publications, Inc. FERC
Fluid Mechanics 9-3d2 Fluid Dynamics Example (FEIM): A pipe draws water from a reservoir and discharges it freely 30 m below the surface. The flow is frictionless. What is the total specific energy at an elevation of 15 m below the surface? What is the velocity at the discharge? Professional Publications, Inc. FERC
Fluid Mechanics 9-3d3 Fluid Dynamics Let the discharge level be defined as z = 0, so the energy is entirely potential energy at the surface. � � E surface = z surface g = (30 m) 9.81m � = 294.3 J/kg � 2 s � � (Note that m 2 /s 2 is equivalent to J/kg.) The specific energy must be the same 15 m below the surface as at the surface. E 15 m = E surface = 294.3 J/kg The energy at discharge is entirely kinetic, so E discharge = 0 + 0 + 1 2 v 2 � � (2) 294.3 J v = � = 24.3 m/s � kg � � Professional Publications, Inc. FERC
Fluid Mechanics 9-3e Fluid Dynamics Flow of a Real Fluid • Bernoulli equation + head loss due to friction ( h f is the head loss due to friction) Professional Publications, Inc. FERC
Fluid Mechanics 9-3f Fluid Dynamics Fluid Flow Distribution If the flow is laminar (no turbulence) and the pipe is circular, then the velocity distribution is: r = the distance from the center of the pipe v = the velocity at r R = the radius of the pipe v max = the velocity at the center of the pipe Professional Publications, Inc. FERC
Fluid Mechanics 9-3g Fluid Dynamics Reynolds Number For a Newtonian fluid: D = hydraulic diameter = 4R H � = kinematic viscosity µ = dynamic viscosity For a pseudoplastic or dilatant fluid: Professional Publications, Inc. FERC
Fluid Mechanics 9-3h Fluid Dynamics Example (FEIM): What is the Reynolds number for water flowing through an open channel 2 m wide when the flow is 1 m deep? The flow rate is 800 L/s. The kinematic viscosity is 1.23 × 10 -6 m 2 /s. D = 4R H = 4 A p = (4)(1 m)(2 m) 2 m + 1 m + 1 m = 2 m 800L v = Q s 2 = 0.4 m/s A = 2 m � 0.4m � (2 m) � � s Re = v D � � = 6.5 � 10 5 � = � 6 m 2 1.23 � 10 s Professional Publications, Inc. FERC
Fluid Mechanics 9-3i Fluid Dynamics Hydraulic Gradient • The decrease in pressure head per unit length of pipe Professional Publications, Inc. FERC
Fluid Mechanics 9-4a Head Loss in Conduits and Pipes Darcy Equation • calculates friction head loss Moody (Stanton) Diagram: Professional Publications, Inc. FERC
Recommend
More recommend