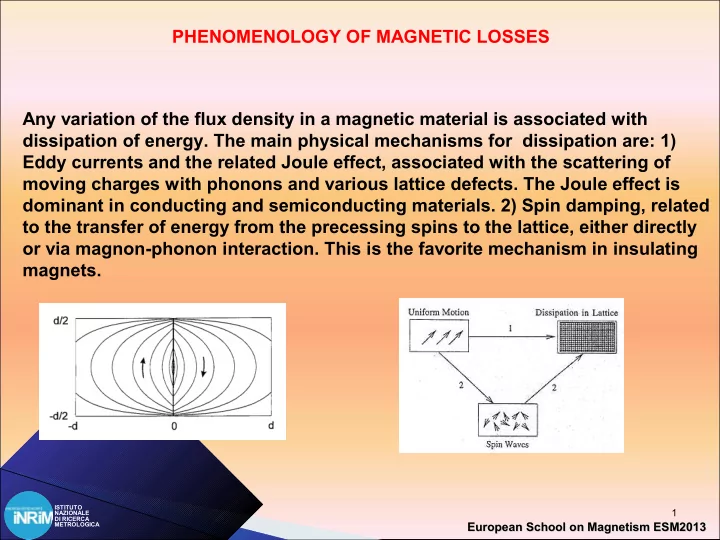
PHENOMENOLOGY OF MAGNETIC LOSSES Any variation of the flux density - PowerPoint PPT Presentation

PHENOMENOLOGY OF MAGNETIC LOSSES Any variation of the flux density in a magnetic material is associated with dissipation of energy. The main physical mechanisms for dissipation are: 1) Eddy currents and the related Joule effect, associated with the scattering of moving charges with phonons and various lattice defects. The Joule effect is dominant in conducting and semiconducting materials. 2) Spin damping, related to the transfer of energy from the precessing spins to the lattice, either directly or via magnon-phonon interaction. This is the favorite mechanism in insulating magnets. ISTITUTO ISTITUTO 1 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

Magnetic losses are conveniently defined under given time periodic law for the rate of change of the magnetization. Typically, one has to deal with either sinusoidal or triangular B ( t ) waveform. The viscous character of the magnetization process makes attaining a given peak polarization under defined B ( t ) law increasingly difficult with increasing the magnetizing frequency. There is an obvious practical appeal to the study of magnetic losses, because there is a stringent need for ther prediction in most electrical/electronic applications. Besides the unnecessary waste of energy, the fast increase of the power loss with the magnetizing frequency (somewhat ∝ f 2 ) can make overheating the main obstacle to high-frequency applications ( P = c v d T /d t ). For sample of volume V subjected to periodic excitation at the frequency f = 1/ T, we can express the eddy-current energy loss per unit volume on a period as 2 j ( r , t ) 3 d r T ∫ ∫ = W dt σ V V 0 The space-time behavior of the current density j ( r , t ) is hopelessly complicated. We need to focus on the general features of the loss behavior, to be treated from a statistical viewpoint. ISTITUTO ISTITUTO 2 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

∫ ∫ ∫ ∫ Grain-oriented = = µ + = W H dB H dH H dJ H dJ 1.5 o Fe-(3wt%)Si d = 0.30 mm 1.0 J p = 1.7 T H and X conjugate work variables = + δ dU HdX Q 0.5 0.25 Hz J (T) ∫ dU 0.0 = 0 Isothermal cycle: -0.5 ∫ ∫ = − δ HdX Q 150 Hz -1.0 -1.5 600 Grain-oriented 400 Hz Fe-(3wt%)Si -150 -100 -50 0 50 100 150 500 3 ) d = 0.30 mm Energy loss (J/m H m (A/m) J p = 1.7 T 400 W exc 300 W = W hyst + W cl + W exc 200 W cl W h 100 = + + 1 / 2 W ( J , f ) W ( J ) k f k f p hyst p cl exc 0 0 100 200 300 400 Frequency (Hz) ISTITUTO ISTITUTO 3 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

Amorphous ribbon Soft ferrite ring ISTITUTO ISTITUTO 4 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

Soft ferrite ring ∼ 20 kW/kg Nanocrystalline ribbon ISTITUTO ISTITUTO 5 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

nonoriented Fe-Si Excess energy loss under generic J p = 1.5 T, d = 0.35 mm 100 polarization rate d J /d t T T W exc ∫ ∫  = = σ 3 / 2 W p ( t ) dt GSV | J ( t ) | d t W (mJ/kg) exc exc o 0 0 50 W cl 80 nonoriented Fe-Si J p = 1.5 T, d = 0.35 mm W hyst 60 ( W - W cl ) (mJ/kg) 0 0 100 200 300 400 500 f (Hz) 40 W exc Sinusoidal induction 20 ( ) = + π σ + σ 2 2 2 3 / 2 1 / 2 W W / 6 d J f 8 . 76 GSV J f h p o p W hyst 0 0 5 10 15 20 25 1/2 (Hz) f ISTITUTO ISTITUTO 6 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

The hysteresis (quasi-static) energy loss cannot be generally predicted from knowledge of the structural properties and the intrinsic magnetic parameters. At low inductions, however, magnetic hysteresis can usually be described by the analytical Rayleigh law. ( H p , J p ) Pure Ni foil 0,06 b 0,04 2 2 = + − J ( H ) ( a bH ) H ( H H )  p p 2 0,02 0,00 J (T) 2 -0,02 = + J aH bH p p p -0,04 -0,06 -300 -200 -100 0 100 200 300 a ≡ initial permeability, resulting from H (A/m) domain wall bending and moment rotations ISTITUTO ISTITUTO 7 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

The dynamic loss can be formally assessed in a linear (or, in practice, quasi- linear) material, with defined DC permeability. Dissipative phenomena bring about a time delay of the B ( t ) waveform with respect to the H ( t ) waveform and the resulting hysteresis loop takes an elliptical shape 0.010 Co 67 Fe 4 B 14.5 Si 14.5 J p = 10 mT = ω H ( t ) H cos t 0.005 p ( ) = ω − δ = B ( t ) B cos t 0.000 J (T) p = δ ω + δ ω B cos cos t B sin sin t p p -0.005 Bm 20Hz B 20kHz B 200kHz B 500kHz B 1MHz B 2MHz The associated energy loss (area B 5MHz -0.010 of the elliptical loops) is -4 -3 -2 -1 0 1 2 3 4 H (A/m) = ∫ dB ( t ) T ⋅ = π δ W H ( t ) dt H B sin p p dt 0 ISTITUTO ISTITUTO 8 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

When dealing with a linear system we can attack in a simple way the phenomenology of energy loss and the related concepts of complex permeability, quality factor, and equivalent L - R circuit i s R s δ R p L p j ω L s i s L s R s i s ISTITUTO ISTITUTO 9 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

The concept of loss separation can be given a solid physical justification in terms of characteristic space-time scales of the magnetization process (G. Bertotti, Hysteresis in Magn etism ). It is valid in both conducting and non- conducting magnetic materials. W = W hyst + W cl + W exc = + + 1 / 2 y W ( J , f ) W ( J ) k f k f p hyst p cl exc z x B(t) W cl is easily calculated in the simple case of a thin lamination (width >> thickness) under time-varying induction (e. g., B ( t ) = B p sin ω t ) uniform across the sheet cross-section. ISTITUTO ISTITUTO 10 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

The full approach to the classical eddy current losses, valid for whatever lamination thickness and material conductivity is based on the diffusion equation y for the internal magnetic field. z d B ∇ × = − x ∇ × = − H j E dt H a (t) ∂ ∂ H E dH = − = − σ = − µ j E H ( t ) = H a ( t )- H eddy ( t ) dy dy dt ∂ 2 H dH and the boundary conditions = σ µ 2 dy dt = = ± H ( t ) H ( t ) for y d / 2 a ∂ ∂ = = H / y 0 at y 0 ISTITUTO ISTITUTO 11 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

The concept of magnetic losses and hysteresis are associated with that of lag in time of induction with respect to the field. We can therefore talk aboy a time constant (or a distribution of time constants). The simplest case of relaxation is one 1.0 where the change of the magnetization with time is 0.8 proportional to its deviation from the equilibrium value 0.6 M / M 0 = − dM / dt k ( M M ( t )) 0.4 0 / ) − t τ = − M ( t ) ( 1 e M 0.2 0 with τ = 1/ k 0.0 0 1 2 3 4 5 6 t / τ If relaxation is due to eddy currents, the time constant will depend on conductivity, permeability, and sample size. ISTITUTO ISTITUTO 12 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA

Bibliography •G. Bertotti, Hysteresis in Magnetism , Academic Press, 1988. •G. Bertotti, IEEE Trans. Magn . 24 (1988) 621. •G. Bertotti, J. Appl. Phys . 57 (1985) 2110. •J.B. Goodenough, IEEE Trans. Magn . 38 (2002) 3398. •F. Fiorillo, C. Appino, M. Pasquale, in The Science of Hysteresis (G. Bertotti and I. Mayergoyz, eds., Academic Press, 2006), vol III, p.1. •R.H. Pry and C.P. Bean, J. Appl. Phys . 29 (1958) 532. • H.J. Williams, W. Shockley, C. Kittel, Phys. Rev. 80 (1950) 1090. •B.D. Cullity and C.D. Graham, Introduction to magnetic materials, Wiley, 2011. •F. Fiorillo and A. Novikov, IEEE Trans. Magn . 26 (1990) 2904. •G. Bertotti and I. Mayergoyz, eds., The Science of Hysteresis, Academic Press, 2006. ISTITUTO ISTITUTO 13 NAZIONALE NAZIONALE DI RICERCA DI RICERCA European School on Magnetism ESM2013 European School on Magnetism ESM2013 METROLOGICA METROLOGICA