Magnetics Design 3.1 Important magnetic equations 3.2 Magnetic - PDF document

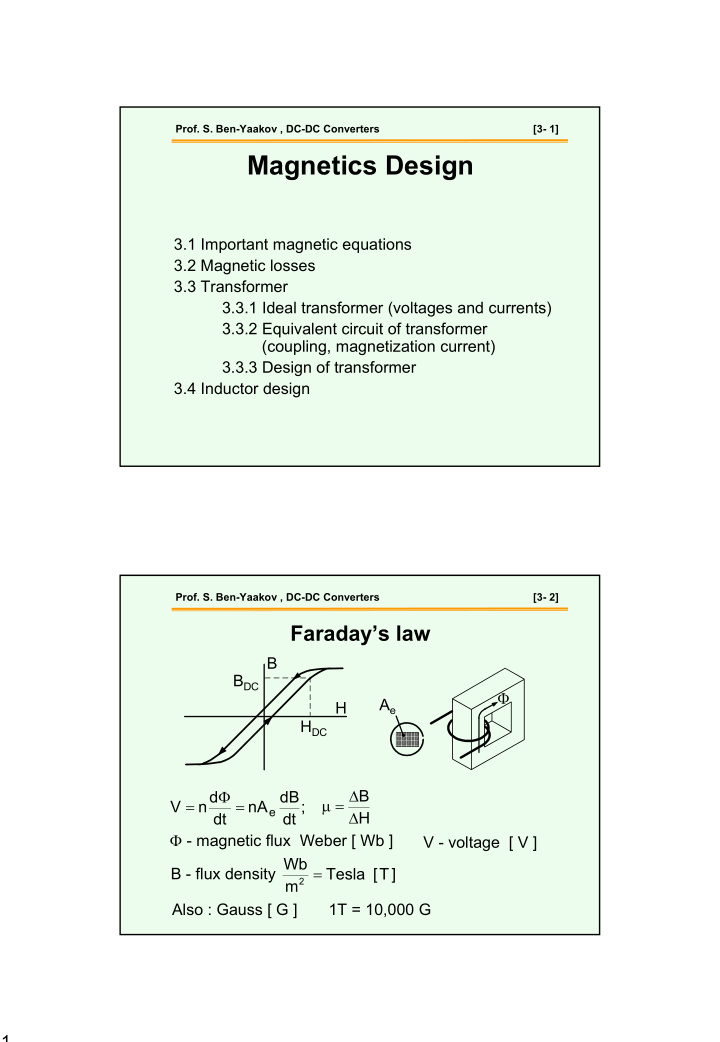
Prof. S. Ben-Yaakov , DC-DC Converters [3- 1] Magnetics Design 3.1 Important magnetic equations 3.2 Magnetic losses 3.3 Transformer 3.3.1 Ideal transformer (voltages and currents) 3.3.2 Equivalent circuit of transformer (coupling, magnetization current) 3.3.3 Design of transformer 3.4 Inductor design Prof. S. Ben-Yaakov , DC-DC Converters [3- 2] Faraday’s law B B DC Φ A e H H DC ∆ Φ d dB B µ = = = V n nA ; e ∆ H dt dt Φ - magnetic flux Weber [ Wb ] V - voltage [ V ] Wb 2 = B - flux density Tesla [ T ] m Also : Gauss [ G ] 1T = 10,000 G 1

Prof. S. Ben-Yaakov , DC-DC Converters [3- 3] Ampere’s law H - magnetic field [ A/m ] l e I ∫ = ⋅ Hd l n I n ⋅ = ⋅ n I H l e ⋅ n I = [ A/m ] H l e Prof. S. Ben-Yaakov , DC-DC Converters [3- 4] Magnetic losses mW B P B DC 3 cm H H DC ∆ B Magnetic losses ~ ∆ B “Good number” = 100mW/cm 3 =100KW/m 3 2

Prof. S. Ben-Yaakov , DC-DC Converters [3- 5] Magnetic Losses � “Good number”=100mW/cm 3 =100 kW/m 3 Prof. S. Ben-Yaakov , DC-DC Converters [3- 6] Magnetic losses mW B P B DC 3 cm H H DC ∆ B Magnetic losses ~ ∆ B “Good number” = 100mW/cm 3 =100KW/m 3 3

Prof. S. Ben-Yaakov , DC-DC Converters [3- 7] Magnetic Losses Prof. S. Ben-Yaakov , DC-DC Converters [3- 8] Magnetic Losses Curves for constant loss: 500mW/cm 3 � Figure of merit B*f � Each material has optimum operating temperature � (minimum loss) 4

Prof. S. Ben-Yaakov , DC-DC Converters [3- 9] Transformer currents I 2 I 1 I 1 I 2 n 2 n 1 n 1 n 2 I = n = 1 2 n I n I For ideal transformer 1 1 2 2 I n 2 1 = At any given moment n I n I 1 1 2 2 I 1 ,I 2 opposite direction. No magnetic energy stored due to useful currents I 1 , I 2 (they cancel each other) Prof. S. Ben-Yaakov , DC-DC Converters [3- 10] Transformer voltages n 1 n 2 V 1 V 2 φ φ φ d d = 1 = 2 V n V n 1 1 2 2 dt dt φ = φ Assu min g 1 2 φ φ d d 1 = 2 dt dt V = n 1 1 V n 2 2 5

Prof. S. Ben-Yaakov , DC-DC Converters [3- 11] Voltages Since each winding also represents an inductance, = therefore for any winding V 0 n Permissible voltages: AC only on any winding V A S 1 S 1 t S 2 S 2 V B S 1 S 1 t S 2 S 2 V C S 1 S 1 t S 2 S 2 Prof. S. Ben-Yaakov , DC-DC Converters [3- 12] Equivalent circuit (preliminary) Ideal Ideal L lkg1 L lkg1 L m2 L m1 1:n 1:n Ideal 2 2 = L L n L lkg2 m m 1 L m1 → ∞ Ideal transformer L 1:n 6

Prof. S. Ben-Yaakov , DC-DC Converters [3- 13] Leakage � Leakage inductance is the � Leakage inductance uncoupled magnetic flux L kg1 L kg2 1:n I 1 I 2 n 1 V 1 V 2 L m1 n 2 ideal � Relationship between L lkg , M and k (coupling coefficient). = ⋅ M k L 1 L 2 ≅ − L L ( 1 k ) lkg 1 m 1 ≅ ⋅ 2 L L n lkg 2 lkg 1 Prof. S. Ben-Yaakov , DC-DC Converters [3- 14] Leakage L lkg1 L lkg2 1:n V o L m1 ideal V ′ V = o L lkg1 L' lkg2 o 2 n L ′ V' o = lkg 2 L L m1 lkg 2 2 n 7

Prof. S. Ben-Yaakov , DC-DC Converters [3- 15] Magnetization Current V in L lkg2 I 1 Ideal t V o I 2 I m V in R V o t L m1 I 2 1:n t I m t I 1 t Prof. S. Ben-Yaakov , DC-DC Converters [3- 16] Transformer B sat+ B I 1 I 2 B max+ n 1 V 1 V 2 n 2 ∆Β H B max- B sat- 1. B max ( could be symmetrical or asymmetrical ) 2. B max < B sat 3. In most case ( high frequency ) B max limit by magnetic losses. Φ d dB = = V n n A 1 1 1 e dt dt 8

Prof. S. Ben-Yaakov , DC-DC Converters [3- 17] Symmetrical operation V 1 1 = ∫ B Vdt V m n A 1 e + = − B B t on max max T s V t B m on ∆ = = B 2 B B max+ max n A 1 e { } V , t m on n = 1 2 B A B max- max e 1 n A ~ t ~ T 1 e on on → S t f 2 s Prof. S. Ben-Yaakov , DC-DC Converters [3- 18] Skin effect High Frequency DC δ − skin depth δ R AC > 72 1 δ = ( mm ) R f DC f in Hz 9

Prof. S. Ben-Yaakov , DC-DC Converters [3- 19] Skin Effect Solutions Litz wire Tape Prof. S. Ben-Yaakov , DC-DC Converters [3- 20] Proximity effect I I Current crowding due to magnetic fields 10

Prof. S. Ben-Yaakov , DC-DC Converters [3- 21] Aw [ ] ⋅ + ⋅ w n w n = A 1 A 2 2 A 1 w k k - filling factor k<1 I 1 A 1 = rms w J A w w A J - current density A/m 2 J ≅ 4.5 A/mm 2 A w - winding area n I = 1 I 2 1 n 2 Prof. S. Ben-Yaakov , DC-DC Converters [3- 22] Ap n I JkA = = 1 1 ⋅ A rms 2 n w { } w 1 ⋅ JkA V , t Jk 2 I = 1 w 1 on rms { } V , t ⋅ I 2 2 B A n = 1 on 1 rms max e 1 2 B A max e { } ⋅ V , t 2 I = = A A A 1 on 1 rms { } Jk p w e 2 B max { } ⋅ V , t 2 I 1 on 1 = A rms p ∆ ⋅ B Jk { } ⋅ V , D 2 I 1 on 1 = A rms p ⋅ ∆ ⋅ f B Jk s 11

Prof. S. Ben-Yaakov , DC-DC Converters [3- 23] Transformer design stages { } ⋅ V , D 2 I = 1 on 1 1. Calculate A p A rms p ⋅ ∆ ⋅ f B Jk s In symmetrical operation In asymmetrical operation ∆ B = B max + - B max - ∆ B = B max - 0 2. Look for core { } V , t n = m on 3. Calculate n 1 by: 1 2 B A max e 4. Calculate n 2 Prof. S. Ben-Yaakov , DC-DC Converters [3- 24] Inductor design Need to store energy I ( in transformer n 1 ·I 1 = n 2 ·I 2 ) L B µ = µ o µ r µ o - air (vacuum) permeability µ H µ r - relative permeability 12

Prof. S. Ben-Yaakov , DC-DC Converters [3- 25] Permeability Henry µ r of ferrites ∼ 2000 - 4000 µ o = 1.26·10 -6 m B = µ H B B o µ r 1 If µ is high B will reach quickly B sat µ H r 2 Need to slower µ H o µ < µ r 2 r 1 Prof. S. Ben-Yaakov , DC-DC Converters [3- 26] Gaps Discrete Distributed µ r µ o air gap air gap Φ Same Φ magnetic lines in ferromagnetic material and in air. << l l l g e e l g + ≅ l l l g e e 13

Prof. S. Ben-Yaakov , DC-DC Converters [3- 27] Current Crowding Current crowding due to magnetic fields R AC high around gap Prof. S. Ben-Yaakov , DC-DC Converters [3- 28] Inductance with Gap << l l l g e e l g + ≅ l l l g e e Φ = constant B ≅ constant B B = = H H B l B l g g m e µ µ = + H l e o m µ µ m o = = + nI H H H l l l e m e g g 14

Prof. S. Ben-Yaakov , DC-DC Converters [3- 29] Inductance with Gap B B l l = + g H e l e µ µ m o B µ e = � Dividing out l e and defining H B B B = = m + a H µ µ l e e m µ o l g Prof. S. Ben-Yaakov , DC-DC Converters [3- 30] Gap Calculation   l   e + µ 1 1 1   rm = + l 1   g µ µ   = l e m   e µ µ     l o re   e l µ   g   rm l   g 1 1 1   = + l   µ µ µ µ µ e   l   re o rm o rm   µ e l     g o µ = l   g re   l   e + µ 1 1 1   rm = + l   g µ µ   l re rm   e     l   l l g   e < µ µ ≈ e If   rm re l l   g g 15

Prof. S. Ben-Yaakov , DC-DC Converters [3- 31] Inductance Φ dI d V = = L V n dt dt Φ dI d = L n dt dt L-? Φ d dB dH n dI = = µ = µ n nA nA nA e e e dt dt dt dt l e 2 µ 2 A µ dI n A dI n = = L e L e dt dt l l e e Prof. S. Ben-Yaakov , DC-DC Converters [3- 32] Two windings on same core 2   L n 1 1 =   L n   2 2 Inductor design B B max H 16

Prof. S. Ben-Yaakov , DC-DC Converters [3- 33] Saturation Limits Φ dI d = L n dt dt  dI   dB  I B ∫ ∫ pk = max L   dt nA   dt e  dt   dt  0 0 L I pk = nA e B max LI pk n = quick design and check A B e max LI JkA pk = w A n = e nB I max rms Prof. S. Ben-Yaakov , DC-DC Converters [3- 34] Ap LI I pk rms = = A A A p e w B Jk max 2 ≈ LI I LI pk rms 2 LI = Energy stored 2 Air gapped core Design 1. Calculate A p 2. Choose a core 3. Iterate 4. Calculate ( or increase gap until L is as required ) l g 17

Prof. S. Ben-Yaakov , DC-DC Converters [3- 35] Cores � Transformer core � Inductor core air gap Prof. S. Ben-Yaakov , DC-DC Converters [3- 36] Cores 1. E - core 2. TOROID 3. ARENCO 4. POT 18

Prof. S. Ben-Yaakov , DC-DC Converters [3- 37] Commercial cores Prof. S. Ben-Yaakov , DC-DC Converters [3- 38] Distributed gap core � The concept of A L H H y y L = A ( sometime ) turn 1000 turns 2 A = ⋅ L for n turns: L n L Distributed air gap 19

Prof. S. Ben-Yaakov , DC-DC Converters [3- 39] A L Prof. S. Ben-Yaakov , DC-DC Converters [3- 40] Toroid Data 20

Prof. S. Ben-Yaakov , DC-DC Converters [3- 41] Permeability change 1 Amp/m =79.5 Oe L decreases with DC current ! Prof. S. Ben-Yaakov , DC-DC Converters [3- 42] Losses Misleading notations ! ∆ B NOT B “Good number”=100mW/cm 3 These curves are measured by feeding ac signals. If the current is composed of DC + ripple, core loss is due only to ripple component ! DC bias tend to increase loss 21