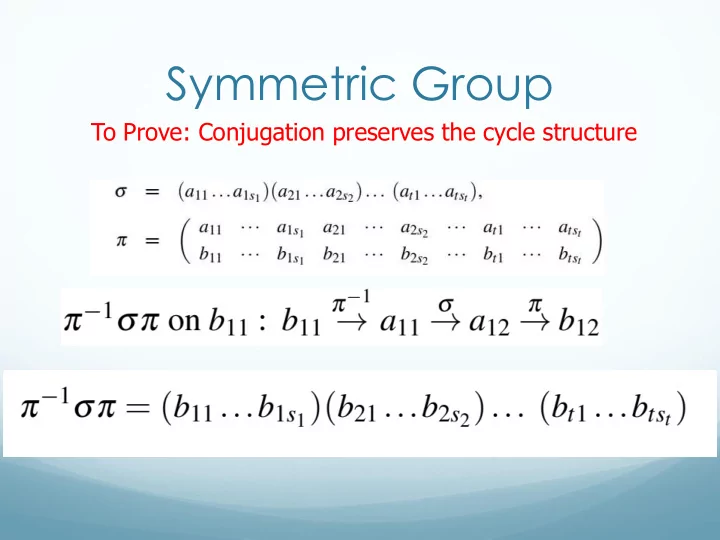
Symmetric Group To Prove: Conjugation preserves the cycle structure - PowerPoint PPT Presentation

Symmetric Group To Prove: Conjugation preserves the cycle structure

Cayley Theorem proof  For a finite group G={g 1 = e, g 2, g 3, ….g n }  Define a map to symmetry Π g defined as  Show that Π g is isomorphic to G and hence G is a subgroup of the symmetry group of degree n.

Semi-direct products

Generators of alternating groups Show that the alternating group is generated by the set of all the three-cycles on n letters.

Bilateral axis  If the principal axis is in the plane of mirror symmetry, then it is called bilateral axis  Principal axis is bilateral if we have U 2 two-fold axis perpendicular to principal axis  Point group with the bilateral n-fold axis requires C k n to be conjugate to C n-k n  Using this, write the conjugate classes of Dihedral D n and C nv groups

Dihedral groups

Tetrahedral, cubic groups  Principal axis is bilateral if we have Try to write the group elements of the proper group symmetry of the cube called Octohedral symmetry and denoted as O  Similarly, proper group symmetries of the regular Tetrahedron is denoted as T  Then including improper reflection planes, we have O h, T d – try to write the conjugacy classes

Tetrahedral molecule C 2 Three C 2 C 3 Four 3-fold axes Pure Rotations give group T

a tetrahedral Structure has total 24 symmetry operations Including reflections σ is the mirror (reflection) plane S 4 is a rotation by 90 ° followed by a six mirror reflection planes (6 σ) mirror reflection

Representation of What is reducible and irreducible representation ?

Examples  Write two-dimensional matrix representation for the group C 3v  Write 3-dimensional matrix representation for symmetric group of degree 3  What is the difference  Can we a matrix S such that the above 3-dimensional matrices can be brought to block diagonal form?

Reducible Representation  If we can find a S which diagonalises or block diagonalises n * n matrix representations of the group elements of the group  Is there such S ? That may become tedious if matrix size is large.  Further, for a given group how many non-trivial representations which cannot be further broken into diagonal or block diagonal form? Such representations are called irreducible representations (irreps).

Reducible and Irreducible Bring it to block-diagonal form if not diagonal Each block-diagonal component is called irreducible representation We discussed in class, (i) two-dimensional rep of C 3v (ii) another three-dimensional rep of C 3v Claim : 2d rep is irreducible but 3d rep is reducible.

Notations  Characters χ (trace of matrices)do not change under similarity transformations . Characters will be same for all the group elements within the same class .  For abelian groups, number of classes=h (order of G)  Aim is to find the number of irreducible representations Г α (g), their dimensions ℓ α for every group G and the characters χ α (g)  Г red (g)= ∑ a α Г α (g) where a α gives # of times irrep α appears in the reducible representation  Postulates: (i) Number of Г α (g) is equal to number of classes (p) ∑ ( ℓ α ) 2 = h (ii)

Great Orthogonality theorem number of elements in class i

Character Tables  Using the orthogonality properties of the great orthogonality theorem, let us work out the character table C 2v and for symmetric group of degree 3  Write character table for C 4 and C 4v

Mulliken Symbol Notation  One-dimensional irreps are denoted by A if character for C n is 1  One dimensional irreps are denoted by B if character for C n is -1  Two dimensional irreps are denoted by E  Three dimensional irreps are denoted by F or T  We introduce subscript g(gerade) or u(ungerade) depending on σ h character is +1 or -1 respectively

Character table

Symmetric group and irrep diagrams  Recall number of classes is equal to number of irreps. So, the same Young diagrams used for cycle structure can be used to denote irreps.  Symmetrizer – horizontal row of boxes-trivial representation is denoted by this diagram  Antisymmetrizer- vertical column of boxes-A 2 of S 3 is denoted by vertical column of 3 boxes  Other diagrams are called mixed representations.

Mixed representation diagrams  How do we determine the dimensions of any Young diagram. Symmetrizer and antisymmetrizer diagrams are 1-dimensional irreps. To find dimensions of mixed rep of ς (n) , use hook formula:  Count the number of boxes the hook for every box traverses. I have indicated two hooks- one traversing 1box and the other traversing 4 boxes. Do for all boxes. Dimension of irrep diagram is n! / hook number

Resolve reducible into irreps  For reducible rep : Г red (g)= ∑ a α Г α (g) where a α gives # of times irrep α appears in the reducible representation

Irreps in reducible representation Using the orthogonality property, a α for reducible representation Г red is Typically, a system with symmetry group G when acted by an external perturbation will no longer have G as symmetry. In fact, it will be subgroup K C G. Then the irreps of G will be reducible representation for K

Regular representation-definition  Take the multiplication table of as rows and columns of matrix representation  Write the 4 * 4 matrix elements using where δ (e)= 1 and zero otherwise Are they diagonal matrices? Are they reducible representation?  Write the characters of the regular representations (trace of the matrix Г ) denoted as χ reg (g) and obtain a α  Please work this out and we will discuss in the next class

Hamiltonian and symmetry  For system with symmetry group G, Hamiltonian H commutes with the elements of G : [H,g]=0  Implies, Ψ(x) and g Ψ(x) will have same energy eigenvalue  If Ψ(x) is a non -degenerate eigenfunction of H, then g Ψ(x) = c Ψ(x)  If Ψ(x) is one of the degenerate eigenfunction of H, then g Ψ(x)= linear combination of the set of degenerate eigenfunctions

Character table and degeneracy  Character table gives the characters and dimensions of the irreducible representations α’s  Г α (g) irrep for group G will act on ℓ α dimensional basis states ξ i where i takes 1,2 , …. ℓ α .  Г α (g) ξ i = will be linear combinations of ξ i ’s  Both Г α (g) ξ i and ξ i have same energy if H is invariant under the group symmetry G- hence dimensionality ℓ α > 1 of irreps indicate degenerate eigenfunctions  Relook at particle in a 2-d square box and its group symmetry which allows 2-fold degenerate wavefunctions

Character table and basis states g z= (+1) z is eigenvalue eqn with eigenvalue +1 for all g which are characters of irrep A 1. Similarly acting on axial vectors and x,y coordinates will shown the eigenvalues matching with the other irreps as we discussed in class.