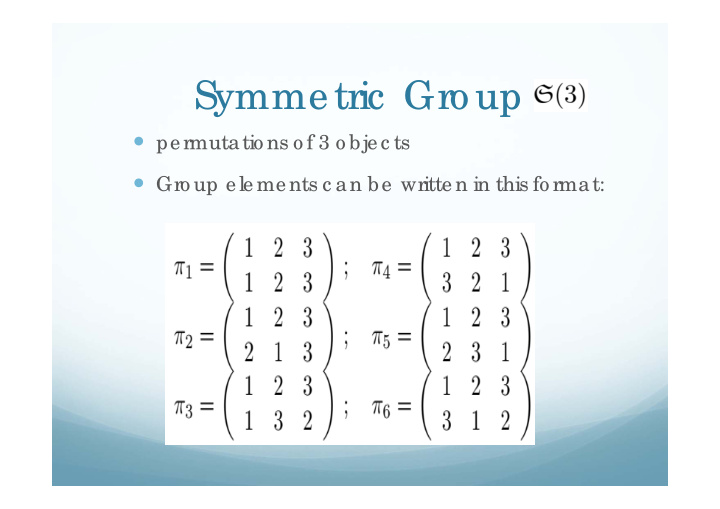
Symmetric Gro up pe rmutatio ns o f 3 o bje c ts Gro up elements c - PowerPoint PPT Presentation

Symmetric Gro up  pe rmutatio ns o f 3 o bje c ts  Gro up elements c an be written in this fo rmat:

Symmetric Gro up No te

Symmetric group  Product operation  Is the group elements {e,a,b,ab,b,b 2 } isomorphic to the above permutation elements?

Symmetric group  Order of , which is a symmetric group involving permutation of n objects, is n!  is called symmetric group of degree n  Subgroups of are called permutation groups  Cayley’s theorem states that every finite group is isomorphic to a permutation group embedded inside  Any permutation element can be equivalently represented as a product of disjoint permutation cycles

Symmetric group  Consider the following permutation element  This can be written in the following disjoint cycle structure  Cycle decomposition is useful for multiplication of two permutation elements

Symmetric Gro up Two-cycle is called transposition . Inverse of the transposition is the same element. Inverse of 3-cycle (123) is (132). Why? Every n-cycle can be written as product of transpositions

Symmetric Gro up Note that the product of the two permutation elements have six-cycle structure. Of course the elements are different.

Symmetric Gro up Any k-cycle can be broken into products of transpositions (2-cycle) Depending on the odd or even number of transpositions, permutation element is called odd or even permutation

Symmetric group  Any permutation element will have where where k runs from 1 to n such that  All permutation elements with the above cycle structure can be shown to be conjugate elements ( prove)  Total number of permutation elements( within the conjugacy class given by the cycle structure) is

Symmetric group  The number of conjugacy classes in the symmetric group is equal to the number of ways of partitioning integer n  For example, n=5 can be broken into 7 distinct conjugacy classes  Convenient way of diagrammatically representing the conjugacy classes using Young diagrams  1-cycles by single box, 2-cycle by double vertical box and so on  Identity element for n=5 is five 1-cycles denoted by

Symmetric group  Product of two 2-cycles and one 1-cycle will be represented by  One 5-cycle will be

Symmetric group  Set of even permutation elements form a group known as alternating group  Conjugate elements of even permutation elements will always be even which implies  is an invariant or normal subgroup  Factor group  Show that there are only two cosets possible or the factor group has only two elements [e, (1,2)]

Direct Product groups  For two groups, direct product group is  Example  Note that the elements of both the groups commute and order of G is product of order of the two groups

Semi-Direct product groups  Let K be invariant subgroup of G and T be another subgroup of G such that identity element is the only common element between K and T  Then, G is the semi-direct product group denoted by  Show that T are coset elements  Example

Symmetry of a molecule  Rotations and reflections which leaves the molecule invariant  Axis of rotational symmetry  Plane of symmetry- two types  Plane perpendicular to axis (horizontal mirror plane)-  Plane containing the axis (vertical mirror plane)-  Roto-reflection symmetry-  There could be diagonal plane of symmetry (cube)-

Improper symmetry operations Mirror planes =>  h => mirror plane perpendicular to a principal axis of rotation  v => mirror plane containing principal axis of rotation  d => mirror plane bisects dihedral angle made by the principal axis of rotation and two adjacent C2 axes perpendicular to principal rotation axis

Rotoreflection Improper axis of rotation => S n  rotation about n axis followed by reflection about the plane of symmetry (check it generates abelian group)

Point Groups  The set containing elementary operations plus various symmetry operations as a result of composing elementary operations forms a group called Point group .  At least one atom in the molecule is fixed under the symmetry operations- hence the name point group  Number of elements in the point group is finite  By Cayley’s theorem, point groups(symmetry of non- linear molecule) are isomorphic to subgroups of symmetry group

Schoenflies Notation

Water molecule Symmetry σ v ( xz ) C 2 Group symmetry is

Group symmetry? Ammonia Molecule

Methane symmetry? Group

Streographic projection

Streographic projection

Streographic projection

Streographic projection D2h D3 C3v C3

Symmetries of a cube

Embed tetrahedron in cube

Tetrahedral molecule C 2 Three C 2 C 3 Four 3-fold axes Pure Rotations give group T

a tetrahedral Structure has total 24 symmetry operations Including reflections σ is the mirror (reflection) plane S 4 is a rotation by 90 ° followed by a six mirror reflection planes (6 σ ) mirror reflection

Representation of