Searching Binary search (log n ) Better Can we achieve (1) - PDF document

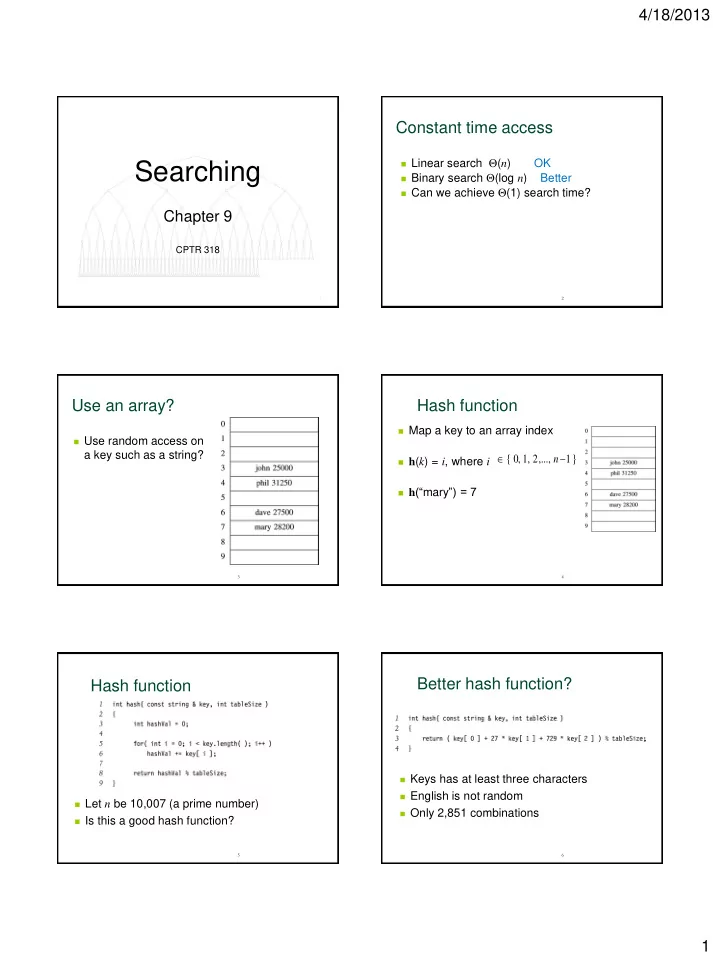
4/18/2013 Constant time access  Linear search Θ ( n ) OK Searching  Binary search Θ (log n ) Better  Can we achieve Θ (1) search time? Chapter 9 CPTR 318 1 2 Use an array? Hash function  Map a key to an array index  Use random access on a key such as a string?   { 0 , 1 , 2 ,..., n 1 }  h ( k ) = i , where i  h (“ mary ”) = 7 3 4 Better hash function? Hash function  Keys has at least three characters  English is not random  Let n be 10,007 (a prime number)  Only 2,851 combinations  Is this a good hash function? 5 6 1

4/18/2013 Better hash function Another Hash Function int hashpjw(char *s) { char *p; unsigned h = 0, g; for ( p = s; *p; p++ ) { h = (h << 4) + (*p); if ( g = h & 0xf0000000 ) { h ^= g >> 24; h ^= g; } } Aho, Sethi, and Ullman return h % PRIME; } 7 What about collisions? Perfect Hash Functions  What if two keys map to the same  Hash function is injective (1-1) location?  Possible only when values to store are fixed  Strategies: and known in advance  Open hashing (separate chaining)  To find a perfect hash function for n keys: Θ ( n )  Closed hashing (open addressing, or  Such a function can be evaluated in Θ (1) time probing)  Minimal perfect hash function  Linear probing  Range is n  Quadratic probing  Rehashing 10 Separate chaining Load factor  α (some authors use λ )  hash( x ) = x % 10  The ratio of the number of elements in the hash table to the table size  Average cost 1 + α /2 11 12 2

4/18/2013 Probing hash tables Disadvantages of separate chaining  Uses linked lists, so time to • Given a collision, find an empty cell via a dynamically allocate and collision resolution strategy deallocate nodes • Try cells h 0 ( x ), h 1 ( x ), h 2 ( x ),... until an empty  Requires implementing a cell is found second data structure (the • h i (x) = ( h ( x ) + f ( i )) % n linked list) • f (0) = 0  Space required to store the pointers • Load factor should be α < 0.5 14 Linear probing Linear probing  hash( x ) = x % 10  Add 89, 18, 49, 58, 69 • f is a linear function • Commonly, f ( i ) = i • Try sequential cells until an open cell is found 15 16 Problem with linear probing  Primary clustering  Number of probes  Insertion/unsuccessful search:   1 1    1    α 2   2 ( 1 )  Successful search:   1 1     1   α   2 ( 1 ) 17 3

4/18/2013 Quadratic probing Other strategies • f is a quadratic function  Double hashing • Commonly, f ( i ) = i 2  Apply second hash function • Eliminates the primary clustering problem  f ( i ) = i ∙ hash 2 ( x )  Rehashing  When the load factor gets high create a new table twice the size with new hash function  Rehash the existing values into the new table  Can be used with quadratic probing  Expensive to rehash, but amortized cost is low CPTR 314 20 How hard is it to … ? How hard is it to … ? Data Structure Find an List Find maximum Data Structure Find an List Find maximum element elements in (or minimum) element elements in (or minimum) order element order element Θ ( n ) Θ ( n 2 ) Θ ( n ) Θ ( n ) Θ ( n 2 ) Θ ( n ) Unordered list Unordered list Θ (log n ) Θ ( n ) Θ (log n ) Θ (log n ) Θ ( n ) Θ (log n ) Binary search tree Binary search tree Ordered list Θ (log n ) Θ ( n ) Θ ( 1 ) Ordered list Θ (log n ) Θ ( n ) Θ ( 1 ) Θ (1) Θ (?) Θ (?) Θ (1) Θ (?) Θ (?) Hash table Hash table How hard is it to … ? How hard is it to … ? Data Structure Find an List Find maximum Data Structure Find an List Find maximum element elements in (or minimum) element elements in (or minimum) order element order element Θ ( n ) Θ ( n 2 ) Θ ( n ) Θ ( n ) Θ ( n 2 ) Θ ( n ) Unordered list Unordered list Θ (log n ) Θ ( n ) Θ (log n ) Θ (log n ) Θ ( n ) Θ (log n ) Binary search tree Binary search tree Θ (log n ) Θ ( n ) Θ ( 1 ) Θ (log n ) Θ ( n ) Θ ( 1 ) Ordered list Ordered list Θ (1) Θ (?) Θ (?) Θ (1) Θ (?) Θ (?) Hash table Hash table 4

4/18/2013 How hard is it to … ? How hard is it to … ? Data Structure Find an List Find maximum Data Structure Find an List Find maximum element elements in (or minimum) element elements in (or minimum) order element order element Unordered list Θ ( n ) Θ ( n 2 ) Θ ( n ) Unordered list Θ ( n ) Θ ( n 2 ) Θ ( n ) Θ (log n ) Θ ( n ) Θ (log n ) Θ (log n ) Θ ( n ) Θ (log n ) Binary search tree Binary search tree Θ (log n ) Θ ( n ) Θ ( 1 ) Θ (log n ) Θ ( n ) Θ ( 1 ) Ordered list Ordered list Θ (1) Θ (?) Θ (?) Θ (1) Θ (?) Θ (?) Hash table Hash table How hard is it to … ? How hard is it to … ? Data Structure Find an List Find maximum Data Structure Find an List Find maximum element elements in (or minimum) element elements in (or minimum) order element order element Θ ( n ) Θ ( n 2 ) Θ ( n ) Θ ( n ) Θ ( n 2 ) Θ ( n ) Unordered list Unordered list Θ (log n ) Θ ( n ) Θ (log n ) Θ (log n ) Θ ( n ) Θ (log n ) Binary search tree Binary search tree Ordered list Θ (log n ) Θ ( n ) Θ ( 1 ) Ordered list Θ (log n ) Θ ( n ) Θ ( 1 ) Θ (1) Θ (?) Θ (?) Θ (1) Θ (?) Θ (?) Hash table Hash table How hard is it to … ? How hard is it to … ? Data Structure Find an List Find maximum Data Structure Find an List Find maximum element elements in (or minimum) element elements in (or minimum) order element order element Θ ( n ) Θ ( n 2 ) Θ ( n ) Θ ( n ) Θ ( n 2 ) Θ ( n ) Unordered list Unordered list Θ (log n ) Θ ( n ) Θ (log n ) Θ (log n ) Θ ( n ) Θ (log n ) Binary search tree Binary search tree Θ (log n ) Θ ( n ) Θ ( 1 ) Θ (log n ) Θ ( n ) Θ ( 1 ) Ordered list Ordered list Θ (1) Θ (?) Θ (?) Θ (1) Θ (?) Θ (?) Hash table Hash table 5

4/18/2013 How hard is it to … ? How hard is it to … ? Data Structure Find an List Find maximum Data Structure Find an List Find maximum element elements in (or minimum) element elements in (or minimum) order element order element Unordered list Θ ( n ) Θ ( n 2 ) Θ ( n ) Unordered list Θ ( n ) Θ ( n 2 ) Θ ( n ) Θ (log n ) Θ ( n ) Θ (log n ) Θ (log n ) Θ ( n ) Θ (log n ) Binary search tree Binary search tree Θ (log n ) Θ ( n ) Θ ( 1 ) Θ (log n ) Θ ( n ) Θ ( 1 ) Ordered list Ordered list Θ (1) Θ (?) Θ (?) Θ (1) Θ (?) Θ (?) Hash table Hash table How hard is it to … ? How hard is it to … ? Data Structure Find an List Find maximum Data Structure Find an List Find maximum element elements in (or minimum) element elements in (or minimum) order element order element Θ ( n ) Θ ( n 2 ) Θ ( n ) Θ ( n ) Θ ( n 2 ) Θ ( n ) Unordered list Unordered list Θ (log n ) Θ ( n ) Θ (log n ) Θ (log n ) Θ ( n ) Θ (log n ) Binary search tree Binary search tree Ordered list Θ (log n ) Θ ( n ) Θ ( 1 ) Ordered list Θ (log n ) Θ ( n ) Θ ( 1 ) Θ (1) Θ (?) Θ (?) Θ (1) Θ ( n 2 ) Θ ( n ) Hash table Hash table 6